by Tiwari Academy
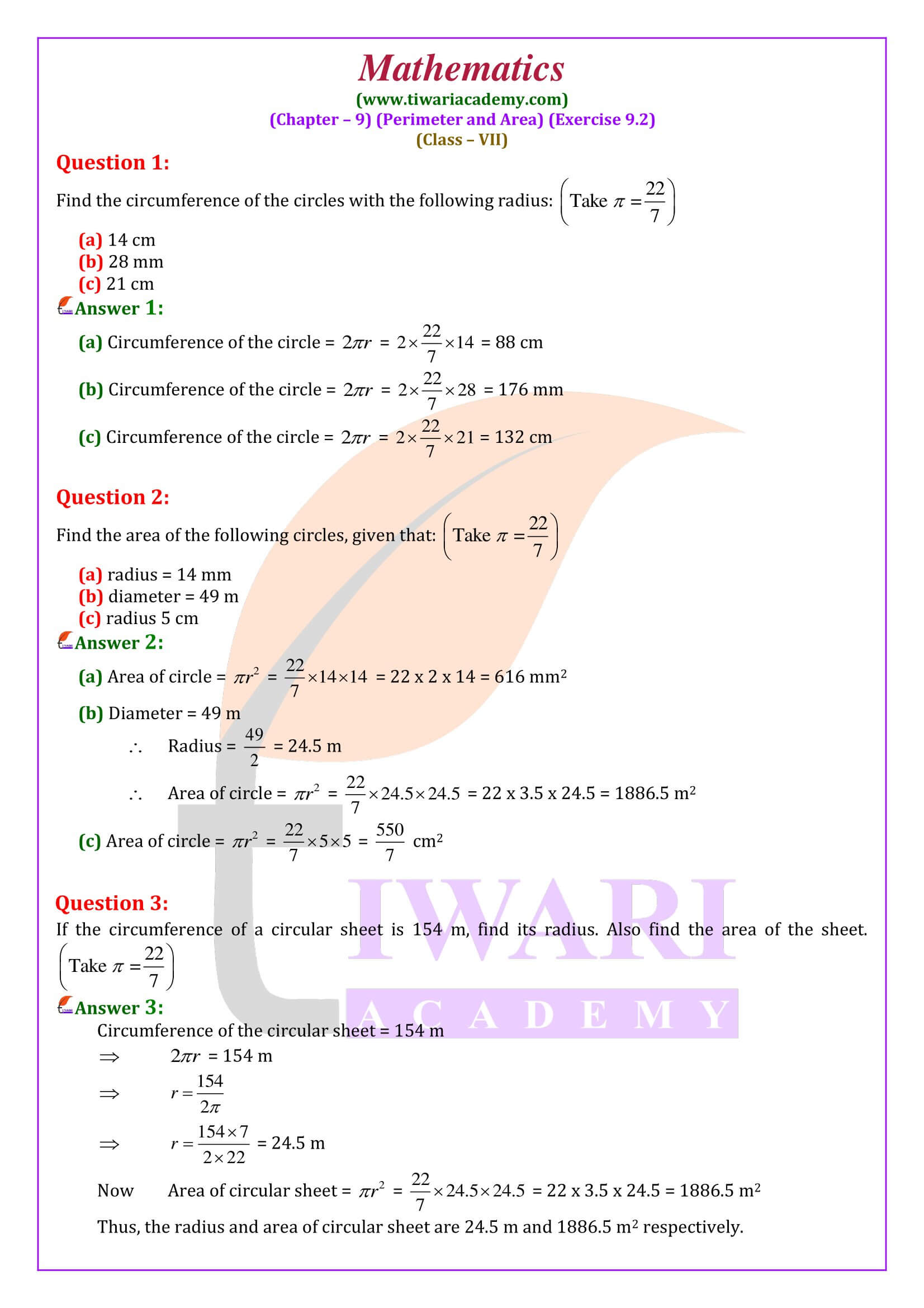
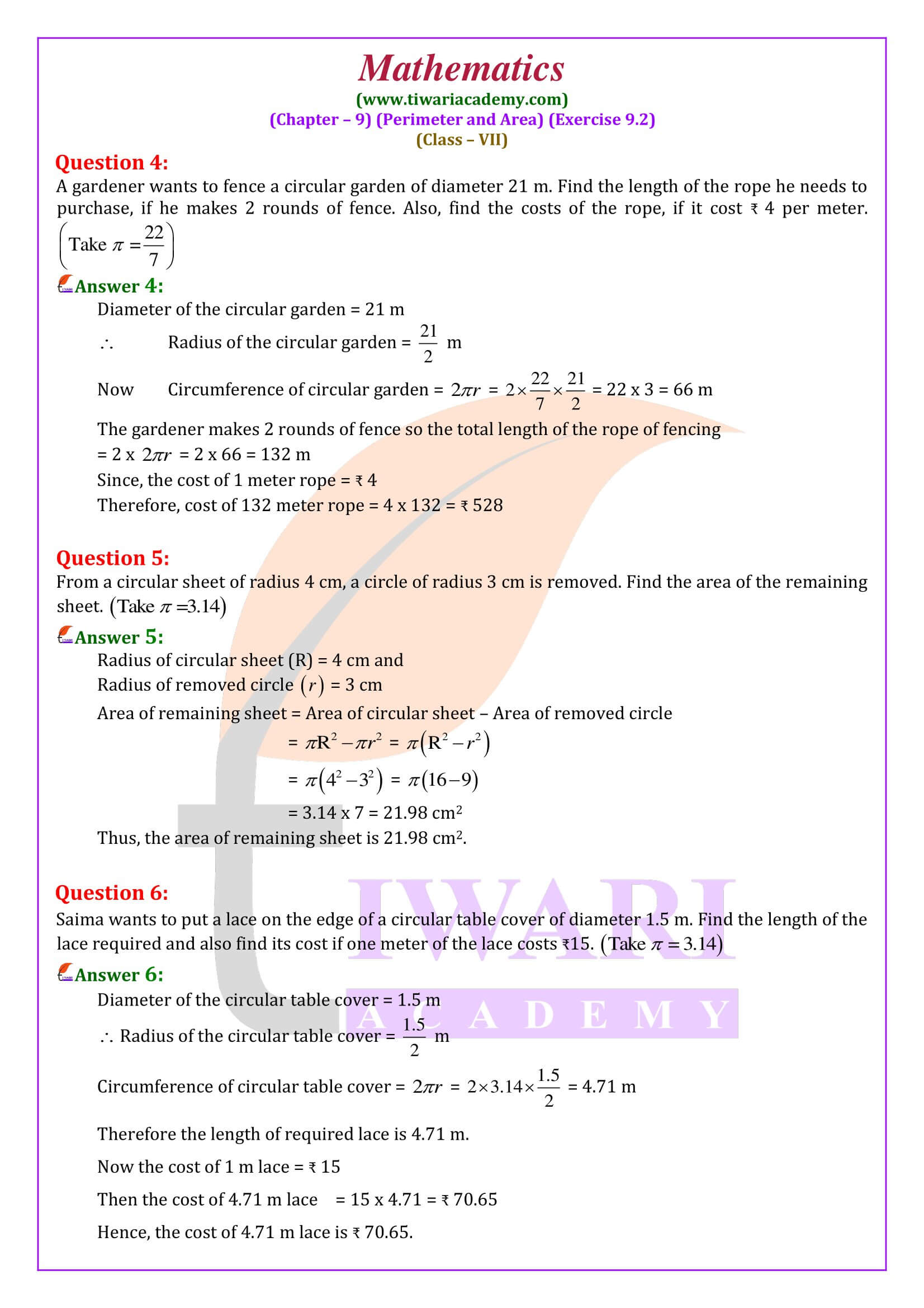
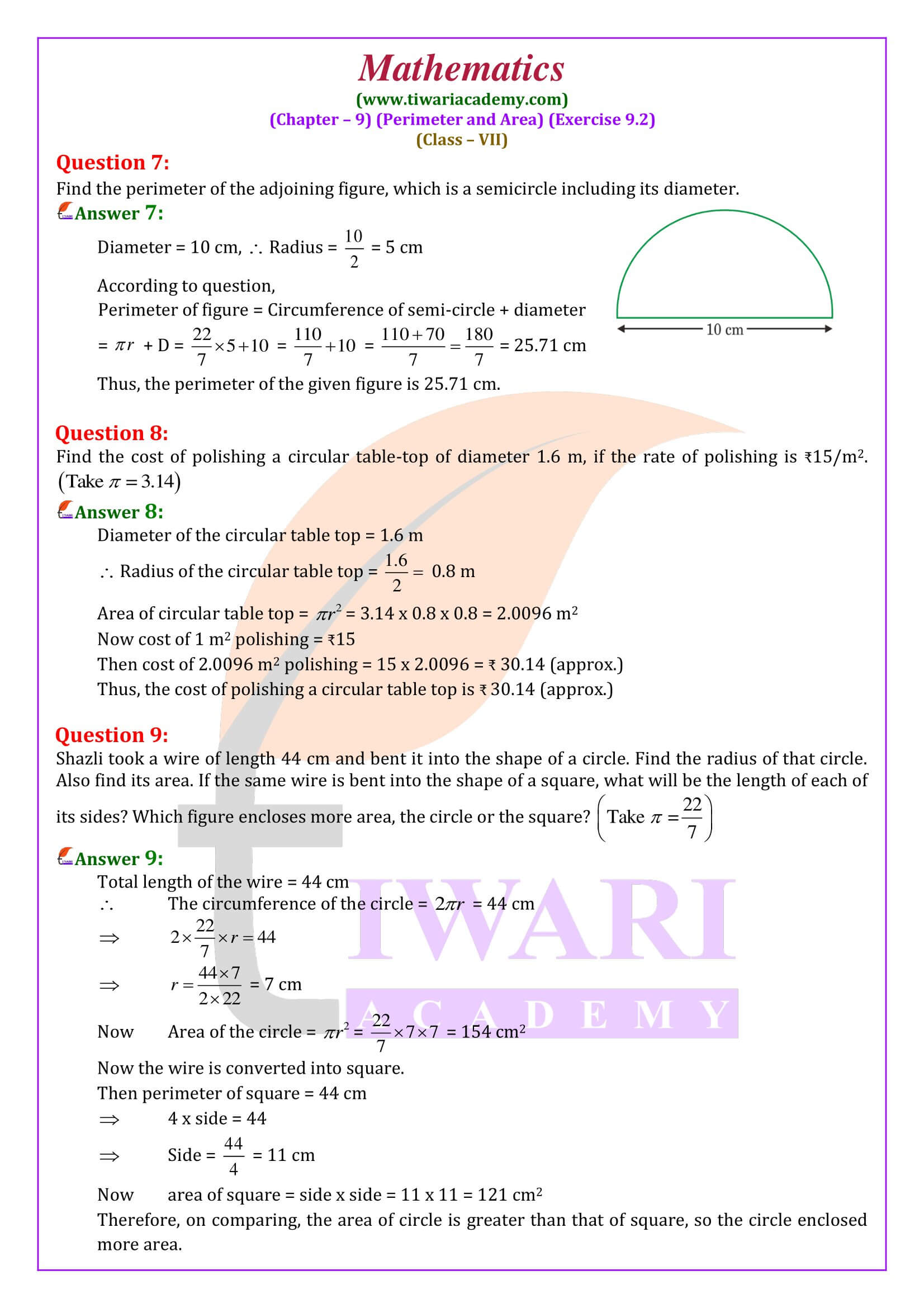
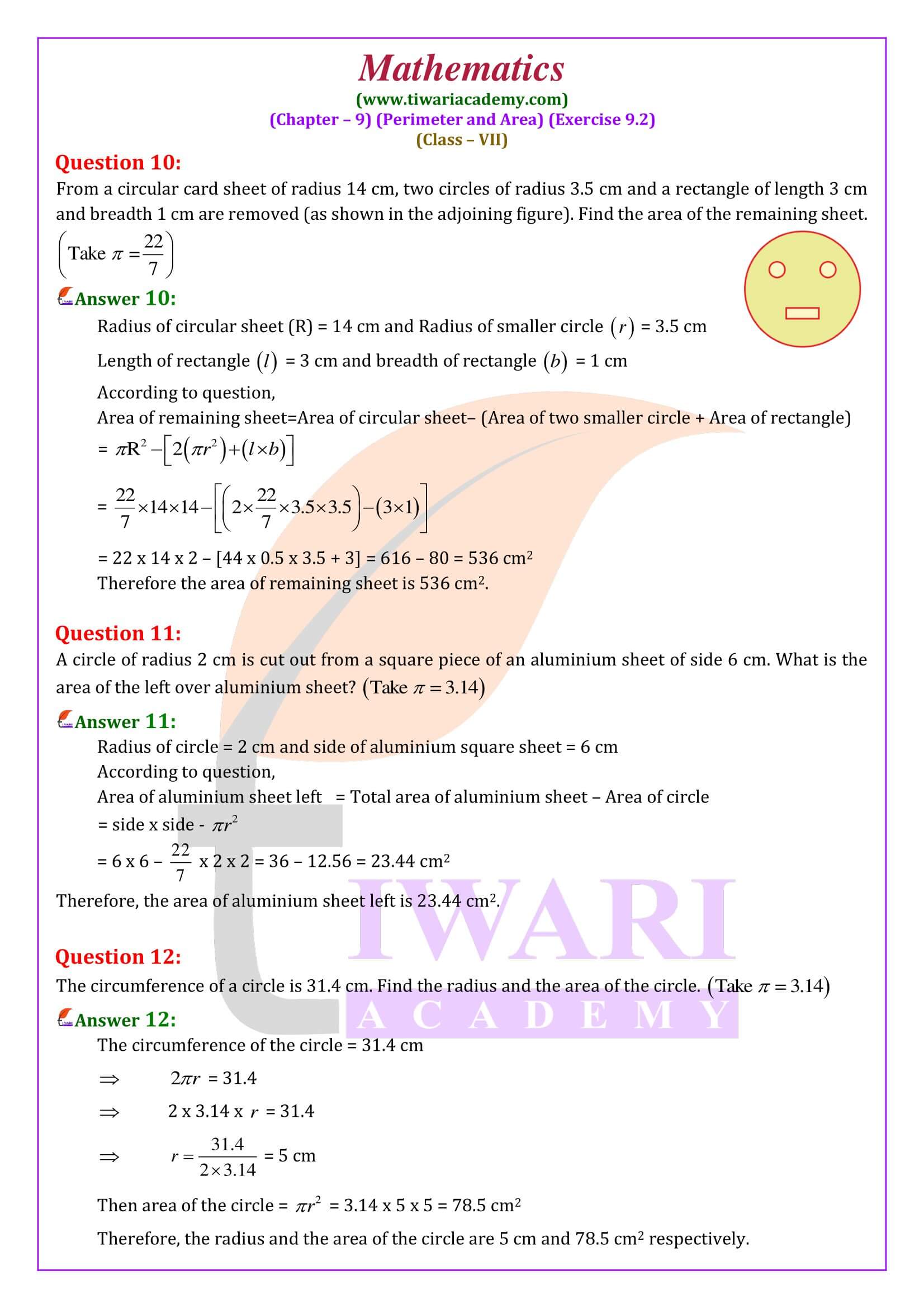
NCERT Solutions for Class 7 Maths Chapter 9 Exercise 9.2 Perimeter and Area in Hindi and English Medium for CBSE 2024-25. Get here the revised solutions of ex. 9.2 class 7th Maths based on new syllabus and latest NCERT textbook issued for academic session 2024-25.
NCERT Class 7 Maths Exercise 9.2 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 9 | Exercise: 9.2 |
| Topic Name: | Perimeter and Area |
| Content Mode: | Text, Images and Videos |
| Session: | CBSE 2024-25 |
| Medium: | English and Hindi Medium |
Class 7 Maths Chapter 9 Exercise 9.2 Solution
All the solutions are updated according to latest NCERT Books issued for academic session 2024-25. There are total of 17 questions given in 7th math exercise 9.2 which is based on the concepts of circumference and area of circles. Some questions are tricky but most of the questions are easy to solve.
Offline App for Class 7 Maths
Class 7 Maths Chapter 9 Exercise 9.2 Solution in Videos
Class 7 Maths App Hindi Medium
Class 7 Maths Exercise 9.2 Extra Questions
One side of a parallelogram is 14 cm. Its distance from the opposite side is 16.5 cm. Find the area of parallelogram.
Here, base = 14 cm and height = 16.5 cm.
So, area of the parallelogram = base x height
= (14 x 16.5) cm2 = 231 cm²
Find the area of a rhombus. the lengths of whose diagonals are 36 cm and 22.5 cm.
Area of the rhombus = ½ x (product of diagonals)
= ½ x 36 x 22.5 cm² = 405 cm²
Hence, the area of the rhombus is 405 cm²
Areas of Parallelogram and Rhombus
FORMULAE:
(i) Area of a parallelogram = (base x height) sq units.
PROOF: Let ABCD be a parallelogram. Join BD.
Then, BD divides the parallelogram into equal triangles.
Draw DE Ʇ AB
Area of parallelogram ABCD= 2 x (area of triangle ABC)
= 2 x (1/2 x AB x DE) sq units = (AB x DE) sq units
= (base ´ height) sq units.
Class 7 Maths Exercise 9.2 Important Questions
Find the area of a triangle in which base = 25 cm and height = 14 cm.
Here, base = 25 cm and height = 14 cm.
So, area of the triangle = ½ x base x height
= ½ x 25 x 14 cm² = 175 cm²
What is the relationship between a parallelogram and a triangle?
We see that each triangle takes up precisely one half of the parallelogram. From this, we see that the area of a triangle is one half the area of a parallelogram, or the area of a parallelogram is two times the area of a triangle.
Can a parallelogram have right angles?
In a parallelogram, if one of the angles is a right angle, all four angles must be right angles. If a four-sided figure has one right angle and at least one angle of a different measure, it is not a parallelogram; it is a trapezoid.
Area of a rhombus = 1/2 x (product of diagonals)
PROOF:
Let ABCD be a rhombus whose diagonals AC and BD intersect at a point O.
Let AC = d1 and BD = d2
We know that the diagonals of a rhombus bisect each other at right angles.
Now, in right triangle AOB, we have:
area (triangle AOB) = (1/2 x OA x OB) = ½ x d1 x d2)
so, area of rhombus ABCD = 4 x area (triangle AOB) = 4 x (1/8 x d1 x d2) = d1 x d2 sq units.
So, area fo a rhombus = ½ x product of diagonals sq units
Areas of Triangles
FORMULAE
(i) Consider a triangle in which the base and the corresponding height (or altitude) are given.
Then. area of the triangle = ½ x base x height sq units.
(ii) In a right triangle, the sides other than the hypotenuse are called its legs.
Then. area of a right triangle = (1/2 x product of legs) sq units.
(iii) Let each side of an equilateral triangle be a unit.
Then, area of the equilateral triangle = √(2/3) a2 sq units.
Heron’s formula
Let a, b, c be the sides of a triangle ABC. Then, s = ½ x (a + b + c).
A Greek mathematician, Heron, gave the formula:
Area of triangle ABC = √{s(s – a) (s – b) (s – c)} sq units)
