by Tiwari Academy
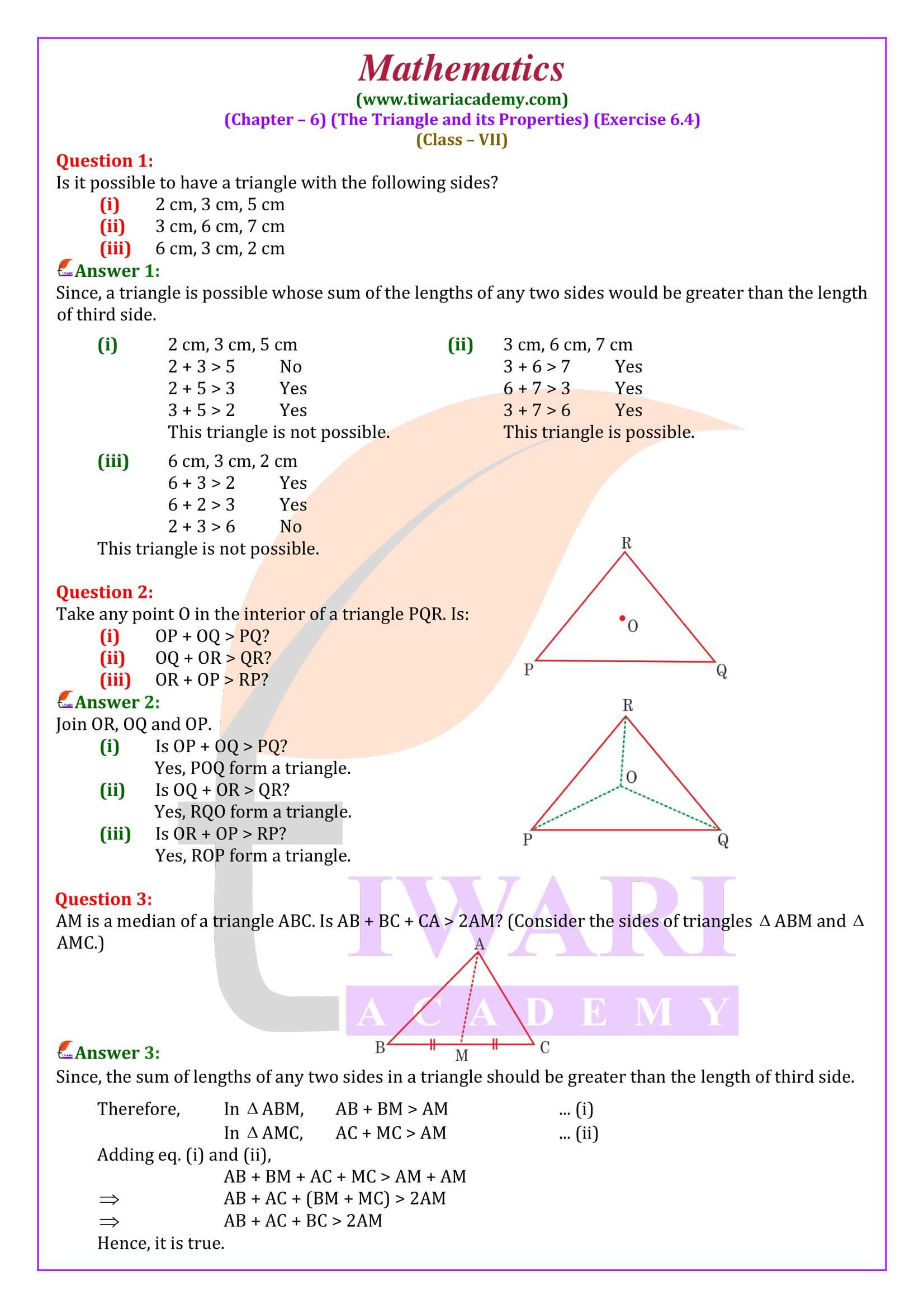
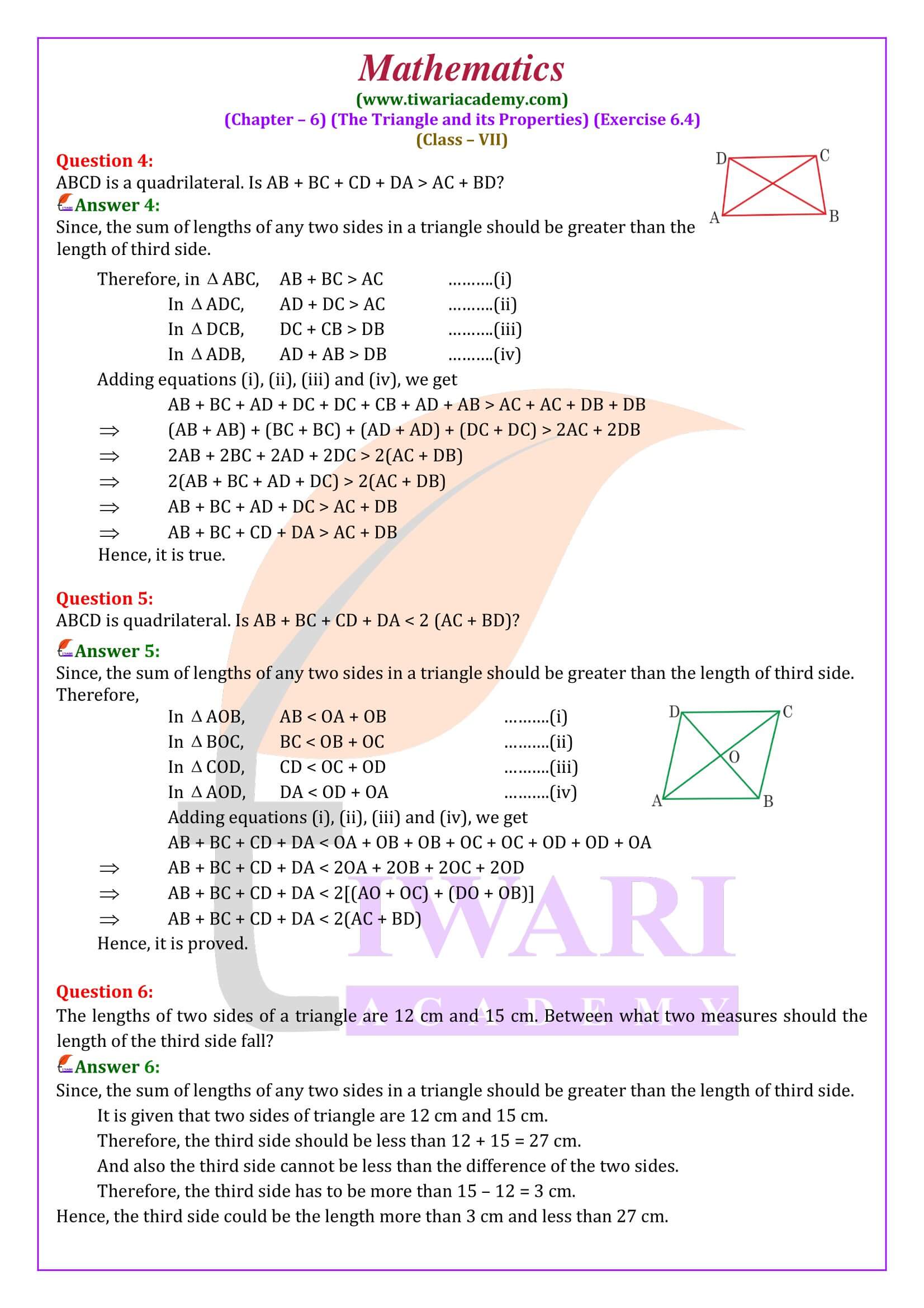
NCERT Solutions for Class 7 Maths Chapter 6 Exercise 6.4 Triangles and its Properties in Hindi and English Medium for CBSE Session 2024-25. According to new syllabus, the solution of ex. 6.4 class 7th mathematics are updated on the basis of NCERT books issued for academic year 2024-25.
NCERT Class 7 Maths Exercise 6.4 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 6 | Exercise: 6.4 |
| Topic: | Triangles and its Properties |
| Content Type: | PDF, Images and Videos Format |
| Session: | CBSE 2024-25 |
| Medium: | Hindi and English Medium |
Class 7 Maths Chapter 6 Exercise 6.4 Solution
All the solutions and study material are in updated format based on latest NCERT Books for 2024-25. Prepare here to solve your doubts and get confident in chapter 6 triangles using PDF and Videos solutions free without any login or password. All the contents on Tiwari Academy is free to use.
Free App for 7th Maths
Class 7 Maths Chapter 6 Exercise 6.4 Solution in Videos
Class 7 Maths App in Hindi
Class 7 Maths Exercise 6.4 Important Questions
How do you solve Pythagorean theorem questions?
Add the square of the two sides. If this sum is equal to the square of the hypotenuse, the triangle is right. If the two sides of the equation are not equal, the triangle is not right. Since the equation is not true, the triangle is not right.
How is Pythagoras theorem proved?
The greater significance of Pythagoras’ Theorem is that it holds for all right-angled triangles, large or small — all infinitely many of them. Mathematical reasoning is powerful enough to cut through finite bounds; a single argument can qualify Pythagoras’ formula as true for every conceivable right-angled triangle.
How do we use Pythagoras Theorem now a days?
The Pythagorean Theorem is useful for two-dimensional navigation. You can use it and two lengths to find the shortest distance. The distances north and west will be the two legs of the triangle, and the shortest line connecting them will be the diagonal. The same principles can be used for air navigation.
Show that triangle ABC, is a right angle triangle if a = 6 cm, b = 8 cm and c = 10 cm.
Let a = 6 cm, b = 8 cm and c = 10 cm.
Then, 62 + 82 = 36 + 64 = 100 = (10)
Or, a2 + b2 = c2.
Now, draw triangle ABC such that BC = 6 cm, CA =8 cm and AB =10 cm.
Measure ∠C. You will find that ∠C = 90°.
So, triangle ABC is right-angled at C.
Pythagoras
Pythagoras was an eminent Greek philosopher who was born in 580 BC and died in 500 BC. He gave a wonderful relation between the lengths of the sides of a right triangle, which is known as Pythagoras theorem.
Pythagoras’ Theorem
In a right angled triangle has one of its angles equals to 90°. The side opposite to the right angle is called the hypotenuse. The other two sides that contain right angle are called the base and perpendicular of the right angled triangle.
Thus, in a right triangle ABC in which ∠C = 90°,
we have: AB² = BC² + AC².
Thus, if AB = c, BC = a and AC = b,
we have: c² = a² + b²
In order to verify the above result, we perform the experiment given below.
(Hypotenuse)² = (Base)² + (Perpendicular)²
Thus, Pythagoras theorem states that, “In a right angled triangle, the square of the hypotenuse is equal to the sum of the squares of base and perpendicular.”
Remark: The two sides of a right triangle other than the hypotenuse are called its legs.
Converse of Pythagoras’ Theorem
If the square of one side of a triangle is equal to the sum of the squares of the other two sides, then the triangle is right-angled triangle.
Thus, in a triangle ABC, if AB² = BC² + CA², then the triangle is right-angled at C.
(i) Let a = 3 cm, b = 4 cm and c = 5 cm.
Then, 3² + 4² = 9 + 16 = 25 = 5²
a² + b² = c².
Now, draw triangle ABC such that BC = 3 cm, CA = 4 cm and AB = 5 cm.
Measure ∠C. You will find that ∠C = 90°.
So, triangle ABC is right-angled at C.
