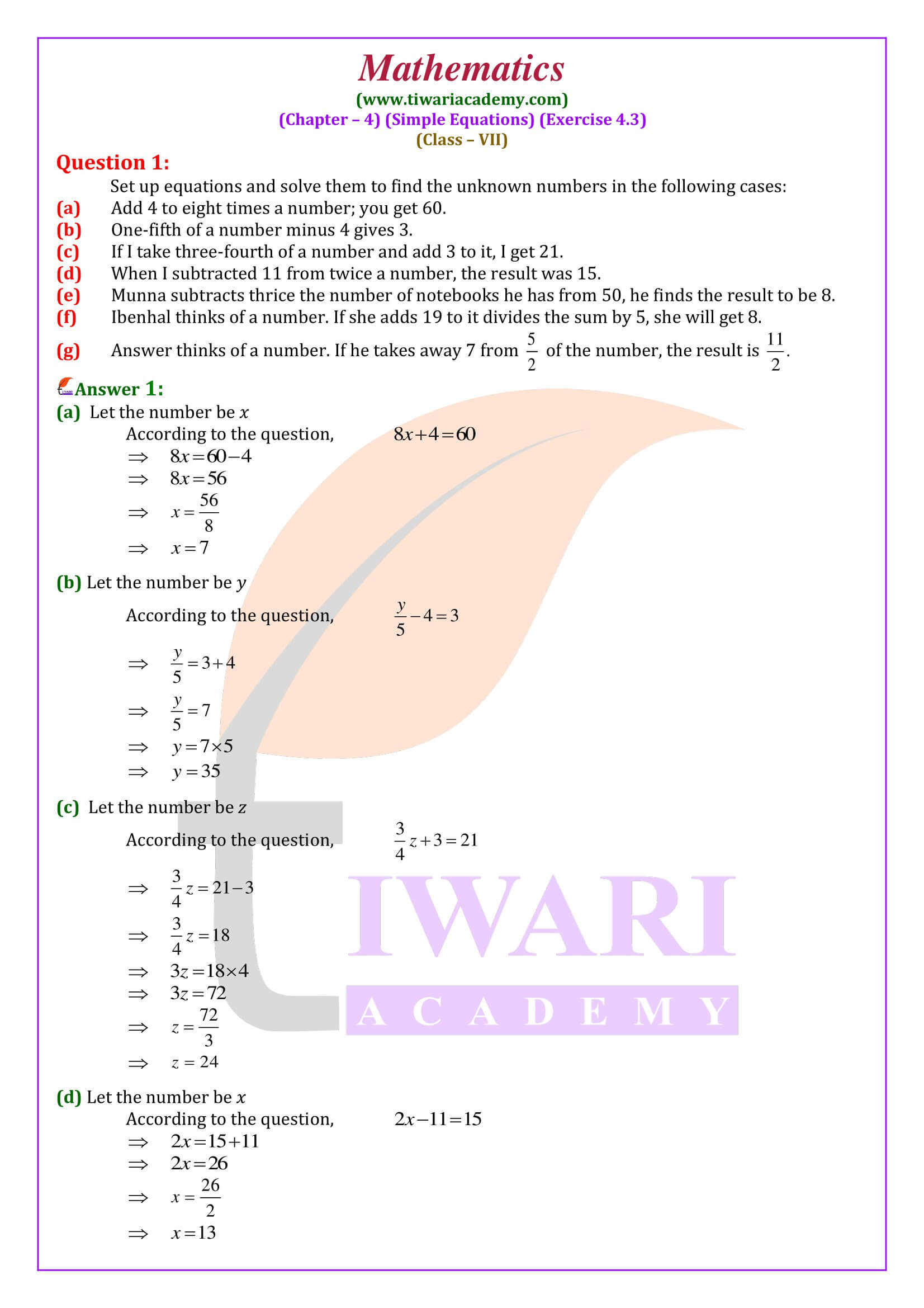
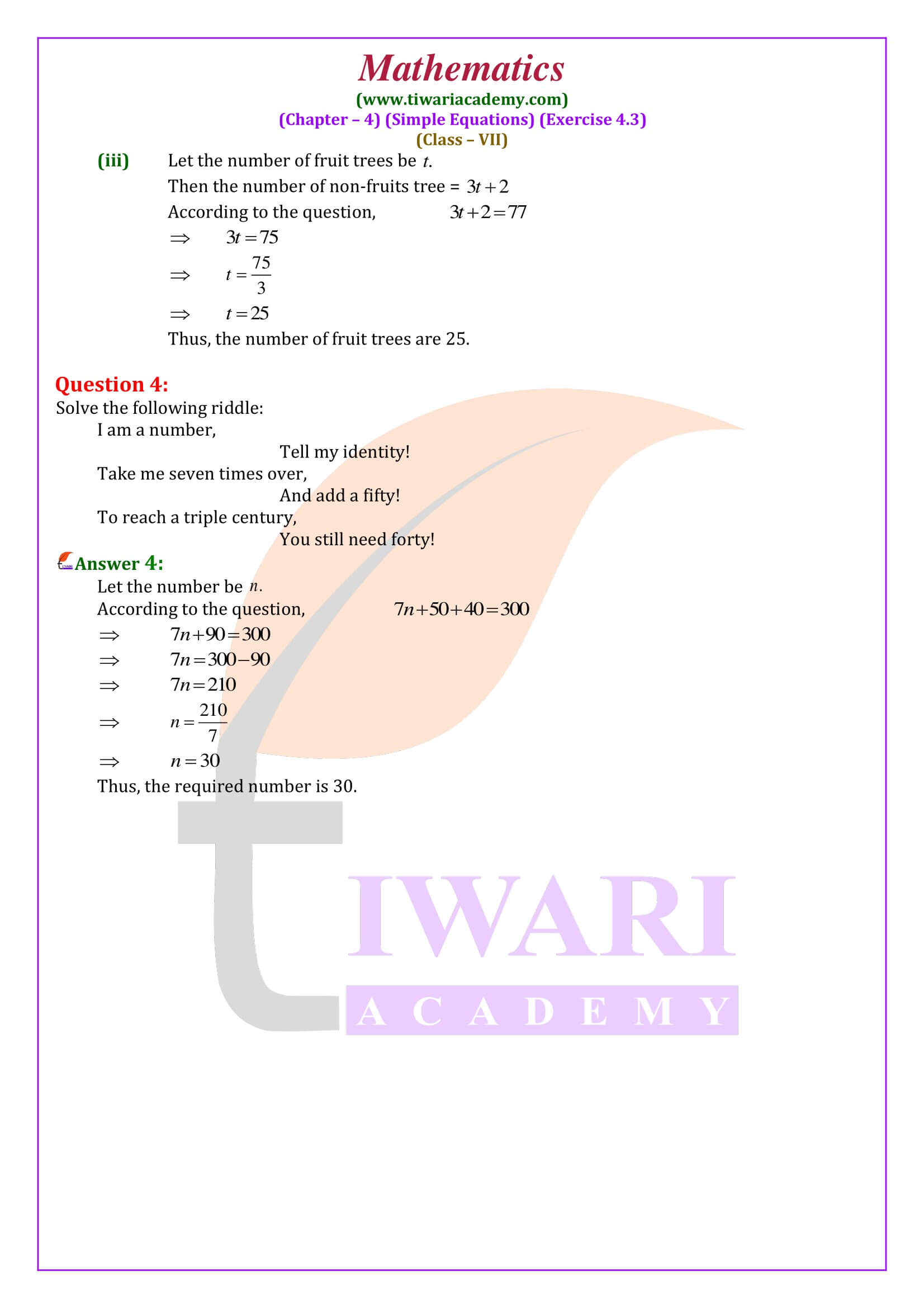
NCERT Solutions for Class 7 Maths Chapter 4 Exercise 4.3 Simple Equations in Hindi and English Medium updated for CBSE 2025-26. All the question answers and explanation of ex. 4.3 class 7th mathematics are revised according to new textbooks issued for academic year 2025-26.
NCERT Class 7 Maths Exercise 4.3 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 4 | Exercise: 4.3 |
| Topic Name: | Simple Equations |
| Content: | NCERT Textbook’s Solution |
| Academic Year: | CBSE 2025-26 |
| Medium: | Hindi and English medium |
Class 7 Maths Chapter 4 Exercise 4.3 Solution
Take help from videos solutions, if you have doubt in PDF file format solution. Class 7 math NCERT exercise 4.3 contains the practical problem based on daily life situation simple equation type questions. All the word problem based on simple equations are easy to understand but tricky to solve.
Class 7 Maths Chapter 4 Exercise 4.3 Solution in Videos
Applications of Simple Equations to Practical Situations
We have already seen examples in which we have taken statements in everyday language and converted them into simple equations. We also have learnt how to solve simple equations. Thus we are ready to solve puzzles/problems from practical situations. The method is first to form equations corresponding to such situations and then to solve those equations to give the solution to the puzzles/problems.
Example
The sum of three times a number and 11 is 32. Find the number.
Ket the be x, then three times the number is 3x and the sum of 3x and 11 is 32. That is, 3x + 11 = 32
To solve this equation, we transpose 11 to RHS, so that
3x = 32 – 11
Or, 3x = 21
Or, x = 21/3 = 7
The required number is 7. (We may check it by taking 3 times 7 and adding 11 to it. It gives 32 as required.)
Class 7 Maths Exercise 4.3 Important Questions
Raju’s father’s age is 5 years more than three times Raju’s age. Find Raju’s age, if his father is 44 years old.
Let Raju’s age is x years
Then, Raju’s father age = 3x + 5 = 44
To solve it, we get 3x = 44 – 5 = 39
Or, x = 39/3 = 13 years
That is, Raju’s age is 13 years.
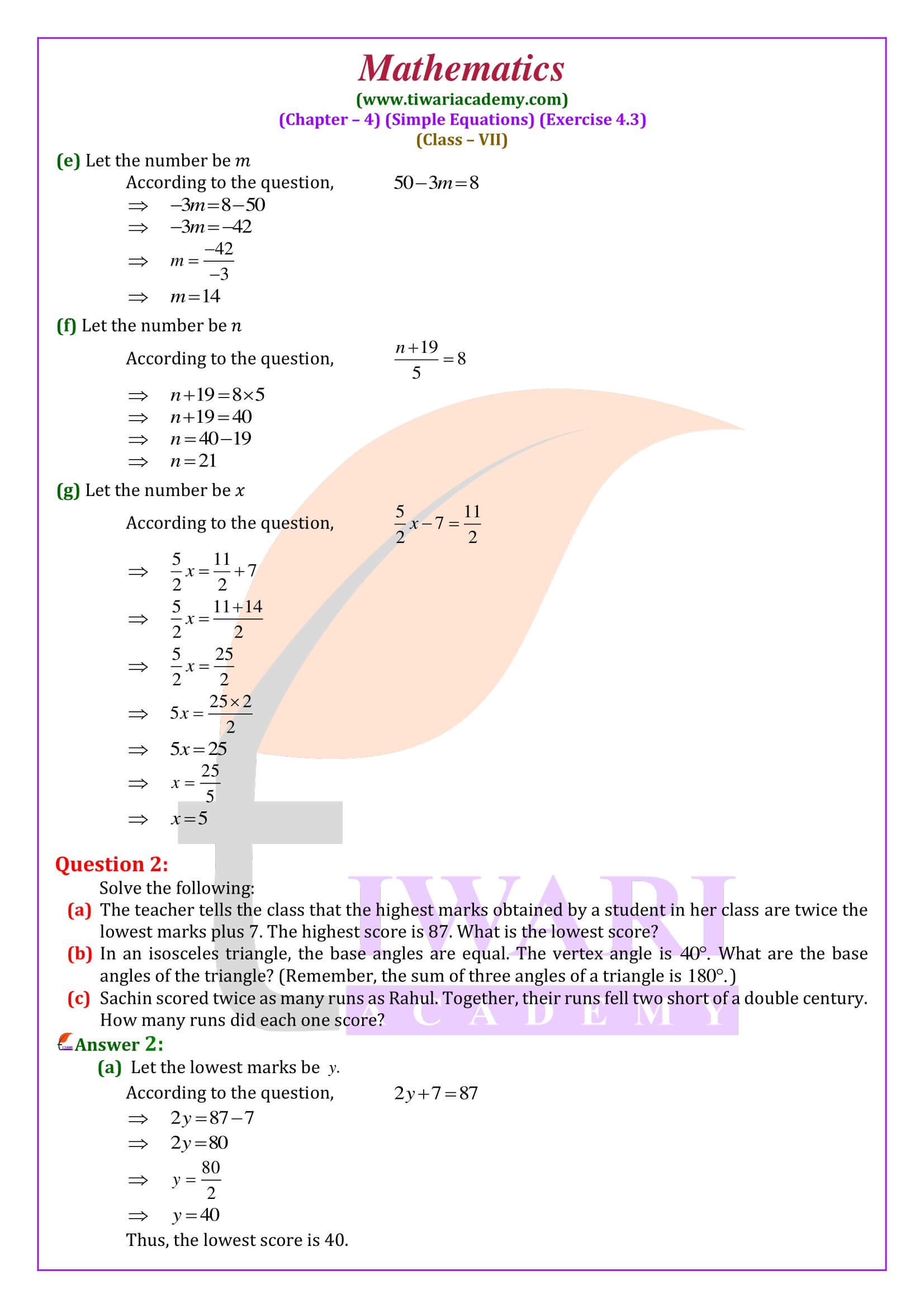
The teacher tells the class that the highest marks obtained by a student in her class is twice the lowest marks plus 7. The highest score is 87. What is the lowest score?
Let the lowest score is x
Then highest score = 2x + 7 = 87
Or, 2x = 87 – 7
Or, x = 80/2 = 40
Hence, the lowest score is 40
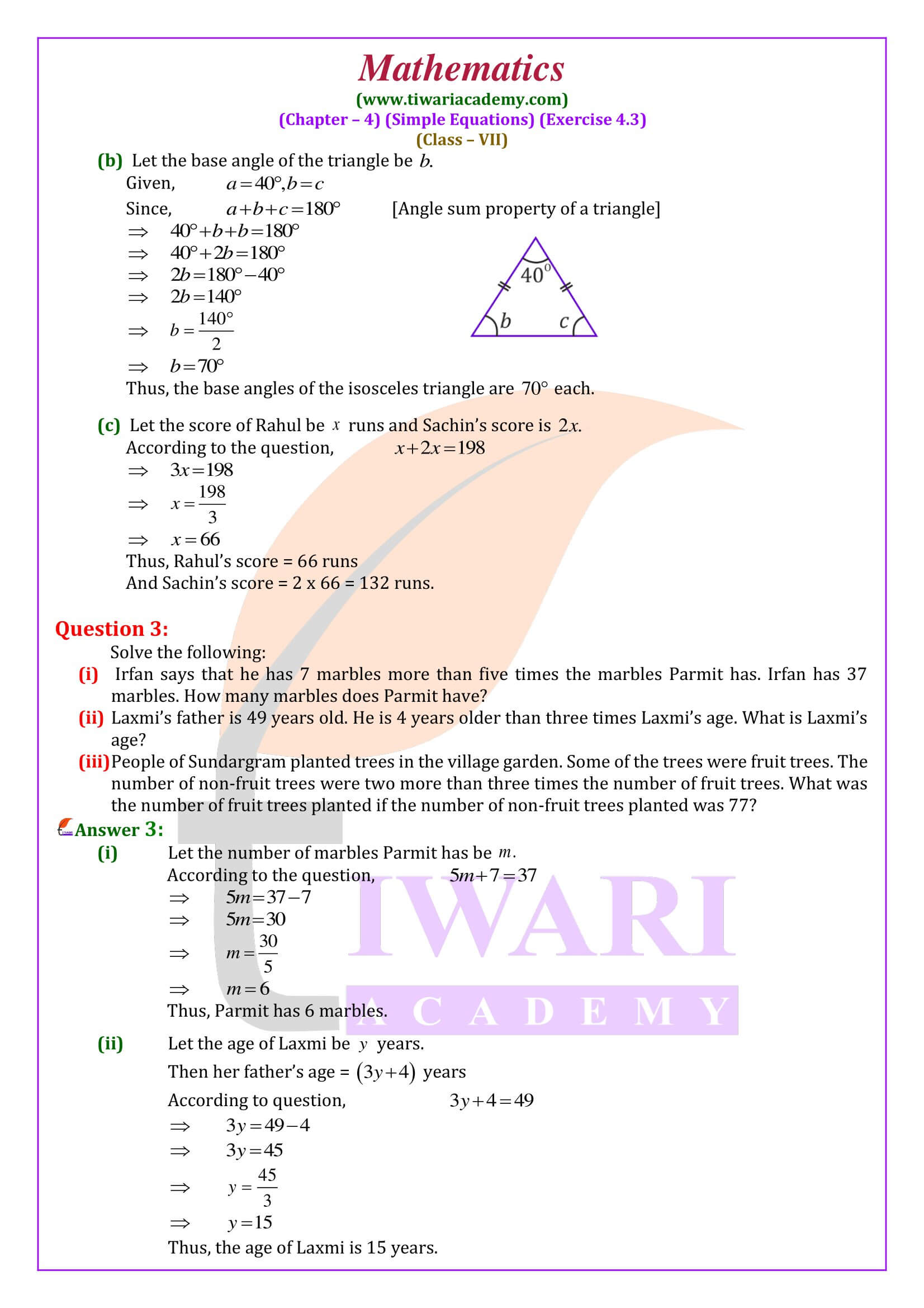
In an isosceles triangle, the base angles are equal. The vertex angle is 40°. What are the base angles of the triangle? (Remember, the sum of three angles of a triangle is 180°).
We know in triangle some of all three angles is 1800
According question both base angles are equal and one angle is 400
Let, the value of one base angle is x
Then, value of both angles = 2x
And sum of all three angles = 2x + 40 = 180
Or 2x = 180 – 40
X = 140/2 = 700
Hence, the value of one base angle is 700
Sachin scored twice as many runs as Rahul. Together, their runs fell two short of a double century. How many runs did each one score?
Let Rahul score x runs
Then, sachin’s score = 2x
And sum of both = x + 2x = 3x = 198
Or, x = 198/3 = 66
Hence, Rahul score 66 runs and Sachin score 132 runs.
Important Notes
1. An equation is a condition on a variable such that two expressions in the variable should have equal value.
2. The value of the variable for which the equation is satisfied is called the solution of the equation.
3. An equation remains the same if the LHS and the RHS are interchanged.
4. In case of the balanced equation, if we
(i) add the same number to both the sides, or
(ii) subtract the same number from both the sides, or
(iii) multiply both sides by the same number, or
(iv) divide both sides by the same number, the balance remains undisturbed, i.e., the value of the LHS remains equal to the value of the RHS
5. The above property gives a systematic method of solving an equation. We carry out a series of identical mathematical operations on the two sides of the equation in such a way that on one of the sides we get just the variable. The last step is the solution of the equation.
6. We also learnt how, using the technique of doing the same mathematical operation (for example adding the same number) on both sides, we could build an equation starting from its solution. Further, we also learnt that we could relate a given equation to some appropriate practical situation and build a practical word problem/puzzle from the equation.
Can students do exercise 4.3 (chapter 4 simple equations) of grade 7th Maths easily?
No, students can’t do exercise 4.3 (chapter 4 simple equations) of grade 7th Maths easily. All sums of exercise 4.3 are the word problems. Most of the students face issues while solving these word problems. Mainly students face issues in understanding the language of the word problems.
How many days, students need to finish exercise 4.3 of class 7th Maths?
To finish exercise 4.3 of class 7th Maths, students need at least 3 days if they give 1-2 hours per day to this exercise. This time can increase or decrease because this time also depends on the student’s ability and speed.
Which questions of exercise 4.3 of 7th standard Maths can students expect in the terminal exams?
Exercise 4.3 of 7th standard Maths has three examples (examples 8, 9, 10) and four questions. All questions and examples of this exercise are very important from the exam point of view. Any question and example of this exercise can come in the terminal exam. Students should solve the complete exercise.
Which problems of exercise 4.3 of grade 7th Maths are the best problems?
Exercise 4.3 of 7th standard Maths has three examples (examples 8, 9, 10) and four questions. Examples 9, 10, and questions 2 (b), 3 (i) (ii), 4 of exercise 4.3 of grade 7th Maths are the best problems.