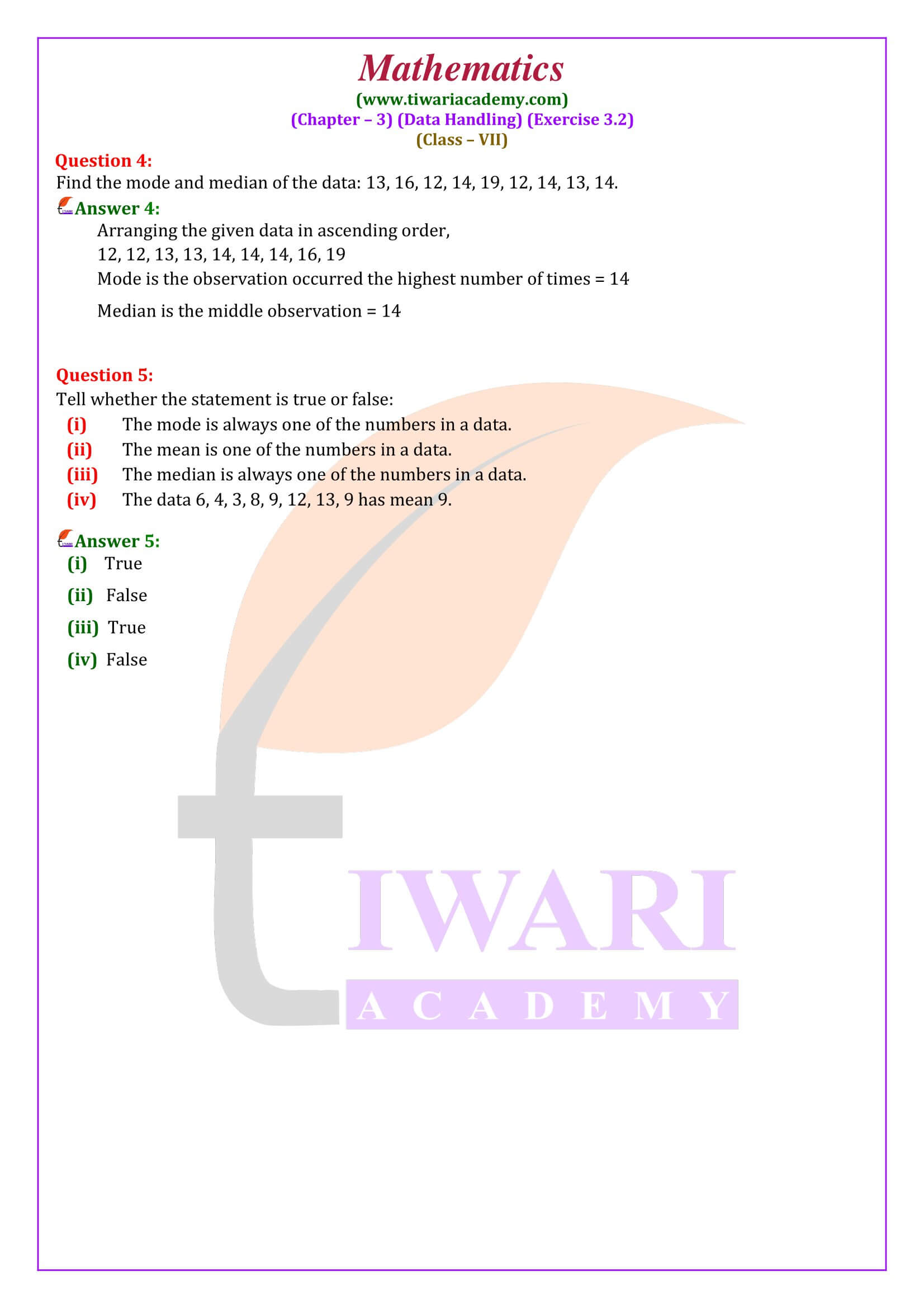
NCERT Solutions for Class 7 Maths Chapter 3 Exercise 3.2 Data Handling in Hindi and English Medium updated and modified for academic year 2025-26. The solutions of ex. 3.2 class 7th mathematics are revised according to new syllabus and textbooks issued for 2025-26 annual examination.
NCERT Class 7 Maths Exercise 3.2 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 3 | Exercise: 3.2 |
| Chapter Name: | Data Handling |
| Content: | Text PDF and Videos Solutions |
| Academic Session: | 2025-26 |
| Medium: | Dual Language – Hindi and English |
Class 7 Maths Chapter 3 Exercise 3.2 Solution
Exercises question answers are given in PDF as well as videos solution in easy and understandable format. Class 7 mathematics exercise 3.2 deals the questions related to Mode and Median. It also shows that how the mean, mode and median are related to each other.
Class 7 Maths Chapter 3 Exercise 3.2 Solution in Videos
Class 7 Maths Exercise 3.2 Extra Questions
The runs scored by 11 members of a cricket team are 25, 39, 53, 18, 65, 72, 0, 46, 31, 08, 34. Find the median score.
Arranging the number of runs in ascending order, we have:
0, 08, 18, 25, 31, 34, 39, 46, 53, 65, 72.
Here n = 11, which is odd.
So, median score = value of (11 + 1)th term = value of 6th term = 34.
Hence, the median score is 34.
Find the median for the following frequency distribution: xi = 3, 6, 10, 12, 7, 15 and fi = 3, 4, 2, 8, 13, 10
Arranging the terms in ascending order, we get:
xi = 3, 6, 7, 10, 12, 15
fi = 3, 4, 13, 2, 8, 10
Number of terms, N = 40.
median = ½ [size of (N/2)th item + size of + {(N +1)/2}th item]
Md. = ½ .{(value of 20th term) + (value of 21st term)}
Md. = ½ (7 + 10)
Hence, median = 8.5
Median of Ungrouped Data
After arranging the given data in ascending or descending order of magnitude, the value of the middle-most observation is called the median of the data.
Class 7 Maths Exercise 3.2 Important Questions
What is a real life example of median?
The median number in a group refers to the point where half the numbers are above the median and the other half are below it. You may hear about the median salary for a country or city. When the average income for a country is discussed, the median is most often used because it represents the middle of a group.
What can a median tell you?
The median provides a helpful measure of the centre of a data set. By comparing the median to the mean, you can get an idea of the distribution of a data set. When the mean and the median are the same, the data set is more or less evenly distributed from the lowest to highest values.
Why do we use median?
The mean value of numerical data is without a doubt the most commonly used statistical measure. Sometimes the median is used as an alternative to the mean. Just like the mean value, the median also represents the location of a set of numerical data by means of a single number.
Method for Finding the Median of an Ungrouped Data
Arrange the data in increasing or decreasing order of magnitude. Let the total number of observations be n.
Case 1: When n is odd:
Median = value of ½ x (n + 1)th observation.
Case 2: When n is even:
Median = ½ x {(n/2)th observation + (n/2 + 1)th observation}
Median of Discrete Series
First arrange the terms in ascending or descending order. Now, prepare a cumulative frequency table. Let the total frequency be N.
(i) If N is odd, then
median = size of {(N +1)/2}th item
(ii) If N is even, then
median = ½ [size of (N/2)th item + size of + {(N +1)/2}th item]
What is the core motive of exercise 3.2 of grade 7th Maths?
The core motive of exercise 3.2 of grade 7th Maths is to teach students the following things:
1. MODE (The mode of a set of observations is the observation that occurs most often.)
2. MEDIAN (Median refers to the value which lies in the middle of the data (when arranged in an increasing or decreasing order) with half of the observations above it and the other half below it).
Are there any examples in exercise 3.2 of class 7th Maths?
Yes, there are four examples (examples 4, 5, 6, 7) in exercise 3.2 of class 7th Maths. All examples are good and help students to solve similar exercise questions.
Which problems of exercise 3.2 of class 7th Maths teacher can give in the school exams?
Exercise 3.2 of class 7th Maths has four examples (examples 4, 5, 6, 7) and 5 questions. All problems of this exercise are good, interesting, and important. Teachers can give any problem of exercise 3.2 of class 7th Maths in the school exams.
How is exercise 3.2 of class 7th Maths, simple or complicated?
Exercise 3.2 of class 7th Maths is not at all complicated. This exercise is very simple. This exercise is also not lengthy. Students can easily and quickly solve all questions and examples of this exercise.