by Tiwari Academy
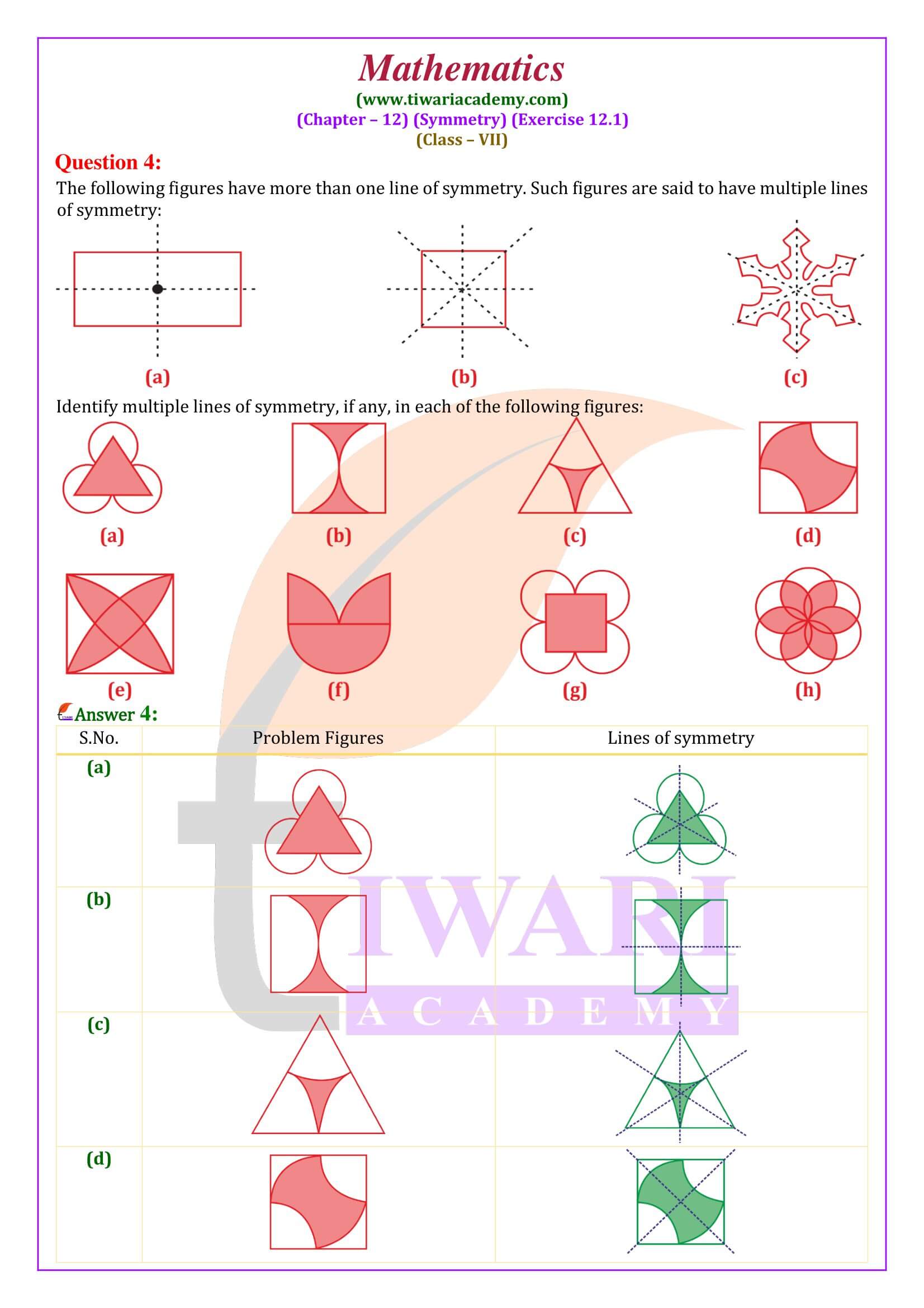
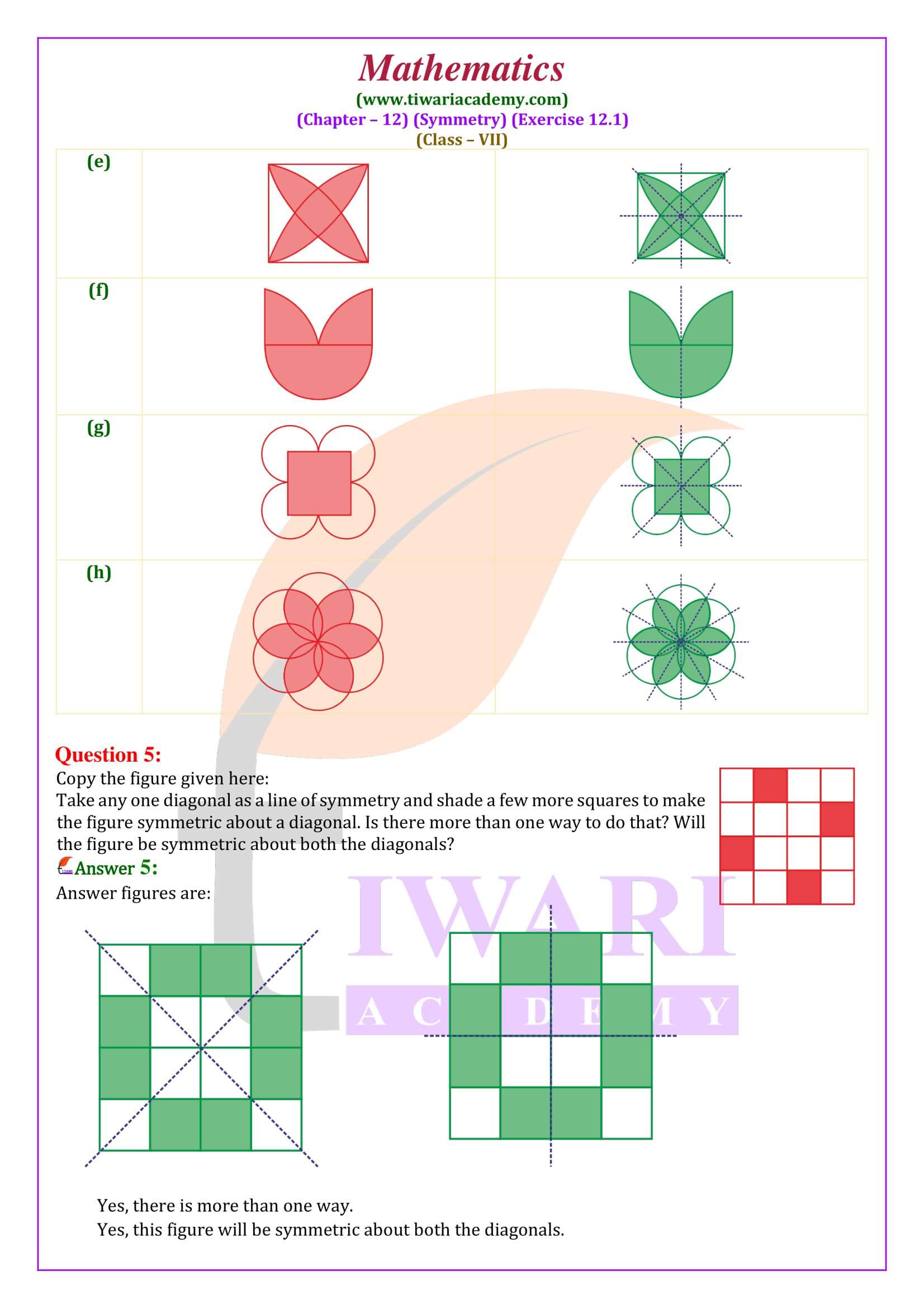
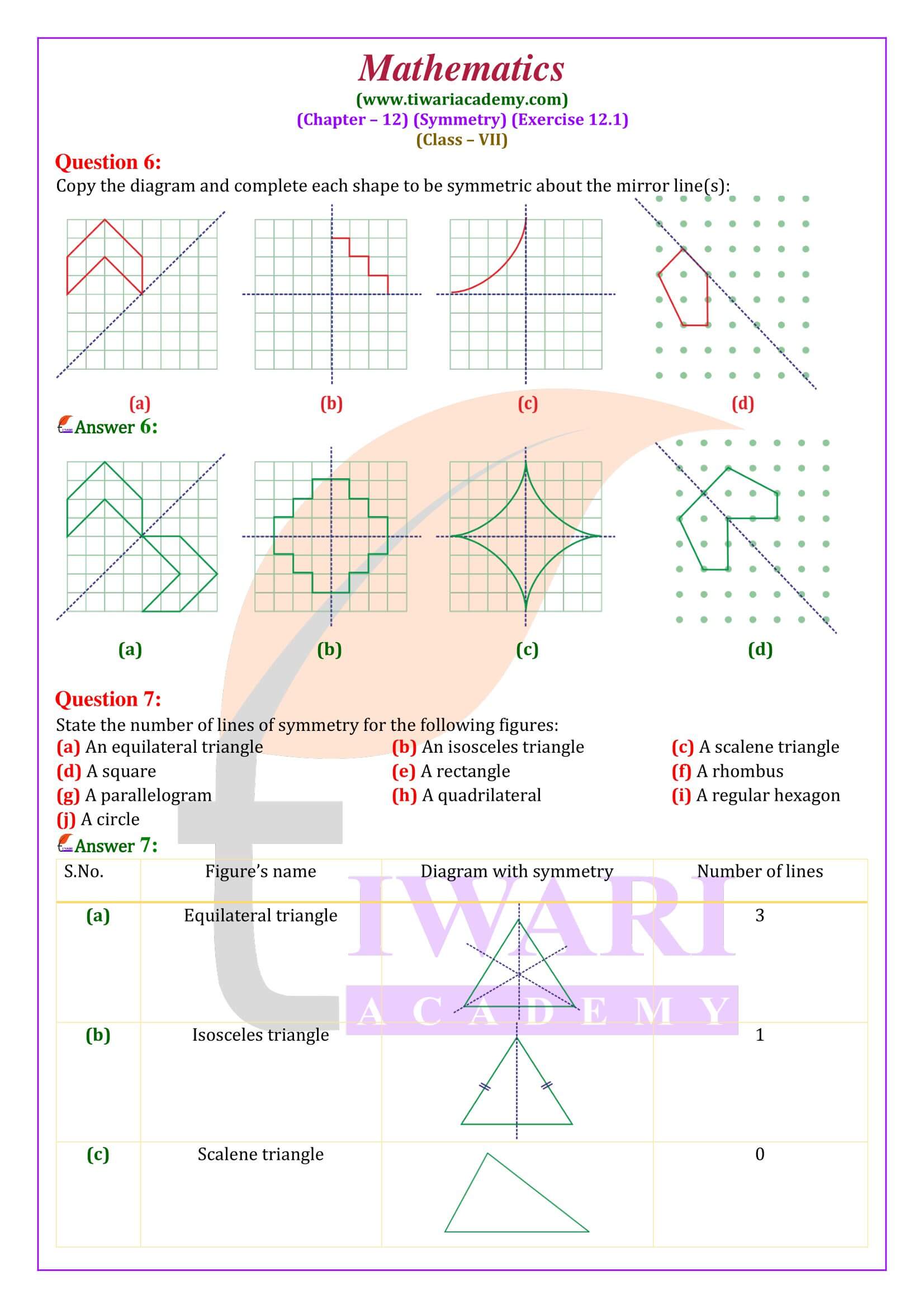
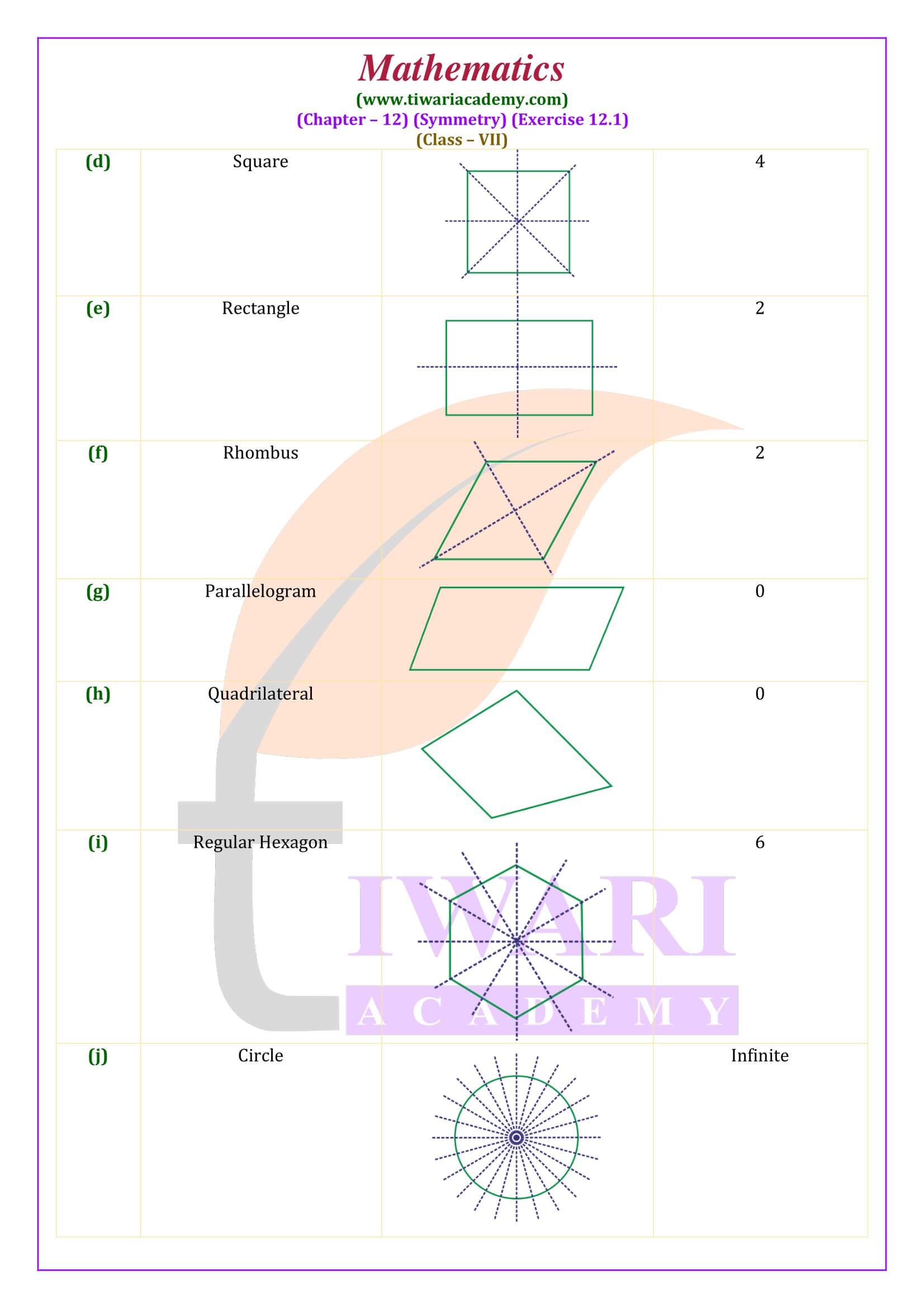
NCERT Solutions for Class 7 Maths Chapter 12 Exercise 12.1 Symmetry updated for academic session 2024-25 in Hindi and English Medium. Get the solutions of ex. 12.1 class VII mathematics in modified format based on revised syllabus and new textbooks issued for CBSE 2024-25 examination.
Class 7 Maths Exercise 12.1 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 12 | Exercise: 12.1 |
| Topic Name: | Symmetry |
| Content Type: | Images, PDF and Videos format |
| Academic Year: | CBSE 2024-25 |
| Medium: | Hindi and English Medium |
Class 7 Maths Chapter 12 Exercise 12.1 Solution
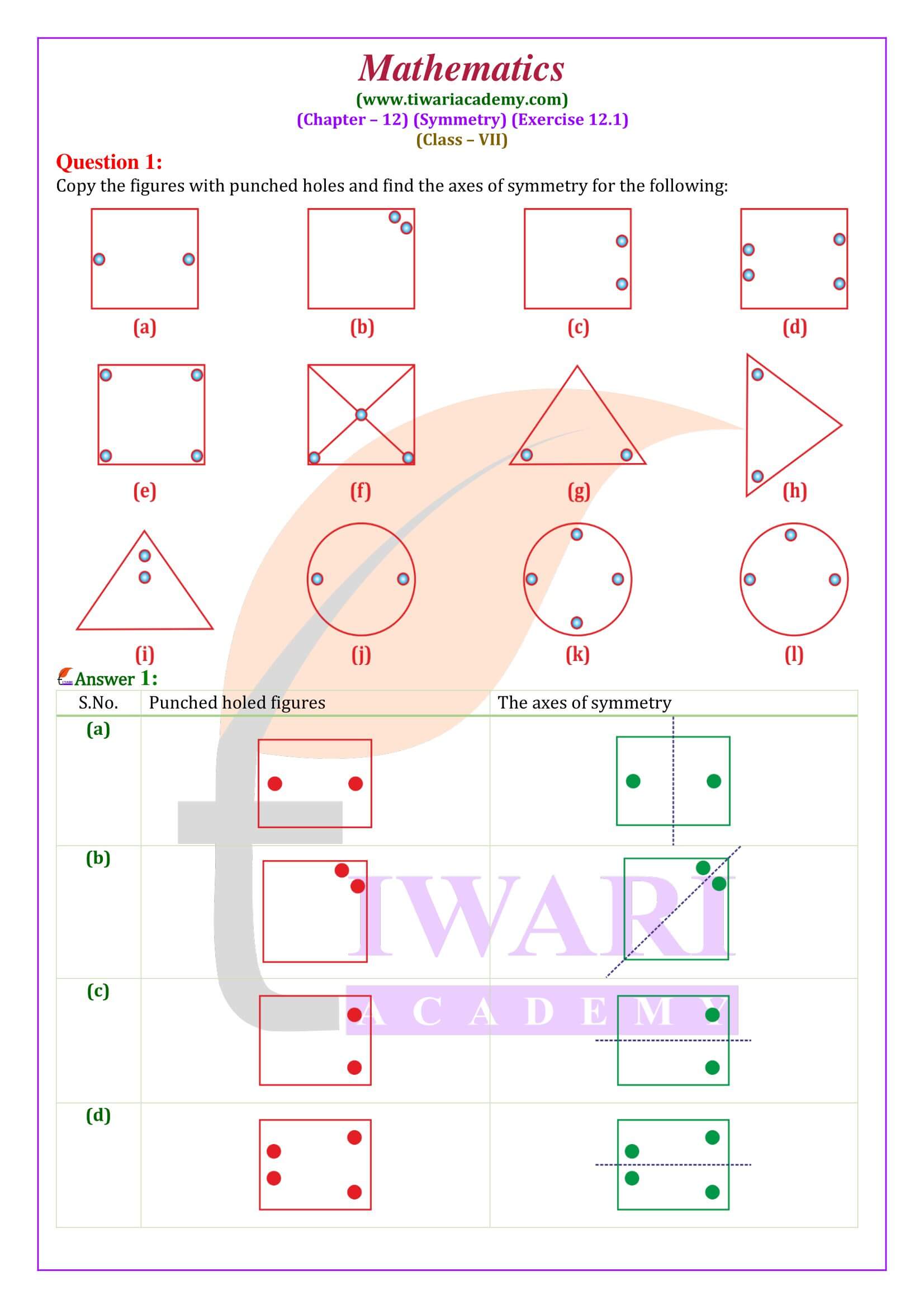
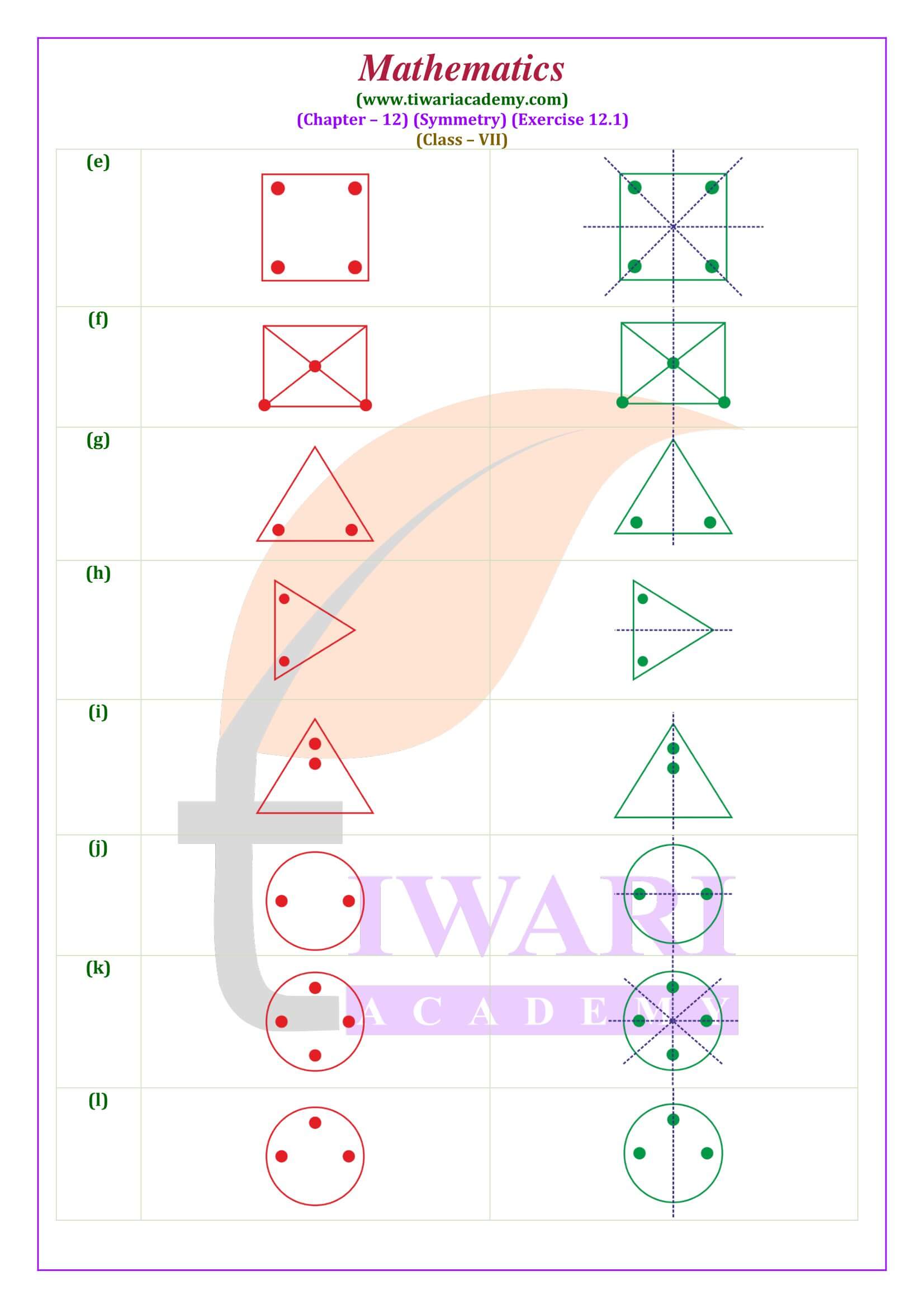
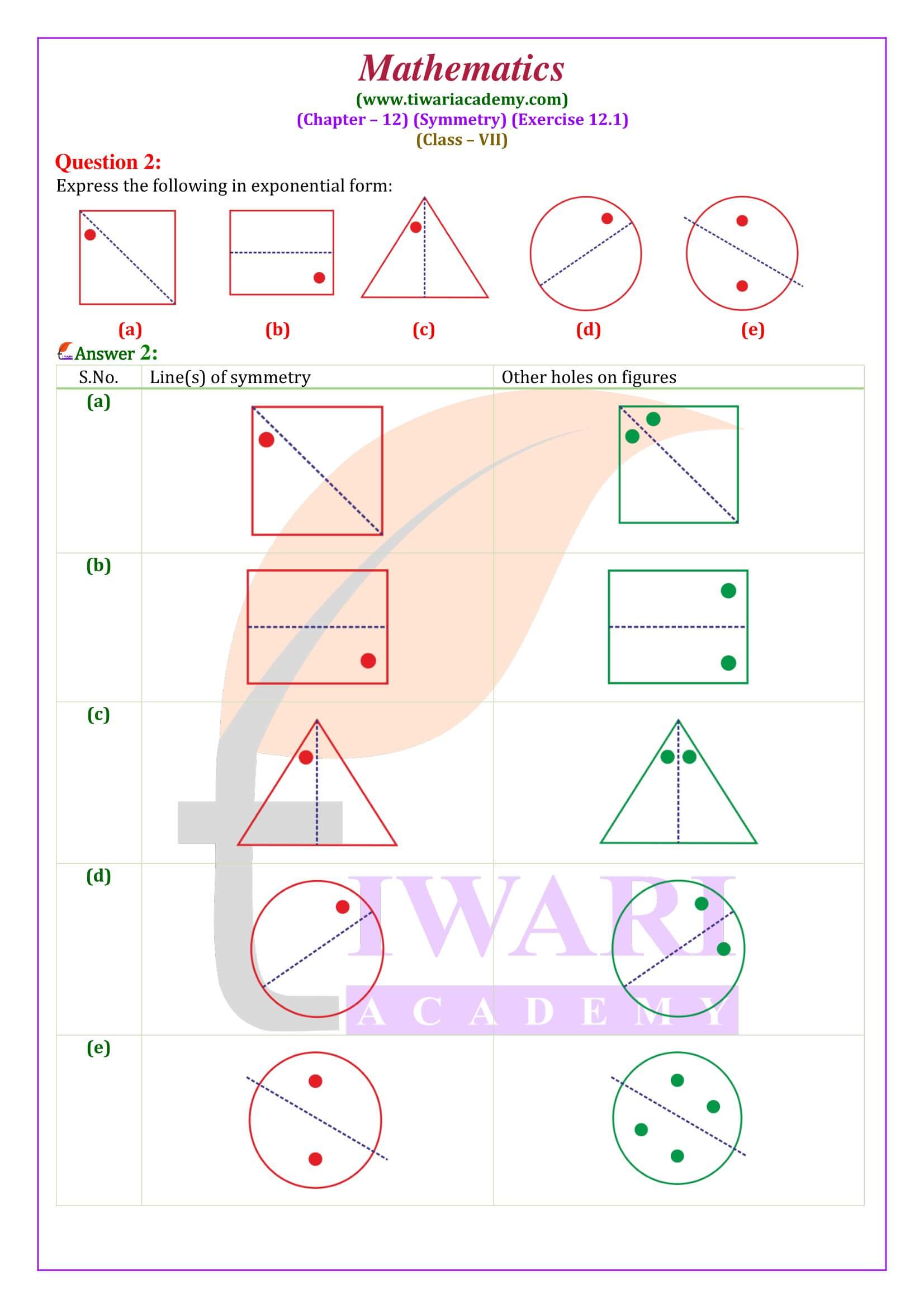
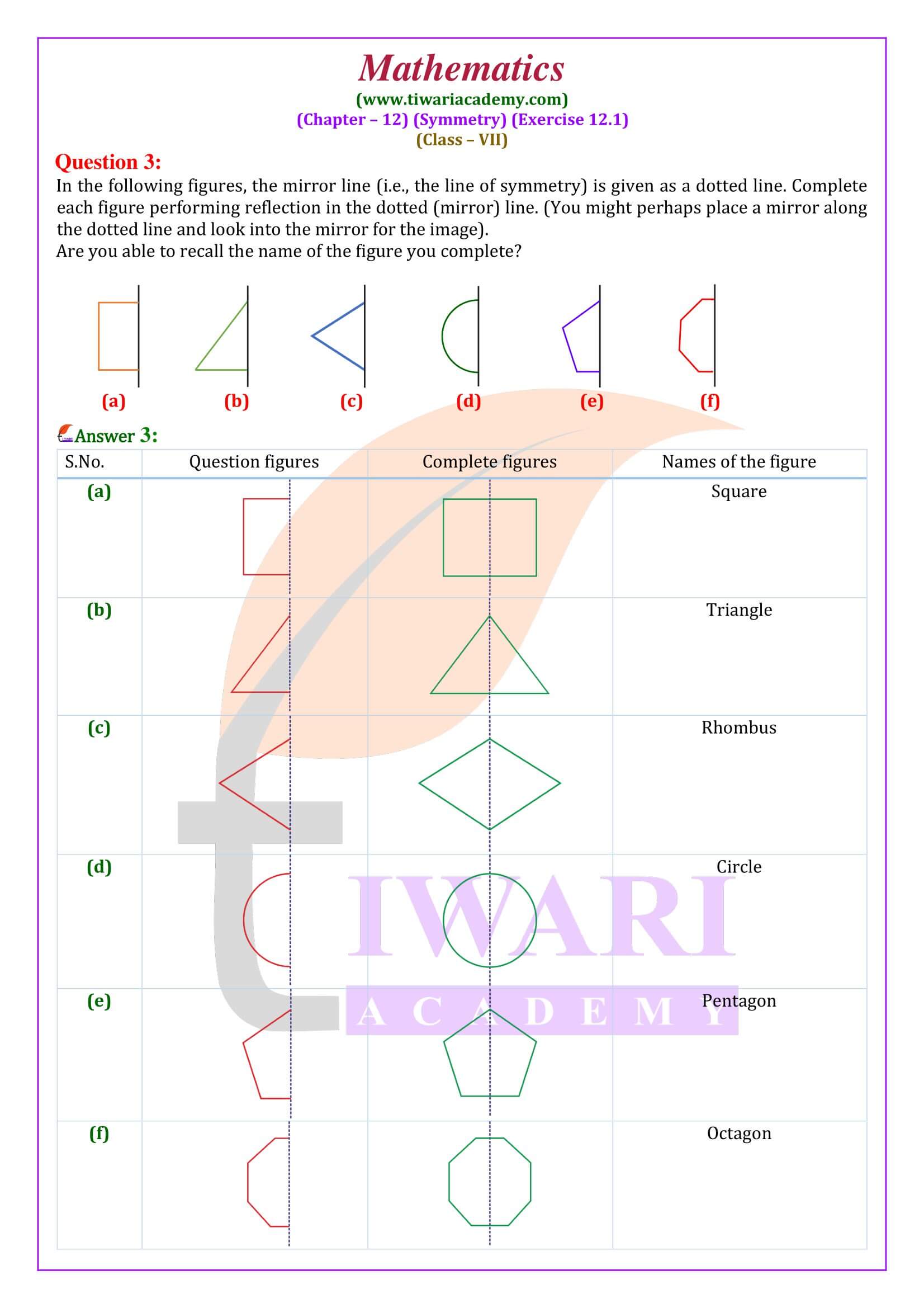
All the solutions are available in PDF and Videos format based on latest NCERT books issued for 2024-25. In class 7 math exercise 12.1 we will learn to draw or find the axis of symmetry in geometrical figures. All the questions are interesting to do and easy to understand.
Download Class 7 Maths App
Class 7 Maths Chapter 12 Exercise 12.1 Solution in Videos
Class 7 Maths App Hindi Medium
Class 7 Maths Exercise 12.1 Important Questions
How is symmetry important in everyday life?
Symmetry is something that we observe in many places in our daily lives without even noticing it. It is easily noticeable in various arts, buildings, and monuments. Nature uses symmetry to make things beautiful. Symmetry in everyday refers to a sense of harmonious and beautiful proportion and balance.
Which triangle has no line of symmetry?
A scalene triangle is a triangle with no lines of symmetry while an isosceles triangle has at least one line of symmetry and an equilateral triangle has three lines of symmetry.
What shape has the greatest lines of symmetry?
A shape can have more than one line of symmetry. Thus a rectangle has two lines of symmetry, an equilateral triangle has three lines of symmetry, and a square has four. A circle has an infinite number of lines of symmetry since it can be folded about any diameter.
Symmetry
Symmetry is an important geometrical concept which is used in every field of activity. The professionals, artist, architects and many other make use of the concept of symmetry. The shapes which are evenly balanced and are in perfect proportion are said to be in symmetry.
Line of Symmetry
If there is a line about which the figure may be folded so that two parts of figure will coincide, then the line so formed by folding the figure is called line of symmetry.
The line of symmetry is also known as axis of symmetry
The concept of line of symmetry is closely related to the reflection in mirror. If we place a mirror along the line of symmetry, then the image of one side of the figure is exactly same as the figure on other side of the line of symmetry. This is the reason why the line of symmetry of a figure is also called reflection symmetry.
Examples:
1. A line segment is symmetrical about its perpendicular bisector.
2. A given angle having equal arms is symmetrical about the bisector of the angle.
3. An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides.
4. Let ABCD be a kite in which AB = AD and BC = DC. Then, kite ABCD is symmetrical about the diagonal AC
5. A semicircle ABC has on line of symmetry, namely the perpendicular bisector of diameter AB.
6. Let ABCD be an isosceles trapezium in which AB PDC and AD = BC. Let E and F be the midpoints of AB and DC respectively.
7. A rectangle has two lines of symmetry, each one of which is the line joining the midpoints of opposite sides.
8. A rhombus is symmetrical about each one of its diagonals.
9. A square has 4 lines of symmetry, namely, the diagonals and the lines joining the midpoints of its opposite sides.
10. An equilateral triangle is symmetrical about each one of the bisectors of its interior angles.
11. A circle is symmetrical about each one of its diameters.
