by Tiwari Academy
NCERT Solutions for Class 7 Maths Chapter 11 Exercise 11.1 Exponents and Powers in Hindi and English medium updated for CBSE session 2024-25. The modified solutions of ex. 11.1 class VII mathematics are given here based on new syllabus and latest textbooks issued for academic year 2024-25.
Class 7 Maths Exercise 11.1 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 11 | Exercise: 11.1 |
| Chapter Name: | Exponents and Powers |
| Content Type: | PDF and Videos Solution |
| Session: | CBSE 2024-25 |
| Medium: | Hindi and English Medium |
Class 7 Maths Chapter 11 Exercise 11.1 Solution
All the solutions are given in PDF file format as well as videos format free to use without any format login. Class 7 math NCERT solutions is available in English Medium and Hindi Medium format. Student can use these solutions and study material free of cost.
Class 7 Maths Offline App
Class 7 Maths Chapter 11 Exercise 11.1 Solution in Videos
Class 7 Maths App in Hindi
Class 7 Maths Exercise 11.1 Extra Questions
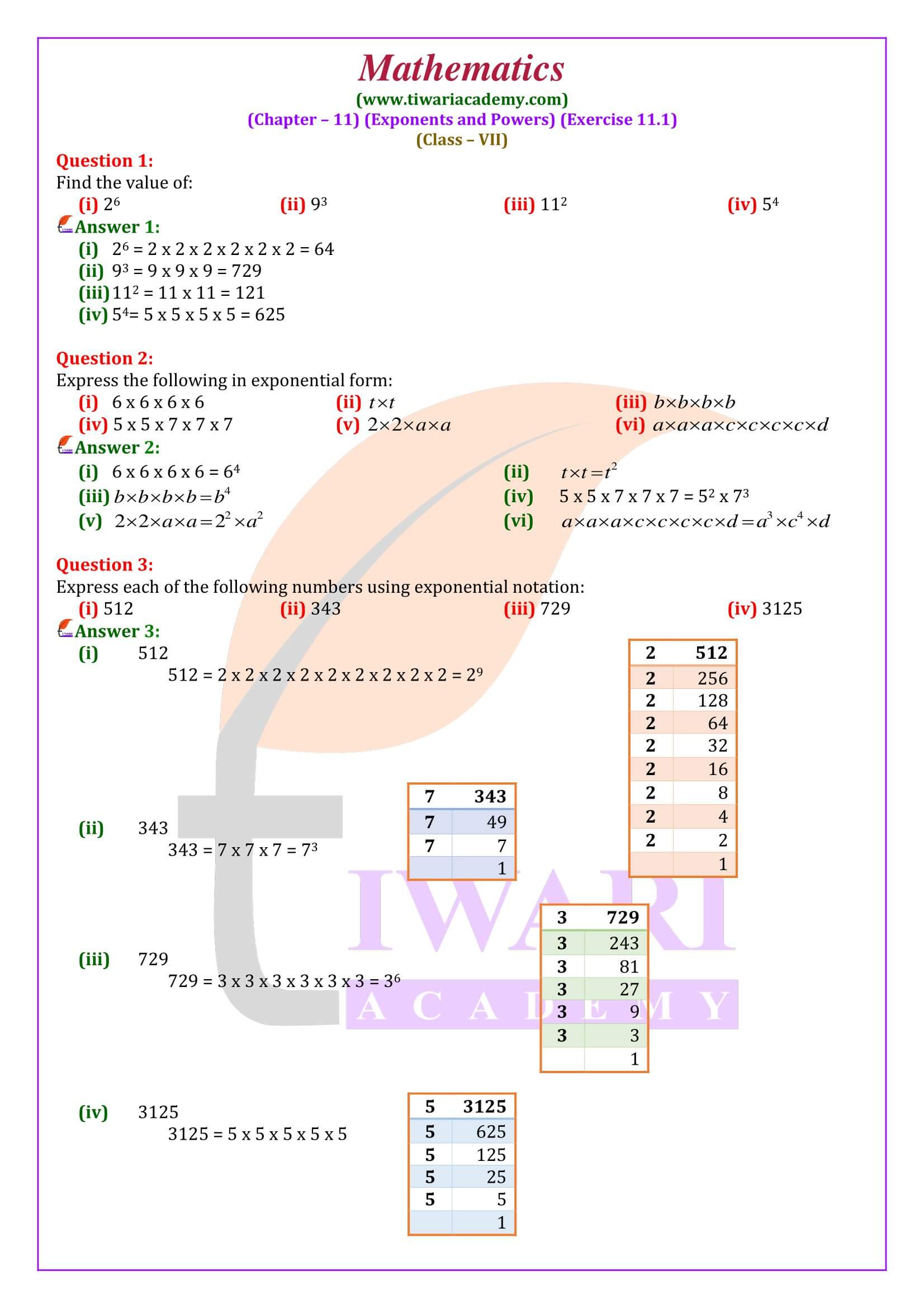
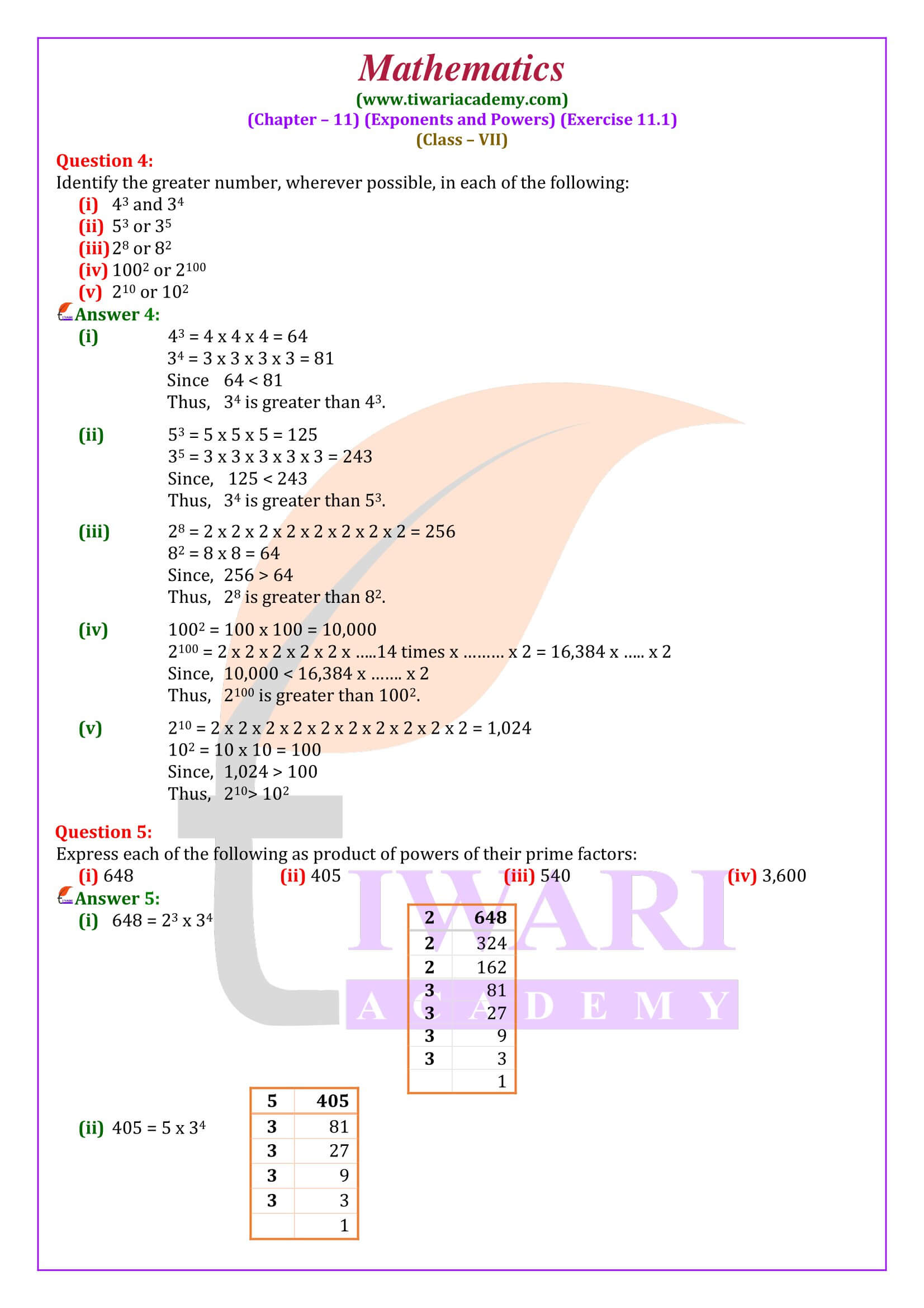
Express the following numbers as a product of powers of prime factors: (i) 81, (ii) 1000
(i) 81 = 3 x 3 x 3 x 3 = 3⁴
Thus, 81 = 3⁴
(ii) 1000 = 2 x 2 x 2 x 5 x 5 x 5 = 2³ x 5³
Thus, 1000 = 2³ x 5³
Write the reciprocal of each of the following in exponential form: (i) (2/5)⁶ (ii) (-3/7)⁸⁹ (iii) 3⁸
We know that the reciprocal of (a/b)ᵐ is (b/a)ᵐ
Therefore,
(i) Reciprocal of (2/5)⁶ = (5/2)⁶
(ii) reciprocal of (-3/7)⁸⁹ = (-7/3)⁸⁹
(iii) reciprocal of 3⁸ = (1/3)⁸
Exponents and Powers
An expression that represents repeated multiplication of the same factor is called a power. The number 5 is called the base, and the number 2 is called the exponent. The exponent corresponds to the number of times the base is used as a factor.
Example:
5 x 5 x 5 x 5 as 5 and read it as 5 raised to the power 4. In 5⁴, we call 5 the base and 4 the exponent (or index). The number of times a number is multiplied by itself is called the exponent.
Similarly, (-6) x (-6) x (-6) is written as (-6). In (-6)³, the base is (-6) and the exponent is 3.
This notation is called exponential form or power notation.
Similarly, the product of a rational number multiplied several times by itself can be expressed in the same notation.
Thus, (2/3)⁴ = 2/3 X 2/3 X 2/3 X 2/3
If a/b is a rational number, then
(a/b)² = 2/3 X 2/3 = (2 X 2)/(3 X 3)
In general, we have:
(a/b)ⁿ = (a)ⁿ /(b)ⁿ for every positive integer n
Class 7 Maths Exercise 11.1 Important Questions
How are exponents used in daily life?
Another example of using exponents in real life is when you calculate the area of any square. If you say “My room is twelve foot by twelve-foot square”, you’re meaning your room is 12 feet × 12 feet — 12 feet multiplied by itself — which can be written as (12 ft)2. And that simplifies to 144 square feet.
How does the exponents relate to one another?
Adding the exponents is just a short cut! The “power rule” tells us that to raise a power to a power, just multiply the exponents. Here you see that 52 raised to the 3rd power is equal to 56. The quotient rule tells us that we can divide two powers with the same base by subtracting the exponents.
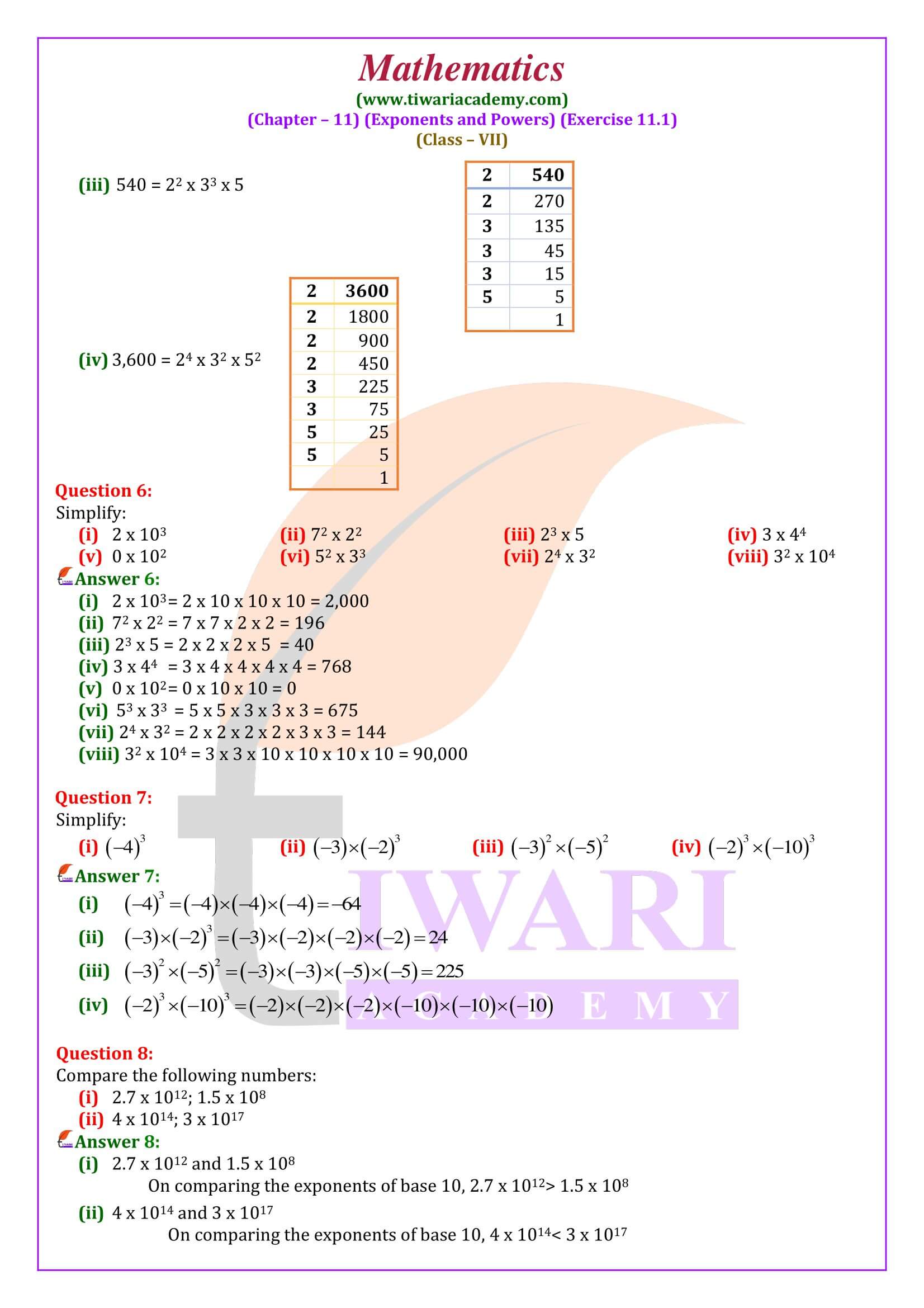
Simplify and express the result in exponential form: (i) (3/4) ⁷ x (3/4) ⁵ (ii) (-2/5) ¹¹ x (-2/5) ⁴
We have:
(i) (3/4) ⁷ x (3/4) ⁵ = (3/4) ⁷⁺⁵ = (3/4)¹²
(ii) (-2/5) ¹¹ x (-2/5) ⁴ = (ii) (-2/5) ¹¹⁺⁴ = (-2/5) ¹⁵
Reciprocal of a Rational Number:
If a/b is a nonzero rational number, then its reciprocal is b/a and we write, (a/b)⁻¹ = b/a
Now, (a/b)ᵐ = aᵐ / bᵐ
Reciprocal of (a/b)ᵐ = (b/a)ᵐ
Laws of Exponents
The laws of exponents on integers can be extended to rational numbers.
Thus, for any rational number a/b and positive integers m and n, we have:
(i) (a/b)ᵐ X (a/b)ⁿ = (a/b)ᵐ⁺ ⁿ
(ii) If m > n, then (a/b)ᵐ / (a/b) ⁿ = (a/b)ᵐ⁻n
(iii) If m < n, then (a/b)ᵐ / (a/b)ⁿ = 1/(a/b)ᵐ ⁻ ⁿ
(iv) {(a/b) ᵐ }ⁿ = (a/b) ᵐⁿ
(v) (a/b)⁻ ⁿ = 1/(a/b)ⁿ = 1/ (aⁿ /bⁿ) = bⁿ /aⁿ = (b/a) ⁿ
Thus, (a/b)⁻ ⁿ = (b/a) ⁿ
(vi) (a/b)₀ = 1
(vii) (a/b)⁻¹ = (b/a)
