NCERT Solutions for Class 7 Maths Chapter 10 Exercise 10.2 Algebraic Expression in Hindi and English Medium updated for CBSE and State board session 2025-26. The solution of ex. 10.2 class 7th mathematics is revised according to new syllabus and textbooks issued for CBSE 2025-26.
NCERT Class 7 Maths Exercise 10.2 Solution in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 10 | Exercise: 10.2 |
| Topic Name: | Algebraic Expressions |
| Content: | Exercise and Extra Questions |
| Session: | CBSE 2025-26 |
| Medium: | English and Hindi Medium |
Class 7 Maths Chapter 10 Exercise 10.2 Solution
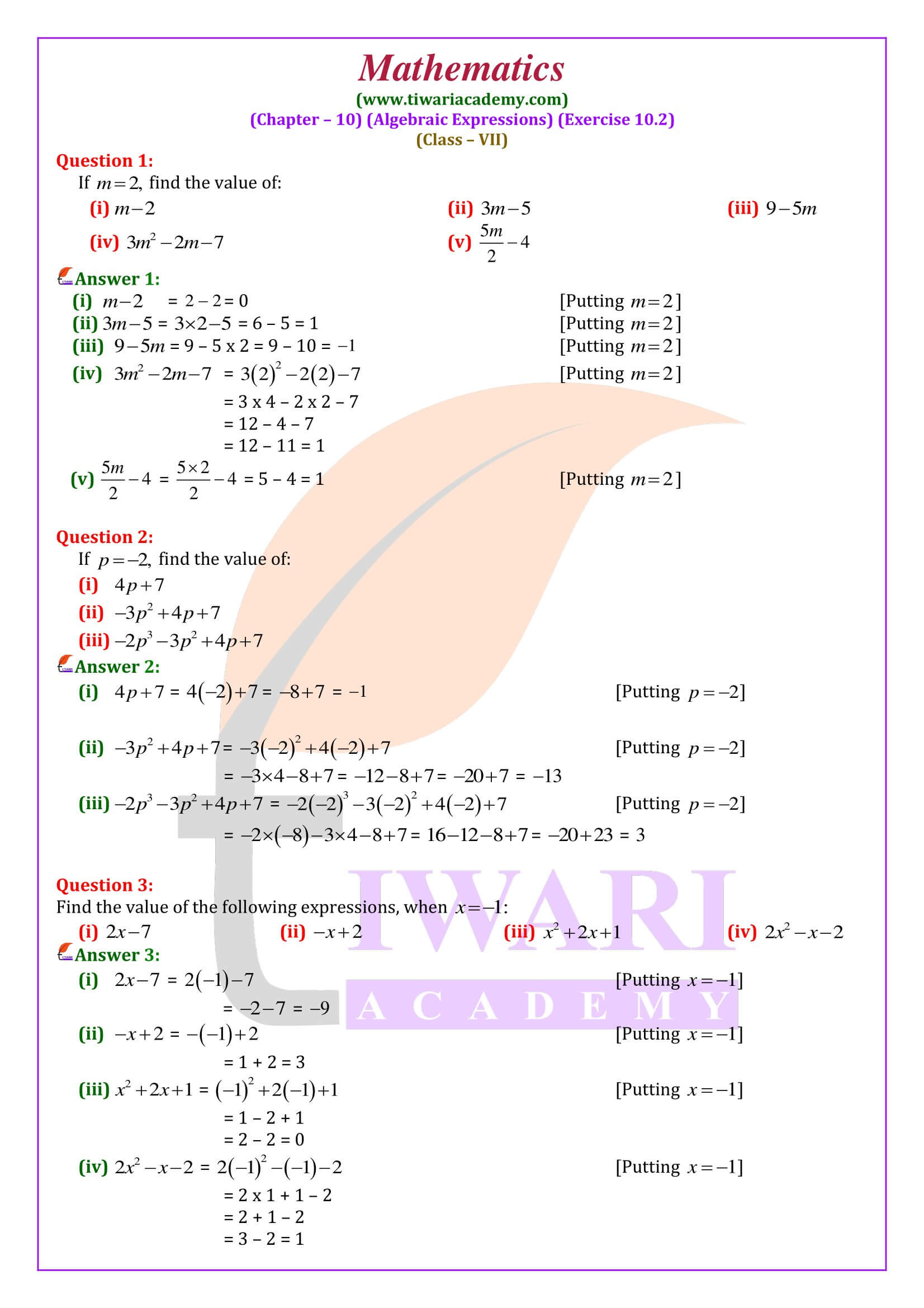
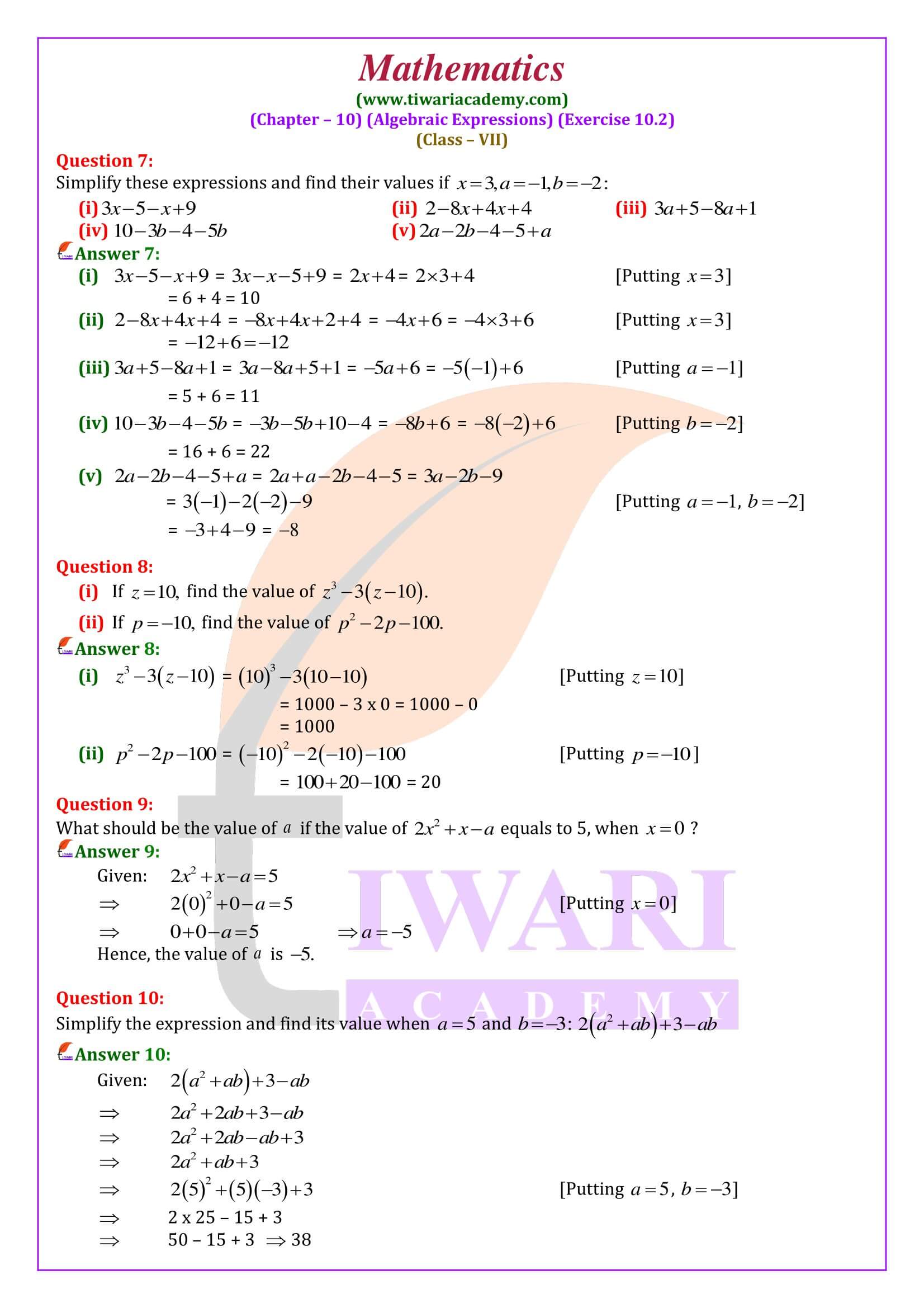
All the contents are prepared according to the latest NCERT Books for academic session 2025-26. There are only 10 questions in class 7 math exercise 10.2 and all are simple to solve or understand. Here we will learn to evaluate an algebraic expression using the values of given variables.
Class 7 Maths Chapter 10 Exercise 10.2 Solution in Videos
Class 7 Maths Exercise 10.2 Extra Questions
Multiply: (i) 6ab by 4b, (ii) 5ab² c³ by 7a² b c, (iii) -6x² yz by 2/3 xy³ z²
We have:
(i) 6ab x 4b = (6 x 4) x (a x b x b) = 24ab¹⁺¹ = 24ab²
(ii) 5ab² c³ x 7a² b c = (5 x 7) x (a x a² x b² x b x c³ x c)
= 35 x a¹⁺² x b²⁺¹ x c³⁺¹ = 35 a³ b³ c⁴
(iii) -6x² yz by 2/3 xy³ z² = – 6 x 2/3 x (x² X x X y X y³ x z X z²)
= -4 X x²⁺¹ y³⁺¹ X z¹⁺² = – 4x³ y4 z³
Multiply: (i) -8ab² c, 3a² b and -1/6, (ii) (5/8) a³ b², 12a² b and 6c
We have:
(i) -8ab² c X 3a² b X -1/6 = -8 X 3 X -1/6 (a X a² X b² X b X c)
= 4 X (a¹⁺² X b²⁺¹ X c)
= 4 a³ b³ c
(ii) (5/8) a³ b², 12a² b and 6c
= (5/8) X 12 X 6 (a³ X a² X b² X b X 6c)
= 45 a⁵ b³ c
Multiplication of Algebraic Expressions
Rules for multiplying of algebraic expressions:
(i) The product of two factors with like signs is positive, and the product of two factors with unlike signs is negative.
(ii) If a is any variable and m, n are positive integers then aᵐ x aⁿ = aᵐ⁺ⁿ
Thus, x³ X x⁵ = x³⁺⁵ = x⁸
Class 7 Maths Exercise 10.2 Important Questions
What is the rule of multiplication in algebra?
The multiplication/division rule for equations tell us that every term on both sides of an equation can be multiplied or divided by the same term (except zero) without changing the solution set of the equation.
How can algebraic expressions be used in real life?
Most of the times, physical and chemical sciences employ the basics of algebraic equations. In the case of computer sciences, the algorithms are based on the algebraic operations only. Moreover, algebra is involved in the field of art and architecture to calculate correct proportions so as to put forth a masterpiece.
How do you simplify algebraic expressions?
Here are the basic steps to follow to simplify an algebraic expression:
(i) Remove parentheses by multiplying factors.
(ii) Use exponent rules to remove parentheses in terms with exponents.
(iii) Combine like terms by adding coefficients.
(iv) Combine the constants.
Multiplication of Monomials
Rules:
(i) The coefficient of the product of two monomials is equal to the product of their coefficients.
(ii) The variable part in the product two monomials is equal to the product of the variables in the given monomials.
These rules may be extended for the product of three or more monomials.