by Tiwari Academy
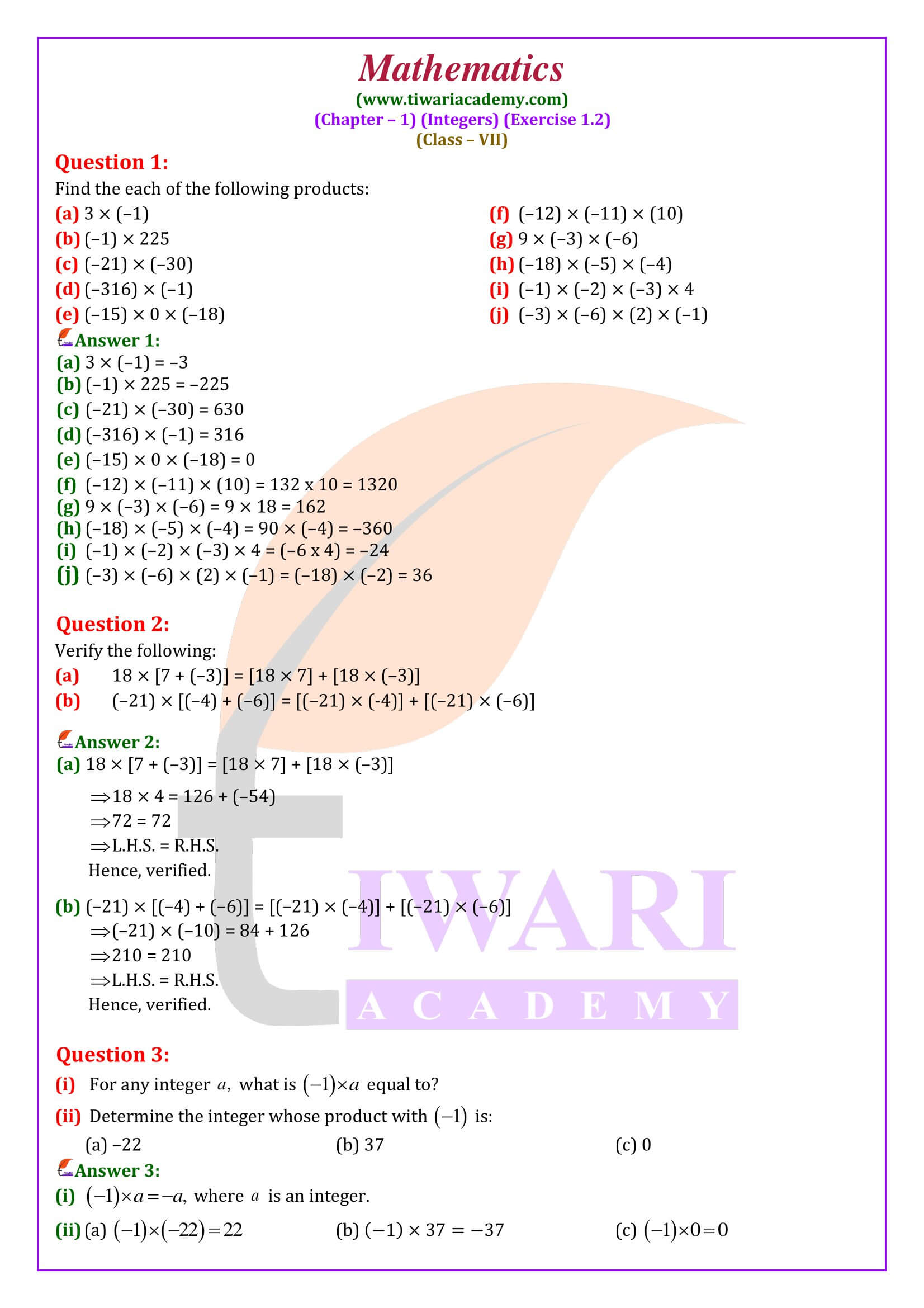
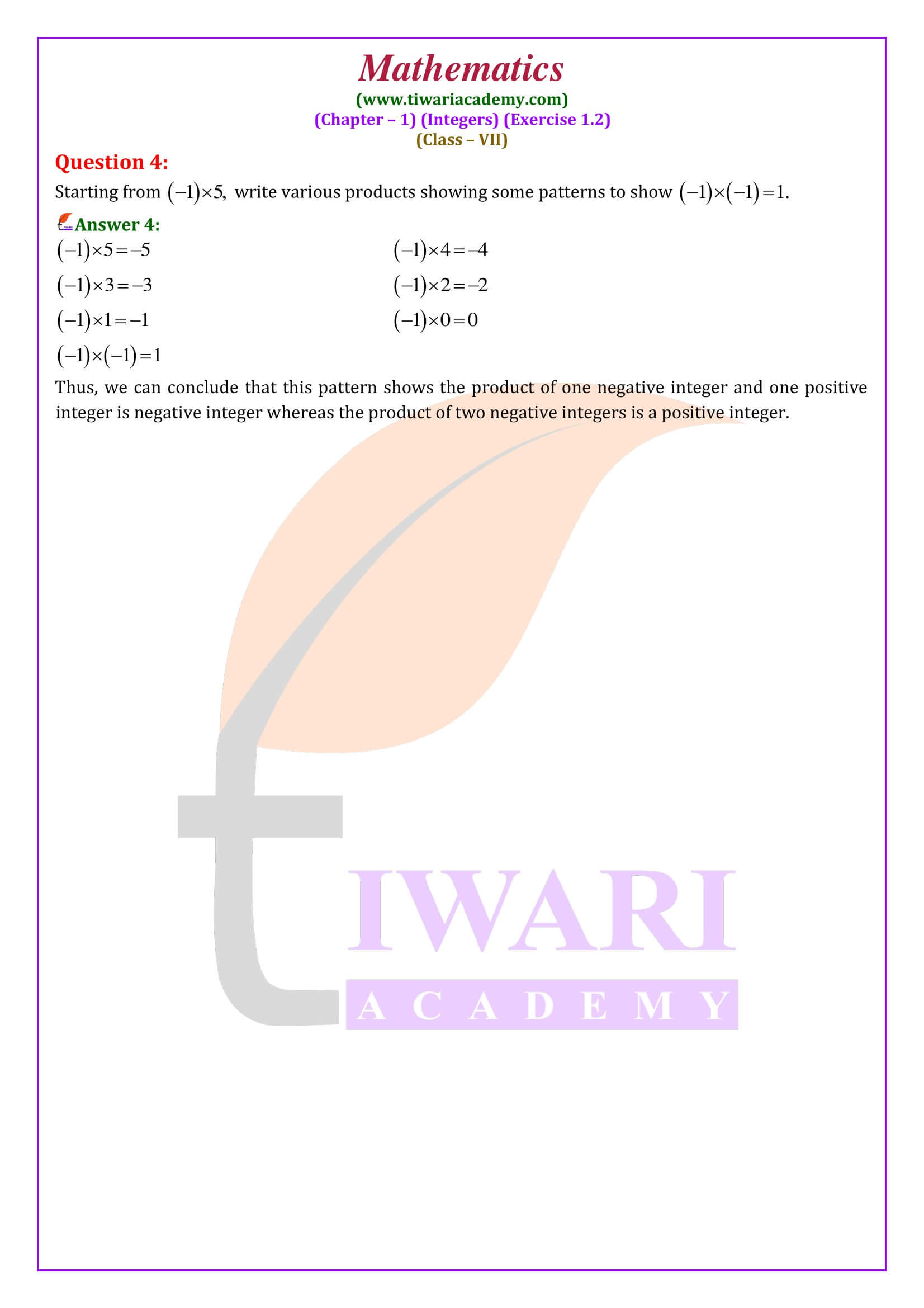
NCERT Solutions for Class 7 Maths Chapter 1 Exercise 1.2 Integers in Hindi Medium and English Medium updated and modified for academic session 2024-25. Get here the solutions of ex. 1.2 class 7th mathematics based on new NCERT textbooks published for 2024-25 exams.
NCERT Solutions Class 7 Maths Exercise 1.2 in Hindi and English Medium
| Class: 7 | Mathematics |
| Chapter: 1 | Exercise: 1.2 |
| Topic Name: | Integers |
| Content: | NCERT Book’s Solutions |
| Academic Session: | CBSE 2024-25 |
| Medium: | English and Hindi medium |
Class 7 Maths Chapter 1 Exercise 1.2 Solution
All the solutions are useful for state board students also. Class 7 mathematics exercise 1.2 illustrate the variety of questions based on Distributive Property, Commutative Property, and Associative Property. Some word problems based on daily life are also given at the end of exercise 1.2, which are interesting to solve.
CBSE App for Class 7 Solution
Multiplication of Integers
Rule 1. To find the product of two integers with unlike signs, we find the product of their values regardless of their signs and give a minus sign to the product.
Properties of Multiplication of Integers
I. Closure Property for Multiplication:
The product of two integers is always an integer.
Example:
(i) 7 x 5 = 35, which is an integer.
(ii) (- 8) x 4 = 32, which is an integer.
(iii) 9 x (- 6) = – 54, which is an integer.
(iv) (- 8) x (- 7) = 56, which is an integer.
For any two integers a and b, we have: (a x b) = (b x a).
Example:
(i) 5 x (- 8) = – 40 and (- 8) x 5 = – 40.
So, 5 x (- 8) = (- 8) x 5.
(ii) (- 9) x (- 7) = 63 and (- 7) x (- 9) = 63.
So, (- 9) x (- 7) = (- 7) x (- 9).
Class 7 Maths Exercise 1.2 Important Questions
Find each of the following products: (i) 6 x (- 5) (ii) (- 7) x 9 (iii) 35 x (- 18) (iv) (- 42) x20
We have:
(i) 6 x (- 5) = – 30.
(ii) (- 7) x 9 = – 63.
(iii) 35 x (- 18) = – (35 x 18) = -630.
(iv) (- 42) x 20 = – (42 x 20) = -840.
What are the properties of multiplication of integers?
The properties of multiplication of integers are:
- Closure property.
- Commutative property.
- Multiplication by zero.
- Multiplicative identity.
- Associative property.
- Distributive property
How do you multiply positive and negative integers?
There are two simple rules to remember: When you multiply a negative number by a positive number then the product is always negative. When you multiply two negative numbers or two positive numbers then the product is always positive. 3 times 4 equals 12.
Simplify: (i) 8 x (- 15) + 8 x 6 (ii) (- 18) x 7 + (- 18 x (- 4)
Using the distributive laws, we get:
(i) 8 x (- 15) + 8 x 6 = 8 x {(- 15) + 6}
Because, [ a x b + a x c = a x (b + c)} = 8 x (- 9) = – 72.
(ii) (- 18) x 7 + (- 18) x (- 4) = (- 18) x {7 + (- 4)}
Because, [ a x b + a x c = a x (b + c)] = (- 18) x 3 = – 54.
III. Associative Law for Multiplication
For any integers a, b and c, we have: (a x b) x c = a x (b x c).
Example:
(i) Consider the integers 3, – 5 and – 8.
We have: {3 x (- 5)} x (- 8) = (- 15) x (- 8) = 120
and 3 x {(- 5) x (- 8)} = (3 x 40) = 120.
So, {3 x (- 5)} x (- 8) = 3 x {(- 5) x (- 8)}.
(ii) Consider the integers (- 8), (- 6) and (- 5).
We have:
{(- 8) x (- 6)} x (- 5) = 48 x (- 5) = – 240.
And (- 8) x {(- 6) x (- 5)} = (- 8) x 30 = – 240.
So, {(- 8) x (- 6)} x (- 5) = (- 8) x {(- 6) x (- 5)}.
IV. Distributive Law of Multiplication over Addition
For any integers a, b and c, we have: a x (b + c) = (a x b) + (a x c).
Example:
(i) Consider the integers 5, (- 6) and (- 8). We have:
5 x {(- 6) + (- 8)} = 5 x (- 14) = -70
And {5 x (- 6)} + {5 x (- 8)} = (- 30) + (- 40) = – 70.
So, 5 x {(- 6) + (- 8)} = {(5 x (- 6)} + {5 x (- 8)}.
V. Existence of Multiplicative Identity
For every integer a, we have: (a x 1) = (1 x a) = a. 1 is called the multiplicative identity for integers.
Examples:
(i) (12 x 1) = 12.
(ii) (- 16) x 1 = – 16.
VI. Existence of Multiplicative Inverse
Multiplicative inverse of a non-zero integer a is the number
1/a, a.(1/a) = (1/a). a = 1
Examples:
(i) Multiplicative inverse of 6 is 1/6.
(ii) Multiplicative inverse of – 6 is – 1/6.
VII. Property of Zero
For every integer a, we have:
(a x 0) = (0 x a) = 0.
Example:
(i) 8 x 0 = 0 x 8 = 0.
(ii) (- 6) x 0 = 0 x (- 6) = 0.
Which important topics do students study in exercise 1.2 of grade 7th Maths?
The important topics that students study in exercise 1.2 of grade 7th Maths are:
1. Multiplication of Integers
a. Multiplication of a Positive and a Negative Integer
b. Multiplication of two Negative Integers
c. Product of three or more Negative Integers
2. Properties of Multiplication of Integers
a. Closure under Multiplication
b. Commutativity of Multiplication
c. Multiplication by Zero
d. Multiplicative Identity
e. Associativity for Multiplication
f. Distributive Property
g. Making Multiplication Easier
Is exercise 1.2 of class 7th Maths important for the school exams?
Yes, exercise 1.2 of class 7th Maths is very important for the school exams. Every year questions come from this exercise in the school exams. All the questions of this exercise are important and can come in the exams. But, questions 1, 2 (a), 5 (a), 6, 7, and 8 are the most important.
How many total examples and questions are there in exercise 1.2 of class 7th Maths?
There are 4 examples (examples 2, 3, 4, 5) and 9 questions in exercise 1.2 of class 7th Maths. All the questions and examples of this exercise are nice, logical, and interesting. All questions of this exercise are distinct from each other.
How much time, students need to do exercise 1.2 of class 7th Maths?
Students need a maximum of 2-3 days to complete exercise 1.2 (chapter 1) of class 7th mathematics if they give at least 1 hour per day to this exercise. This time depends on many factors like student’s working speed, efficiency, and capability, etc.
