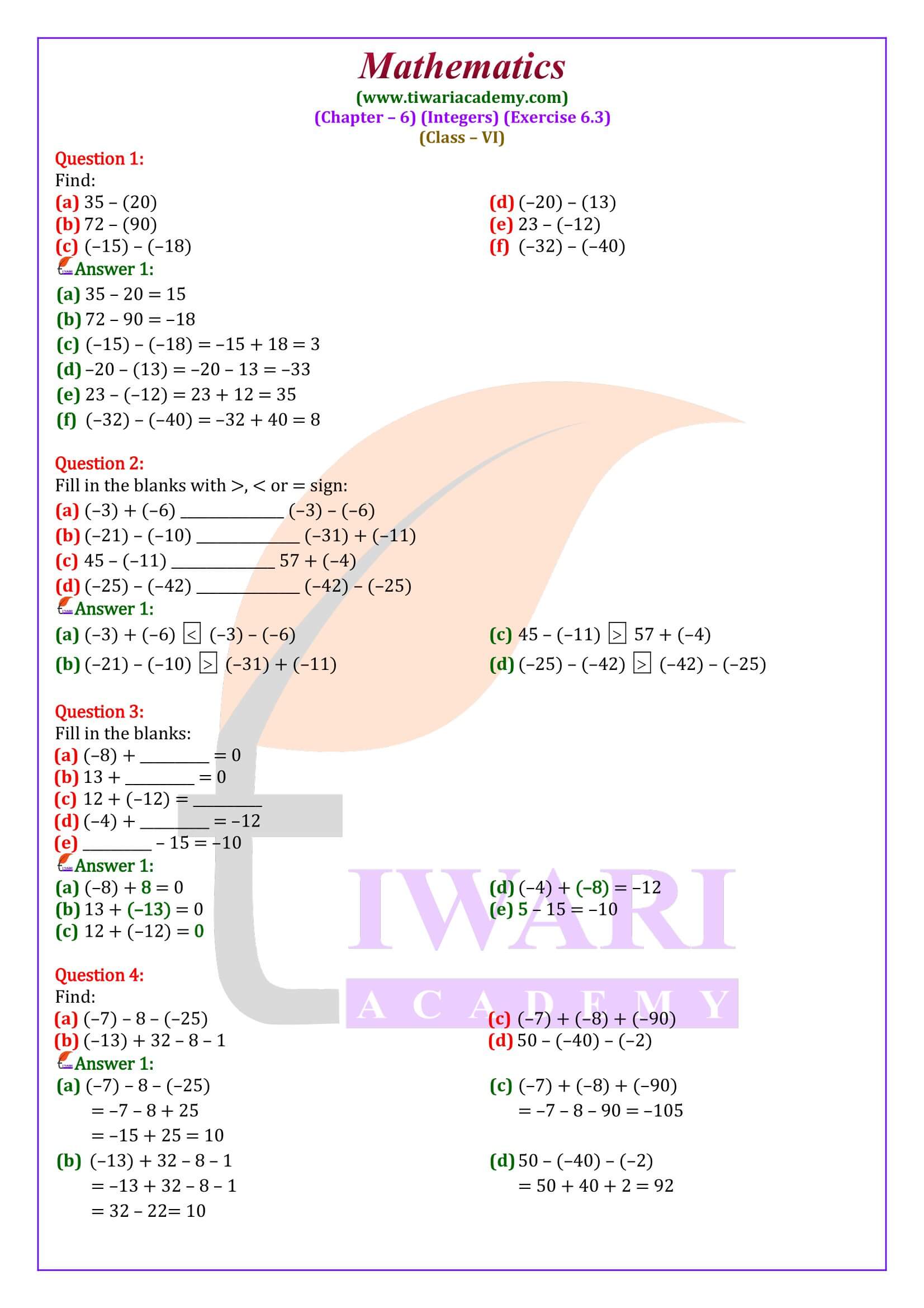
NCERT Solutions for Class 6 Maths Chapter 6 Exercise 6.3 in English and Hindi Medium updated for CBSE 2025-26 session. Get here the revised solutions of ex. 6.3 class 6 Maths based on the latest NCERT books and rationalised syllabus for CBSE 2025-26.
6th Maths Exercise 6.3 Solution in Hindi and English Medium
Class 6 Maths Chapter 6 Exercise 6.3 Solution
Class VI Mathematics Ex. 6.3 of NCERT textbook chapter 6 Integers in Hindi and English Medium free to use online or download in PDF file format. All the NCERT (https://ncert.nic.in/) solutions for class 6 mathematics are updated for academic session 2025-26 in PDF and videos format free to use without any login or password. There are only four questions in CBSE class 6 mathematics exercise 6.3 containing the questions of addition and subtraction of integers.
| Class: 6 | Mathematics |
| Chapter: 6 | Exercise: 6.3 |
| Chapter Name: | Integers |
| Content: | NCERT Questions Solution |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English |
Properties of Addition of Integers
Property- 1 (Closure Property):
The sum of two integers is also an integer, i.e., for any of two integers a and b, a + b is also an integer. Examples:
- (i) 3 + 5 = 8 and 8 is an integer.
- (ii) 5 + (–2) = 3 and 3 is an integer.
- (iii) (–9) + (–3) = –12 and –12 is an integer.
Property- 2 (Commutative Property):
The sum of two integers remains unchanged if they interchange their places, i.e., for two integers a and b, a + b = b + a.
Examples:
(i) 4 + (–6) = –2 and (–6) + 4 = –2 \ 4 + (–6) = (–6) + 4 (ii) 38 + (–25) = 13 and (–25) + 38 = 13
So, 38 + (–25) = (–25) + 38.
Property- 3 (Associative Property):
The addition of integers is associative, i.e., for any three integers a, b and c, we have, (a + b) + c = a + (b + c)
Example:
Consider the integers – 6, 3 and –17.
We have, [(–6) + 3] –17 = –3 – 17 = –20
and (–6) + [3 + (–17)] = –6 – 14 = –20 \ [(–6) + 3] – 17 = (–6) + [3 + (–17)].
Property 4:
If a is any integer, then a + 0 = a and 0 + a = a.
Examples:
(i) 4 + 0 = 4 and 0 + 4 = 4 \ 4 + 0 = 4 = 0 + 4
(ii) (–6) + 0 = –6 and 0 + (–6) = –6 \ (–6) + 0 = –6 = 0 + (–6).
Property 5.
For every integer a, there exists its opposite –a such that a + (–a) = 0 = (–a) + a.
Examples:
3 + (–3) = 0 and (–3) + 3 = 0 \ 3 + (–3) = 0 = (–3) + 3. Thus, additive inverse of 3 is –3 and the additive inverse of –3 is 3. Property 6. Every integer has a successor and a predecessor, i.e., for every integer a, a – 1 and a + 1 are integers which are known as predecessor and successor respectively.
Class 6 Maths Exercise 6.3 Important Questions
Write successor and predecessor of 6 and -3.
(i) 7 is successor of 6 and the predecessor of 6 is 5.
(ii) –4 and –2 are respectively the predecessor and successor of –3.
Subtract: (i) 6 from 9 (ii) –8 from –2 (iii) –5 from 12 Solution: (i) In order to subtract 6 from 9, we change the sign of 6 and add it to 9. Thus, 9 – 6 = 9 + (–6) = 3 (ii) Using the rule of subtraction –2 – (–8) = –2 + 8 = 6 (iii) Using the rule of subtraction 12 – (–5) = 12 + 5 = 17.
(i) In order to subtract 6 from 9, we change the sign of 6 and add it to 9. Thus, 9 – 6 = 9 + (–6) = 3
(ii) Using the rule of subtraction –2 – (–8) = –2 + 8 = 6
(iii) Using the rule of subtraction 12 – (–5) = 12 + 5 = 17.
Find the value of: (i) 832 + (–183) + (–832) + 213 + 2150, (ii) –18 – [(–42) + (–9) – 5]
(i) 832 + (–183) + (–832) + 213 + 2150 = 832 – 183 – 832 + 213 + 2150
= 832 – 183 – 832 + 213 + 2150
= – 183 – 832 + 832 + 213 + 2150 (collecting the integers with ‘+’ and ‘–’ signs)
= – 1015 + 3195 = 2180
(ii) –18 – [(–42) + (–9) – 5] = – 18 – [(–42) + (–9) – 5]
= – 18 – [–42 – 9 – 5] = – 18 – [– 56]
= – 18 + 56 = 38.
Calculate: 5 + 45 – 3 – 28 – 34 + 25 – 21 + 42 – 38
We can write 5 + 45 + 25 + 42 – 3 – 28 – 34 – 21 – 38
= 117 – 124 = -7
Subtraction of Integers
subtraction is the reverse operation of addition. For example, subtracting 4 from 8 is same as finding a number which when added to 4 gives 8. Clearly, the answer is 4.
Thus, 8 – 4 = 4.
Thus, we find that to subtract –3 from 7, we add the negative or additive inverse of –3 to 7.
In fact, this is the rule for subtraction of integers as stated below:
Rule:
If a and b are two integers, then to subtract b from a, we change the sign of b and add it to a, i.e., a – b = a + (–b).
Thus, for subtracting two integers, we take the additive inverse of the integer to be subtracted and add it to the another integer.
Property 1:
If a and b are integers, then a – b is also an integer.
Examples:
(i) 3 – 6 = –3 which is an integer.
(ii) 3 – (–6) = 9 which is an integer.
Property 2:
Subtraction of integers is not commutative, i.e., for any two integers a and b, a – b ≠ b – a.
Examples:
(i) 4 – 2 ≠ 2 – 4
(ii) – 9 – (–6) ≠ – 6 – (–9)
Property 3:
For every integer a, a – 0 = a, i.e., 0 is the right identity for subtraction.
Class 6 Maths Exercise 6.3 Extra Questions with Answer
What is the closure property of addition of integers?
(Closure Property): The sum of two integers is also an integer, i.e., for any of two integers a and b, a + b is also an integer. Examples:
3 + 5 = 8 and 8 is an integer.
Explain associative property of addition of integers.
Associative Property:
The addition of integers is associative, i.e., for any three integers a, b and c,
we have, (a + b) + c = a + (b + c)
Example:
Consider the integers – 6, 3 and –17. We have, [(–6) + 3] –17 = –3 – 17 = –20 and (–6) + [3 + (–17)] = –6 – 14 = –20 \ [(–6) + 3] – 17 = (–6) + [3 + (–17)].
The sum of two integers is 64. If one of the integers is –25, find the other.
Sum of two integers is 64, one integer is -25
Let second integer is a, then a + (-25) = 64
So, another integer will be 64 – (-25) = 64 + 25 = 89
We know that the addition of integers is commutative and associative. As a consequence of these two properties of addition and the subtraction of integers, we are now in a position to find the values of the expression containing many terms with plus and minus sign, by using the following steps.
- Put all terms containing plus sign together and add them.
- Put all terms containing minus sign together and add them.
- Find the difference of the absolute values of the two sums obtained in steps 1 and 2.
- Give the result of step 3, the sign of the sum having large absolute value.
Is exercise 6.3 of class 6th Maths important from the exam point of view?
Yes, exercise 6.3 of class 6th Maths is important from the exam point of view. Exercise 6.3 of grade 6th Maths has 4 short questions. Questions 1, 3 have 5 parts each, and questions 2, 4 have 4 parts each. Question 2, 3, and 4 are the most important and easy questions of exercise 6.3 of 6th standard Maths.
Are there any examples in exercise 6.3 of Class 6 Maths?
Yes, there are three examples (examples 7, 8, 9) in exercise 6.3 of class 6th Maths. All three examples of this exercise are nice and easy. Students can easily and quickly solve these examples.
What will students study in exercise 6.3 of class 6 Maths?
In exercise 6.3 of class 6th Maths, students will study how to Subtract Integers with the help of a Number Line. This exercise is easy, logical, and interesting.
What types of questions will come from exercise 6.3 of grade 6 Maths NCERT?
Exercise 6.3 of grade 6th Maths has 4 questions and 3 examples. From exercise 6.3 of grade 6th Maths, short answer type questions like fill in the blanks, true or false, yes or no, one-word answer, MCQ, match the following, etc will come in the school exams.