NCERT Solutions for Class 6 Maths Chapter 6 Exercise 6.1 in Hindi and English Medium updated for CBSE session 2025-26. The question’s explanation of 6.1 class 6th Maths are revised according to new syllabus and updated NCERT textbook for CBSE 2025-26 examination.
6th Maths Exercise 6.1 Solution in Hindi and English Medium
Class 6 Maths Chapter 6 Exercise 6.1 Solution
Class VI Mathematics NCERT (https://ncert.nic.in/) Exercise 6.1 of chapter 6 Integers in Hindi and English Medium free to use online or download in PDF file format for session 2025-26. All the solutions and book for class 6 mathematics exercise 6.1 are updated for academic session 2025-26. NCERT Class 6 Maths Exercise 6.1 give a brief idea about plotting numbers on number line. All the questions are solved step by step with suitable diagrams.
| Class: 6 | Mathematics |
| Chapter: 6 | Exercise: 6.1 |
| Chapter Name: | Integers |
| Content: | Text PDF and Videos Solutions |
| Medium: | English and Hindi Medium |
Need for Integers and Their Introduction
We know that if we subtract a whole number from another whole number, the difference may not always be a whole number. In fact, when a smaller number is subtracted from a larger number, we get a whole number. For example, 5 – 4 = 1, 25 – 13 = 12, 40 – 26 = 14 etc. are whole numbers. But, we have no whole numbers to represent 6 – 8, 16 – 40, 15 – 20 etc. So, there is a need to extend our number system so that we have numbers to represent such differences.
Corresponding to natural numbers1, 2, 3, 4, 5, 6, …etc.; we introduce new numbers –1, –2, –3, –4, –5, –6, …etc., called minus one, minus two, minus three, minus four, minus five etc. respectively such that 1 + (–1) = 0, 2 + (–2) = 0, 3 + (–3) = 0 and so on. –1 is also called negative of 1 and –1 and 1 are called the opposites of each other.
Combining these new numbers with whole numbers, we obtain a new collection of numbers which is written as …, –3, –2, –1, 0, 1, 2, 3, … These numbers are called integers.
The numbers 1, 2, 3, 4, 5, …, i.e., natural numbers are called positive integers. The numbers –1, –2, –3, –4, –5, …are called negative integers. The number zero is an integer, which is neither positive nor negative.
Use of Integers in Day-to-Day Life Situations
Use of integers is quite common in our day-to-day life. For example, positive and negative integers are used to represent concepts such as height above and below sea level; profit and loss; rise and fall in prices etc. In mathematical terms, we represent above sea level, profit, rise in price etc., by positive integers and height below sea level, loss, fall in price etc., by negative integers.
For example:
if the height 5 km above sea level is represented by +5, then the height below sea level will be represented by –5. Similarly, if ‘a profit of Rs. 50 in a transaction’ is represented by +50, then ‘a loss of Rs 50 in a transaction’ will be represented by –50.
Representation of Integers on The Number Line
In order to represent integers on a number line, we draw a line and mark a point O almost in the middle of it as shown in Fig., Now, we let off equal distances on right hand side as well as on the left hand side of O. On the right side, we label to the point of divisions as 1, 2, 3, 4, 5 etc. and the point O as 0. Since, negative integers are opposite of positive integers, namely natural numbers, therefore, we represent negative integers –1, –2, –3, –4, –5 etc.in the opposite direction, i.e., on the left side on the number line.
Clearly, 1 and –1 are at equal distances from 0 but in the opposite directions. Similarly, 2 and –2 are at equal distances from 0 but in opposite directions and so on.
Class 6 Maths Exercise 6.1 Important Questions
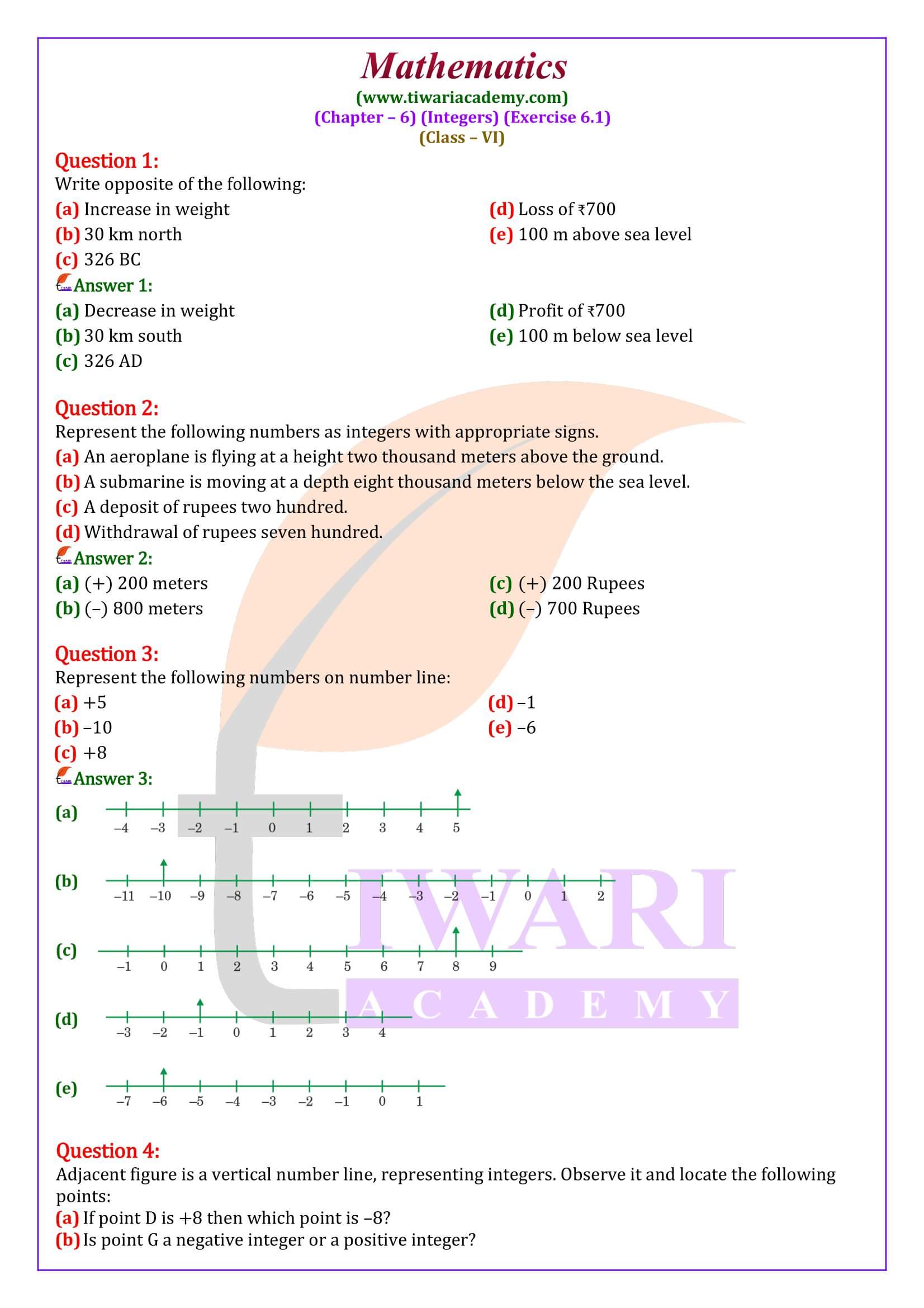
Write the opposite of each of the following: (i) Increase in population (ii) Depositing money in bank (iii) Going east (iv) Gaining a weight of 12 kg (v) A loss of Rs 60 (vi) 8 km above sea level (vii) 70 (viii) –24.
Opposite of given terms is as:
- (i) Decrease in population
- (ii) Drawing money from bank
- (iii) Going west
- (iv) loosening a weight of 12 kg
- (v) A profit of Rs 60
- (vi) 8 km below sea level
- (vii) -70
- (viii) +24.
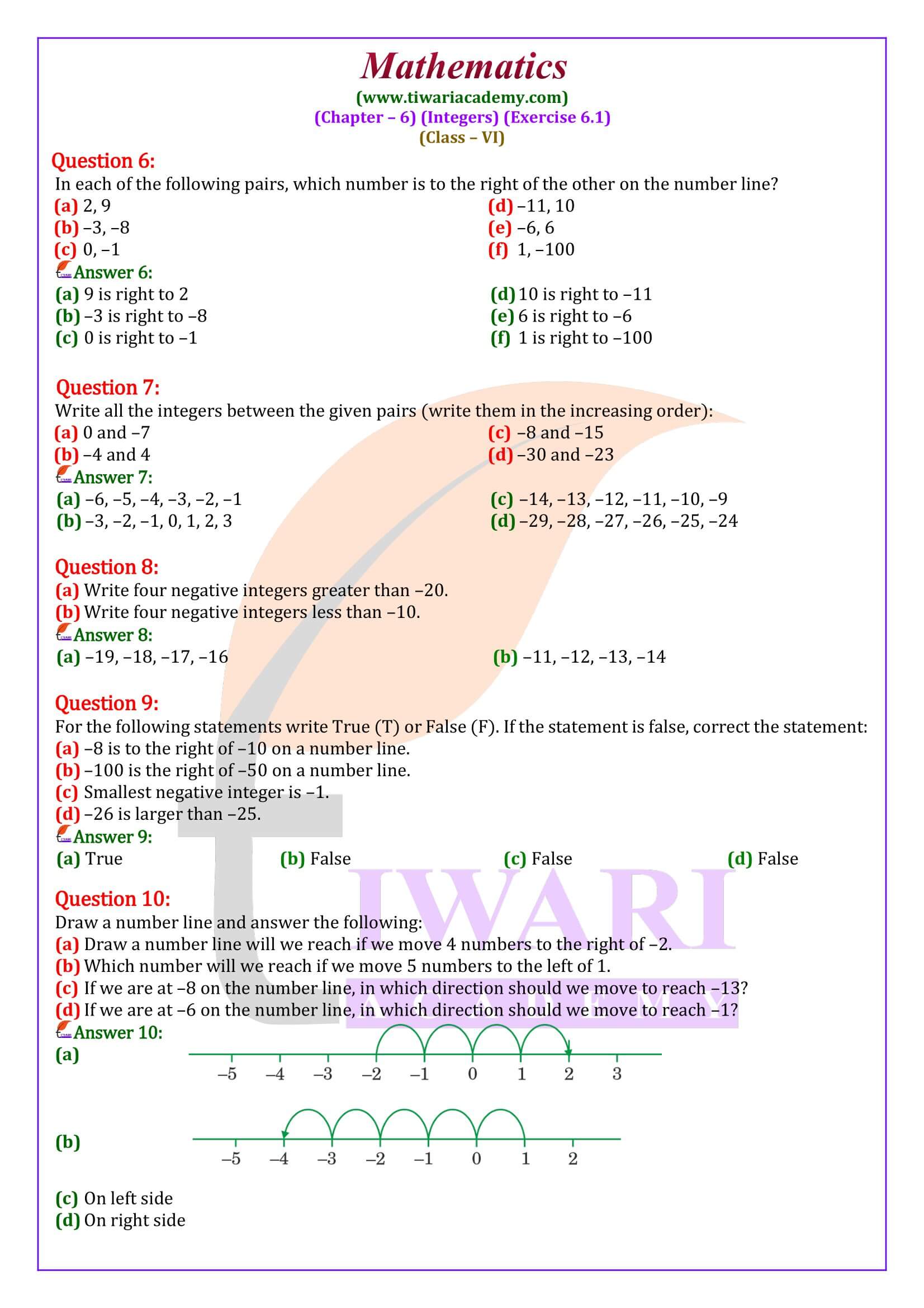
Which number is smaller in each of the following pairs? (i) 7, –7 (ii) 0, –14 (iii) –13, –8 (iv) 217, –445
(i) We know that on the number line –7 is to the left of 7. So –7 < 7. (ii) Since 0 is greater than every negative integer, therefore –14 < 0. (iii) We know that on the number line –13 is to the left of –8. So, –13 < –8. (iv) Since every negative integer is less than every positive integer. Therefore, –445 < 217.
Negative of a Negative Integer
Take a coin. Put it on a table with face ‘tail’ up. Turn the coin so that the face ‘head’ is up. Now turn the coin again. What do you find? This brings the face ‘tail’ up, i.e., the coin is in its original position. Therefore, negative of a negative integer is a positive integer, i.e., – (–a) = a for any integer a.
Ordering of Integers
We have learnt that a whole number on the number line is greater than every whole number to its left. Thus, 7 > 4.
If we, now, follow the same criterion for the number line extended for integers, then an integer is greater than every integer to its left.
Thus, 3 > 2, since 3 is to the right of 2;
1 > 0, since 1 is to the right of 0;
0 > –2, since 0 is to the right of –2;
–4 > –5, since –4 is to the right of –5 on the number line.
Class 6 Maths Exercise 6.2 Extra Questions with Answer
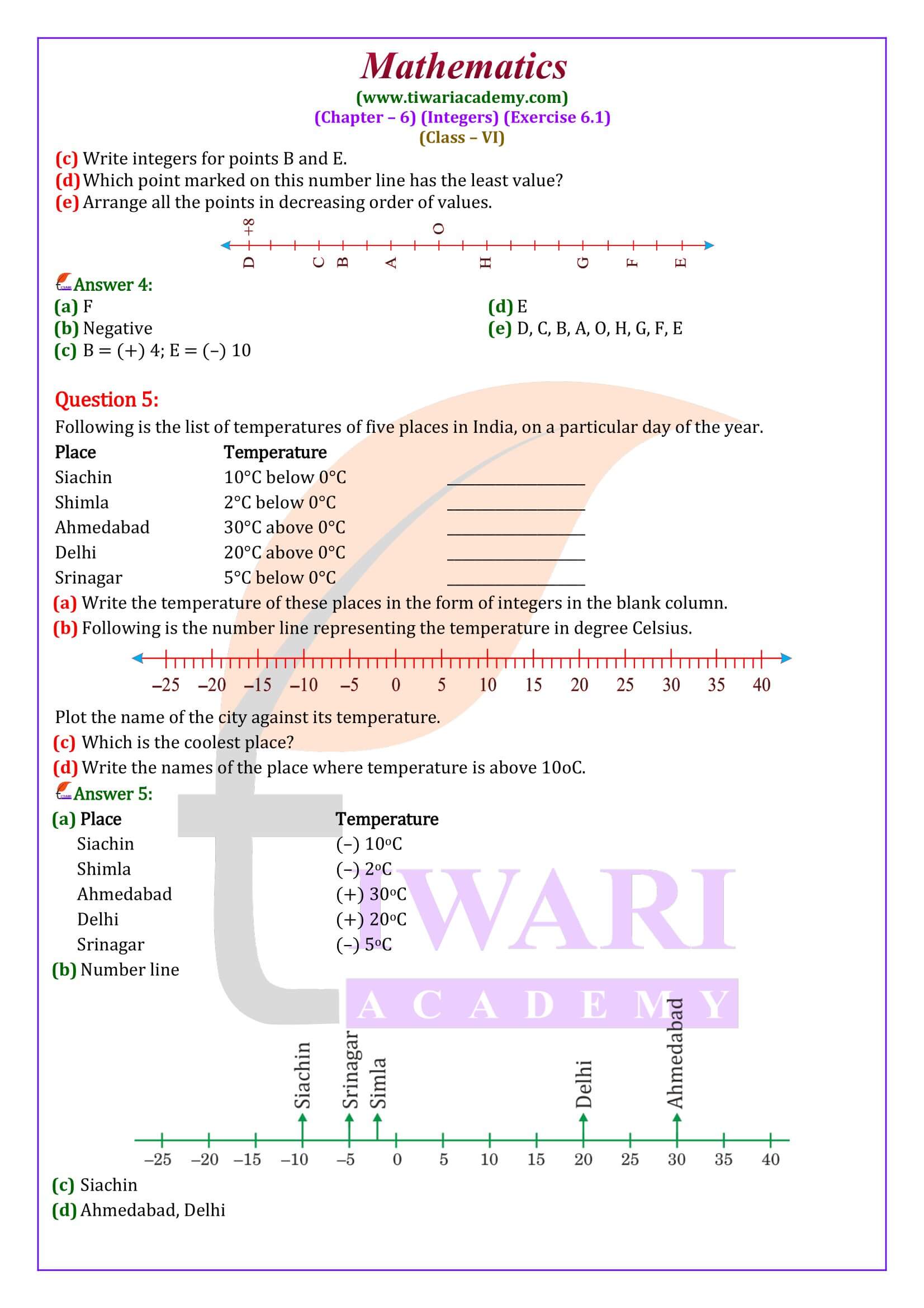
Indicate the following by using integers: (i) A profit of Rs 450, (ii) 10° C below zero, (iii) A deposit of Rs 4000, (iv) 3 km below sea level.
(i) +450
(ii) -10
(iii) +4000
(iv) -3
Which number is smaller in each of the following pairs? (i) 0, –7 (ii) 8, –5 (iii) –12, 6 (iv) –350, 2
(i) 0 > –7
(ii) 8 > –5
(iii) –12 < 6
(iv) –350 < 2
Arrange the following integers in the increasing order: –7, –13, 3, 7, 0, –9, 12
Increasing order of given numbers is as:
-13 < -9 <-7 < 0 < 3 < 7 < 12
In general, the following results are quite obvious:
(i) Every positive integer is greater than every negative integer.
(ii) Every positive integer is greater than zero.
(iii) Every negative integer is less than zero.
(iv) If a and b are any two integers such that a > b, then –a < –b.
(v) If a and b are any two integers such that a < b, then –a > –b.
For example, 6 > 4 and –6 < –4. Also 6 < 9 and –6 > –9.
Are there any examples in exercise 6.1 of 6th Maths?
Yes, there are two examples (examples 1, 2) in exercise 6.1 of class 6th Maths. Both the examples of this exercise are nice and easy. Students can easily and quickly solve these examples.
Which questions of exercise 6.1 of class 6 Maths can students expect in exams?
Exercise 6.1 of class 6th Maths has 10 ten questions. All questions are important at their place. But, the most important questions of this exercise that students can expect in the school exams are questions 2, 4, 7, 8, 9, 10.
Is exercise 6.1 of NCERT 6 Maths complicated to solve and understand?
Exercise 6.1 of grade 6th Maths is not very easy and not very difficult. It lies in between simple and complicated. Sometimes most students face problems in understanding the language of questions. However, the difficulty level of any exercise depends on students also. According to some students exercise 6.1 is easy, some students find exercise 6.1 in between easy and hard, and some find it complicated.
Is exercise 6.1 of class 6th Maths lengthy?
Exercise 6.1 of class 6th Maths is not very lengthy. This exercise has 10 questions. Students need a maximum of 2 days to complete exercise 6.1 of class 6th Maths if they give 1 hour per day to this exercise. This time may increase or decrease according to the student’s working speed.