NCERT Solutions for Class 6 Maths Chapter 3 Exercise 3.7 in Hindi and English Medium modified and updated for session 2025-26 CBSE and State board. The questions of ex. 3.7 class 9th Maths are revised according to new NCERT books issued for new academic year 2025-26.
6th Maths Exercise 3.7 Solution in Hindi and English Medium
Class 6 Maths Chapter 3 Exercise 3.7 Solution
Class VI Mathematics NCERT textbook Ex. 3.7 of chapter 3 Playing with Numbers in English Medium as well as Hindi Medium updated for CBSE Session 2025-26. Prepare for school exams 2021 with the help of PDF solutions and Videos. Please suggest us, if any, changes to be made in Tiwari Academy website or app, so that it become more user friendly.
| Class: 6 | Mathematics |
| Chapter: 3 | Exercise: 3.7 |
| Chapter Name: | Playing with Numbers |
| Medium: | Hindi and English |
| Content Mode: | Online and Offline |
| Academic Session: | 2025-26 |
Tests for Divisibility of Numbers
In order to find whether a given number is divisible by another number, we perform actual division and see whether the remainder is zero or not. Up till now, we were using this procedure to check whether a number is divisible by another number or not. But this process is time consuming. There are certain tests for divisibility of numbers by any of the numbers 2, 3, 4, 5, 6, 7, 8, 9, 10 and 11 such that by simply examining the digits of the given number, one can easily determine whether a given number is divisible by any of these numbers or not.
Class 6 Maths Exercise 3.7 Extra Questions with Answer
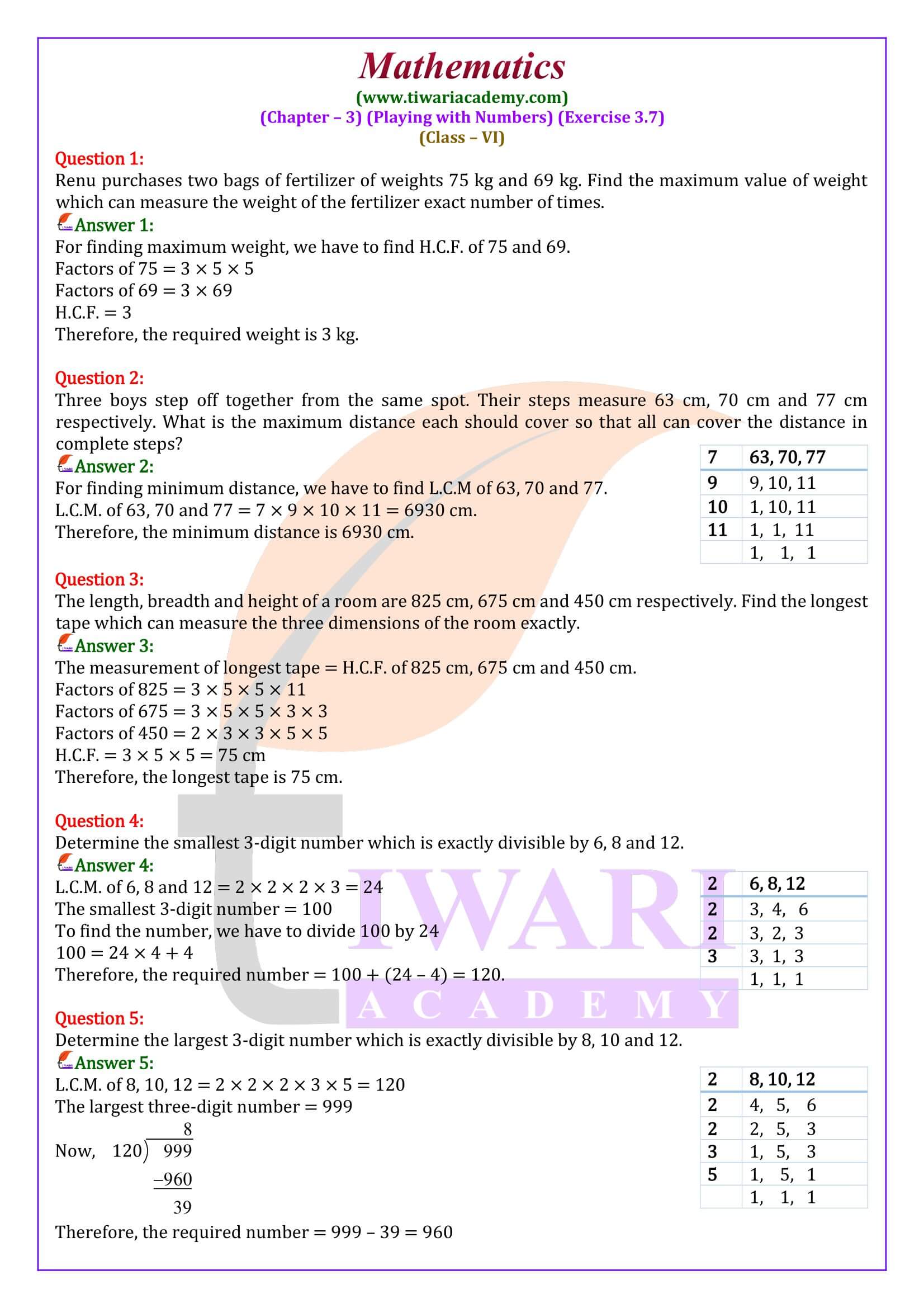
Can two numbers have 24 as their HCF and 200 as their LCM? Give reasons in support of your answer?
According the property, the HCF of given numbers is always a factor of their LCM. Here if we divide 200 by 24, the answer is not a whole number. Hence there are no two such numbers which HCF is 24 and LCM is 200.
Find the HCF and LCM of the following pairs of numbers: 220, 376.
HCF of 220, 376 is
Factors of 220 are 2 x 2 x 5 x 11
And factors of 376 are 2 x 2 x 2 x 47
Hence, HCF is 4
Now LCM of 220 and 376 is
| 2 | 220, 376 |
| 2 | 110, 188 |
| 2 | 55, 94 |
| 5 | 55, 47 |
| 11, 47 |
LCM of 220 and 376 is 2 x 2 x 2 x 5 x 11 x 47 = 20680
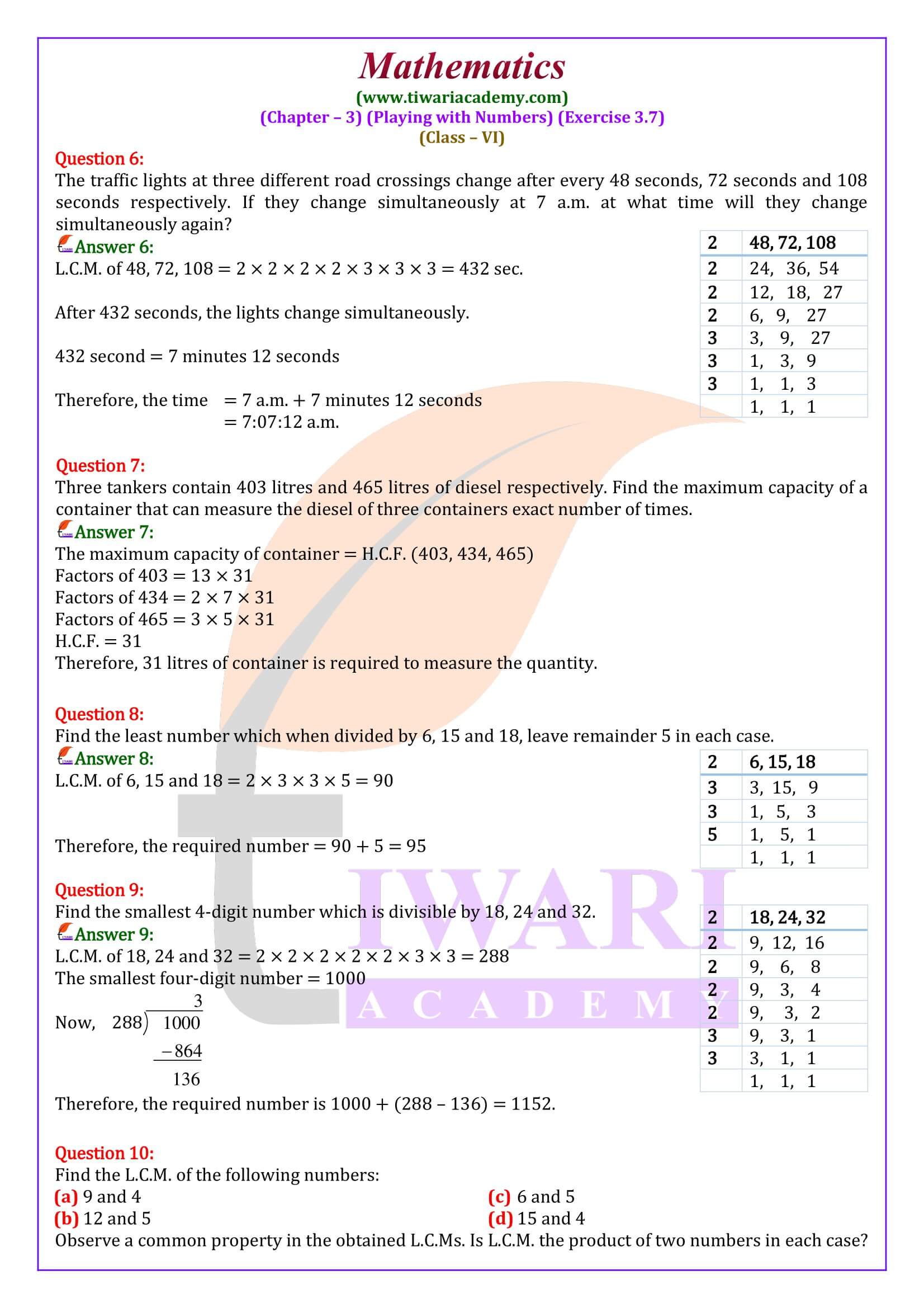
define the fifth property of HCF and LCM. Give reasons in support of your answer.
If a number, say a, is a factor of another number, say b, then HCF of a and b is a and their LCM is b
We assume a = 3 and b = 12
Factor of 3 are 1 x 3
And factors of 12 are 4 x 3
Hence, HCF is 3
And LCM is 3 x 4 = 12
Let us learn about these tests
| Test of Divisibility by | Condition | Example |
|---|---|---|
| 2 | A number is divisible by 2, if its 26, 242, 780, 1540 etc.are divisible last digit is even or 0. | 26, 242, 780, 1540 etc.are divisible by 2. |
| 3 | A number is divisible by 3, if the sum of its digits is divisible by 3. | In 5049:5 + 0 + 4 + 9 = 18 which is divisible by 3 and in 5232 :5 + 2 + 3 + 2 = 12 which is also divisible by 3. |
| 4 | A number is divisible by 4, if the number formed by the last two digits is a multiple of 4 or divisible by 4. | 516, 868, 1064, 123572 etc. are all divisible by 4. |
| 5 | A number is divisible by 5, if the digit at its unit place is either 5 or 0. | 150, 1345, 36590, 29395 etc. are all divisible by 5. |
| 6 | A number is divisible by 6, if the number is divisible by 2 and 3. | 360, 2358, 5922, 10014 etc.are all divisible by 6. |
| Test of Divisibility by | Condition | Example |
|---|---|---|
| 7 | A number is divisible by 7, if the difference between twice the last digit and the number formed by other digits is either 0 or a multiple of 7. | Consider 1267.Last digit = 7.Twice the last digit = 14.Number formed by other digits = 126.Difference = 126 – 14 = 112 which is a multiple of 7. so, 1267 is divisible by 7. |
| 8 | A number is divisible by 8, if the number formed by its last three digits is divisible by 8. | Consider 3128.Number formed by last 3 digits = 128, which is divisible by 8. so, 3128 is divisible by 8. |
| 9 | A number is divisible by 9, if the sum of its digits is divisible by 9. | The number 2718 is divisible by 9 as 2 + 7 + 1 + 8 = 18 is divisible by 9. |
| 10 | A number is divisible by 10, if the last digit (i.e., the digit at unit’s place) is 0. | 320, 850, 91380 etc.are all divisible by 10. |
| 11 | A number is divisible by 11, if the difference between the sums of alternate digits is 0 or a multiple of 11. | Consider 2162215.Sum of digits in odd places is = 2 + 6 + 2 + 5 = 15. Sum of digits in even places = 1 + 2 + 1 = 4.Difference = 15 – 4 = 11. The number is divisible by 11 |
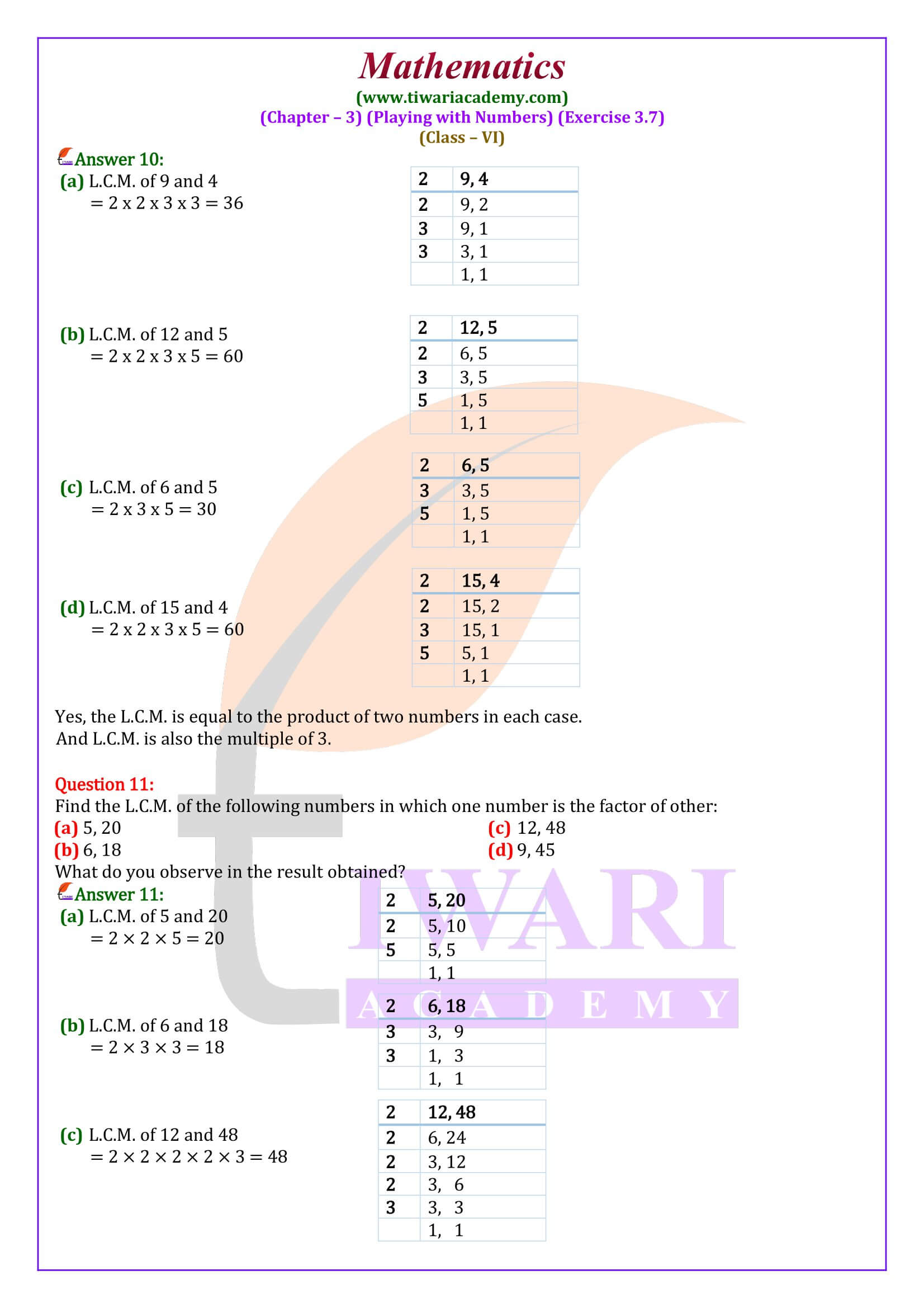
What is the core motive of exercise 3.7 of Class 6 Maths?
The core motive of exercise 3.7 of grade 6th Maths is to teach students the following things:
- How to find the Lowest Common Multiple (LCM) (The Lowest Common Multiple (LCM) of two or more given numbers is the lowest (or smallest or least) of their common multiples)
- How to solve some word problems on HCF and LCM.
Are there any examples in exercise 3.7 of class 6th Maths?
Yes, there are seven examples (examples 8, 9, 10, 11, 12, 13, 14) in exercise 3.7 of class 6th Maths. All examples are good and help students to solve similar exercise questions.
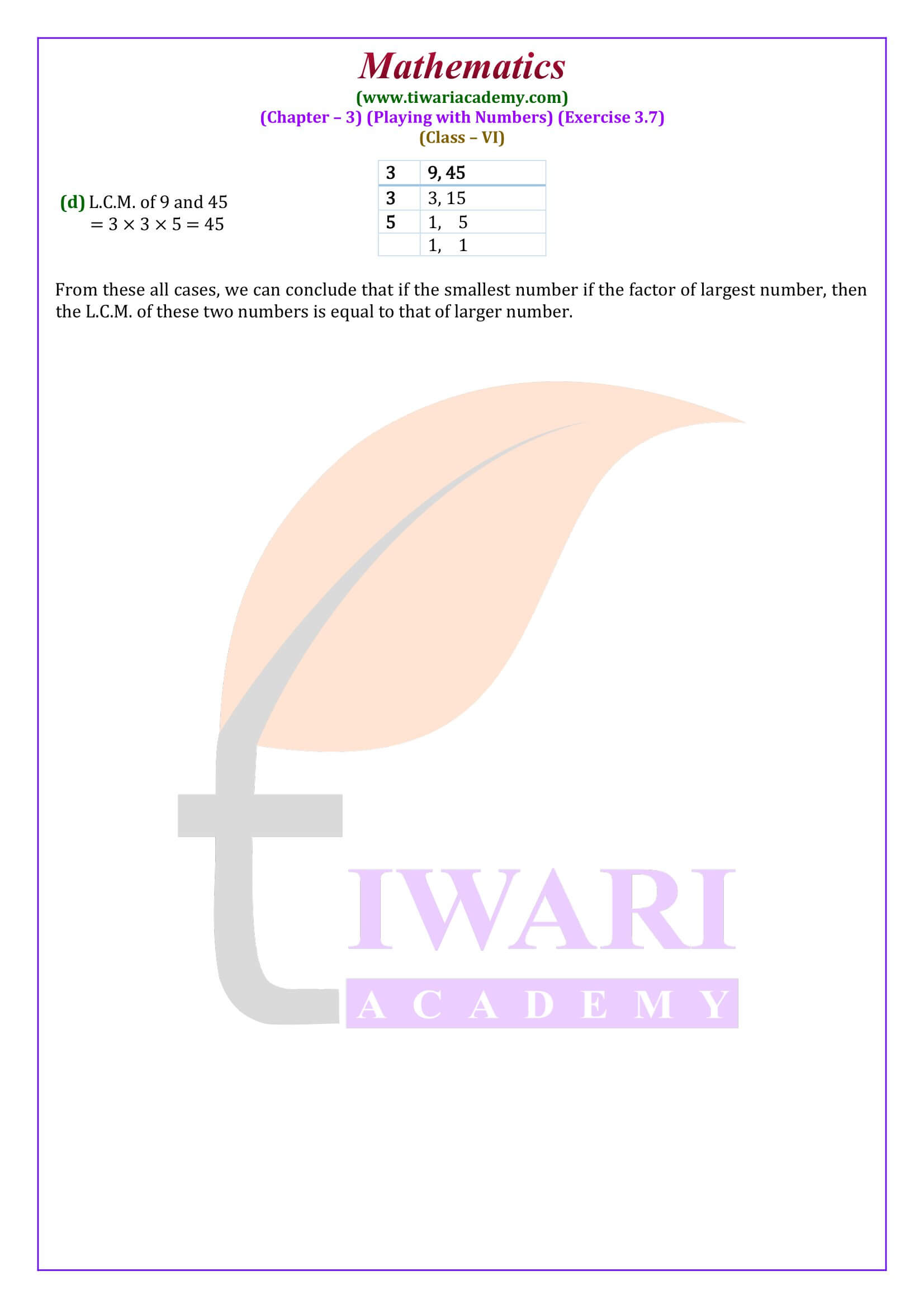
Which problems of exercise 3.7 of class 6th Maths teacher can give in the school exams?
Exercise 3.7 of class 6th Maths has seven examples (examples 8, 9, 10, 11, 12, 13, 14) and 11 questions. All problems of this exercise are good and interesting. The problems of exercise 3.7 that teachers can give in the school exams are questions 1, 2, 3, 4, 5, 6, 7, 9, and examples 10, 14.
What is the difficulty level of exercise 3.7 of class 6 Maths?
Exercise 3.7 of class 6th Maths is a little hard compared to other exercises of chapter 3 of class 6th Maths. In this exercise, students mainly face problems while solving word problems. This exercise needs a lot of practice.