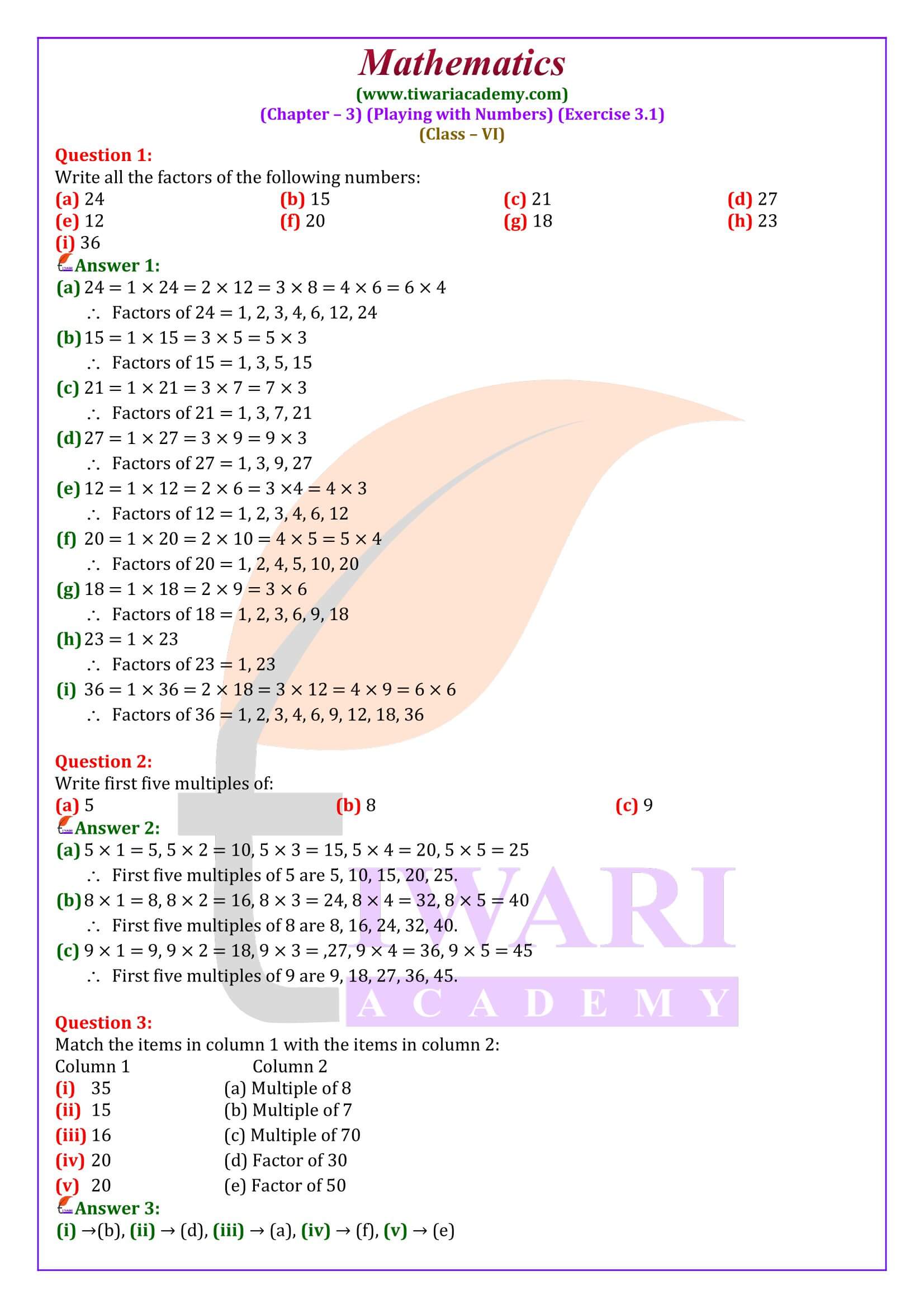
NCERT Solutions for Class 6 Maths Chapter 3 Exercise 3.1 in Hindi and English Medium updated modified for session 2025-26. The question answers of ex. 3.1 class 6th Maths are revised according to new syllabus and latest textbooks released for academic year 2025-26.
6th Maths Exercise 3.1 Solution in Hindi and English Medium
Class 6 Maths Chapter 3 Exercise 3.1 Solution
Class VI Mathematics NCERT Book Ex. 3.1 of chapter 3 Playing with Numbers updated for CBSE Board and State board student academic session 2025-26. Videos related to 6th Math NCERT ex. 3.1 is also given in solution as well as explanation format, where each question is explained properly.
| Class: 6 | Mathematics |
| Chapter: 3 | Exercise: 3.1 |
| Chapter Name: | Playing with Numbers |
| Medium: | Hindi and English Medium |
| Content: | NCERT Exercise Solutions |
| Session: | CBSE 2025-26 |
| Content Type: | Text, Images and Videos Format |
Factors and Multiples
Factor:
A factor of a number is an exact divisor of that number. In other words, a factor of a number is that number which completely divides the number without leaving a remainder.
Example: We know that, 12 = 4 × 3, 12 = 2 × 6, also, 12 = 1 × 12
This shows that each of the numbers 1, 2, 3, 4, 6 and 12 exactly divides 12.
Therefore, 1, 2, 3, 4, 6 and 12 are all factors of 12.
Multiple
A multiple of a number is a number obtained by multiplying it by a natural number.
If we multiply 2 by 1, 2, 3, 4, … , we get 2 × 1 = 2, 2 × 2 = 4, 2 × 3 = 6, 2 × 4 = 8, … .
Thus, 2, 4, 6, 8, … are multiples of 2.
Clearly, a number is a multiple of each of its factors.
Properties of Factors and Multiples
Property 1: 1 is a factor of every number
We have, 1 = 1 × 1; 2 = 1 × 2; 3 = 1 × 3; 9 = 1 × 9 and so on. Thus, 1 is a factor of every number.
Property 2: Every number is a factor of itself
We have, 1 = 1 × 1; 4 = 4 × 1; 12 = 12 × 1; 67 = 67 × 1 and so on.
We can express every number in this way. Hence, every number is a factor of itself.
Property 3: Every factor of a number is less than or equal to the number
Factors of 21 are 1, 3, 7 and 21 itself. Out of these numbers 21, itself is the largest factor and all other factors are less than 21.
The above property is also evident from the fact that divisors of a number are less than or equal to the number itself.
Property 4: Factors of a given number are finite
By property 3, every factor of a number is less than or equal to the number.
Hence, factors of a given number are finite.
Property 5: Every multiple of a number is greater than or equal to that number
Multiples of 3 are 3, 6, 9, 12, 15, ….
In fact, multiplies of a number are obtained by multiplying the number by 1, 2, 3, 4, 5, …. Therefore, the smallest multiple of a number is the number itself. Hence, every multiple of a number is greater than or equal to the number itself.
Property 6: Every number is a multiple of itself
We have, 5 = 5 × 1; 13 = 13 × 1; 18 = 18 × 1 and so on.
So, every number is a multiple of itself.
Property 7: The number of multiples of a given number is infinite
Multiples of 9 are 9, 18, 27, 36, 45, 54, 63, 72, …. Clearly, it is a never ending list.
Hence, the number of multiples of a given number is infinite.
Types of Numbers
Even Numbers:
All multiples of 2 are called even numbers.
Example: 2, 4, 6, 8, 10, 12, 14 etc. are all even numbers.
(ii) Odd Numbers:
Numbers which are not multiples of 2 are called odd numbers.
Example: 1, 3, 5, 7, 9, 11, 13, 15 etc. are all odd numbers.
(iii) Perfect Numbers:
If the sum of all the factors of a number is two times the number, then the number is called a perfect number.
Example: 6 is a perfect number, since the factors of 6 are 1, 2, 3, 6 and (1 + 2 + 3 + 6) = (2 × 6). 28 is a perfect number, since the factors of 28 are 1, 2, 4, 7, 14, 28 and (1 + 2 + 4 + 7 + 14 + 28) = (2 × 28).
(iv) Prime Numbers:
Each of the numbers which has exactly two factors, namely, 1 and itself, is called a prime number.
Example: The numbers 2, 3, 5, 7, 11, 13, 17 etc. are all prime numbers.
(v) Co-prime Numbers:
Two numbers are said to be co-prime numbers if they do not have a common factor other than 1.
Example: (2, 3); (3, 4); (5, 6); (8, 13) etc. are pairs of co-prime numbers.
Note: Any two prime numbers are always co-primes, but two co-primes need not be both prime numbers. For example, 14, 15 are co-primes, while none of 14 and 15 is a prime number.
(vi) Composite Numbers:
Numbers having more than two factors are known as composite numbers.
Each of the numbers 4, 6, 8, 9, 10, 12, 14, 15, …are composite numbers.
Class 6 Maths Exercise 3.1 Important Questions
What are prime numbers? List all prime numbers between 1 and 25.
Prime Numbers:
Each of the numbers which has exactly two factors, namely, 1 and itself, is called a prime number. In other words, we can say that prime numbers are only divisible by 1 or itself. Prime numbers between 1 and 25.
Example: The numbers 2, 3, 5, 7, 11, 13, 17, 19, 23 are all prime numbers lies between 1 and 25.
What is the smallest prime number? Is it an even number?
The smallest prime number is 2. Yes, it is an even number.
What are composite numbers? Can a composite number be odd? If yes, write the smallest odd composite number.
Numbers having more than two factors are known as composite numbers.
Each of the numbers 4, 6, 8, 9, 10, 12, 14, 15, …are composite numbers.
Yes, a composite number can be odd. The smallest odd composite number is 9.
What are twin-primes? Write all pairs of twin-primes between 1 and 20.
a pair of prime numbers (such as 3 and 5 or 11 and 13) differing by two.
All pairs of twin-primes between 1 and 20 are 3 and 5, 5 and 7, 11 and 13, 17 and 19.
(vii) Twin Primes:
Two prime numbers which have only one composite number between them are called twin primes.
Example: 3, 5; 5, 7; 11, 13 and 17, 19 etc. are pairs of twin primes between 1 and 20.
(viii) Prime Triplet:
A set of three consecutive prime numbers which have only one composite number between each two of them are called prime triplet.
Example: 3, 5, 7 is the only prime triplet.
Class 6 Maths Exercise 3.1 Extra Questions with Answers
What are co-primes? Give examples of four pairs of co-primes. Are co-primes always prime? If no, illustrate your answer by an example.
Co-prime Numbers:
Two numbers are said to be co-prime numbers if they do not have a common factor other than 1.
Example: (2, 3); (3, 4); (5, 6); (8, 13) etc. are pairs of co-prime numbers.
Any two prime numbers are always co-primes, but two co-primes need not be both prime numbers. For example, 14, 15 are co-primes, while none of 14 and 15 is a prime number.
what is the factor of a number? Give an example.
A factor of a number is an exact divisor of that number. In other words, a factor of a number is that number which completely divides the number without leaving a remainder.
Example: We know that, 12 = 4 × 3, 12 = 2 × 6, also, 12 = 1 × 12
Define composite numbers.
Numbers having more than two factors are known as composite numbers.
Each of the numbers 4, 6, 8, 9, 10, 12, 14, 15, …are composite numbers.
List all the prime numbers between 40 and 60.
The prime numbers between 40 and 60 are: 41, 43, 47, 53, 59.
Some Important Facts
- (i) 1 is neither prime nor composite.
- (ii) 2 is the smallest prime number.
- (iii) 2 is the only even prime number.
- (iv) All other even numbers are composite numbers.
On which concepts questions of exercise 3.1 of class 6 Maths is based?
Questions of exercise 3.1 of class 6th Maths is based on the following concepts:
- Factors and Multiples.
- The factor of a given number is an exact divisor of that given number.
- A number is a multiple of each of its factor.
- 1 is a factor of every number.
- Every number is a factor of itself.
- The factors of a given number are less than or equal to the given number.
- The number of factors are finite.
- The multiples of a number are greater than or equal to that number.
- The number of multiples are infinite.
- Every number is a multiple of itself.
- Perfect Number.
Does exercise 3.1 of grade 6 Maths take longer time?
No, exercise 3.1 of grade 6th Maths is not at all lengthy. It is a short exercise. There are only three examples (examples 1, 2, 3) and four questions in exercise 3.1.
Can students finish exercise 3.1 of class 6 Maths rapidly?
Yes, students can finish exercise 3.1 of class 6th Maths rapidly because this exercise has only three examples and four questions. Students need a maximum of 2 days to finish exercise 3.1 of grade 6th Maths if they honestly give 1 hour per day to this chapter. This time can vary because no students can have the same working speed, ability, efficiency, etc.
Are there any questions of exercise 3.1 of grade 6th Maths that have complete chances to come in the exam?
There are only three examples (examples 1, 2, 3) and four questions in exercise 3.1 of class 6th Maths out of which questions 3, 4, and example 2 have complete chances to come in the exam.