NCERT Solutions for Class 6 Maths Chapter 2 Exercise 2.3 in Hindi and English medium prepared for CBSE and State Board Exams. Explore updated NCERT Solutions for Class 6 Maths, Chapter 2, Exercise 2.3 on Whole Numbers in both Hindi and English. Perfect for CBSE and State Board learners at Tiwari Academy.
6th Maths Exercise 2.3 Solution in Hindi and English Medium
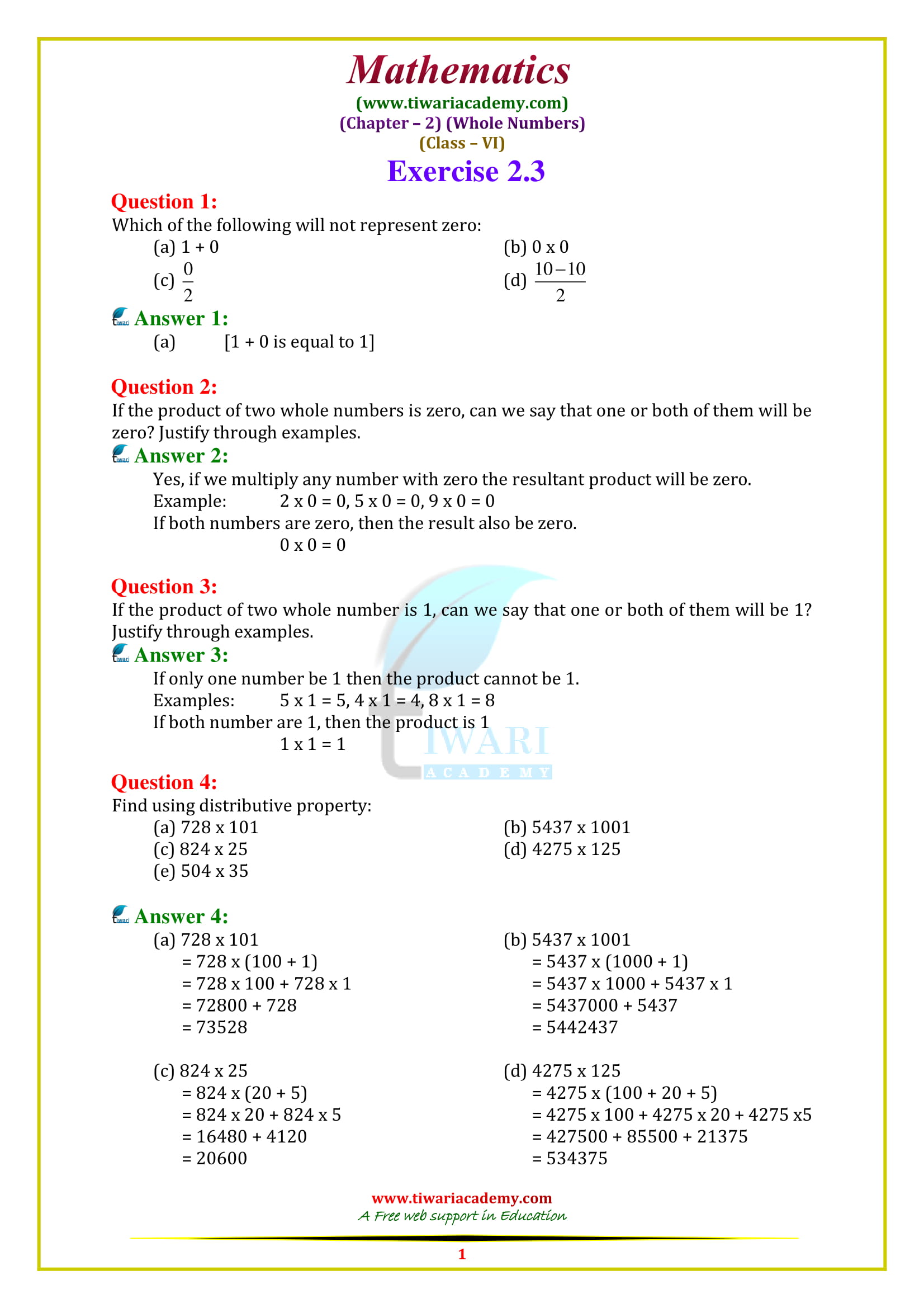
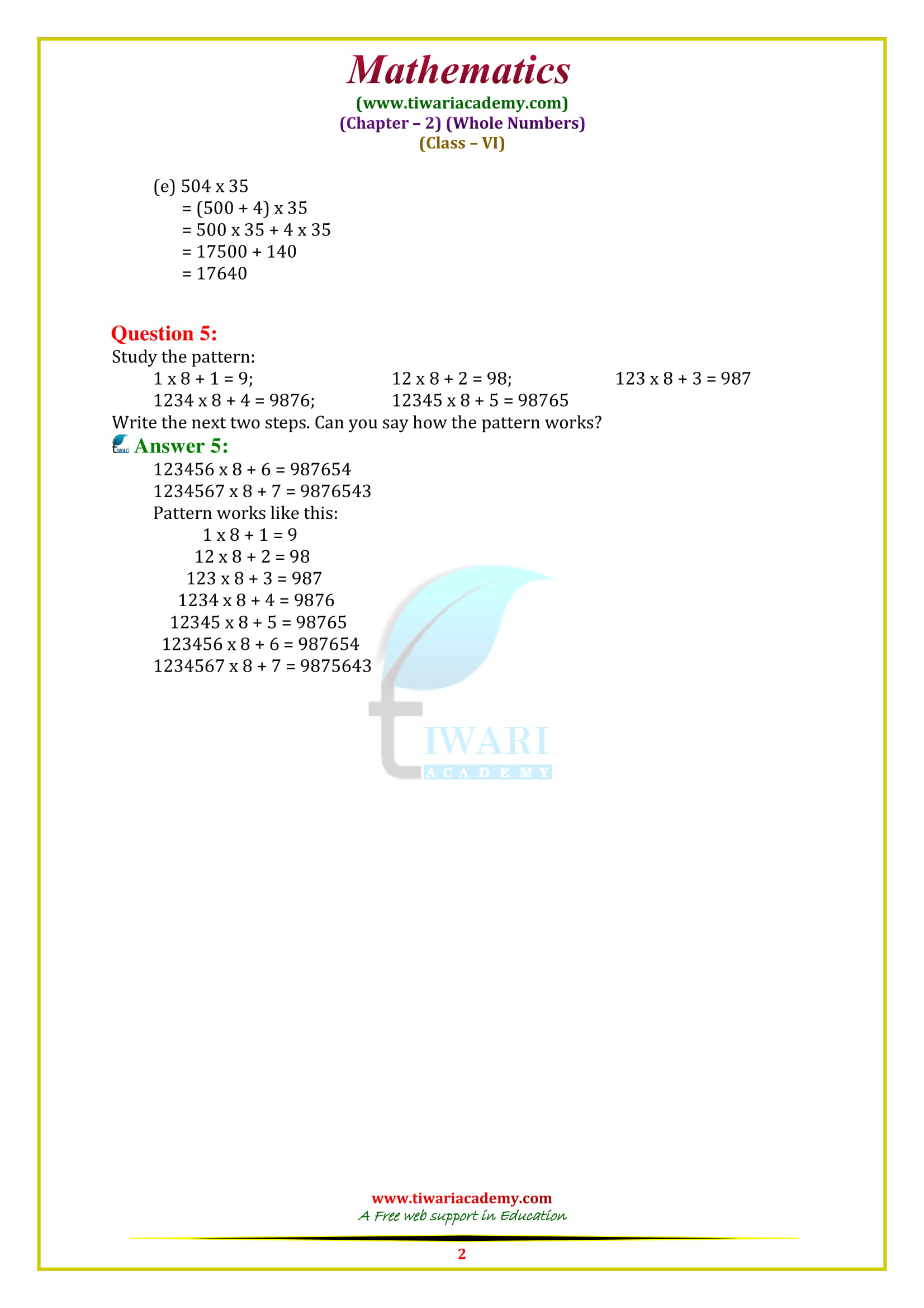
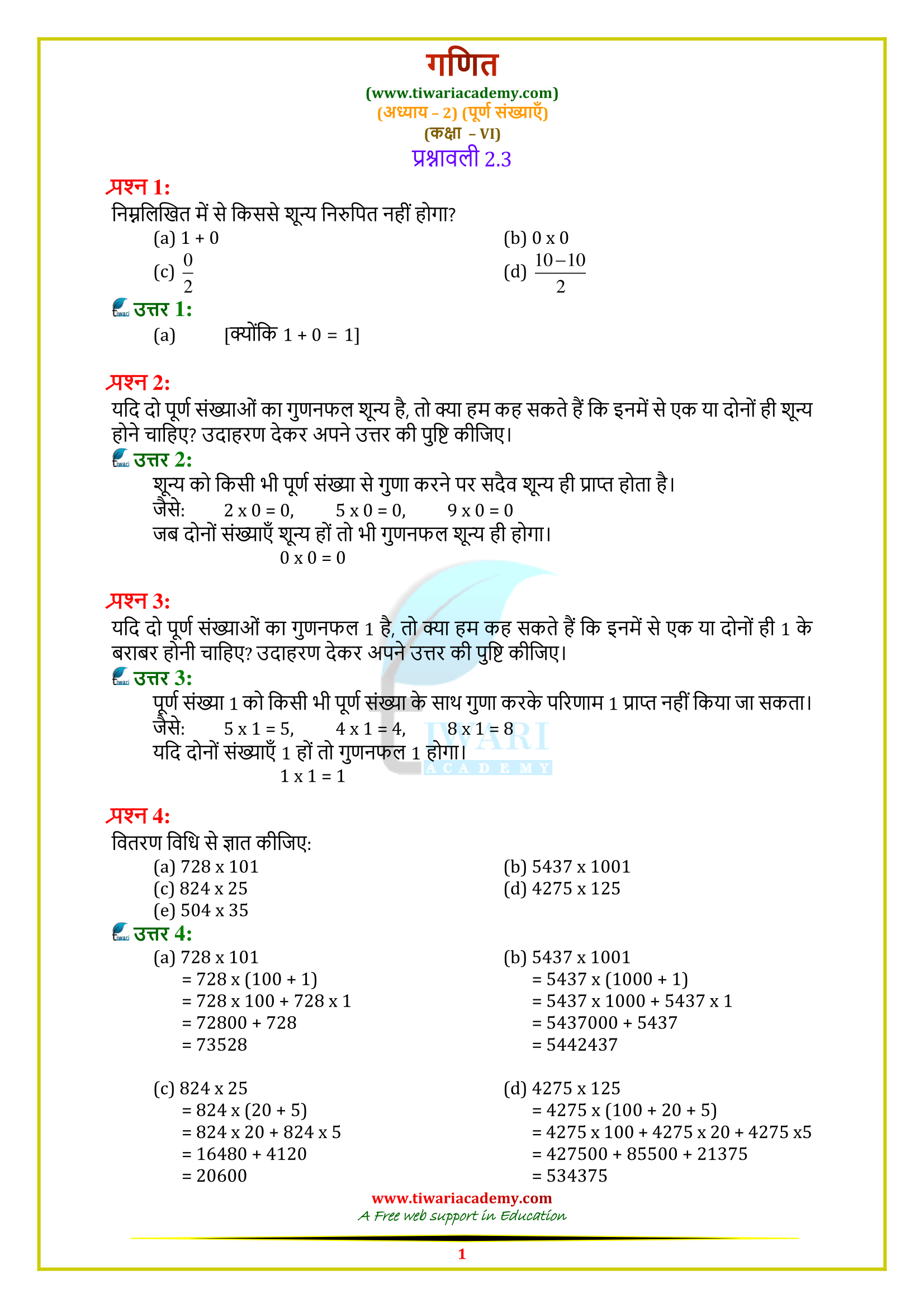
Class 6 Maths Chapter 2 Exercise 2.3 Solution
Class VI Maths textbook Ex. 2.3 of chapter 2 Whole Numbers updated for new academic session 2025-26 free to download or use online. All the contents on Tiwari Academy website are free to use without any login or password. NCERT book solutions Videos related to 6th Maths Exercise 2.3 are also given to use free.
| Class: 6 | Mathematics |
| Chapter: 2 | Exercise: 2.3 |
| Topic Name: | Whole Numbers |
| Medium: | English and Hindi |
| Content Type: | Text and Online Videos |
Properties of Multiplication
Take few pairs of whole numbers and multiply these numbers in two different orders to see whether the product remains the same.
(a × b) = (b × a)
4 × 8 = 32 = 8 × 4 = 32
16 × 9 = 144 = 9 × 16 = 144
25 × 0 = 0 = 0 × 25 = 0
What do we conclude?
We conclude that in whatever order we multiply two whole numbers; the product remains the same. Thus, we have the following property, called the commutative property of multiplication.
Property 2
If a and b are any two whole numbers then a × b = b × a.
Any whole number multiplied by 0 gives the product 0.
Examples, 7 × 0 = 0; 15 × 0 =0, etc.
Thus, we have the following property, called multiplicative property of 0
Property 3
We know that: 6 × 1 = 1 × 6 = 6, 14 × 1 = 1 × 14 = 14, etc.
Thus, any whole number multiplied by 1 gives the number itself.
So, we have the following property called multiplicative property of 1.
Property 4
If a is any whole number, then a × 1 = 1 × a = a.
In order to find the product of three whole numbers, we multiply two of them and multiply this product with the third number.
Example:
Let us take the numbers 2, 3 and 4.
2 × 3 × 4 = (2 × 3) × 4 = 6 × 4 = 24
Changing the arrangement, we have:
2 × 3 × 4 = 2 × (3 × 4) = 2 × 12 = 24.
Thus, (2 × 3) × 4 = 2 × (3 × 4)
What do we conclude?
We conclude that for multiplying whole numbers, we may change the arrangements, but the product remains the same.
In general, we have the following property, called associative property of multiplication.
Property 5
If a, b, c are whole numbers then (a × b) × c = a × (b × c).
Note: We usually write the product of three whole numbers a, b, c as a × b × c. While multiplying three or more numbers, we group them in such a way that the calculation becomes easier. We need not multiply them in the order they are given. We arrange them suitably and multiply.
Class 6 Maths Exercise 2.3 Important Questions
Find the following products: (a) 4 × 220 × 25 (b) 23875 × 25 × 40 × 2
(a) 4 × 220 × 25
= (4 × 25) × 220
= 100 × 220 = 22000
(b) 23875 × 25 × 40 × 2
= (23875 × 2) × (25 × 40)
= 47750 × 1000 = 47750000.
Let us consider the expressions 5 × (8 + 9) and 5 × 8 + 5 × 9.
We have, 5 × (8 + 9) = 5 × 17 = 85 and, 5 × 8 + 5 × 9 = 40 + 45 = 85
Thus, 5 × (8 + 9) = 5 × 8 + 5 × 9
Similarly, we have 10 × (7 + 12) = 10 × 7 + 10 × 12
Find the values of each of the following using various properties of multiplication: (a) 256 × 95 (b) 65 × 72 + 35 × 72 (c) 887 × 10 × 561 – 461 × 8870
(a)256 × 95
= 256 × (100 – 5)
= 256 × 100 – 256 × 5
= 25600 – 1280 = 24320
(b) 65 × 72 + 35 × 72
= (65 + 35) × 72
= 100 × 72 = 7200
(c) 887 × 10 × 561 – 461 × 8870
= (887 × 10) × 561 – 461 × 8870
= 8870 × 561 – 8870 × 461
= 8870 × (561 – 461)
= 8870 × 100
= 887000.
Is the product of an even whole number and an odd whole number, an odd whole number?
Let a is even number = 4
B is odd number = 7
Now a x b = 4 x 7
= 28
Hence, the product of an even whole number and an odd whole number is always even number.
The product of two whole numbers is zero. What do you conclude?
product of two whole numbers is zero, this means one number should be zero.
Thus, we have the following result, called the distributive property of multiplication over addition. Property 6: If a, b, c are whole numbers then a × (b + c) = a × b + a × c. The above property can also be verified over subtraction.
Thus, if a, b, c are any three whole numbers such that b > c, then a × (b – c) = a × b – a × c.
Division
In the earlier classes, we have learnt how to divide a whole number by a smaller non-zero whole number. In this section, we shall discuss division as the inverse of multiplication and as a repeated subtraction.
Division as the Inverse of Multiplication
Let a and b be two whole numbers. Then dividing a by b is same as finding a whole number c which when multiplied by b gives a. Symbolically, we write it as a ÷ b = c Þ a = b × c.
For example, dividing 48 by 8 is same as finding a whole number which when multiplied by 8 gives 48. Clearly, such number is 6.
So, we write 48 ÷ 8 = 6.
Division as Repeated Subtraction
Let a and b be two whole numbers. Then dividing the whole number, a by whole number b is same as determining a whole number c such that when b is subtracted c times from a, we arrive at 0.
Symbolically, we write it as: a ÷ b = c
For example, in order to divide 20 by 4, we subtract 4 repeatedly from 20 till we arrive at 0.
20 – 4 (First time)
16 – 4 (Second time)
12 – 4 (Third time)
8 – 4 (Fourth time)
4 – 4 (Fifth time) 0
Note:
1. If a and b are two whole numbers such that a < b, then we cannot subtract b from a.Thus, a smaller whole number cannot be divided by another large whole number.
2. If a is a non-zero whole number, then by subtracting 0 repeatedly from a we can never arrive at 0. So, division by 0 is not defined.
Properties of Division
Property 1:
If a and b are two positive whole numbers, then a ÷ b is not necessarily a whole number.
Example: Let us divide 26 by 5.
We find that there is no whole number which when multiplied by 5 gives 26.
So, 26 ÷ 5 is not a whole number
Property 2:
If a is a whole number then a ÷ 1 = a
In other words, any whole number divided by 1 gives the quotient as the number itself.
Example: We know that
1 × 2 = 2
2 ÷ 1 = 2
1 × 6 = 6
6 ÷ 1 = 6
1 × 12 = 12
12 ÷ 1 = 12 etc.
Property 3:
In other words, any whole number (other than zero) divided by itself gives quotient 1.
Example: We know that
7 = 7 × 1
7 ÷ 7 = 1
13 = 13 × 1
13 ÷ 13 = 1
29 = 29 × 1
29 ÷ 29 = 1 etc.
Class 6 Maths Exercise 2.3 Extra Questions with Answers
What is the commutative property of multiplication?
In whatever order we multiply two whole numbers; the product remains the same. Thus, we have the following property, called the commutative property of multiplication.
Examples:
4 × 8 = 32 = 8 × 4 = 32
16 × 9 = 144 = 9 × 16 = 144
Find the largest 4-digit number which is exactly divisible by 29.
Largest 4-digit number is 9999, when dividing it by 29 the quotient is 344 and remainder is 23.
Hence, largest 4-digit number which divided completely by 29 is 9999 – 23 = 9976
Does there exist a whole number n such that n ÷ n = n?
Yes, let n = 1
Then 1 ÷ 1 = 1
what do you mean by distributive property of multiplication over addition?
we have the following result, called the distributive property of multiplication over addition. Property 6: If a, b, c are whole numbers then a × (b + c) = a × b + a × c. The above property can also be verified over subtraction.
Thus, if a, b, c are any three whole numbers such that b > c, then a × (b – c) = a × b – a × c.
Example:
Is it necessary that division of a whole number by other whole number the quotient is always a whole number?
No, this is not true, for example, If a and b are two positive whole numbers, then a ÷ b is not necessarily a whole number.
Let us divide 26 by 5.
We find that there is no whole number which when multiplied by 5 gives 26.
So, 26 ÷ 5 is not a whole number.
Property 4:
Zero divided by any whole number (other than zero) gives the quotient as zero.
In other words, if a is a whole number (other than zero) then 0 ÷ a = 0.
Example: We know that
0 × 4 = 0
0 ÷ 4 = 0
0 × 9 = 0
0 ÷ 9 = 0
0 × 23 = 0
0 ÷ 23 = 0 etc
Property 5:
division by zero is not defined.
Example: Let us divide 6 by 0. In order to do this division, we must find a whole number which when multiplied by 0 gives 6. Clearly, no such number can be obtained. We therefore, say that division by 0 is not defined.
Is exercise 2.3 of grade 6th Maths lengthy?
No, exercise 2.3 of grade 6th Maths is not at all lengthy. Exercise 2.3 is the shortest exercise compared to other exercises of chapter 2 of grade 6th Maths. Exercise 2.3 has only 5 questions and no example. Question 1 has 4 parts, and question 4 has five parts.
Which sums of exercise 2.3 of class 6th Maths are expected for the school exams?
Exercise 2.3 of grade 6th Maths has only 5 questions. All the sums of this exercise are important and can come in the school exams, but the most important problems of exercise 2.3 of class 6th Maths that have more chance to come in the school exams are questions 3, 4, 5.
In how much time will the teacher finish exercise 2.3 of class 6th Maths?
Exercise 2.3 of grade 6th Maths has only 5 questions. The teacher needs a maximum of 1 hour to finish exercise 2.3 of class 6th Maths. This time is not fixed. This time can vary because no teachers and students can have the same working speed.
Is exercise 2.3 of class 6th Maths complex to understand?
No, exercise 2.3 of class 6th Maths is not at all complex. It is the easiest exercise. All students can easily solve exercise 2.3. Only in question 5 of exercise 2.3 of class 6th Maths students face a little difficulty.