NCERT Solutions for Class 6 Maths Chapter 2 Exercise 2.2 Whole Numbers in Hindi and English Medium updated for CBSE and State Board Students. Discover up-to-date NCERT Solutions for Class 6 Maths Ch 2 Exercise 2.2 on Whole Numbers in both Hindi and English. Tailored for CBSE and State Board students, available at Tiwari Academy.
6th Maths Exercise 2.2 Solution in Hindi and English Medium
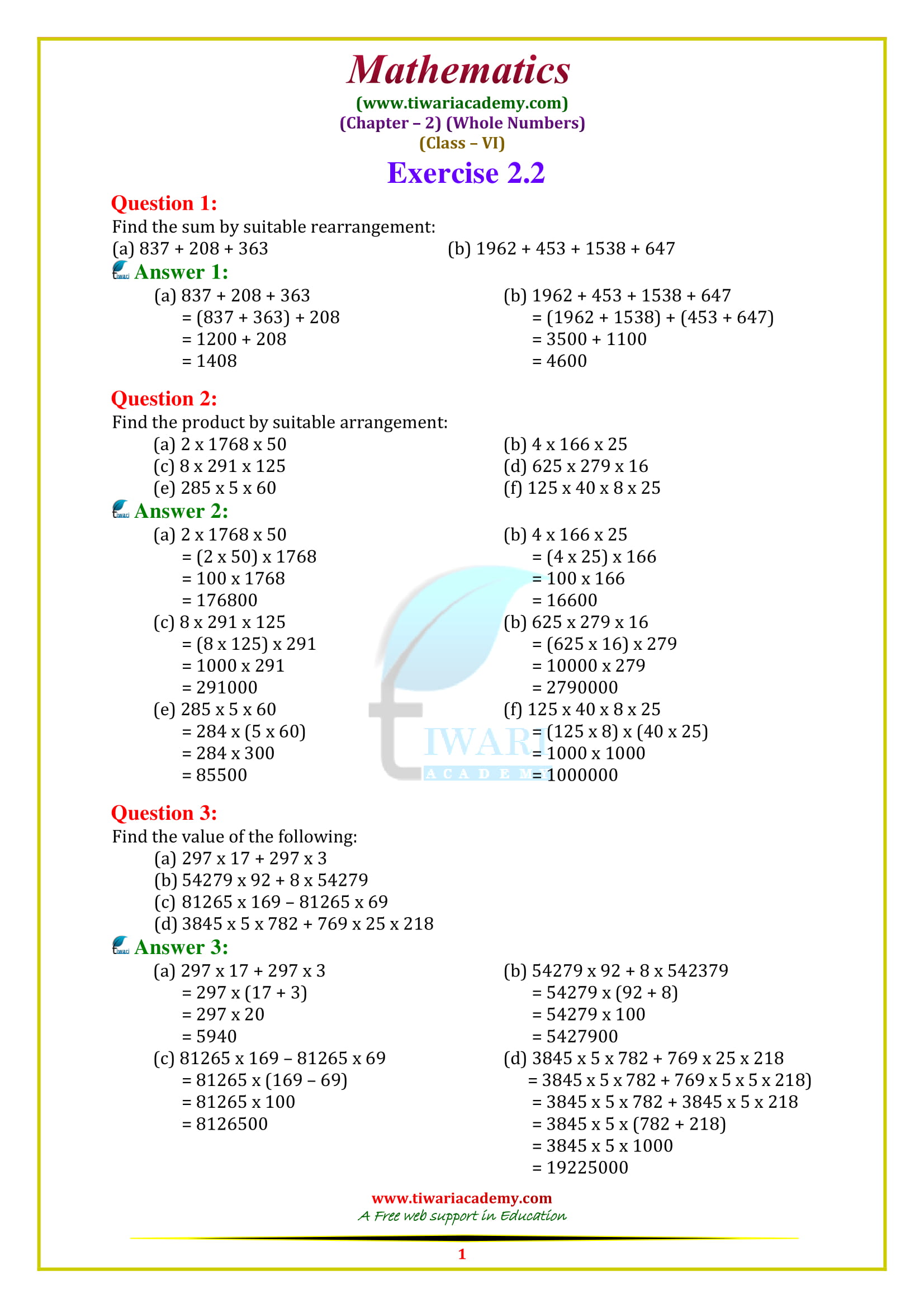
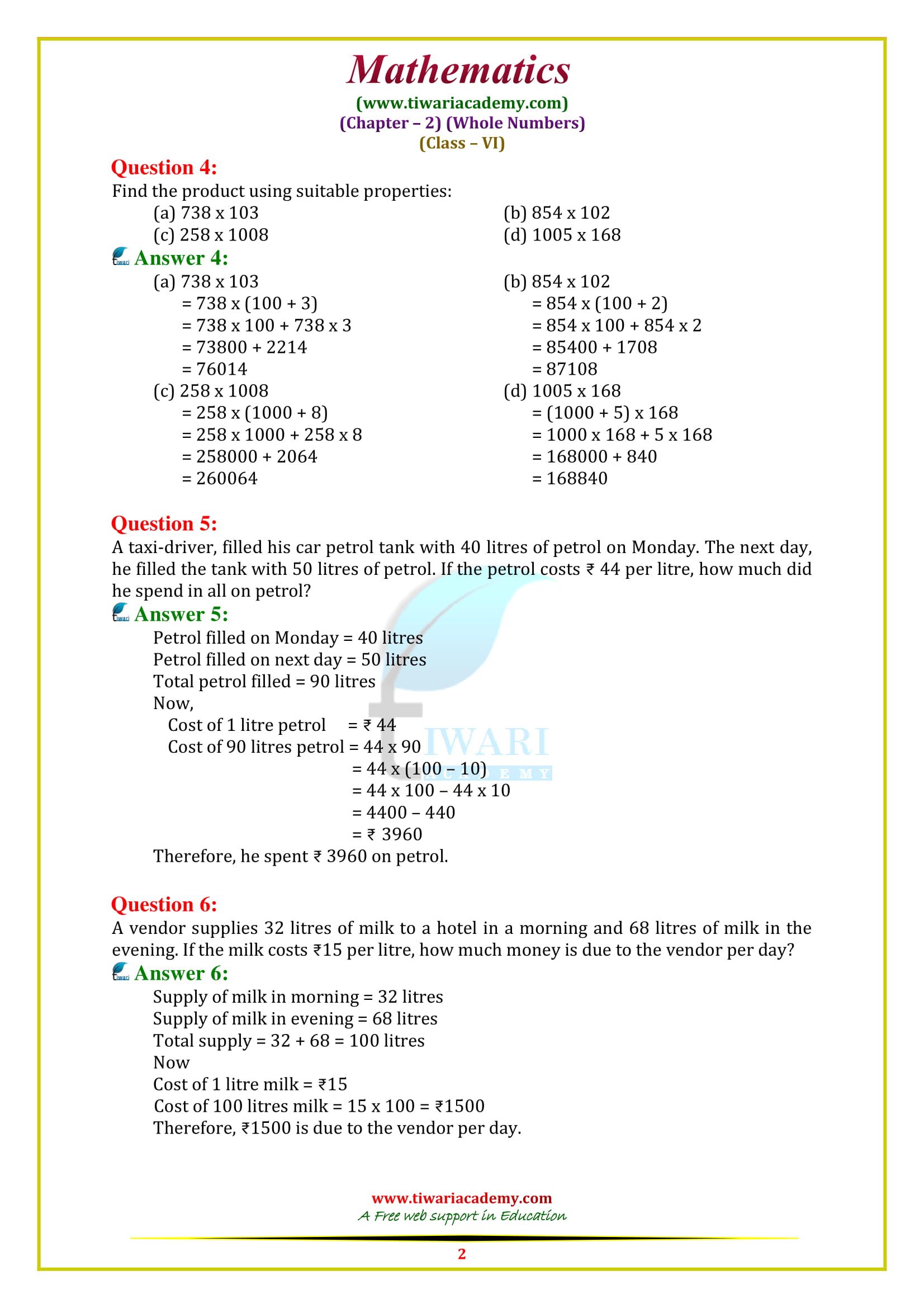
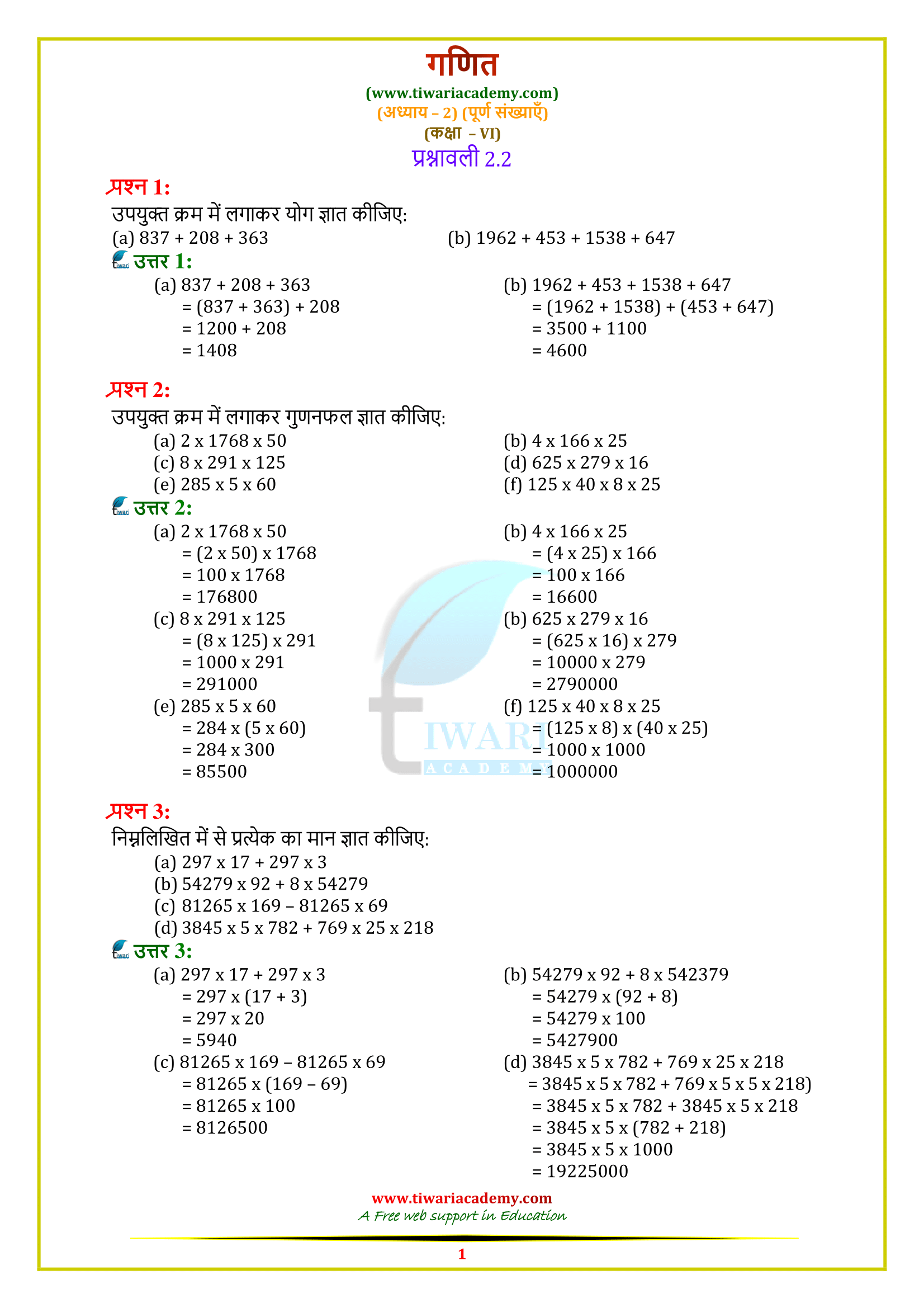
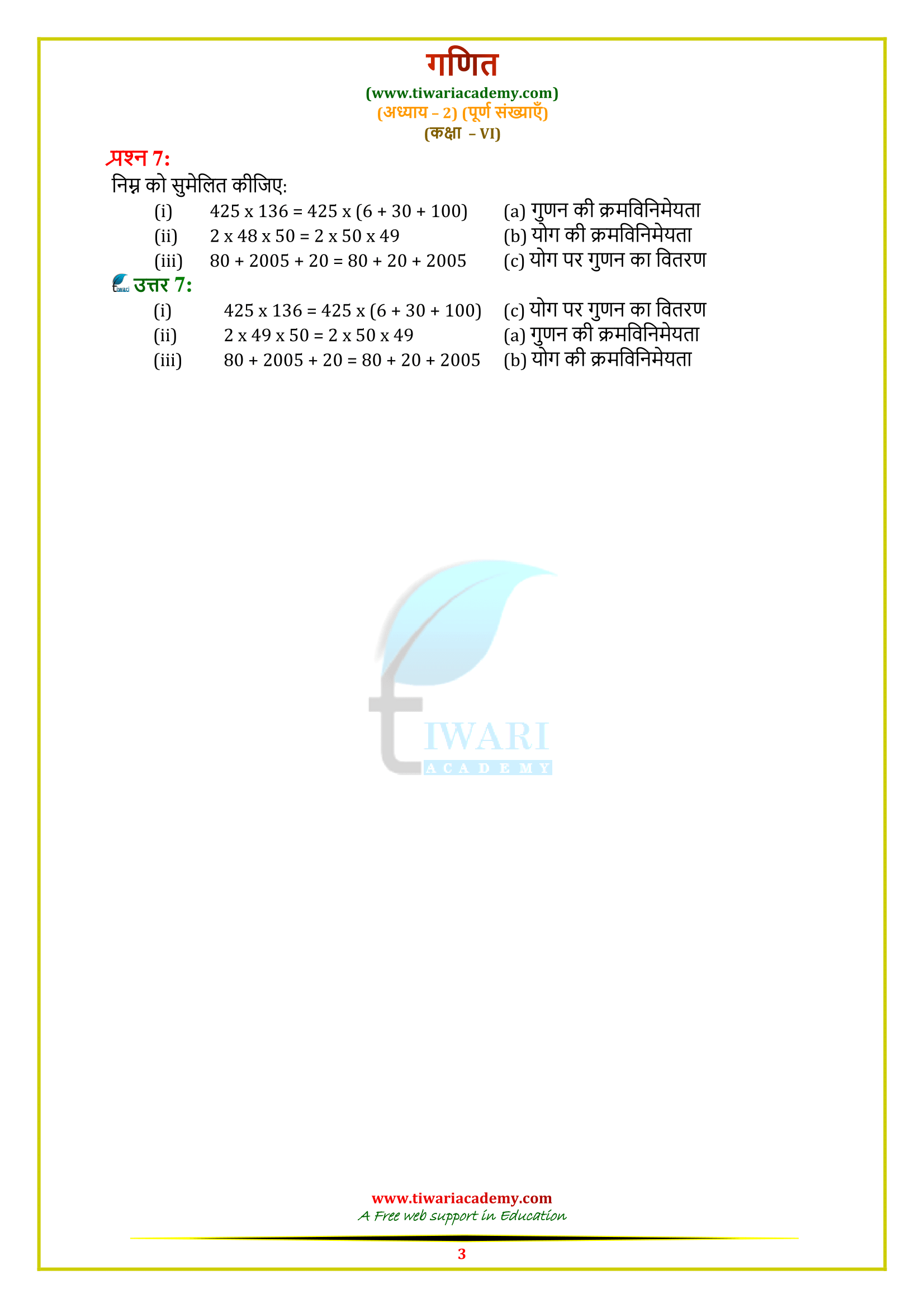
Class 6 Maths Chapter 2 Exercise 2.2 Solution
Class VI Mathematics NCERT textbook Ex. 2.2 of chapter 2 Whole Numbers in PDF file format free to download or use online without any login or password. All the Maths solution is updated for CBSE current academic session providing the best way to solve the questions. Class 6 Maths NCERT solutions are useful for the students studying in state board also.
| Class: 6 | Mathematics |
| Chapter: 2 | Exercise: 2.2 |
| Chapter Name: | Whole Numbers |
| Medium: | Hindi and English |
| Content: | NCERT Exercise Solutions |
Subtraction
We know that subtraction is the inverse process of addition. It means that subtracting 13 from 27 is the same as finding a whole number which when added to 13 gives 27.
Thus, 27 – 13 = ….is the same as …… + 13 = 27
i.e., 27 – 13 = 14 is the same as 14 + 13 = 27
Similarly, 33 – 16 = 17 is the same as 17 + 16 = 33.
Let us see what happens when we subtract 38 from 25. Can you find a whole number which when added to 38 gives 25? No.
Thus, we cannot subtract a greater whole number from a smaller whole number.
Properties of Subtraction
If we subtract two equal whole numbers, we get the whole number 0.
Examples: 5 – 5 = 0, 9 – 9 = 0, 14 – 14 = 0, etc.
Again, if we subtract a smaller whole number from a larger one, the result is clearly a whole number. Examples: 9 – 7 = 2, 10 – 6 = 4, 19 – 16 = 3, etc.
But, what happens if we subtract 14 from 7?
Clearly, there is no whole number which when added to 14, gives 7.
So, (7 – 14) is not a whole number.
Property 1:
If a and b are two whole numbers such that a > b or a = b then a – b is a whole number, otherwise subtraction is not possible in whole numbers.
Let us now examine whether the property 2 of addition holds good for subtraction.
We know that 9 – 3 = 6 but 3 – 9 is not possible in whole numbers.
Again, 18 – 5 = 13 but 13 – 18 is not possible in whole numbers.
Property 2:
If a and b are two whole numbers then, in general, a – b is not equal to b – a.
Let us see whether the property 3 of addition holds good for subtraction.
We know that, 3 – 0 = 3 but 0 – 3 is not defined in whole numbers; 9 – 0 = 9 but 0 – 9 is not defined in whole numbers.
Property 3:
If a is any whole number, then a – 0 = a but 0 – a is not defined in whole numbers.
Let us now examine whether the property 4 of addition holds good for subtraction.
Take three numbers, say 5, 2, 1.
We have, (5 – 2) – 1 = 3 – 1 = 2 and 5 – (2 – 1) = 5 – 1 = 4. Thus, (5 – 2) – 1 is not equal to 5 – (2 – 1).
This shows that the associative law is not applicable to subtraction on whole numbers.
Property 4:
If a, b, c are three whole numbers then, in general, (a – b) – c is not equal to a – (b – c).
Class 6 Maths Exercise 2.2 Important Questions
What is the difference between the largest number of 6-digit and the smallest number of 7-digit?
Largest 6 digits’ number is 999999
Smallest 7 digits’ number is 1000000
Difference = 1000000 – 999999 = 1
The population of a town is 98917. If the number of men is 30642 and that of women is 29622, determine the number of children.
Total population of town = 98917
Number of men = 30642
Number of women = 29622
So number of children = total population – (number of men + number of women)
= 98917 – (30642 + 29622)
= 38653
The digits 9 and 3 of a number 49813 are interchanged. Find the difference between the original number and the new number.
After interchanging digit 9 and 3 we get new number 43819
Now difference of 49813 and 43819 is
49813 – 43819 = 5994
Multiplication
We know that multiplication is a repeated addition. Instead of adding the same number many times, we multiply it by the number equal to the number of times it is added. For example, if we have to add 4 six times. We add like this: 4 + 4 + 4 + 4 + 4 + 4 = 24. This may be written as 4 × 6 = 24, which is read as 4 taken 6 times is equal to 24 or 4 multiplied by 6 is 24. If the numbers to be multiplied are small, we can perform the operation mentally and find the product. But if the numbers are large we use the multiplication tables to find the product as you have learnt in the earlier classes.
Properties of Multiplication
Let us take a few pairs of whole numbers and check in each case whether their product is a whole number.
Class 6 Maths Exercise 2.2 Extra Questions with Answers
What do you mean by Subtraction?
We know that subtraction is the inverse process of addition. It means that subtracting 13 from 27 is the same as finding a whole number which when added to 13 gives 27.
Thus, 27 – 13 = ….is the same as …… + 13 = 27
i.e., 27 – 13 = 14 is the same as 14 + 13 = 27
Is the a – b = b – a?
Let a = 7 and b = 9 (where a and b are two whole numbers) then, in general, a – b is not equal to b – a.
7 – 9 = -2 and 9 – 7 = 2 both are not same
Hence, a – b is not equal to b – a
What is the difference between the largest number of 4-digit and the smallest number of 4-digit?
Largest 4-digit number is 9999
Smallest 4-digit number is 1000
So difference is 9999 – 1000 = 8999
| Multiplication of two whole numbers | Product | Is the product a whole number |
|---|---|---|
| 5 x 6 | 30 | Yes |
| 8 x 9 | 72 | Yes |
| 12 x 0 | 0 | Yes |
| 15 x 1 | 15 | Yes |
What do we conclude?
We conclude that if we multiply two whole numbers, the product is also a whole number.
So, we have the following property, called the closure property of multiplication.
How many properties of the whole number are there in exercise 2.2 of class 6th Maths?
In exercise 2.2 of class 6th Maths, there are four properties of whole numbers.
The five properties of whole numbers are:
- Closure property.
- Commutative property.
- Associative property.
- Distributive property.
- Identity.
Are there any important example in exercise 2.2 of grade 6th Maths?
Yes, there are seven examples in exercise 2.2 of grade 6th Maths. All examples are easy and are solved using properties (closure property, commutative property, associative property, distributive property, identity) mentioned in section 2.4 of chapter 2 of class 6th Maths.
Which questions of exercise 2.2 of class 6th Maths, the teacher use to give in the exams?
Exercise 2.2 of class 6th Maths has seven examples and seven questions. The most important problems of this exercise are questions 3, 4, 6, and 7 and examples 4, 5. These questions and examples have a chance to come in the exams.
Is exercise 2.2 of class 6th Maths easy to understand and solve?
Exercise 2.2 of class 6th Maths is quite easy to understand and solve. This exercise is enjoyable and logical. Once students understand how to solve exercise 2.2, then they rapidly solve this exercise.