NCERT Solutions for Class 6 Maths Chapter 2 Exercise 2.1 in Hindi and English Medium modified and updated for academic year 2025-26 exams. As per the new rationalised syllabus of 6th Maths, there are only one exercise in chapter 2, that is, ex. 2.1 for session 2025-26.
6th Maths Exercise 2.1 Solution in Hindi and English Medium
| Class: 6 | Mathematics |
| Chapter: 2 | Exercise: 2.1 |
| Chapter Name: | Whole Numbers |
| Medium: | Hindi and English medium |
| Session: | CBSE 2025-26 |
| Content Type: | Text, Images and Videos |
Class 6 Maths Chapter 2 Exercise 2.1 Solution
Class VI Mathematics NCERT book Ex. 2.1 of chapter 2 Whole Numbers in Hindi and English Medium updated for new session 2025-26 free to download. Student can use NCERT class 6 Maths Ex. 2.1 solution online or download in PDF file format free. Videos related to exercise 2.1 is also given to explain better.
Natural Numbers
Since our childhood we are familiar with counting numbers 1, 2, 3, …. These are known as natural numbers.
Whole Numbers
All natural numbers along with the number zero form the collection of. That is numbers 0, 1, 2, 3, 4, … are called whole numbers. Clearly, every natural number is a whole number but 0 is a whole number which is not a natural number.
There is no last or greatest whole number.
There are infinitely many whole numbers.
Successor and Predecessor of A Whole Number
The successor of a whole number is the number obtained by adding 1 to it. Clearly, the successor of 0 is 1; the successor of 1 is 2; the successor of 2 is 3. Every whole number has its successor.
Predecessor
The predecessor of a whole number is 1 less than the given number. Clearly, the predecessor of 1 is 0; the predecessor of 2 is 1; the predecessor of 3 is 2. Every whole number (except zero) has its predecessor.
Important Questions on Class 6 Maths Exercise 2.1
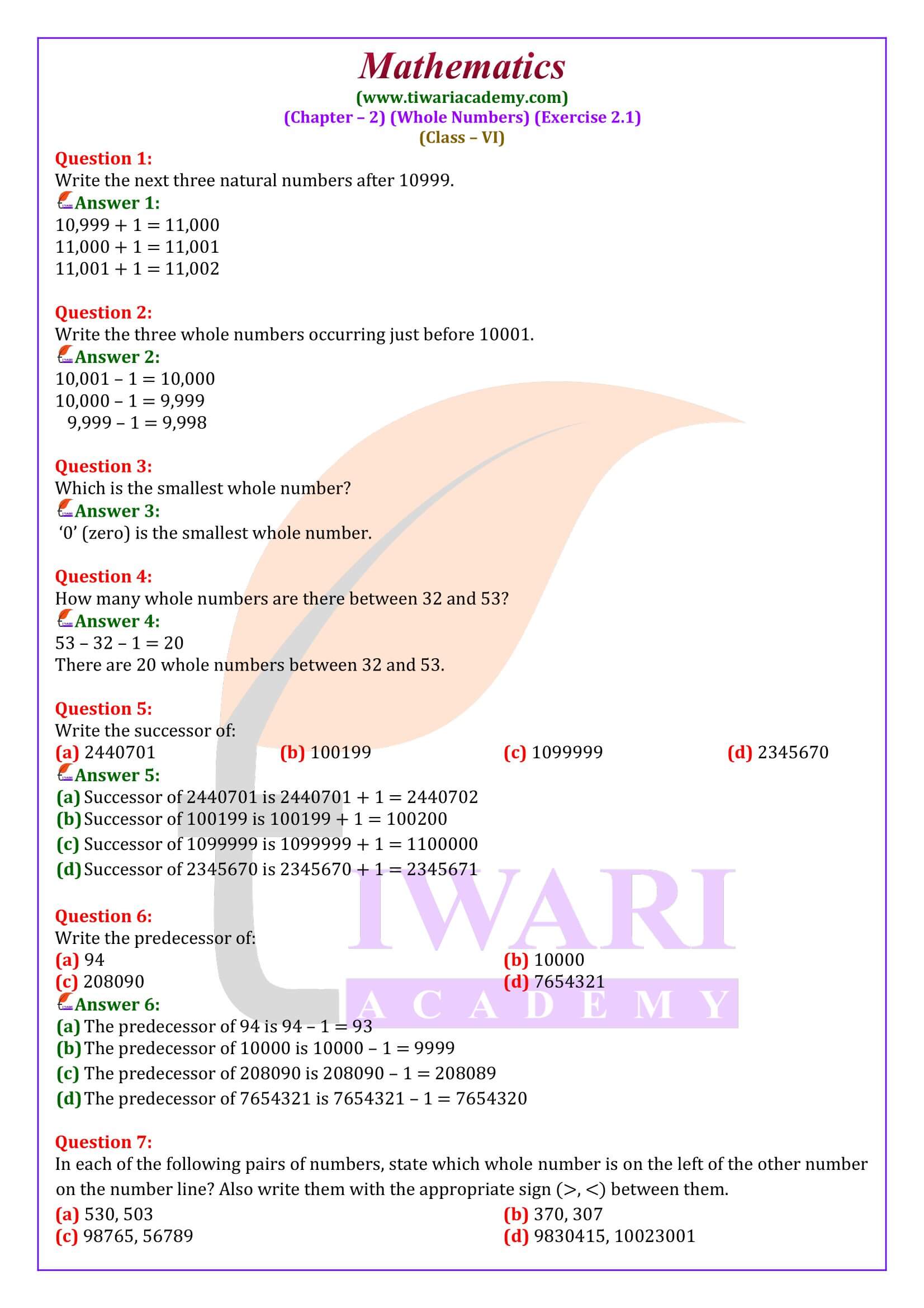
Write the successor and predecessor of: (a) 1000, (b) 999999, (c) 2005499
(a) The successor of 1000 = (1000 + 1) = 1001.
The predecessor 1000 = (1000 – 1) = 999.
(b) The successor of 999999 = (999999 + 1) = 1000000.
The predecessor of 999999 = (999999 – 1) = 999998.
(c) The successor of 2005499 = (2005499 + 1) = 2005500.
The predecessor of 2005499 = (2005499 – 1) = 2005498.
Is the sum of two whole numbers in different order same? (a) 5 + 7 and 7 + 5, (b) 18 + 11 and 11 + 18, (c) 5 + 39 and 39 + 5
(a) 5 + 7 = 12 and 7 + 5 = 12
(b) 18 + 11 = 29 and 11 + 18 = 29
(c) 5 + 39 = 44 and 39 + 5 = 44
Find the sum of 148 + 205 + 152.
We observe that the sum of 148 and 152 is 300, which is a multiple of 100.So, we first add these two numbers and then the result is added to 205 to find the required sum as under:
148 + 205 + 152 = 205 + (148 + 152) = 205 + 300 = 505.
Add together, 27, 103, 373, 297 by convenient grouping.
27 + 103 + 373 + 297 = (27 + 373) + (103 + 297)
= 400 + 400 = 800.
Representation of Whole Numbers On Number Line
We can represent whole numbers by points on a line. In order to represent whole numbers on a number line, we draw a straight line and mark a point O on it. Starting from O, mark points A, B, C, D, E, F etc., on the line at equal distances to the right of O.

Label the point O as 0. Let, OA = 1 unit.
Then AB = BC = CD = DE = EF = 1 unit.
Now, OB = OA + AB = 1 + 1 = 2 units
OC = OB + BC = 2 + 1 = 3 units
OD = OC + CD = 3 + 1 = 4 units and so on.
We note the following facts about whole numbers:
- The number line is endless and every whole number can be represented on it.
- There is no whole number to the left of 0. Every whole number on the right of 0 is greater than 0.
- Thus, 0 is the smallest whole number.
- Every whole number on the number line is greater than every other whole number on its left.
Example: 4 > 3, 25 > 24, 1 > 0. Thus, there is no longer whole number. - Every whole number on the number line is less than every other whole number on its right.
Examples: 0 < 1, 2 < 3, 19 < 20, 85 < 90. - Between any two consecutive whole numbers, there is no whole number. However, between two non-consecutive whole numbers, there always exists a whole number.
Example: 6 lies between 3 and 8. Here, 3 < 6 and 6 < 8. We can express this as 3 < 6 < 7. Also 4, 5, 6, 7 lie between 3 and 8.
Properties of Whole Numbers
We are already familiar with the four basic operations of addition, subtraction, multiplication and division on whole numbers. In this chapter, we shall study the properties of these operations on whole numbers.
Properties of Addition
| Addition of Two Whole Numbers | Sum | Is the Sum a Whole Number |
|---|---|---|
| 6 + 3 | 9 | Yes |
| 8 + 5 | 13 | Yes |
| 5 + 0 | 5 | Yes |
| 17 + 9 | 26 | Yes |
| 53 + 27 | 80 | Yes |
What do we conclude? We conclude that the sum of any two whole numbers is a whole number.
Thus, we have the following property, known as the closure property of addition.
Property 1:
If a and b are any two whole numbers, then a + b is also a whole number.
Let us take a pair of whole numbers. Now, add them in two different orders and see whether the sum remains the same. Repeat it with more pairs.
What do we conclude? We conclude that in whatever order we add two whole numbers; the sum remains the same.
Thus, we have the following property, called the commutative law of addition.
Property 2:
If a and b are any two whole numbers then a + b = b + a.
Take any whole numbers and add them to zero as under:
Example:
- (a) 5 + 0 = 0 + 5 = 5,
- (b) 12 + 0 = 0 + 12 = 12,
- (c) 19 + 0 = 0 + 19 = 19.
What do we observe? We conclude that the sum of any whole number and 0 is the number itself.
This property is called the additive property of zero.
Property 3:
If a is any whole number then a + 0 = 0 + a = a
To find the sum of three whole numbers, we add two of them and add the third number to the sum.
Let us take three whole numbers, say 4, 7, 8.
(4 + 7) + 8 = 11 + 8 = 19; and 4 + (7 + 8) = 4 + 15 = 19.
Thus, (4 + 7) + 8 = 4 + (7 + 8).
Again, take three more numbers, say 5, 9, 13.
(5 + 9) + 13 = 14 + 13 = 27; and 5 + (9 + 13) = 5 + 22 = 27.
Thus, (5 + 9) + 13 = 5 + (9 + 13).
We thus find that in the addition of whole numbers, the manner of associating the numbers does not affect the sum.
So, we have the following property, called the associative law of addition.
Class 6 Maths Exercise 2.1 Extra Questions with answers
What do you mean by whole number?
All natural numbers along with the number zero form the collection of. That is numbers 0, 1, 2, 3, 4, … are called whole numbers. Clearly, every natural number is a whole number but 0 is a whole number which is not a natural number.
write the rules of successor and predecessor.
Successor: The successor of a whole number is the number obtained by adding 1 to it. Clearly, the successor of 0 is 1; the successor of 1 is 2; the successor of 2 is 3. Every whole number has its successor.
Predecessor: The predecessor of a whole number is 1 less than the given number. Clearly, the predecessor of 1 is 0; the predecessor of 2 is 1; the predecessor of 3 is 2. Every whole number (except zero) has its predecessor.
if a and b are whole numbers prove that a + b = b + a.
Let a = 6 and b = 8
Then a + b = 6 + 8 = 14
And b + a = 8 + 6 = 14
Hence, a + b = b + a
Property 4:
If a, b, c are any three whole numbers then (a + b) + c = a + (b + c).
While adding three or more numbers, we group them in such a way that the calculation becomes easier. We need not add them in the given order.
Is exercise 2.1 of class 6th Maths lengthy?
Exercise 2.1 of class 6th Maths contains only eight questions and no examples. So, exercise 2.1 of class 6th Maths is not lengthy. It is a short exercise. Short answer-type questions (1 mark or 2 marks) can come from this exercise in the exams.
Are there any questions in exercise 2.1 of class 6th Maths that teacher can give in the exams?
There are three questions in exercise 2.1 of class 6th Maths that teacher can give in the exams. These questions are questions 3, 7, and 8. In question 3, students have to tell the smallest whole number. In question 7, 4 pairs of numbers are given and students have to state which whole number is on the left of the other number on the number line. Also, write them with the appropriate sign (>,<) between them. In question 8, students have to tell which statement is true and which is false.
Is exercise 2.1 of class 6 Maths simple to solve and understand?
Yes, exercise 2.1 of class 6th Maths is very simple to solve and understand. Students enjoy doing this exercise. Students can also solve this exercise without using pen and paper means students can solve this exercise verbally.
Is it possible to complete exercise 2.1 of class 6th Maths within one or two hours?
Yes, it is possible to complete exercise 2.1 of class 6th Maths within 1 hour because this exercise is short and easy. But, if concepts based on this exercise are not clear to students, they can’t complete exercise 2.1 of class 6th Maths within 1 hour. To finish this exercise within 1 hour, students must know all the concepts based on this exercise.