NCERT Solutions for Class 6 Maths Chapter 12 Exercise 12.3 in Hindi and English medium updated for CBSE 2025-26 exams. Questions explanations and solutions are revised according to rationalised syllabus and new NCERT textbooks issued for academic session 2025-26.
6th Maths Exercise 12.3 Solutions in Hindi and English Medium
| Class: 6 | Mathematics |
| Chapter: 12 | Exercise: 12.3 |
| Topic Name: | Ratio and Proportion |
| Mode of content: | PDF and Videos Format |
| Session: | Academic Year 2025-26 |
| Medium: | Dual Language – Hindi and English |
Class 6 Maths Chapter 12 Exercise 12.3 Solution
Class VI Maths NCERT (https://ncert.nic.in/) Ex. 12.3 Ratio and Proportion updated for CBSE and State Board new session. Question answers and solutions of NCERT Books are given in Hindi and English Medium free to use online or download in PDF file format. In CBSE board class 6 math exercise 12.3 questions are based on unitary method. These are important and useful in daily life activity also. Unitary method is important for next classes also.
Class 6 Maths Chapter 12 Exercise 12.3 Solution in Videos
Class 6 Maths Exercise 12.3 Extra Questions with Answer
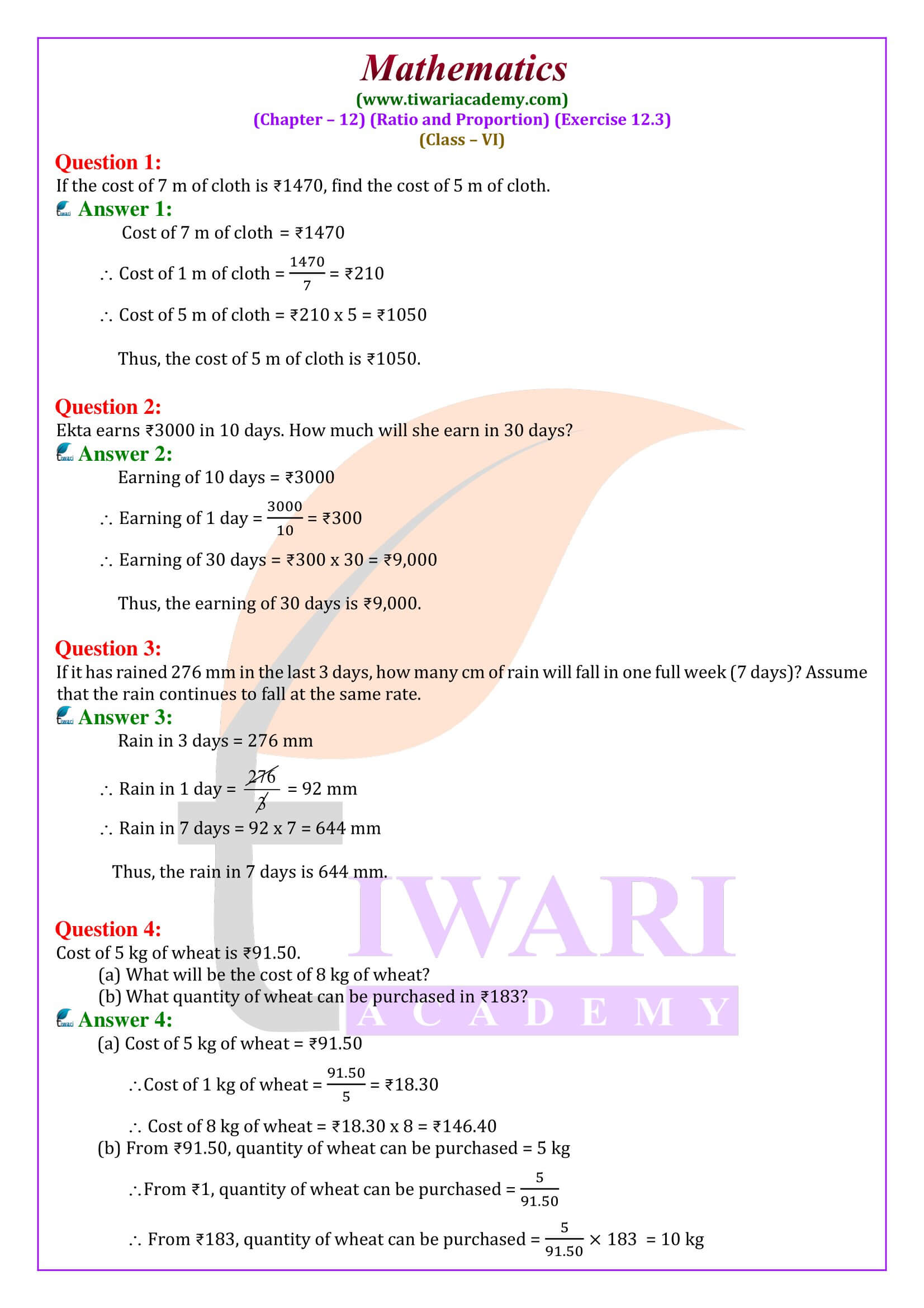
The cost of 4 pens is Rs 28. Find the cost of 7 such pens.
The cost of 4 pens = Rs 28
So, the cost of 1 pen = Rs 28/4 = Rs 7
So, cost of 7 pens = Rs 7 × 7 = Rs 49.
If 11 workers earn Rs 1925 per day, what will be earning of such 28 workers in a day?
Earning of 11 workers in a day = Rs 1925
So, Earning of 1 worker in a day = Rs 1925/11 = Rs 175
So, Earning of 28 workers in a day = Rs 175 × 28 = Rs 4900.
Unitary Method
The method of finding the value of one (unit) article from the value of the given number of articles at first and then the value of the required number of articles is called the unitary method.
We know that less number of articles cost less. So, if the value of any number of articles is given then the value of one article is found by dividing the value of the given number of articles by the given number of articles. In other words,
(value of given number of articles)/ (number of articles)
Class 6 Maths Exercise 12.3 Important Questions
What is unitary method How can we apply it in our daily life?
Unitary method has several uses in our daily life like when we talk about speed, rate or percentage, we invariably relate to unitary method. For instance, when we calculate the average speed of a car, that has traveled 130 kilometers in 2 hours, we end up calculating the distance traveled by the car in 1 hour.
What is unitary method in ratio and proportion?
The order of terms in a proportion is important. For example: 3, 8, 24, 64 are in proportion but 3, 8, 64, 24 are not in proportion. The method in which first we find the value of one unit and then the value of the required number of units is known as unitary method.
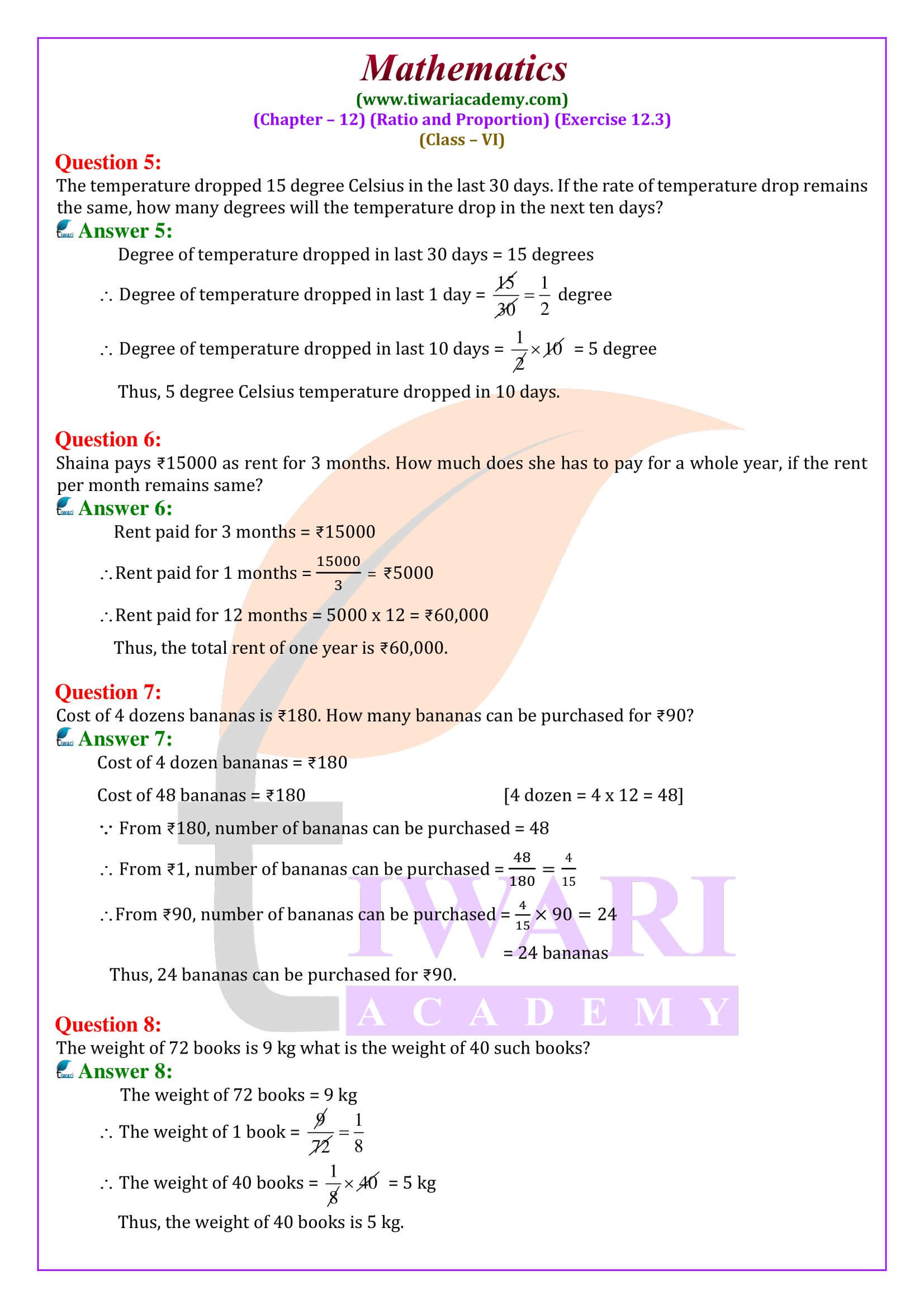
A bike can travel 562 km in 8 litres of petrol. How much distance will it travel in 5 litres of petrol?
In 8 litres of petrol, bike travels = 562 km
So, in 1 litre of petrol, bike will travel = 562/8 km = 70.25 km
So, in 5 litres of petrol, bike will travel = (70.25 × 5) km = 351.25 km
We also know that more articles will cost more. So, if the value of one article is known, then the value of required number of articles is found by multiplying the value of one article by the number of articles. In other words,
value of required number of articles = value of one article × required number of articles
What topic will students study in exercise 12.3 of class 6 Maths?
In exercise 12.3 of class 6th Maths, students study the process of the unitary method (The method in which first we find the value of one unit and then the value of the required number of units is known as the Unitary Method).
How many questions and illustrations are in exercise 12.3 of class 6th Maths?
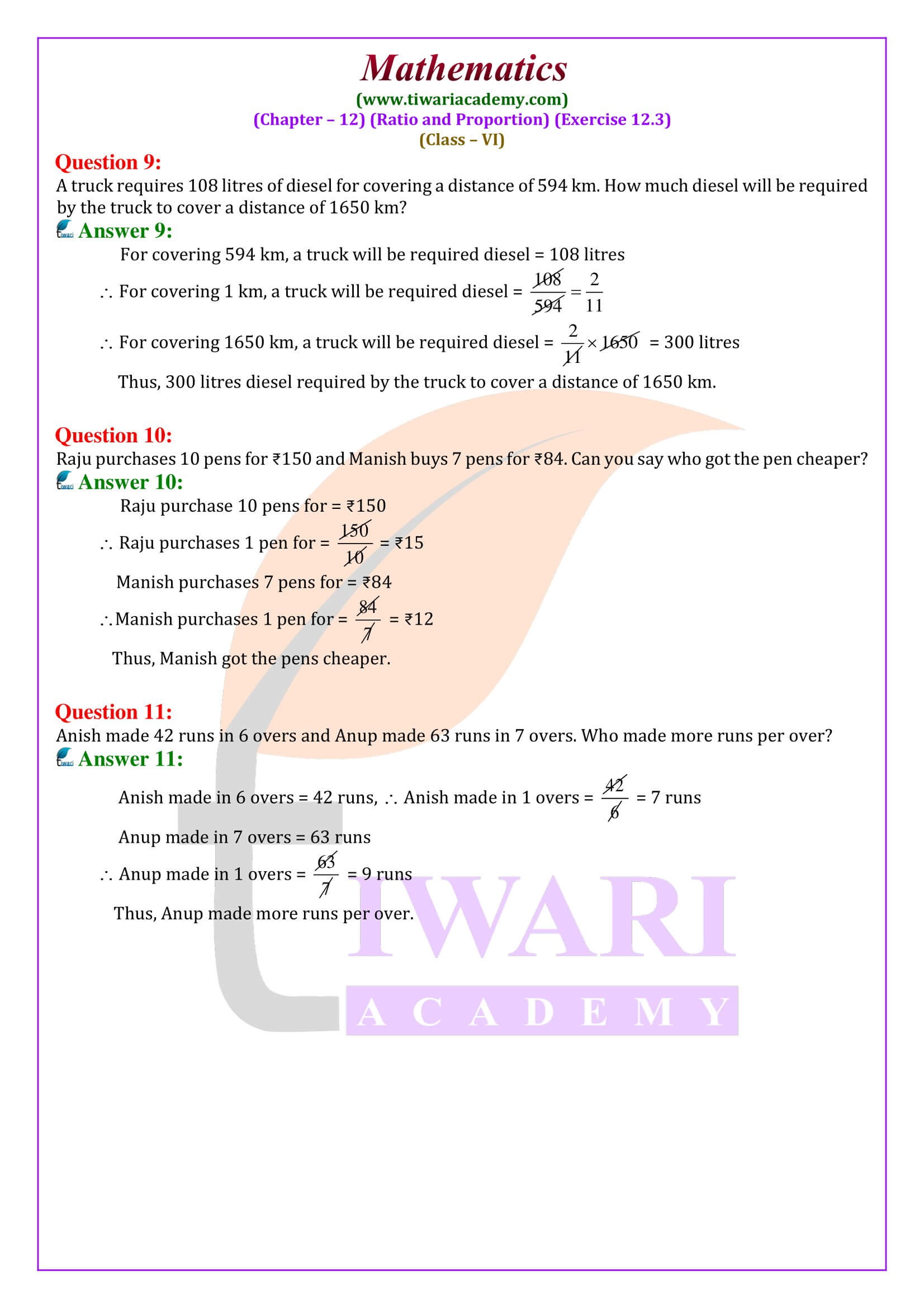
Exercise 12.3 of class 6th Maths has five examples (examples 11, 12, 13, 14, 15) and 11 questions. Examples 13, 15 are important. Questions 7, 9, 11 are important from the exam point of view.
Which problems of exercise 12.3 of 6th standard Maths are tough to understand?
Problems of exercise 12.3 of 6th standard Maths that are tough to understand are example 15 and questions 3, 5, 10, and 11. The languages of these questions are a little tough to understand, according to 6th standard students.
How many days are needed to complete exercise 12.3 of 6th standard Maths?
To complete exercise 12.3 of 6th standard Maths, students need a maximum of 4 days if they give 1-2 hours per day to this exercise because this exercise has 11 questions and 5 examples. This time is an approximate time and can vary, that is, this time can increase or decrease according to the situation.