NCERT Solutions for Class 6 Maths Chapter 1 Exercise 1.3 Knowing Our Numbers in Hindi and English medium updated for CBSE and State board Exams. Ace your exams with NCERT Solutions for Class 6 Maths Ch 1 Exercise 1.3 on Knowing Our Numbers, available in Hindi & English for CBSE and State boards at Tiwari Academy.
6th Maths Exercise 1.3 Solution in Hindi and English medium
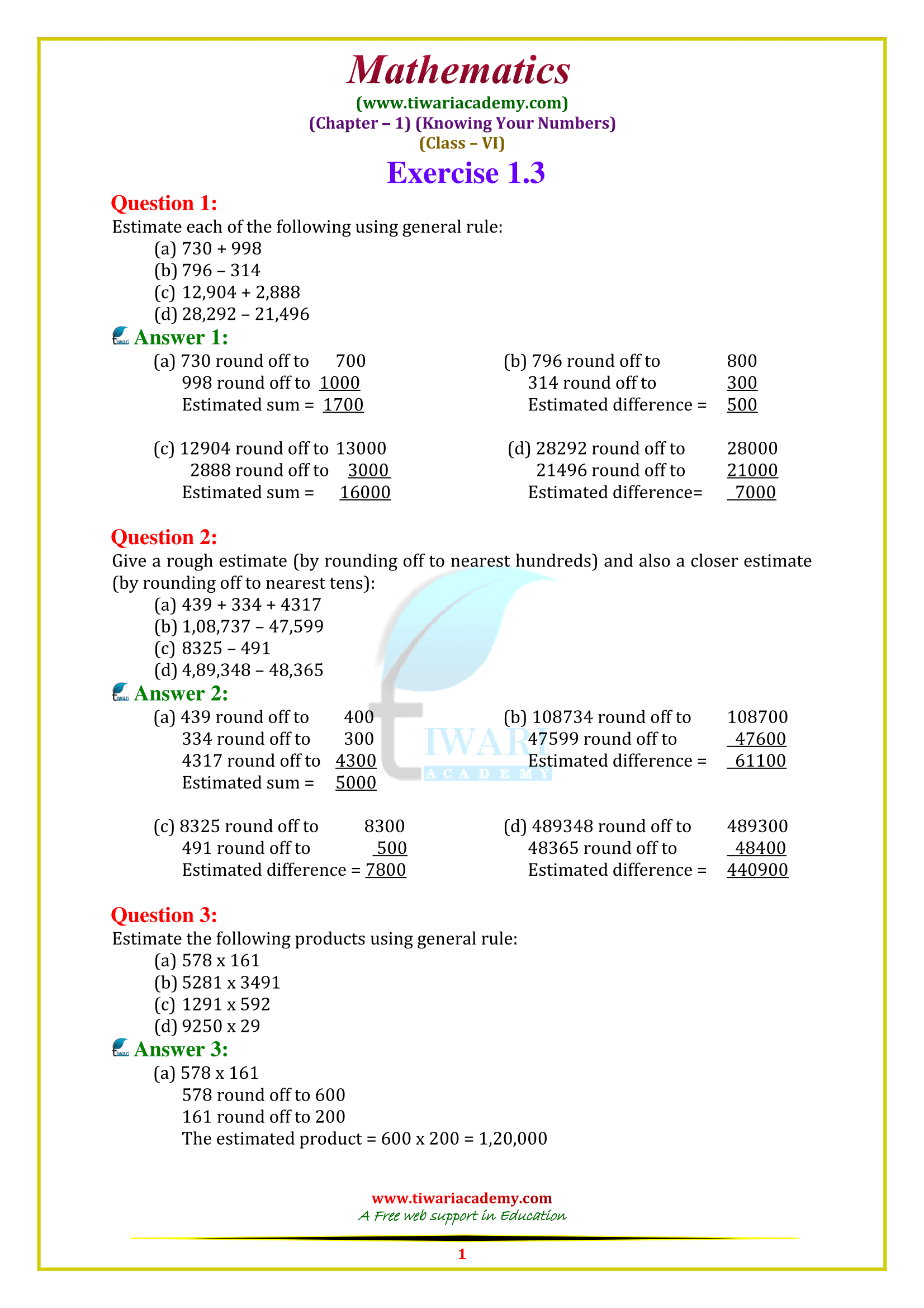
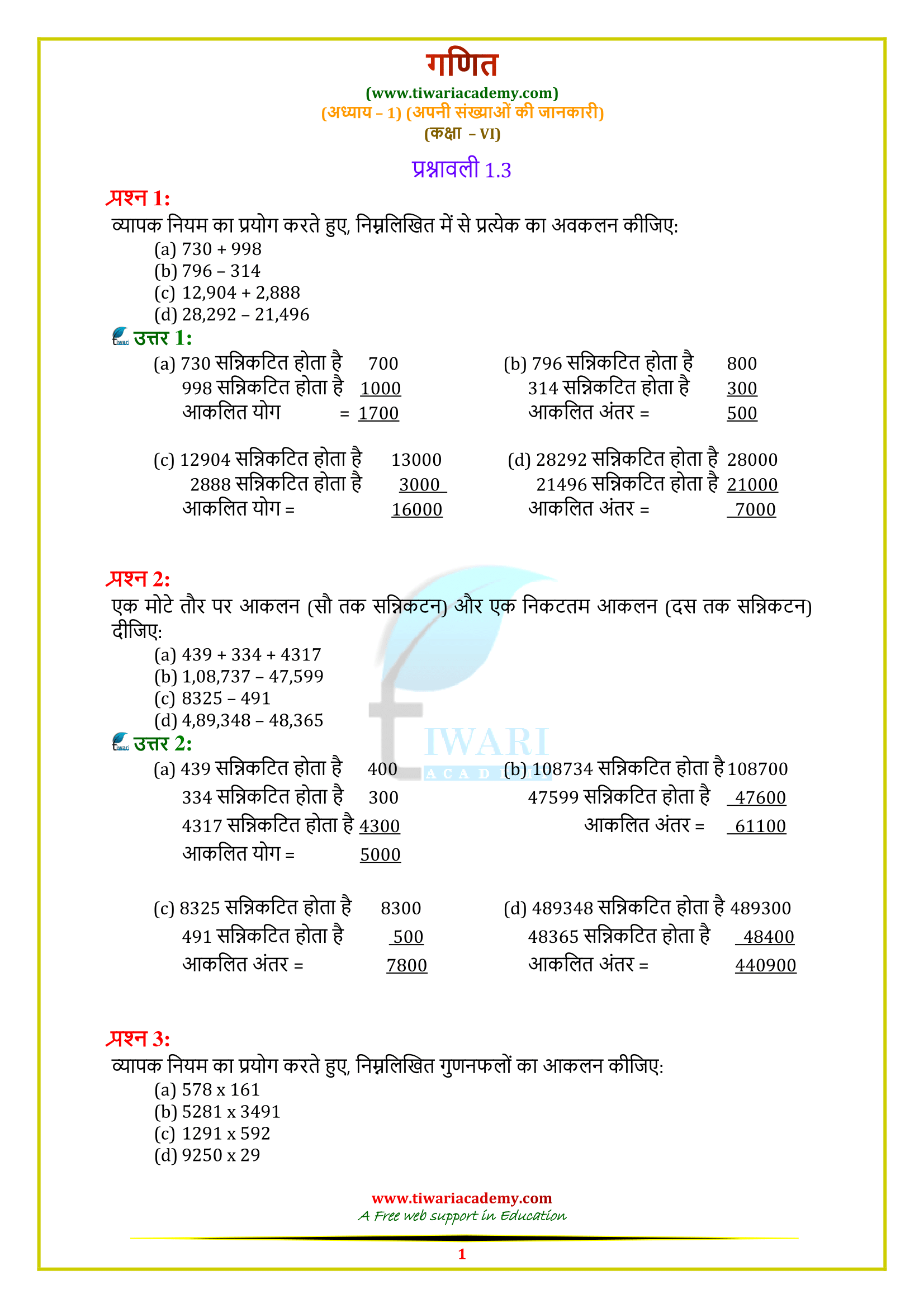
Class 6 Maths Chapter 1 Exercise 1.3 Solution
Class VI Mathematics Ex. 1.3 of Knowing Our Numbers in PDF file format free to download updated for new academic session. CBSE Class 6 Maths Solutions are useful for all the students who are following NCERT Textbooks whether in CBSE board or state boards.
| Class: 6 | Mathematics |
| Chapter: 1 | Exercise: 1.3 |
| Chapter Name: | Knowing Our Numbers |
| Medium: | Hindi and English Medium |
| Content Mode: | Online Text and Videos |
Estimation
In our day-to-day life, we use numbers. In general, we need to state the exact number as an answer to the question which requires us to tell about how many. But in some situations, we need only a rough estimate of a number. Instead of telling the exact number we put forth an approximately estimated number. For example, imagine the marriage of your brother. If you ask your father about the number of guests who will attend the marriage, it will be very difficult for him to tell you the exact number of guests. He may give you a rough estimate. If he has sent invitations to 275 persons, then he may say that around 300 persons will attend the celebration. So, we must know how to estimate (or round off) a number.
Estimating or Rounding Numbers to Nearest 10:
Finding out the multiple of 10 which is nearest to the given number is called the rounding of the given number to the nearest 10.
From the above examples, we find the following rule:
When we round of a given number to the nearest ten, we consider the number at the ones place. If the number at the ones place is 5 or more than 5, the number at tens place is increased by 1 and the number at the ones place changes to zero. If the number at the ones place is less than 5, the number at the tens place remains the same and the number at the ones place changes to zero.
Class 6 Maths Exercise 1.3 Important questions
Round each of the following numbers to the nearest 10: 1. 74 2. 325 3. 828
1. 74 is nearer to 70 than 80, so we round it to 70.
2. 325 is halfway between 320 and 330, so we round it to 330.
3. 828 is nearer to 830 than 820, so we round it to 830.
Round each of the following numbers to the nearest 100: 1. 262 2. 545 3. 9250
262 is nearer to 300 than 200, so we round it to 300.
545 is nearer to 500 than 600, so we round it to 500.
9250 is halfway between 9200 than 9300, so we round it to 9300.
Round each of the following to the nearest 1000: 1. 3287 2. 3845 3. 3500 4. 61780
1. 3287 is nearer to 3000 than 4000, so we round it to 3000.
2. 3845 is nearer to 4000 than 3000, so we round it to 4000.
3. 3500 is halfway between 3000 and 4000, so we round it to 4000.
4. 61780 is nearer to 62000 than 61000, so we round it to 62000.
Estimating or Rounding Numbers to Nearest 10000: 1. 113862 2. 49673 3. 75300 4. 379600
1. 13862 is nearer to 10000 than 20000, so we round it to 10000.
2. 49673 is nearer to 50000 than 40000, so we round it to 50000.
3. 75300 is halfway between 70000 and 80000, so we round it to 80000.
4. 379600 is nearer to 380000 than 370000, so we round it to 380000.
Estimating or Rounding Numbers to Nearest 100:
Finding out the multiple of 100 which is nearest to the given number is called the rounding of the number to the nearest hundred.
From the above examples, we find the following rule:
When we round a given number to the nearest hundred, we consider the number at the ten’s place. If the numbers at the tens place is 5 or more than 5, the number at the hundreds place is increased by 1 and number at the tens place and one’s place change to zeros. If the number at the tens place is less than 5, the number at the hundreds place remains the same and the numbers at the ten’s place and one’s place change to zeros.
Estimating or Rounding Numbers to Nearest 1000:
Finding out the multiple of 1000 which is nearest to the given number is called the rounding of the number to the nearest thousand.
From the above examples, we find the following general rule:
When we round a given number to the required place, we consider the next number at the right side. If this number is 5 or more than 5, then the number at required place is increased by 1 and all the numbers at the right side become zeros. If this number at right side is less than 5, the number at the required place remains the same and all the numbers at the right side become zeros.
Estimating Sum, Difference, Product and Quotient
In our daily life, we come across many situations involving numbers where we need to find an answer more quickly but not very accurate. For example, if you wish to buy certain articles, you need to know the rough estimate of the sum of their prices so that you can demand the approximate amount from your parents.
There are no rigid rules to estimate the sum. However, the procedure of estimation depends on the degree of accuracy required, simplicity of computation and reasonability of the final answer.
Class 6 Maths Exercise 1.3 Solved practice questions
Estimate the sum (4328 + 15659) by estimating the numbers to their nearest hundreds.
Estimating the numbers 4328 and 15659 to their nearest hundreds, we get 4300 and 15700 respectively. We have, 4300 + 15700 = 20000
Estimated sum = 20000.
We have, 4328 + 15659 = 19987.
Clearly, 20000 is more closure to the actual sum. So, 20000 is more reasonable estimate.
Estimate:(4755 – 473).
Let us first round the given numbers to nearest thousands.
4755 rounds to 5000
473 rounds to – 0
Estimated difference = 5000
But this is not a reasonable estimate. To get a closer estimate, let us try to round each number to nearest hundred.
4755 rounds to 4800
473 rounds to – 500
Estimated difference = 4300
This is a better and more meaningful estimate.
Estimate the product:425 × 293 by rounding off each factor to its greatest place.
Clearly, each factor is a three digits’ number. So, we round off each factor to nearest hundreds.
425 rounds to 400. 293 rounds to 300.
Estimated product = 400 × 300 = 120000.
Find the estimated quotient 3256 ÷ 122 by rounding the divisor and dividend to the nearest hundreds.
3256 rounds to 3300. 122 rounds to 100.
Roman Numerals
We know that there are seven distinct symbols (numerals) in this system. These symbols along with corresponding Hindu-Arabic numerals are given below:
| Hindu-Arabic numerals | Roman Numerals |
|---|---|
| 1 | I |
| 5 | V |
| 10 | X |
| 50 | L |
| 100 | C |
| 500 | D |
| 1000 | M |
Using these symbols, we can write any number by following certain rules which are given below:
Rule- 1: If a symbol is repeated, its value is added as many times as it occurs.
Example:
II = 1 + 1 = 2; III = 1 + 1 + 1 = 3 and XXX = 10 + 10 + 10 = 30
Note: A symbol is never repeated more than three times, and the symbols V, L and D are never repeated.
Rule- 2: If a symbol of smaller value is written to the right of a symbol of greater value, we add its value to the value of greater symbol.
Example:
VI = 5 + 1 = 6;
XI = 10 + 1 = 11 and
LXV = 50 + 10 + 5 = 65.
Rule- 3: If a symbol of smaller value is written to the left of a symbol of greater value, its value is subtracted from the value of the greater symbol.
Example: IV = 5 – 1 = 4; IX = 10 – 1 = 9; XL = 50 – 10 = 40 and XC = 100 – 10 = 90.
Notes: The symbols V, L and D are never written to the left of a symbol of greater value. In other words, V, L and D are never subtracted.
The symbol I can be subtracted from V and X only.
The symbol X can be subtracted from L, M and C only.
The symbol C can be subtracted from D and M only.
Rule- 4: If a smaller numeral is placed between two larger numerals, it is always subtracted from the larger numeral immediately following it.
XIV = 10 + (5 – 1) = 14; XIX = 10 + (10 – 1) = 19 and
CXIV = 100 + 10 + (5 – 1) = 114.
If a bar is placed over a numeral, it is multiplied by 1000.
Example: V ̅ = 5000 and L ̅ = 50000.
Important questions on 6th Maths Exercise 1.3
Estimate the sum (4328 + 15659) by estimating the numbers to their nearest hundreds.
Estimating the numbers 4328 and 15659 to their nearest hundreds, we get 4300 and 15700 respectively. We have, 4300 + 15700 = 20000
Estimated sum = 20000.
We have, 4328 + 15659 = 19987.
Clearly, 20000 is more closure to the actual sum. So, 20000 is more reasonable estimate.
Write the following in Roman numerals for: 1. 69 2. 88
1. 69 = 60 + 9 = LX + IX = LXIX
2. 88 = 80 + 8 = LXXX + VIII = LXXXVIII
Write the following in Roman numerals: 1. 69 2. 88 3. 99 4. 235
1. 69 = 60 + 9 = LX + IX = LXIX
2. 88 = 80 + 8 = LXXX + VIII = LXXXVIII
3. 99 = 90 + 9 = XC + IX = XCIX
4. 235 = 200 + 30 + 5 = CC + XXX + V = CCXXXV
Round each of the following numbers to the nearest 10. (a) 13 (b) 727
(a) 13 is the number between 10 and 20, but this is near to the 10, hence round of 13 is 10.
(b) 727 is the number between 720 and 730, but this is near to the 730, hence round of 727 is 730.
Following these rules, we get
| Hindu-Arabic numerals | Roman Numerals |
|---|---|
| 1 | I |
| 2 | II |
| 3 | III |
| 4 | IV |
| 5 | V |
| 6 | VI |
| 7 | VII |
| 8 | VIII |
| Hindu-Arabic numerals | Roman Numerals |
|---|---|
| 9 | IX |
| 10 | X |
| 20 | XX |
| 30 | XXX |
| 40 | XL |
| 50 | L |
| 60 | LX |
| 70 | LXX |
| Hindu-Arabic numerals | Roman Numerals |
|---|---|
| 80 | LXX |
| 90 | LC |
| 100 | C |
| 400 | CD |
| 500 | D |
| 600 | DC |
| 900 | CM |
| 1000 | M |
Note: Roman did not have a symbol for zero (0).
Which is the best sum of exercise 1.3 Class 6 Maths?
Question 2 is the best question of exercise 1.3 of class 6th Maths. This problem is nice and interesting. In this question, students have to give a rough estimate (by rounding off to nearest hundreds) and also a closer estimate (by rounding off to nearest tens) of the given parts.
Is exercise 1.3 of class 6th Maths important for first term exams?
Yes, exercise 1.3 of class 6th Maths is very important for the exams. Every year questions come from this exercise in the exam. All the questions of this exercise are important but, questions 2 and 3 are most important.
How many sums are there in exercise 1.3 of class 6th Maths?
There are 2 examples (examples 5, 6) and 3 questions in exercise 1.3 of class 6th Maths. All the questions and examples of this exercise are nice, logical, and interesting. All questions of this exercise are distinct from each other.
How much time, students need to do exercise 1.3 of class 9th Maths?
Students need a maximum of 2 days to complete exercise 1.3 (chapter 1) of class 6th mathematics if they give at least 1 hour per day to this exercise. This time depends on many factors like student’s working speed, efficiency, and capability.