NCERT Solutions for Class 6 Maths Chapter 1 Exercise 1.2 in Hindi and English Medium modified and updated for academic year 2025-26 exams. The class VI Mathematics solutions of ex. 1.2 is revised as per new NCERT textbooks and following the new rationalised curriculum 2025-26.
6th Maths Exercise 1.2 Solution in Hindi and English Medium
Class 6 Maths Chapter 1 Exercise 1.2 Solution
Class VI Mathematics Ex. 1.2 of chapter 1 Knowing Our Numbers in Hindi and English Medium free to use online or download in PDF file format. All NCERT book question answers are updated for academic session 2025-26 for CBSE and State board students using NCERT Textbooks.
| Class: 6 | Mathematics |
| Chapter: 1 | Exercise: 1.2 |
| Topic Name: | Knowing Our Numbers |
| Medium: | Hindi and English Medium |
| Session: | CBSE 2025-26 |
| Content Type: | Text and Videos |
Comparison of Numbers
We adopt the following rules to compare two large numbers:
Rule- 1: The number with more digits is greater than the number with less digits.
Rule- 2: When two numbers have the same number of digits, compare the digits at the leftmost places. The number with the greater digit is greater. If the digits at the leftmost places are the same then compare the next digits and so on.
Large Numbers in Practice
In earlier classes, we have learnt that centimetre (cm) in used as a unit of measurement of length. We can use this unit for measuring the length of a pen, the width of a book or note book etc. but this unit is too big to measure the thickness of a pen. So, we use another unit called millimetre (mm). Also, centimetre and millimetre are very small units to measure the length of the wall or a room. We use another unit called metre for the same. Even metre is too small unit when we state the distances between two towns or cities. For this we need kilometres (km).
Class 6 Maths Exercise 1.2 Important Questions
Compare the following sets of numbers and mark which is greater? (i). 38402135, 7932814 (ii). 75432812, 75968234
(i). Here, we have to compare 38402135 and 7932814. Clearly, 38402135 contains eight digits while 7932814 contains seven digits. We know that a number with more digits is greater. So 38402135 > 7932814.
(ii). Here, we have to compare 75432812 and 75968234. Both the numbers have eight digits. At the crores place both have the same digit, namely 7. At the ten lakhs place both have the same digit, namely 5. But, at the lakhs place, the first number has 4 while the second has 9. Clearly, 4 < 9. so 75432812 < 75968234.
Arrange the following numbers in ascending order: 2903324, 29018381, 908169, 9058450.
The smallest number is 908169. The next number greater than 908169 is 2903324. The numbers greater than 2903324 in order are 9058450 and 29018381. The numbers when arranged in ascending order are: 908169 < 2903324 < 9058450 < 29018381.
Arrange the following numbers in descending order: 24321805, 2860099, 40652034, 9408252.
The greatest number is 40652034. The next number smaller than 40652034 is 24321805. The numbers smaller than 24321805 in order are 9408252 and 2860099. The numbers when arranged in descending order are: 40652034 > 24321805 > 9408252 > 2860099.
Arrange the following numbers in ascending order: 61845031, 382047961, 28306110, 182010901, 54012519.
The smallest number is 28306110. The next smaller numbers are 54012519, 64845031 and then 182010901 and greatest number is 382047961. The numbers when arranged in ascending order are:
382047961 > 182010901 > 61845031 > 54012519 > 28306110
These units are connected with each other by the following relations:
1 kilometre = 1000 metres (m)
1 metre = 100 centimetres (cm)
1 centimetre = 10 millimetres (mm)
Also, we have:
1 m = 100 cm = 100 × 10 mm = 1000 mm
1 km = 1000 m = 1000 × 100 cm = 100000 cm
1 km = 100000 cm = 100000 × 10 mm = 1000000 mm
Extra Question Answers based on 6th Maths Exercise 1.2
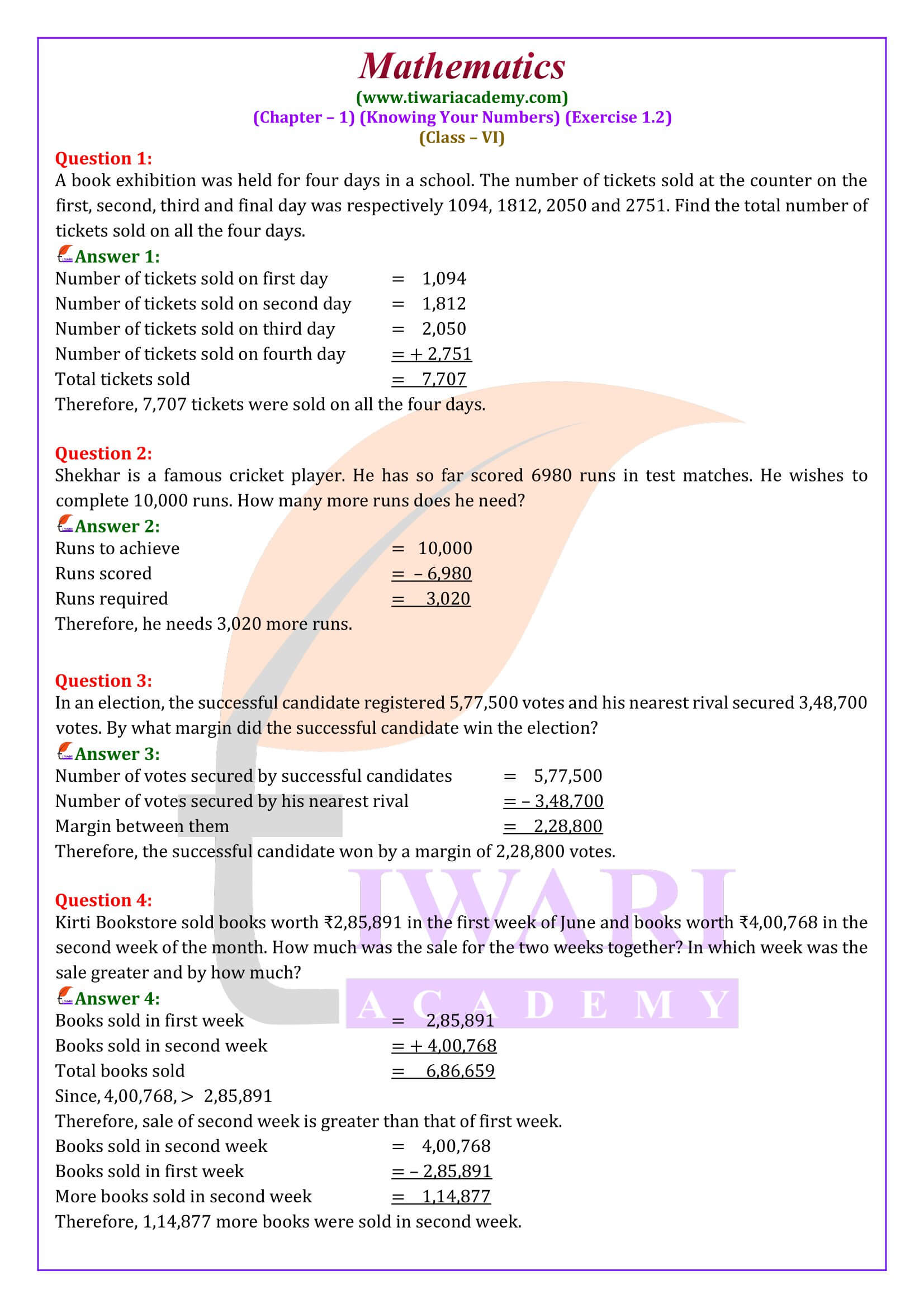
A house building corporation built 8785973 flats in 2006 and 9497934 flats in 2007. How many flats were built in these two years?
Flats built in 2006 = 8 7 8 5 9 7 3
Flats built in 2007 = 9 4 9 7 9 3 4
Total flats built in two years = 8785973 + 9497934 = 1 8 2 8 3 9 0 7
So the corporation built 18283907 flats in two years
6546717 bags of cement were stored in a godown. Out of these, 670745 bags were taken out in January and 721806 bags were taken out in February. How many bags of cement were in stock in the godown after February?
6546717 bags of cement were stored in a godown. Out of these, 670745 bags were taken out in January and 721806 bags were taken out in February. How many bags of cement were in stock in the godown after February?
Ans.: Cement taken out in January = 670745 bags
Cement taken out in February = + 721806 bags
Total cement taken out = 1392551 bags
Cement in godown = 6546717 bags
Cement taken out = 1392551 bags
Balance in stock = 6546717 – 1392551 = 5154166 bags
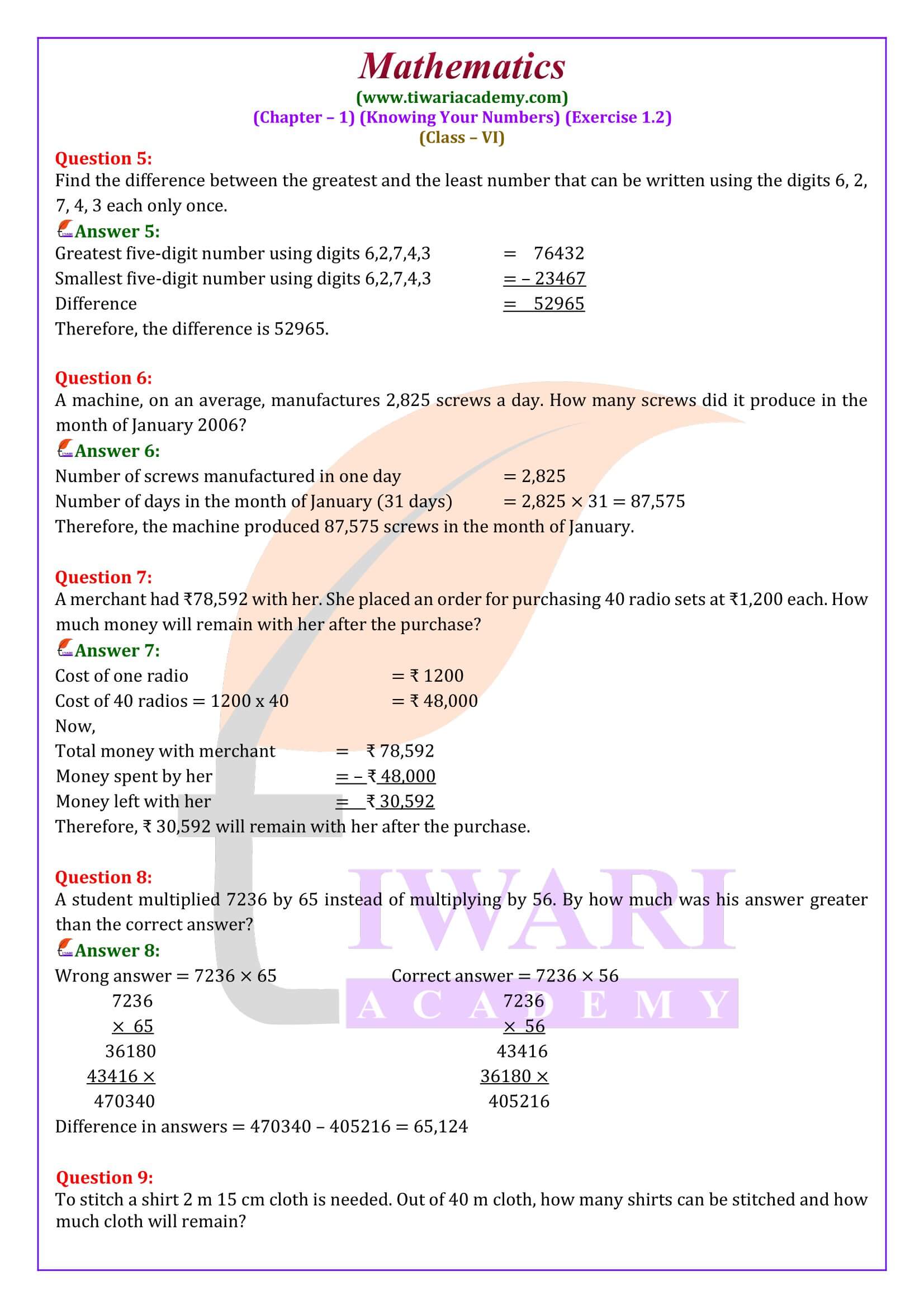
8459 nails can be packed in one carton. How many nails can be packed in such 1704 cartons?
Number of nails in 1 carton = 8459
Number of nails in 1704 cartons = (8459 × 1704) = 14414136
Hence, the number of nails in 1704 cartons is 14414136.
A book of 1456 pages has 995904 words in it. How many words are there in each page, if each page consists of 38 lines. How many words are there in each line?
Number of words in 1456 pages = 995904
Number of words in 1 page = 995904 ÷ 1456 = 684
The number of words in 1 page = 684.
But, each page has 38 lines.
The number of words in 38 lines = 684
The number of words in 1 line = 684 ÷ 38 = 18
Hence, the number of words in 1 line are 18.
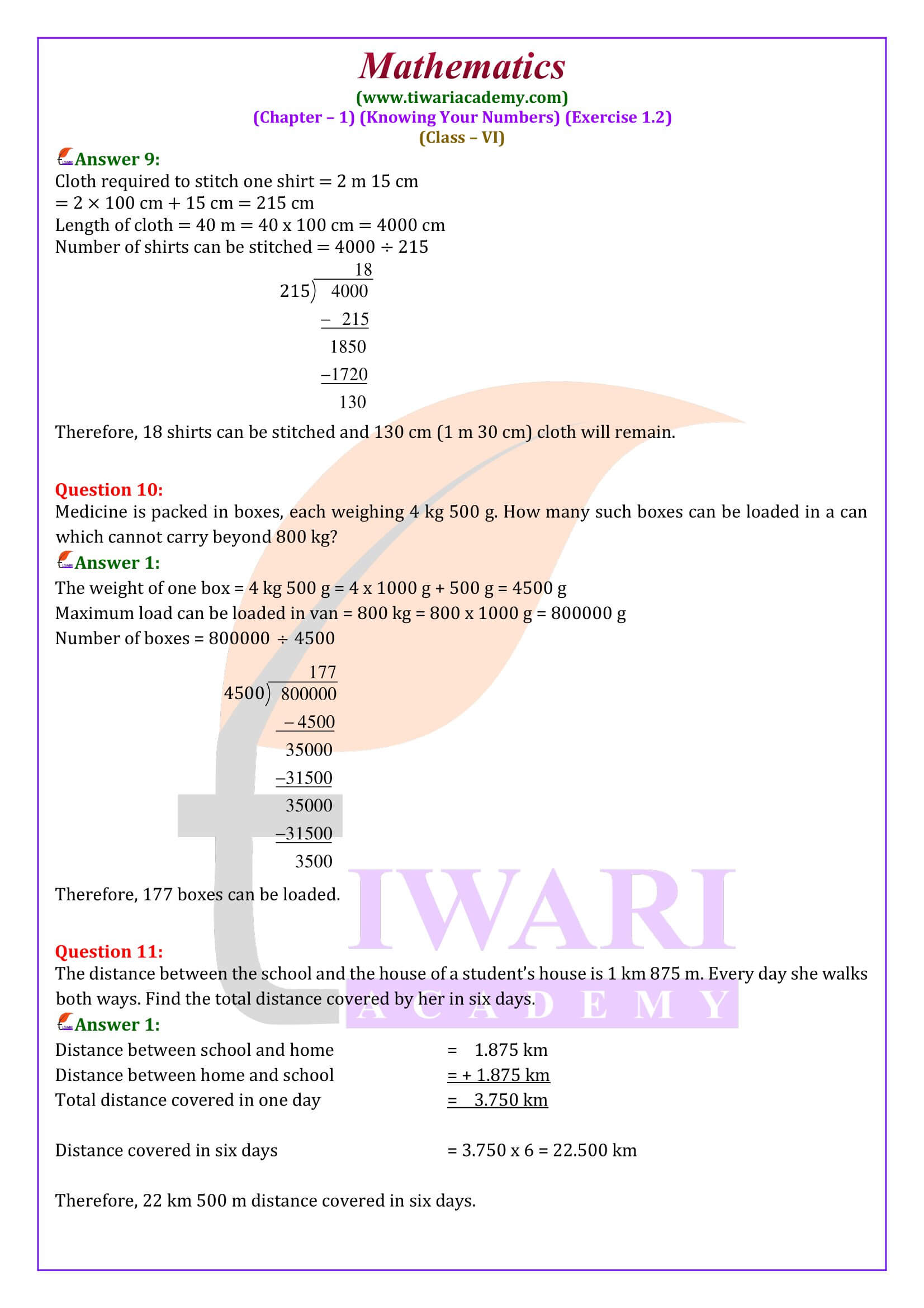
Measurement and Weight
When we go to market to buy wheat, sugar, rice etc. we buy these items in kilograms (kg). But, items like chillies, ginger, garlic etc. which we do not need in large quantities are measured in grams (g). In order to measure the weight of compound or chemicals in medicines, we use smaller unit called milligrams (mg).
Following are the relations between these three units of measurement of weight.
1 kilogram (kg) = 1000 grams (g)
1 gram = 1000 milligrams (mg)
1 kilogram (kg) = 1000 × 1000 milligrams = 1000000 milligrams (mg)
We buy milk in litres (l), whereas liquid medicines etc. are measured in millilitres (ml). Following is the relation between these two units.
1 litre (l) = 1000 millilitres (ml)
Important Question Answers on Class 6 Maths Exercise 1.2
How can we compare multiple digit large numbers?
In case of big numbers to compare multiple numbers first of all we count number of digit. The number which has more number of digits that one it greatest. If the digits are same then we observe left most digit of all the numbers which has biggest one and so on.
Ex.: 382047961 > 182010901 > 61845031 > 54012519 > 28306110
As in above example first and second left numbers have nine digits and remaining have eight digits, but in case of first two numbers left digit of first number is 3 and that of second is 1. So the first one is greatest number.
How can we change 1 kilometre in millimetres?
1 km = 1000 m
1m = 100 cm
1cm = 10 mm
1 km = 1000 m = 100000 cm = 100000 × 10 mm = 1000000 mm
Is 1 kilogram and 1 litre is same or not?
A litre of liquid water has a mass almost exactly equal to one kilogram. Because volume changes with temperature and pressure, and pressure uses units of mass, the definition of a kilogram was changed. At standard pressure, one litre of water has a mass of 0.999975 kg at 4 °C, and 0.997 kg at 25 °C.
Note: That in all the units mentioned above, we have some words common like kilo, milli and centi. It should be noted that kilo is the largest and milli is the smallest. Kilo shows 1000 times greater and milli shows 1000 times smaller. Similarly, centi shows 100 times smaller.
Are there any examples in exercise 1.2 of Maths class 6th?
Yes, there are four examples (examples 1, 2, 3, 4) in exercise 1.2 of grade 6th Maths. All four examples are very simple to solve and understand. Students can easily do these examples.
What kind of questions are there in exercise 1.2 of Maths class 6th?
In exercise 1.2 of Maths 6th standard, there are 12 questions, and all questions are word problems. Students find some word problems easy and some word problems a little tough.
How many days are required to complete exercise 1.2 of class 6th Maths?
If students give 2 hours per day to exercise 1.2 of class 6th Maths then, 2 days are sufficient to complete exercise 1.2 of class 6th Maths because exercise 1.2 has only 4 examples (examples 1, 2, 3, 4) and 12 questions. This time can vary because different students have different working speeds, efficiency and capability.
Which questions of exercise 1.2 of class 6th Maths are considered as the most important questions?
Exercise 1.2 of class 6th Maths has 4 examples (examples 1, 2, 3, 4) and 12 questions. All examples and questions of exercise 1.2 are important. But, the most important problems of this exercise are questions 4, 5, 8, 9, 12, and examples 4.