NCERT Solutions for Class 12 Physics Chapter 12 in Hindi and English.

Updated by Tiwari Academy
on September 9, 2023, 6:37 AM

NCERT Solutions for Class 12 Physics Chapter 12 Atom in English and Hindi Medium updated for new session 2023-24 CBSE and State board. As per the new textbooks published for academic year 2023-24, the additional exercises are not in syllabus. All the solutions of 12th Physics chapter 12 are modified according to new rationialised NCERT books.

NCERT Solutions for Class 12 Physics Chapter 12

Chapter 12 Atom Solutions

Get the Exercises Solutions and Additional Exercises Solutions in PDF format as well as option for study online without downloading for new academic session. UP Board students also take the benefits of UP Board Solutions for Class 12 Physics Chapter 12. Downloads Offline Apps for NCERT Sols of other subjects also for based on new CBSE Curriculum for 2023-24.

Class: 12	Physics
Chapter 12:	Atom
Study Material:	Notes, Exercises, Additional Questons
Material Format:	Text, Videos, PDF and Images
Educational Session:	Year 2023-24
Medium:	Hindi and English

Class 12 Physics Chapter 12 Solutions in English

NCERT Solutions for Class 12 Physics Chapter 12 Atom in PDF form are given below to free download. Visit to Discussion Forum to ask your doubts in educational fact regarding to NIOS or CBSE boards. Download NCERT Books 2023-24 following the latest CBSE Syllabus.

Important Questions for practice

1. The radius of inner most orbit of Hydrogen atom is 5.3 × 10–1m. What are the radii of n = 2 and n = 8 orbits.
2. A metal surface illuminated by 8.5 × 1014 Hz light emits electrons whose maximum energy is 0.52 eV the same surface is illuminated by 12.0 × 1014 Hz light emits elections whose maximum energy is 1.97eV. From these data find work function of the surface and value of Planck’s constant. [Work Function = 3ev]
3. State the law of radioactive disintegration. Hence define disintegration constant and half life period. Establish relation between them.

4. State Bohr’s postulates. Using these postulates, drive an expression for total energy of an electron in the nth orbit of an atom. What does negative of this energy signify?
5. The total energy of an electron in the first excited state of the hydrogen atom is about –3.4 eV. What is (a) the kinetic energy, (b) the potential energy of the electron? (c) Which of the answers above would change if the choice of the zero of potential energy in changed to (i) + 0.5 eV (ii) –0.5 eV.

Questions from Board Papers

1. A 12.5 MeV alpha – particle approaching a gold nucleus is deflected by 180°. What is the closest distance to which it approaches the nucleus?
2. Calculate the radius of the third Bohr orbit of hydrogen atom and energy of electron in that orbit.

3. An electron and a proton are possessing same amount of K.E., which of the two have greater de-Broglie, wavelength? Justify your answer.
4. For what Kinetic energy of a neutron will the associated de Broglie wavelength be 5.6 × 10^-10m?
5. If the frequency of incident light in photoelectric experiment is doubled then does the stopping potential become double or more than double, justify?
6. What will be the distance of closest approach of a 5 MeV proton as it approaches a gold nucleus?

Important Questions on 12th Physics Chapter 12

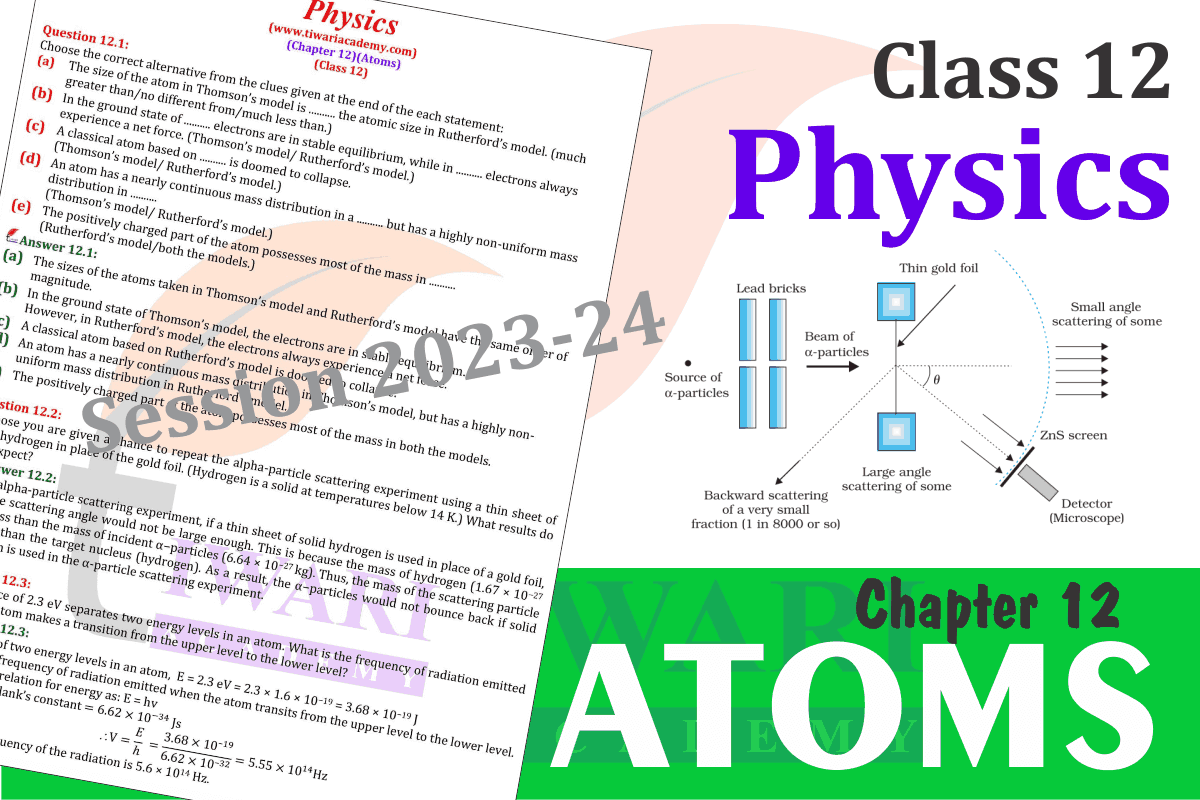
Suppose you are given a chance to repeat the alpha-particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below 14 K.) What results do you expect?

In the alpha-particle scattering experiment, if a thin sheet of solid hydrogen is used in place of a gold foil, then the scattering angle would not be large enough. This is because the mass of hydrogen (1.67 × 10⁻²⁷ kg) is less than the mass of incident α−particles (6.64 × 10⁻²⁷ kg). Thus, the mass of the scattering particle is more than the target nucleus (hydrogen). As a result, the α−particles would not bounce back if solid hydrogen is used in the α-particle scattering experiment.

The ground state energy of hydrogen atom is −13.6 eV. What are the kinetic and potential energies of the electron in this state?

Ground state energy of hydrogen atom, E = − 13.6 eV This is the total energy of a hydrogen atom. Kinetic energy is equal to the negative of the total energy. Kinetic energy = − E = − (− 13.6) = 13.6 eV Potential energy is equal to the negative of two times of kinetic energy. Potential energy = − 2 × (13.6) = − 27.2 eV

Is the average angle of deflection of α-particles by a thin gold foil predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?

About the same, The average angle of deflection of α-particles by a thin gold foil predicted by Thomson’s model is about the same size as predicted by Rutherford’s model. This is because the average angle was taken in both models.

Is the probability of backward scattering (i.e., scattering of α-particles at angles greater than 90°) predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?

Much less. The probability of scattering of α-particles at angles greater than 90° predicted by Thomson’s model is much less than that predicted by Rutherford’s model.

Keeping other factors fixed, it is found experimentally that for small thickness t, the number of α-particles scattered at moderate angles is proportional to t. What clue does this linear dependence on t provide?

Scattering is mainly due to single collisions. The chances of a single collision increases linearly with the number of target atoms. Since the number of target atoms increase with an increase in thickness, the collision probability depends linearly on the thickness of the target.

In which model is it completely wrong to ignore multiple scattering for the calculation of average angle of scattering of α-particles by a thin foil?

Thomson’s model. It is wrong to ignore multiple scattering in Thomson’s model for the calculation of average angle of scattering of α−particles by a thin foil. This is because a single collision causes very little deflection in this model. Hence, the observed average scattering angle can be explained only by considering multiple scattering.

If Bohr’s quantisation postulate (angular momentum = nh/2π) is a basic law of nature, it should be equally valid for the case of planetary motion also. Why then do we never speak of quantisation of orbits of planets around the sun?

We never speak of quantization of orbits of planets around the Sun because the angular momentum associated with planetary motion is largely relative to the value of Planck’s constant (h). The angular momentum of the Earth in its orbit is of the order of 1070h. This leads to a very high value of quantum levels n of the order of 1070. For large values of n, successive energies and angular momenta are relatively very small. Hence, the quantum levels for planetary motion are considered continuous.