NCERT Solutions for Class 12 Maths Chapter 2 Exercise 2.2 Inverse Trigonometric Functions updated and modified for academic session 2025-26 in Hindi and English Medium. The questions of 12th Maths ex. 2.2 are rearranged as per new NCERT textbook published for 2025-26 board exams.
Class 12 Maths Chapter 2 Exercise 2.2 NCERT Solutions
Class 12 Maths Exercise 2.2 Solutions
Class 12 Maths Exercise 2.2 (All Questions)
Class 12 Maths Exercise 2.2 in Hindi
Class 12 Maths Chapter 2 MCQ Solutions
Class 12 Maths Chapter 2 Solutions
Class 12 Maths NCERT Solutions
Class 12 all Subjects NCERT Solutions
| Class: 12 | Mathematics |
| Chapter 2: | Exercise 2.2 |
| Chapter Name: | Inverse Trigonometric Functions |
| Content: | NCERT Exercise Solution |
| Content Mode: | Images, Online Videos and Text Format |
| Medium: | English and Hindi Medium |
Class 12 Maths Exercise 2.2 Solutions in Hindi and English Medium
NCERT Solutions for Class 12 Maths Exercise 2.2
12th Maths Exercise 2.2 Solutions
NCERT Solutions for Class 12 Maths Chapter 2 Exercise 2.2 Inverse Trigonometric Functions in English Medium Online as well as Offline free to use.
Join the discussion forum to discuss your doubts in NIOS or CBSE examinations. Download NCERT Books and Apps for offline use based on CBSE Syllabus.
More about 12 Maths Exercise 2.2
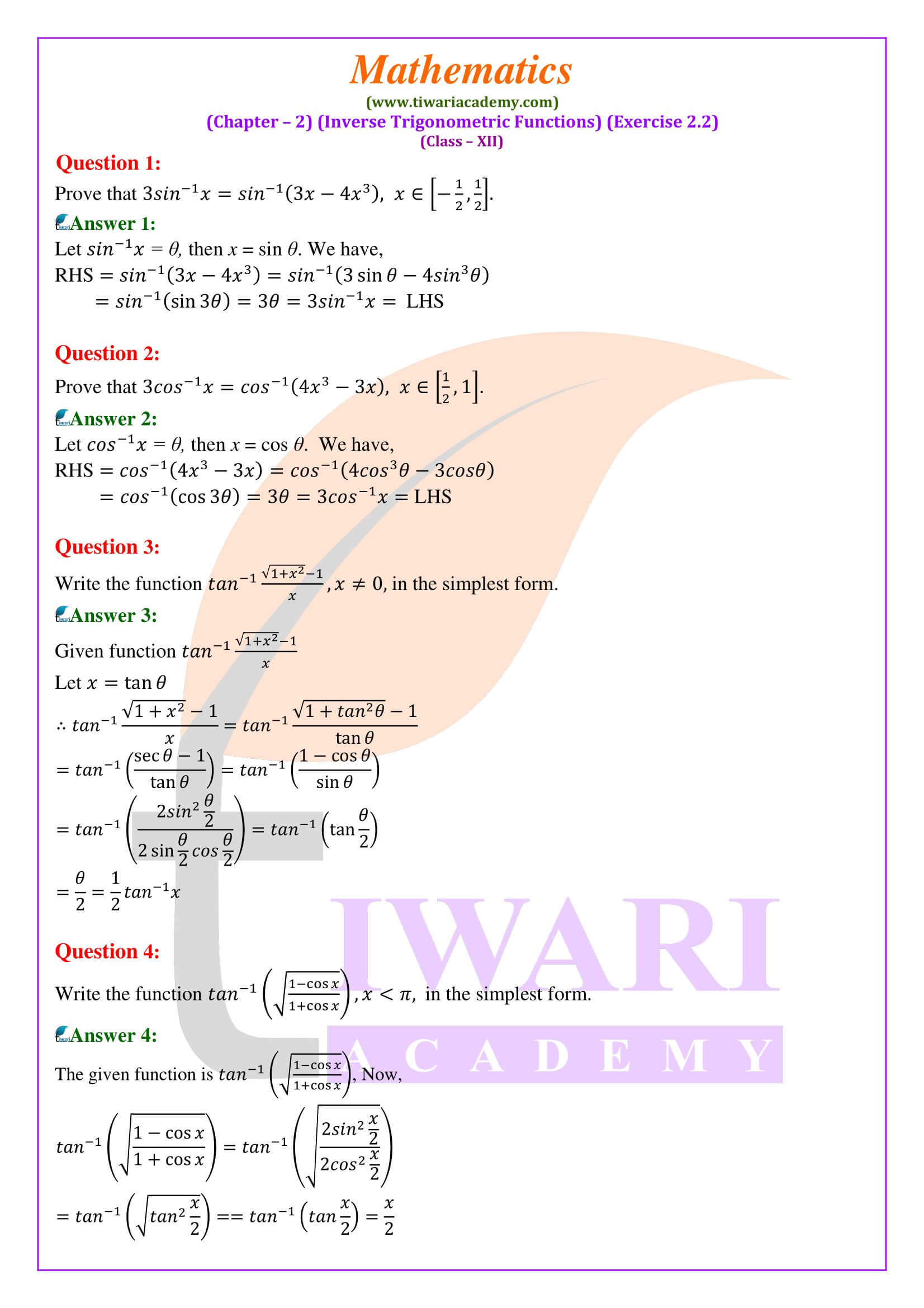
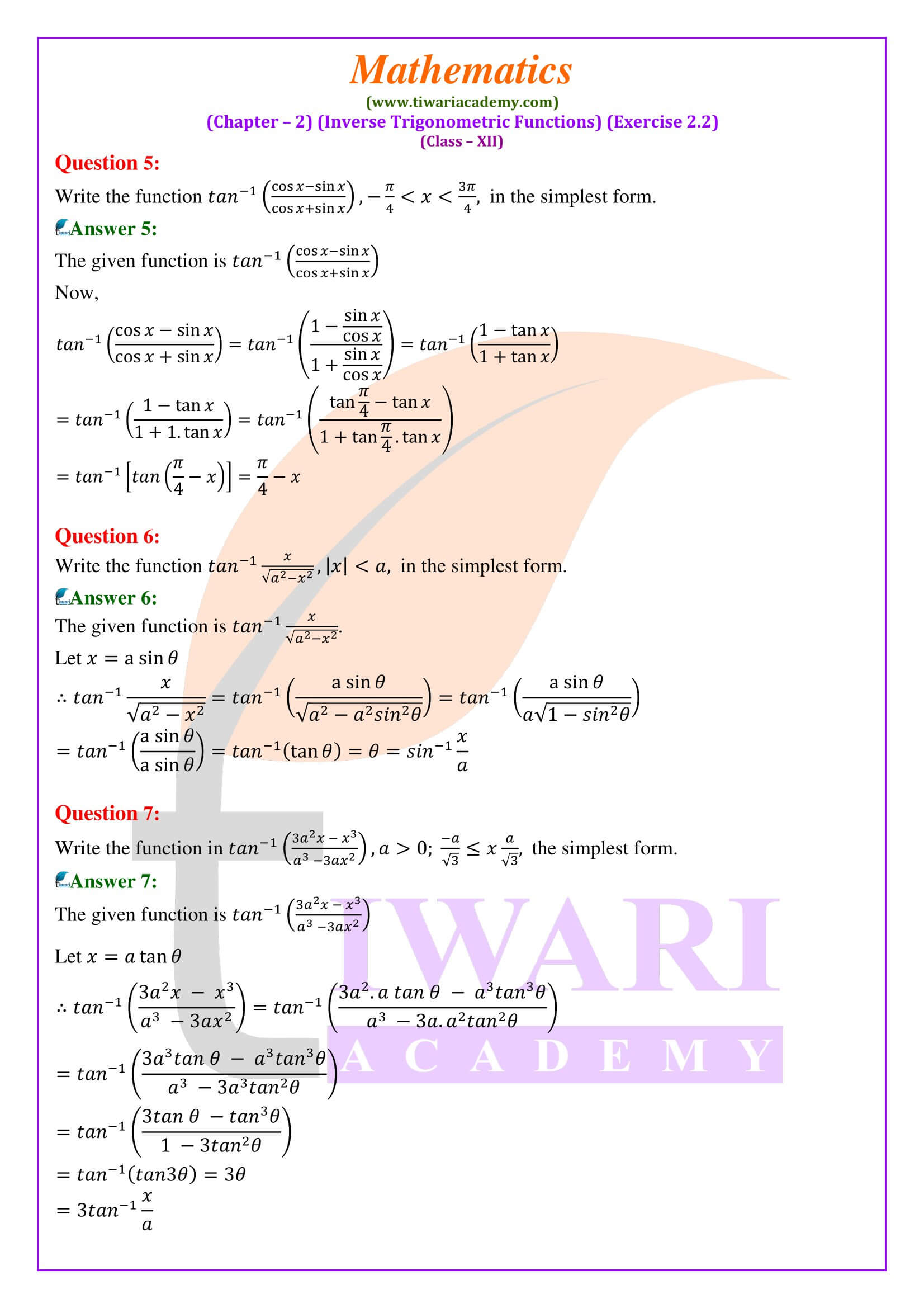
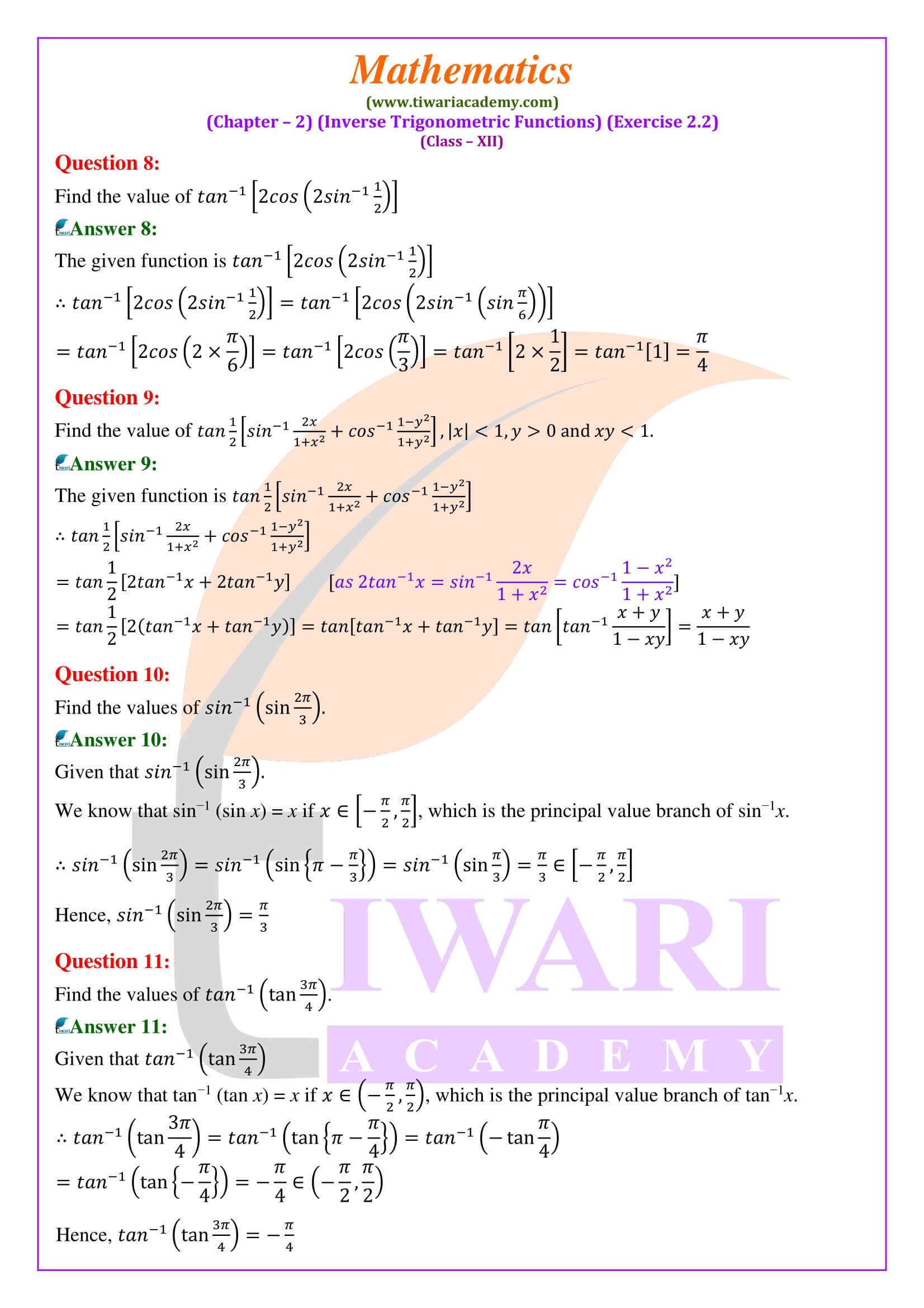
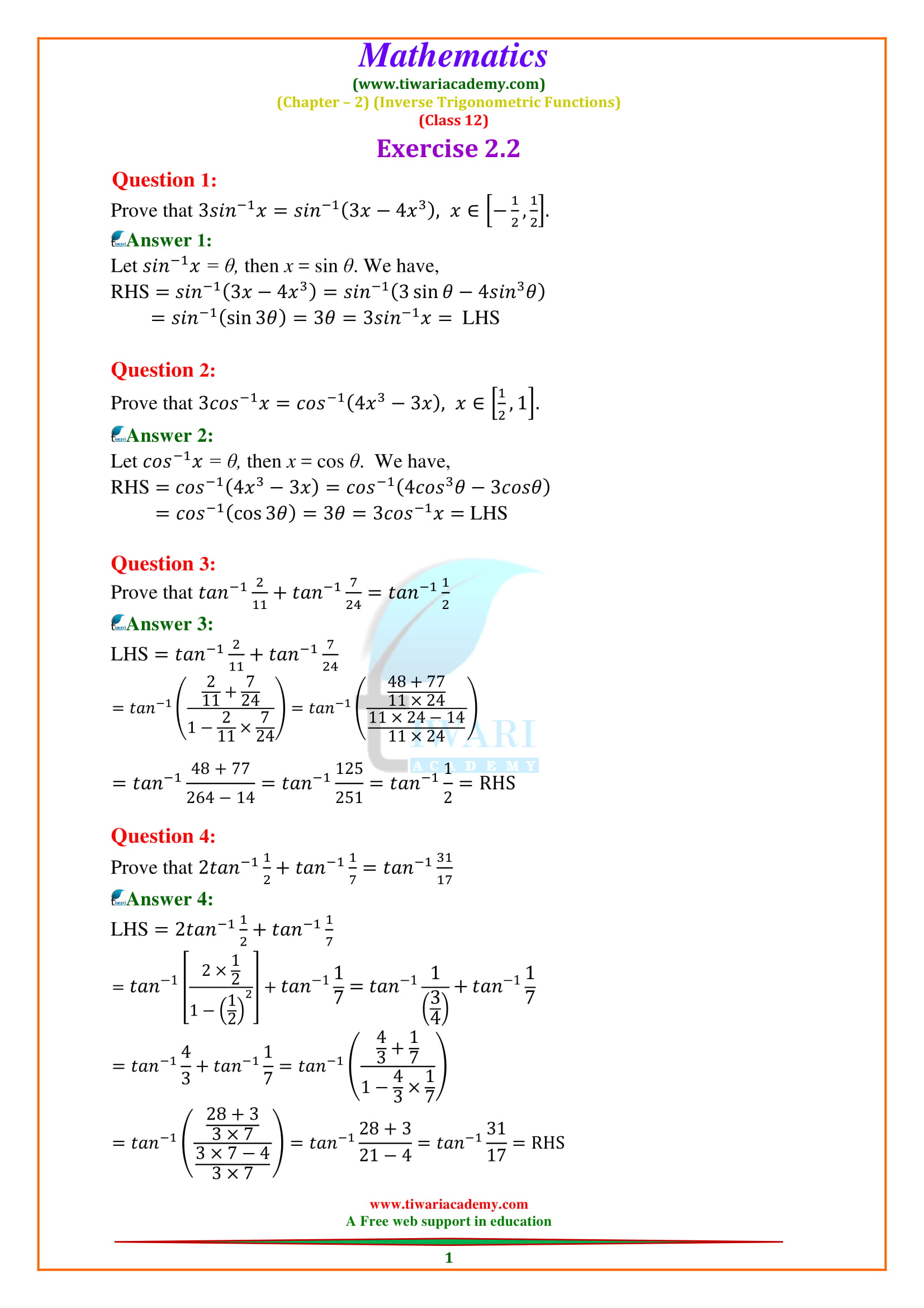
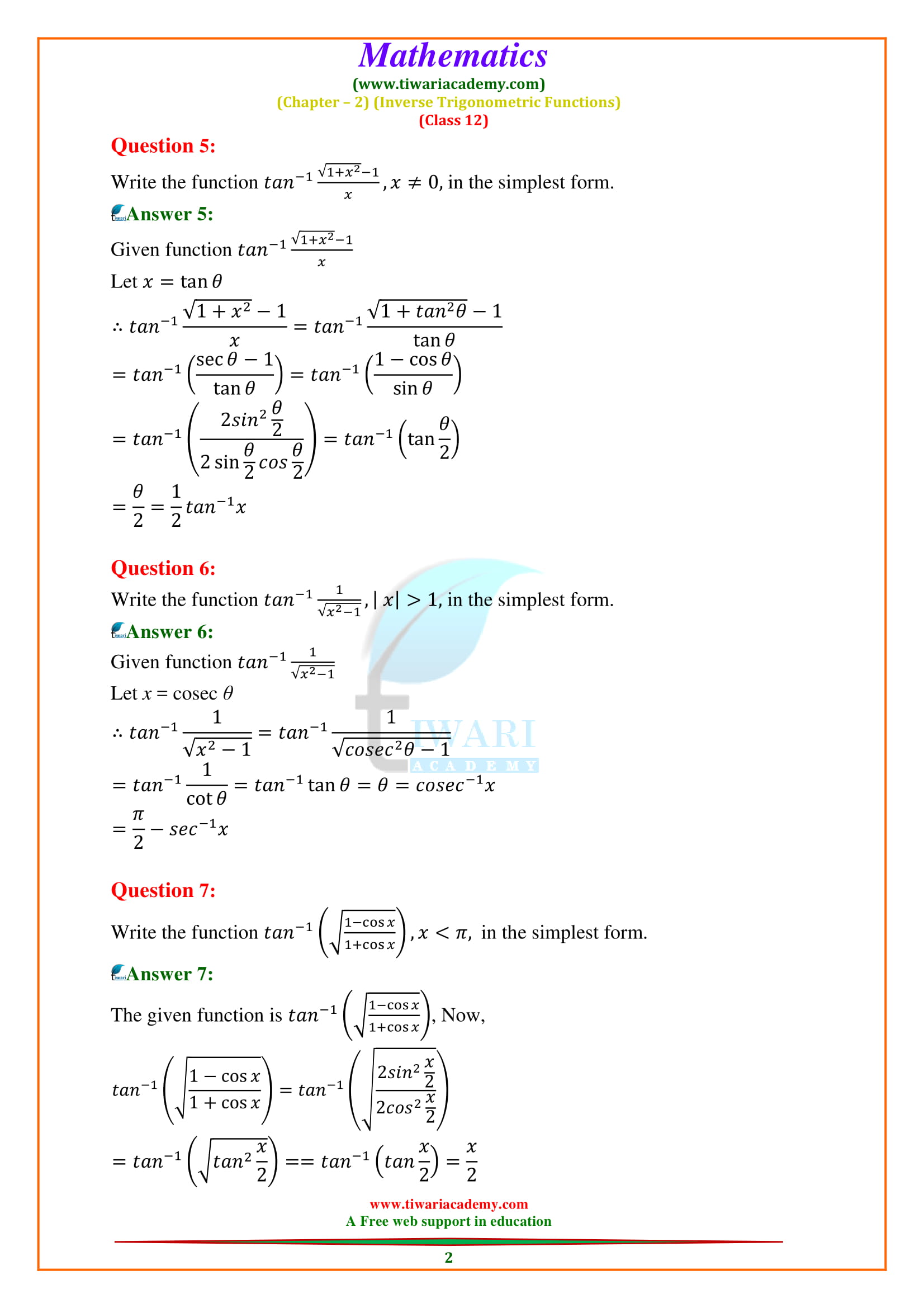
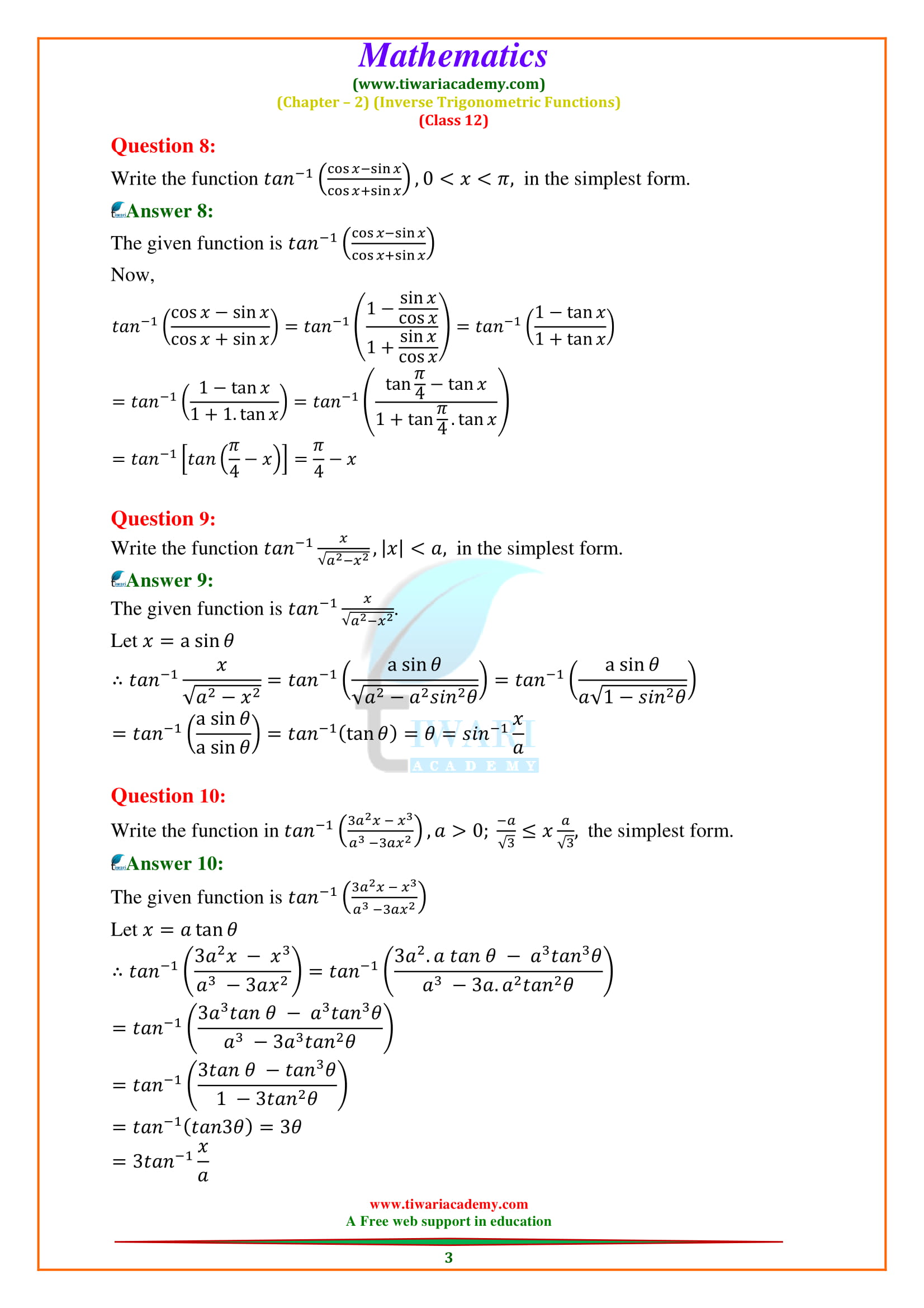
Exercise 2.2 contains the questions based on sin 2x, sin 3x, cos 3x, etc. used in class 11 trigonometry. It also includes the questions based on simplification of inverse trigonometric functions with square roots on the basis of the formula 1 – cos x = 2 sin² x/2 and 1 + cos x = 2 cos² x/2.
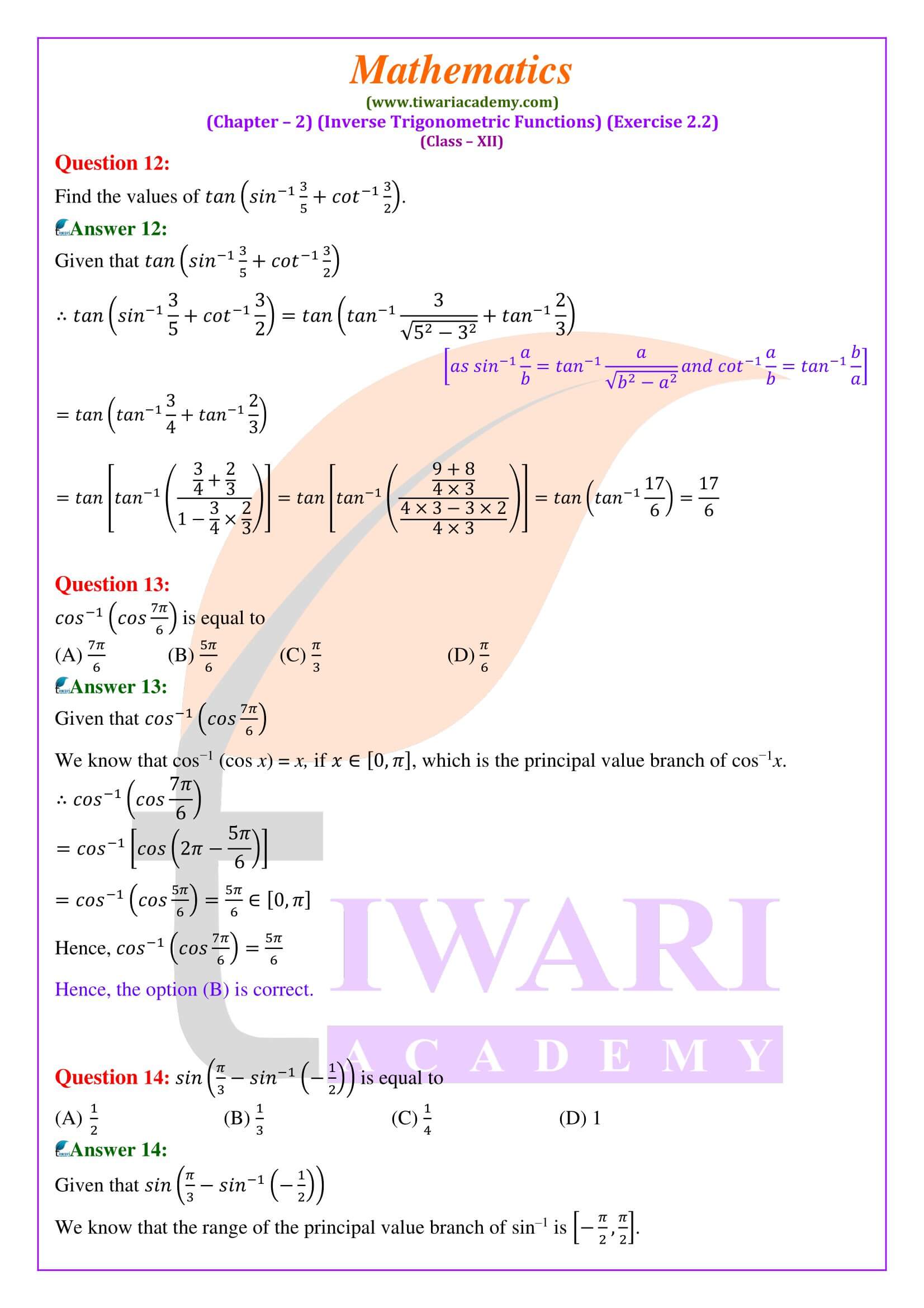
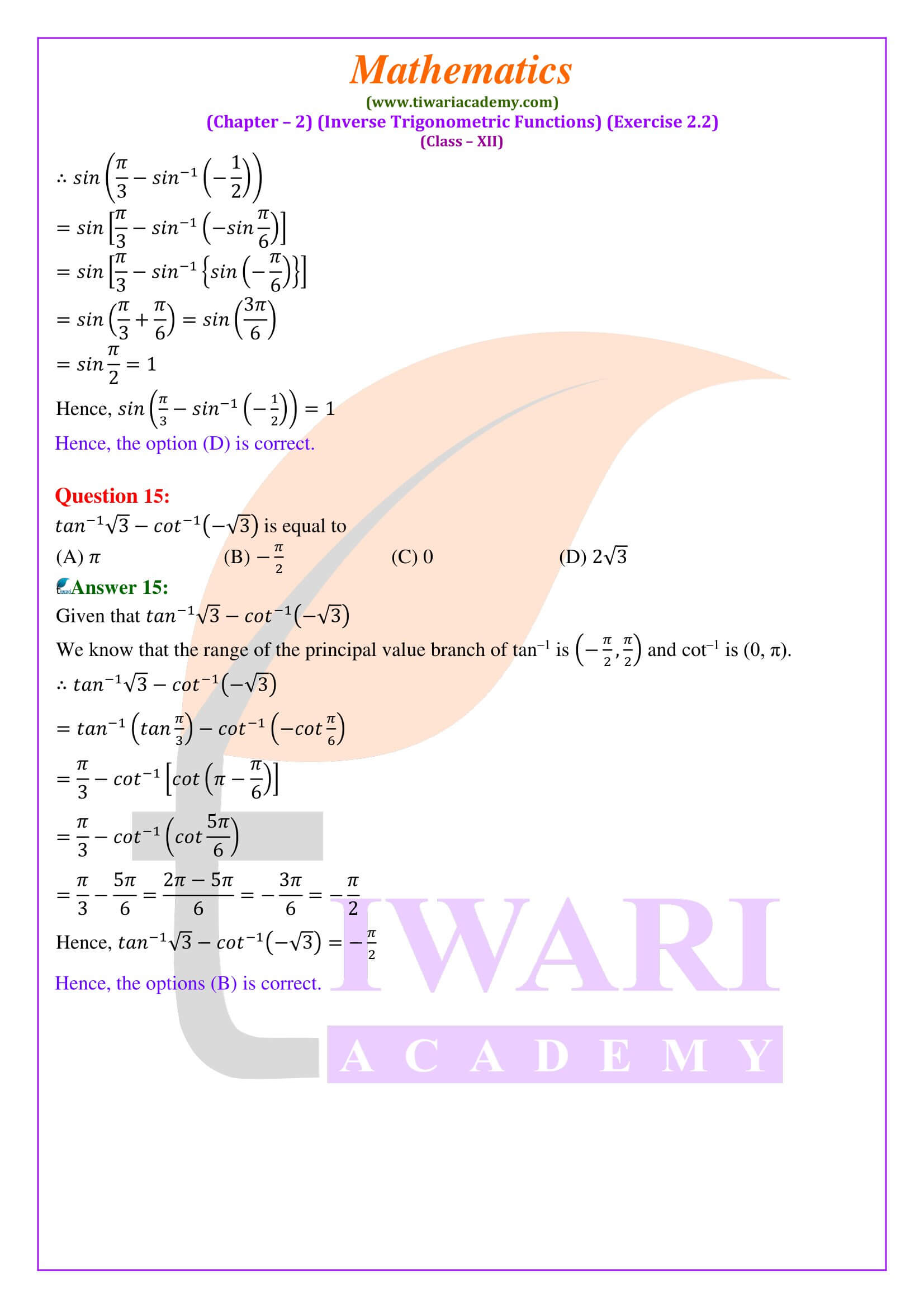
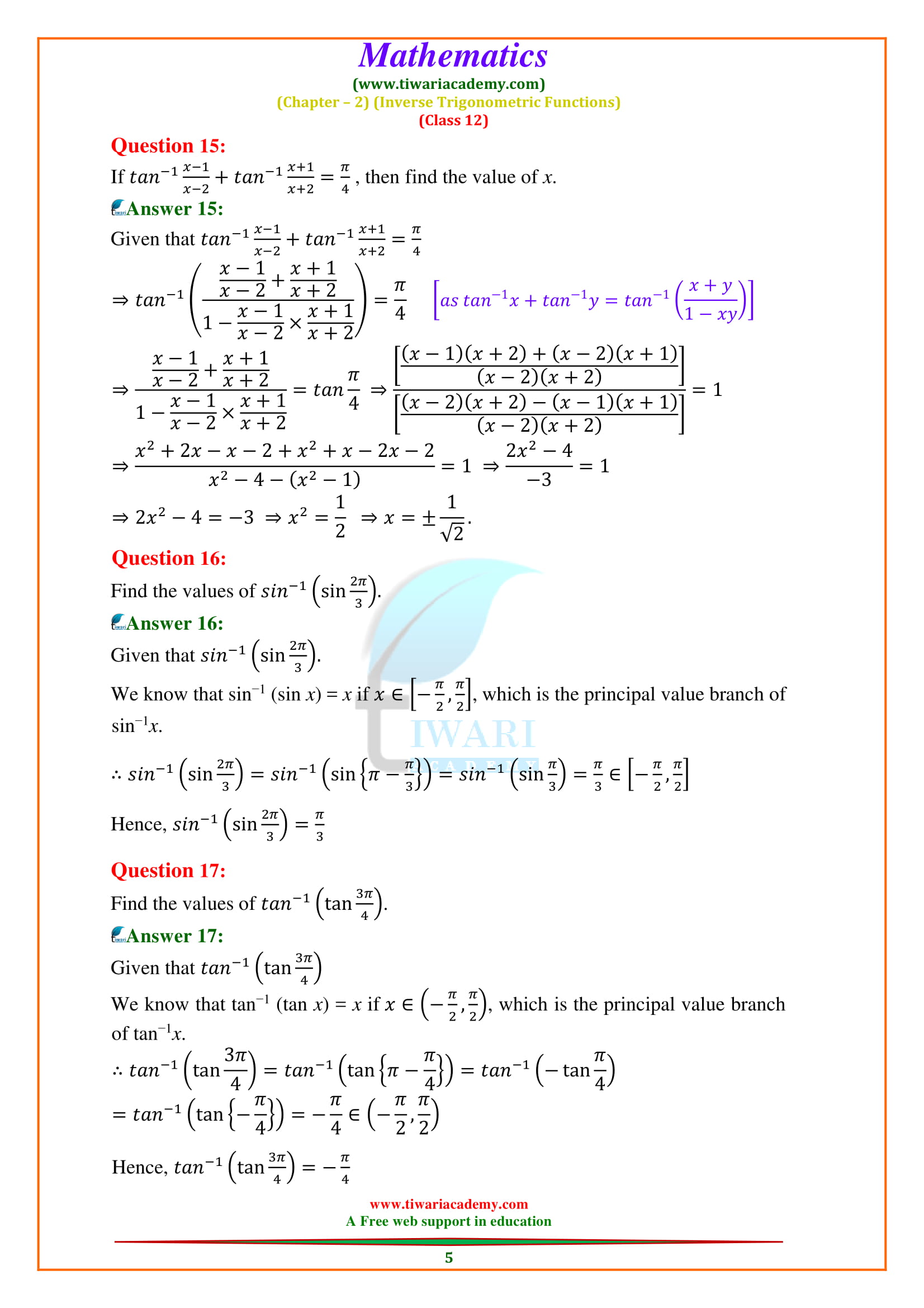
Question number 14 and 15 are the solutions of trigonometric functions. After solving the function, we should test the result whether it is satisfying the equation or not, then we have to finalize the answer.
Feedback and Suggestions
We are uploading NCERT Solutions in Updated form in Hindi Medium and English Medium for all the subject to help the students as much as possible. Our only aim is to assist the students/parents/teacher free of cost at our level best.
If you feel any difficulty, in NIOS or CBSE, during the use of website, please inform us, we will rectify it immediately. Download NCERT Books and offline apps based on latest CBSE Syllabus for new academic session.
Which examples and questions are the most important in exercise 2.2 of class 12th Maths for the board first term exams?
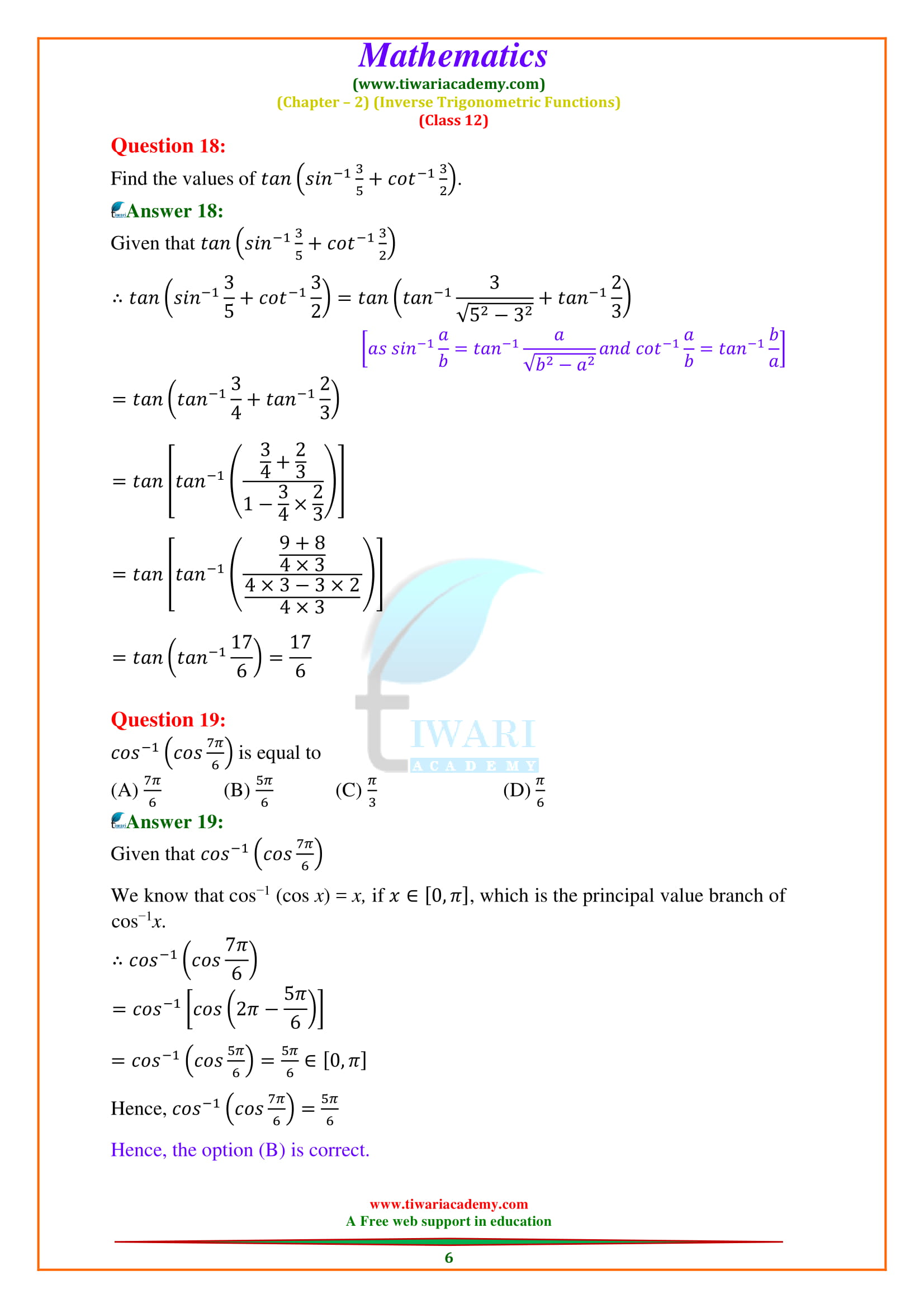
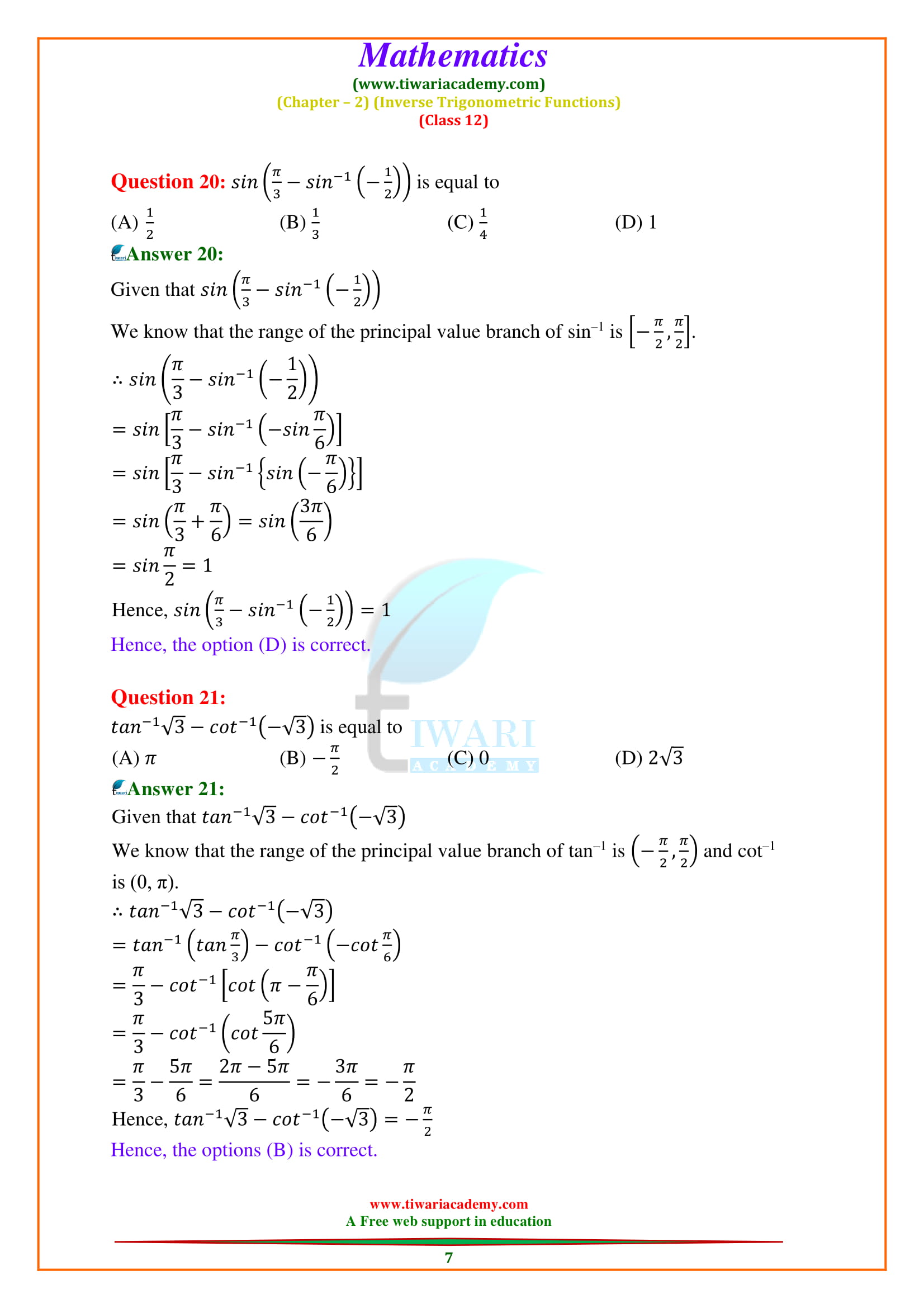
Exercise 2.2 of class 12th Maths has 6 examples (Examples 3, 4, 5, 6, 7, 8) and 21 questions. All the examples and questions of this exercise are essential to do. But examples 3, 5, 6 and questions 1, 2, 3, 4, 5, 6, 7, 8, 10, 12, 13, 14, 15, 18, 19, 20, 21 of exercise 2.2 of class 12th Maths are the most important examples and questions for the board exams because these examples and questions have already been asked in the exams several times.
How many days are needed to complete exercise 2.2 of grade 12th Maths?
If students can give 2 hours per day to exercise 2.2 of class 12th Maths, they need 3-4 days to complete exercise 2.2 of class 12th Maths. This time is an approximate time. This time can vary because no students can have the same working speed, efficiency, capability, etc.
Is exercise 2.2 of class 12th Maths complex for first term exam?
Exercise 2.2 of class 12th Maths is not easy and not complex. It lies in the mid of easy and tough because some examples and questions of this exercise are easy, and some are complicated. However, the difficulty level of any topic/question varies from child to child. So, exercise 2.2 of class 12th Maths is easy, or tough depends on children also. Some children find it difficult, some find it easy, and some find it in the middle of easy and difficult.
What are the main topics to focus in exercise 2.2 of class 12th Maths?
In exercise 2.2 of class 12th Maths, students will learn properties of inverse trigonometric functions and solve questions with the help of these properties.
Properties of inverse trigonometric functions are:
- 1. (i) sin⁻¹(1/x) = cosec⁻¹x, x ≥ 1 or x ≤ – 1
(ii) cos⁻¹(1/x) = sec⁻¹x, x ≥ 1 or x ≤ – 1
(iii) tan⁻¹(1/x) = cot⁻¹x, x > 0 - 2. (i) sin⁻¹(–x) = – sin⁻¹x, x ∈ [– 1, 1]
(ii) tan⁻¹(–x) = – tan⁻¹x, x ∈ R
(iii) cosec⁻¹(–x) = – cosec⁻¹x, | x | ≥ 1 - 3. (i) cos⁻¹(–x) = π – cos⁻¹x , x ∈ [– 1, 1]
(ii) sec⁻¹(–x) = π – sec⁻¹x, | x | ≥ 1
(iii) cot⁻¹(–x) = π – cot⁻¹x, x ∈ R - 4. (i) sin⁻¹x+ cos⁻¹x = π/2 , x ∈ [– 1, 1]
(ii) tan⁻¹x+ cot⁻¹x = π/2, x ∈ R
(iii) cosec⁻¹x+ sec⁻¹x = π/2, | x | ≥ 1 - 5. (i) tan⁻¹x + tan⁻¹y = tan⁻¹(x + y/1 – xy) , xy < 1 (ii) tan⁻¹x – tan⁻¹y = tan⁻¹(x – y/1 + xy) , xy > – 1
(iii) tan⁻¹x+ tan⁻¹y = π + tan⁻¹(x + y/1 – xy), xy > 1; x, y > 0 - 6. (i) 2 tan⁻¹x = sin⁻¹(2x/1+ x²) , | x | ≤ 1
(ii) 2 tan⁻¹x = cos⁻¹(1- x²/1+ x²), x ≥ 0
(iii) 2 tan⁻¹x = tan⁻¹(2x/1-x²), – 1 < x < 1