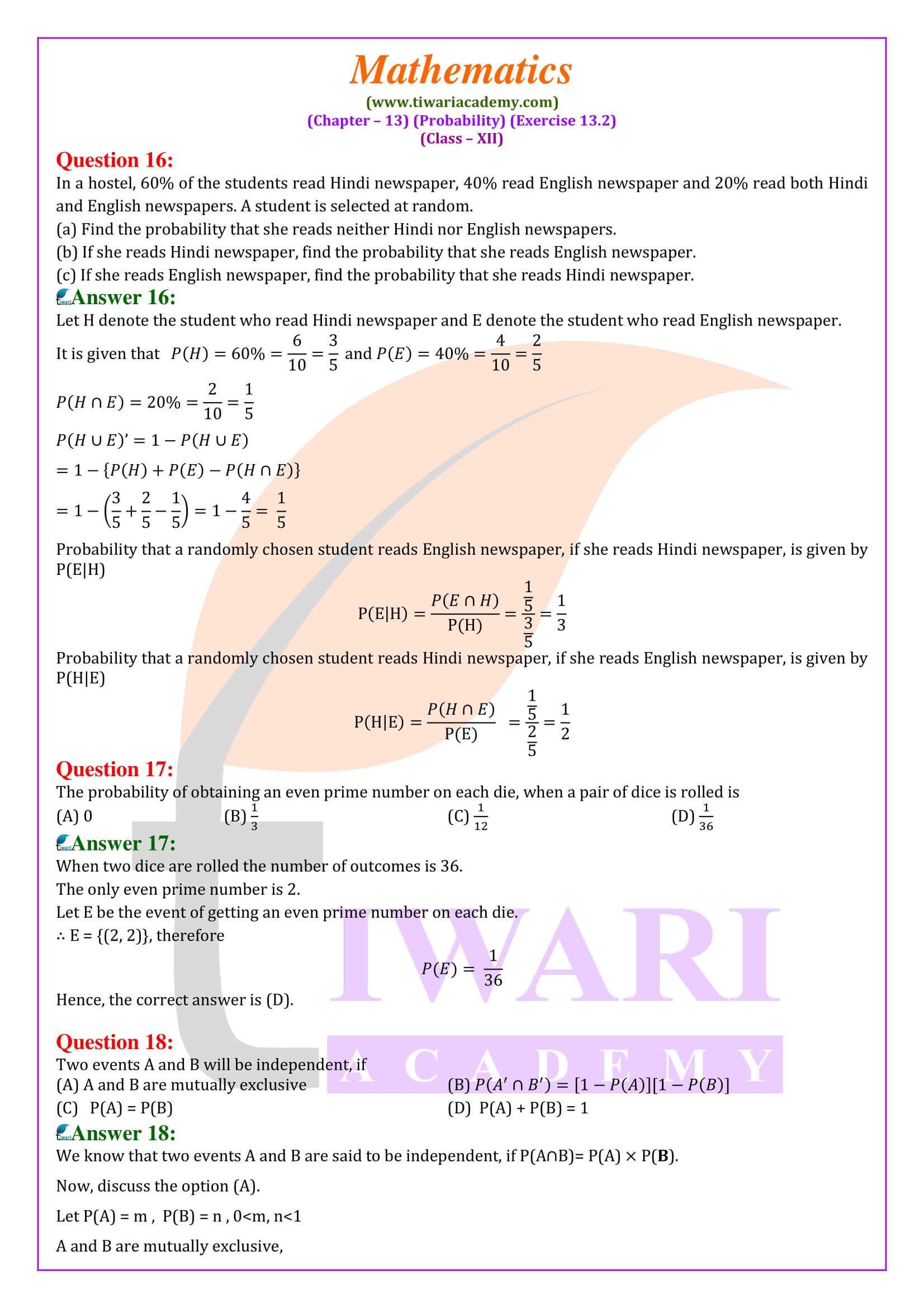
NCERT Solutions for Class 12 Maths Chapter 13 Exercise 13.2 in Hindi and English Medium for academic session 2025-26. All the question answers and explanation of solutions are revised according to new NCERT textbook issued for CBSE Exams 2025-26.
Class 12 Maths Exercise 13.2 in Hindi and English Medium
Class 12 Maths Chapter 13 Exercise 13.2 Solutions
All the contents like PDF solutions and Video solutions are free to use without any registration. No login is required to access the content. We have updated our contents even for state boards like UP board, MP board and other state boards.
| Class: 12 | Mathematics |
| Chapter: 13 | Exercise: 13.2 |
| Topic Name: | Probability |
| Content: | NCERT Exercise Solution |
| Content Type: | Text and Videos Online |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English |
Class 12 Maths Chapter 13 Exercise 13.2 in Videos
Conditional Probability
For mobile, download Class 12 Maths App in English Medium and Class 12 Ganit App in Hindi Medium. So far, in probability, we have discussed ways of finding the probability of events. If we have two events from the same sample location, does information about the occurrence of one event affect the probability of another event? Let us try to answer this question by doing a random experiment in which the result is equally likely. Consider the use of tossing three appropriate coins.
The sample space for the experiment contains 8 digits. Now suppose that the first coin is given a tail, what is the probability of having two heads? With information on the occurrence of the first coin, we believe that cases in which the first coin does not result should be considered when the possibility of two heads is explored. This information reduces our sample space. In other words, the additional information actually tells us that the condition can be considered as a new random experiment for which the sample space contains only those results that are compatible with the occurrence of the event.
Mean of a Random Variable
The mean or expectation of a random variable x is the sum of the products of all possible values of x by their respective probabilities.
Why do we find variance?
The mean of a random variable does not give us information about the variability in the values of the random variable. In fact, if the variance is small, the values of the random variable are close to the mean. Random variables with different probability distributions may have similar means.