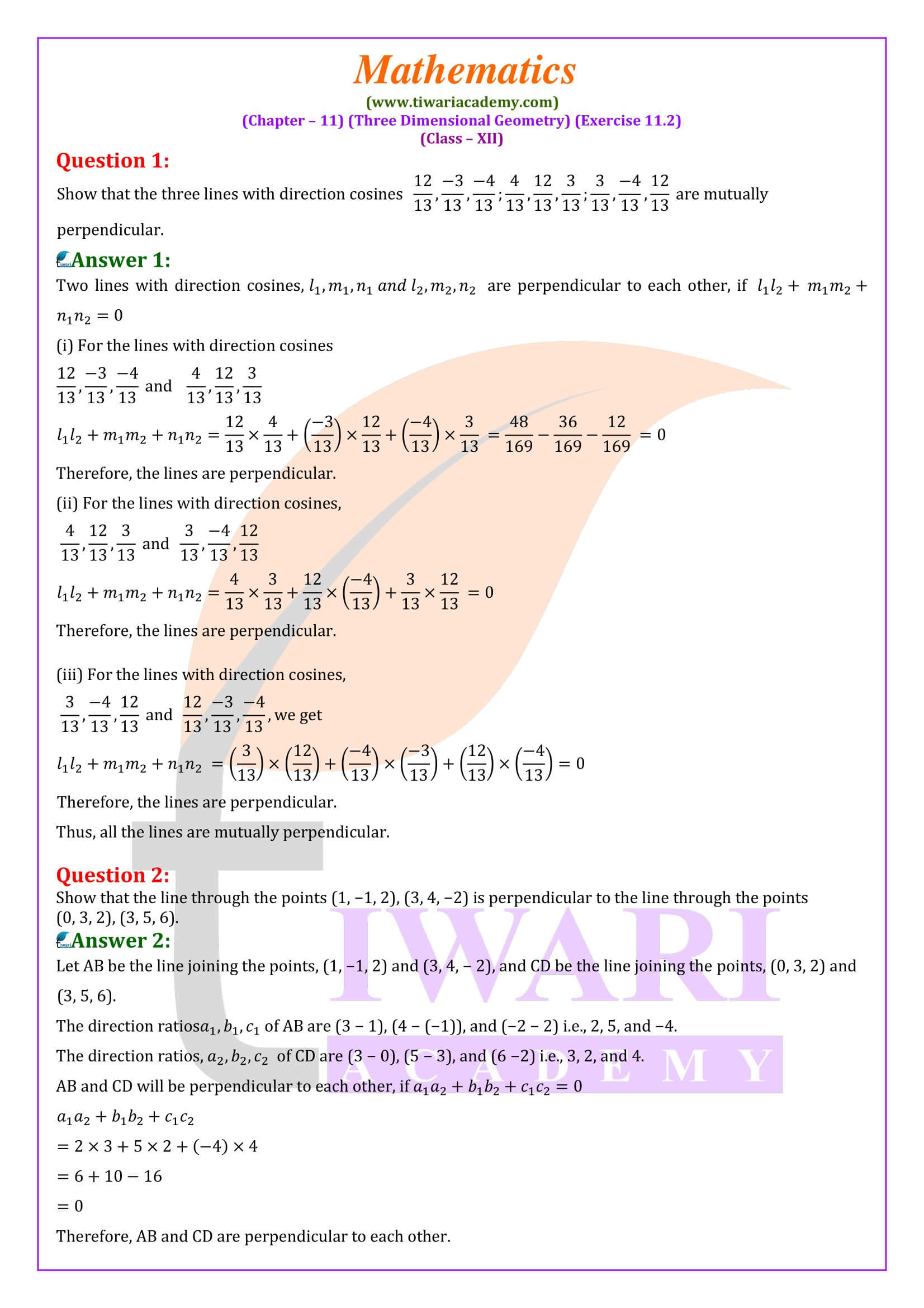
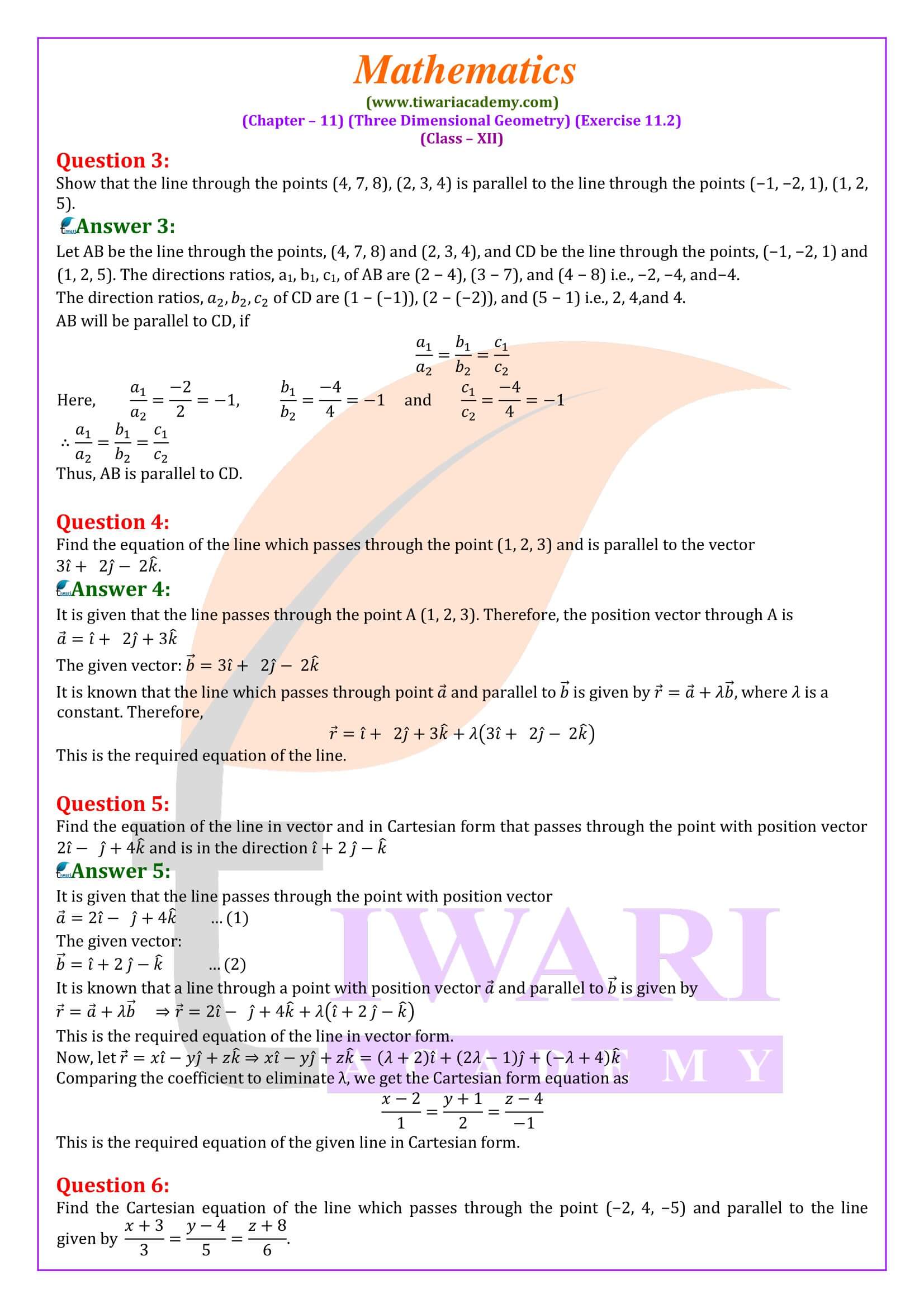
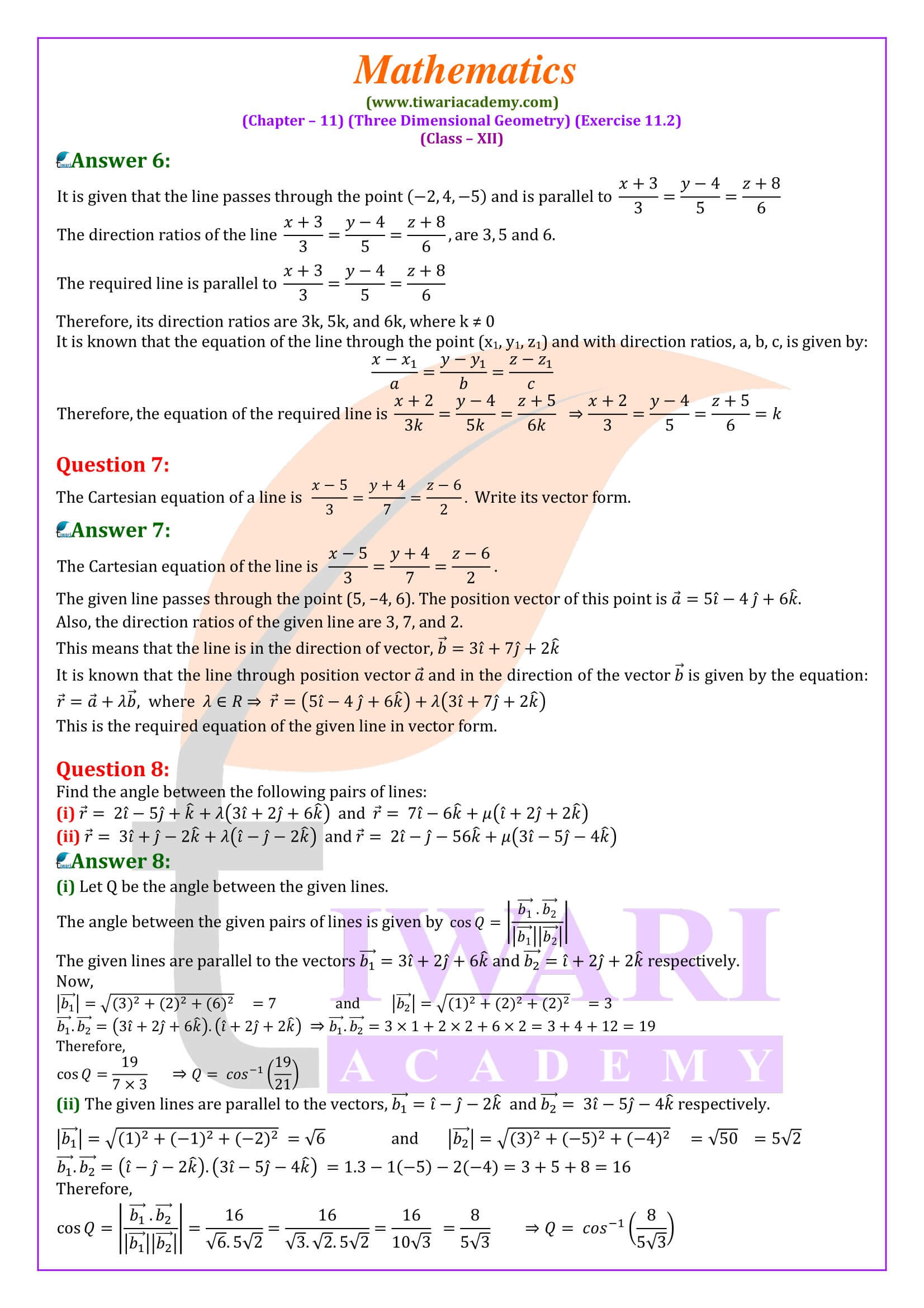
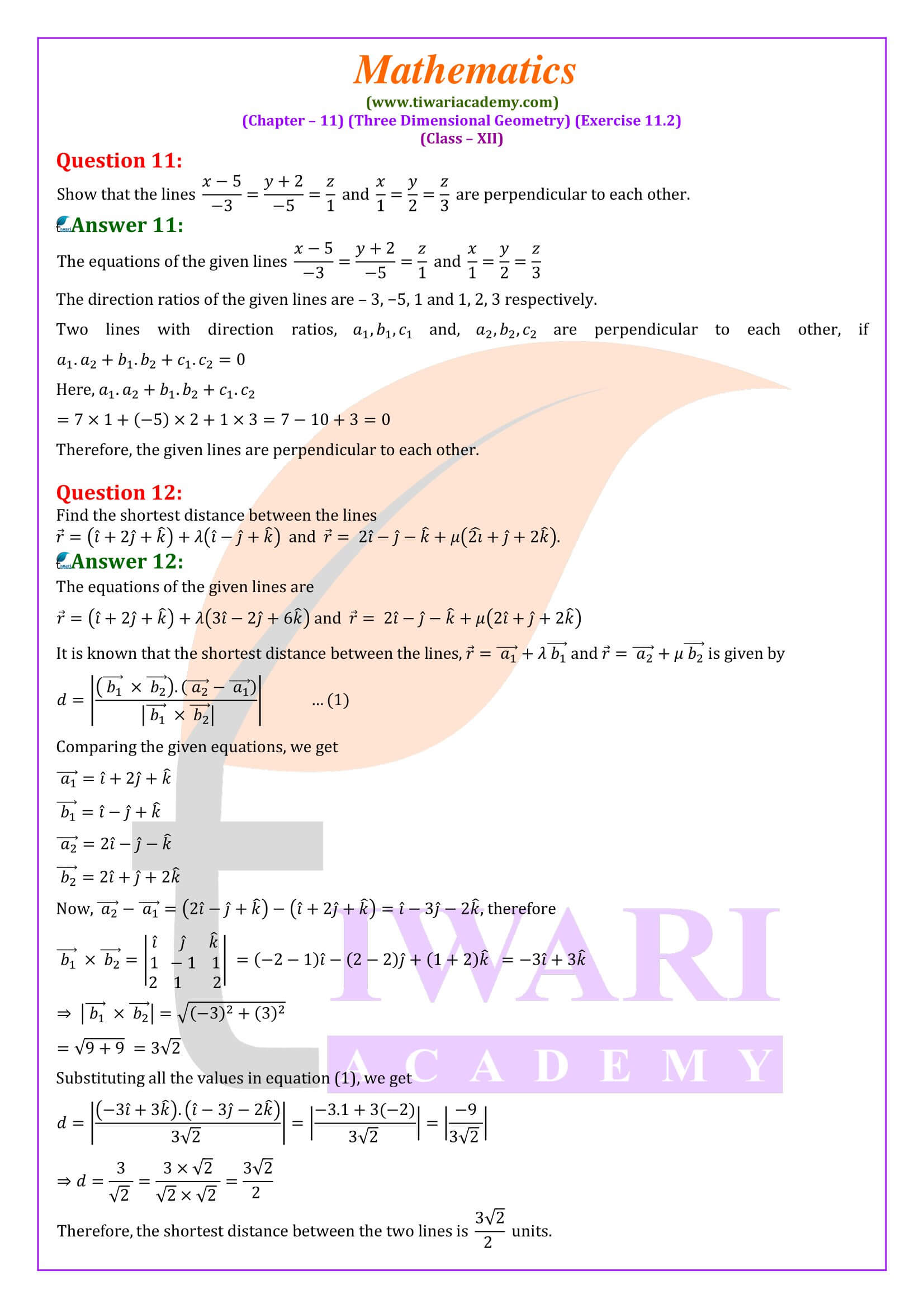
NCERT Solutions for Class 12 Maths Chapter 11 Exercise 11.2 of 3D Three Dimensional Geometry for CBSE 2025-26 exams. Questions of Ex. 11.2 of 12th Maths are revised as per rationalised syllabus and new textbook issued for academic session 2025-26.
Class 12 Maths Exercise 11.2 in Hindi and English Medium
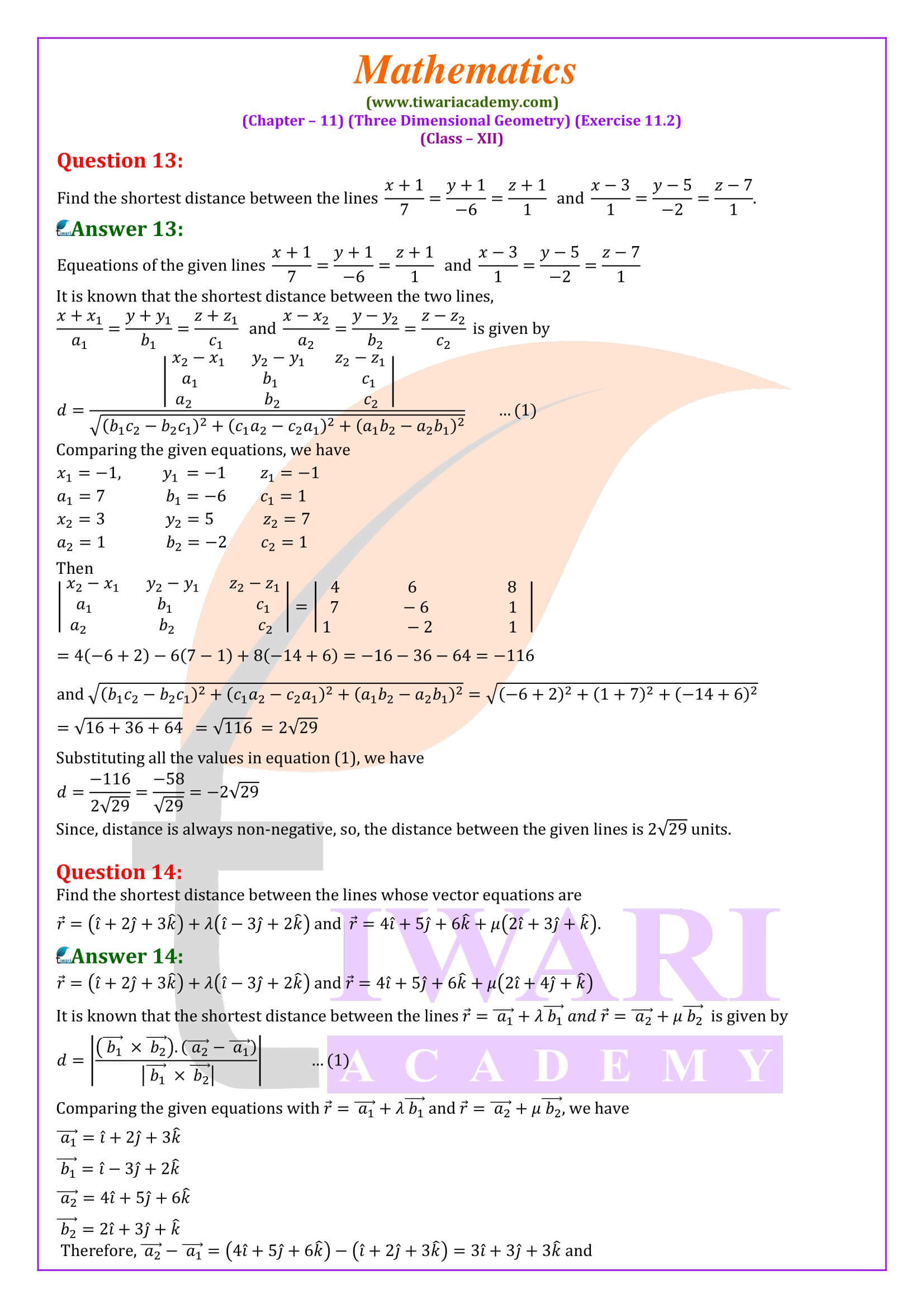
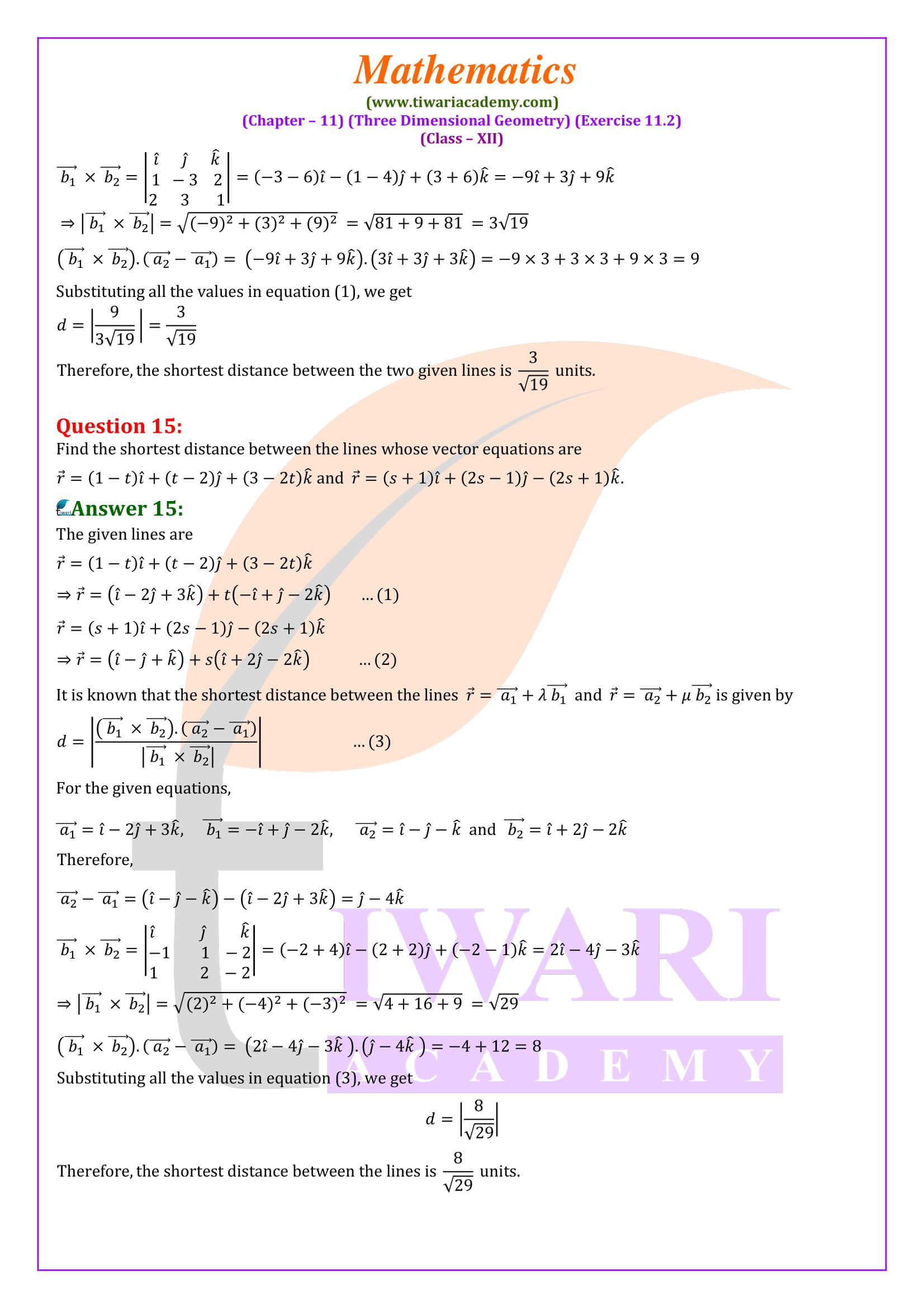
Class 12 Maths Chapter 11 Exercise 11.2 Solutions
| Class: 12 | Mathematics |
| Chapter: 11 | Exercise 11.2 |
| Topic Name: | Three Dimensional Geometry |
| Contents: | Exercise and Extra Questions |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English |
CBSE NCERT Solutions for Class 12 Maths Exercise 11.2
The NCERT Solutions for Class 12 Maths Chapter 11 Exercise 11.2 updated for session 2025-26 are given here in Hindi and English Medium free to use. Videos based on Hindi Medium and English Medium of Exercise 11.2 are available on the page free to download or use online. 12th Maths exercise 11.2 solutions for CBSE and state boards. All the contents are available in Hindi and English Medium free to use online or download in PDF file format free without any login or password.
Class 12 Maths Chapter 11 Exercise 11.2 Solutions in Videos
Elements of 3-D Geometry
A line given in space can extend in two opposite directions, thus it contains two-direction cosines. For a given line in space to have a unique set of direction cosines, we must take the given line as a directed line. These unique direction cosines are denoted by L, M and N. If the given line in space does not pass through the origin, to find its cosine direction, we draw a line through the origin and are parallel to the given line.
How to find a Line in 3-D
Now take one of the lines directed from the origin and find its direction cosine, because the two parallel lines have the same set of direction cosines. Any three numbers that are proportional to the direction corners of a line are called the direction relationships of the line. If l, m, n are directions and a, b, c are the direction relations of a line, then a = λl, b = λm and c = λn, for any nonzero real number λ.
Angle between two lines
Explain that L1 and L2 are two lines that pass through the origin and the direction relation a1, b1, c1 and a2, b2, c2 respectively. Explain that P is a point on L1 and Q is a point on L2. Consider the directed lines OP and OQ. Let A be the acute angle between OP and OQ. Now remember that the directed line segments OP and OQ are vectors with components A1, B1, C1 and A2, B2, C2 respectively. Therefore, the angle A between them is given by the formula CosA.
What are the main concepts on which class 12 Maths Exercise 11.2 is based?
In class 12 Maths Exercise 11.2, we will learn how to find the equation of a line in vector as well as Cartesian plane, angle between two lines and distance between the two lines.
Which is the most important question in Exercise 11.2 of 12th Maths?
Questions number 12 and 15 are the asked so many times in board exams. So, these are important and tricky as well.