Class 11 Maths Exercise 1.3 Solutions
Class 11 Maths Exercise 1.3 in Hindi
Class 11 Maths Books Download in PDF
Class 11 Maths Chapter 1 NCERT Solutions
NCERT Solutions for Class 11 Maths
NCERT Solutions for Class 11 Maths Exercise 1.3 Sets in Hindi and English Medium updated and modified for academic session 2025-26. Get here revised solutions of ex. 1.3 grade 11th mathematics based on textbooks issued by NCERT for 2025-26 examinations.
| Class: 11 | Mathematics |
| Chapter: 1 | Exercise: 1.3 |
| Topic: | Sets |
| Content: | NCERT Exercise Solutions |
| Medium: | Hindi and English Medium |
Class 11 Maths Exercise 1.3 Solutions in Hindi and English Medium
NCERT Solutions for Class 11 Maths Exercise 1.3
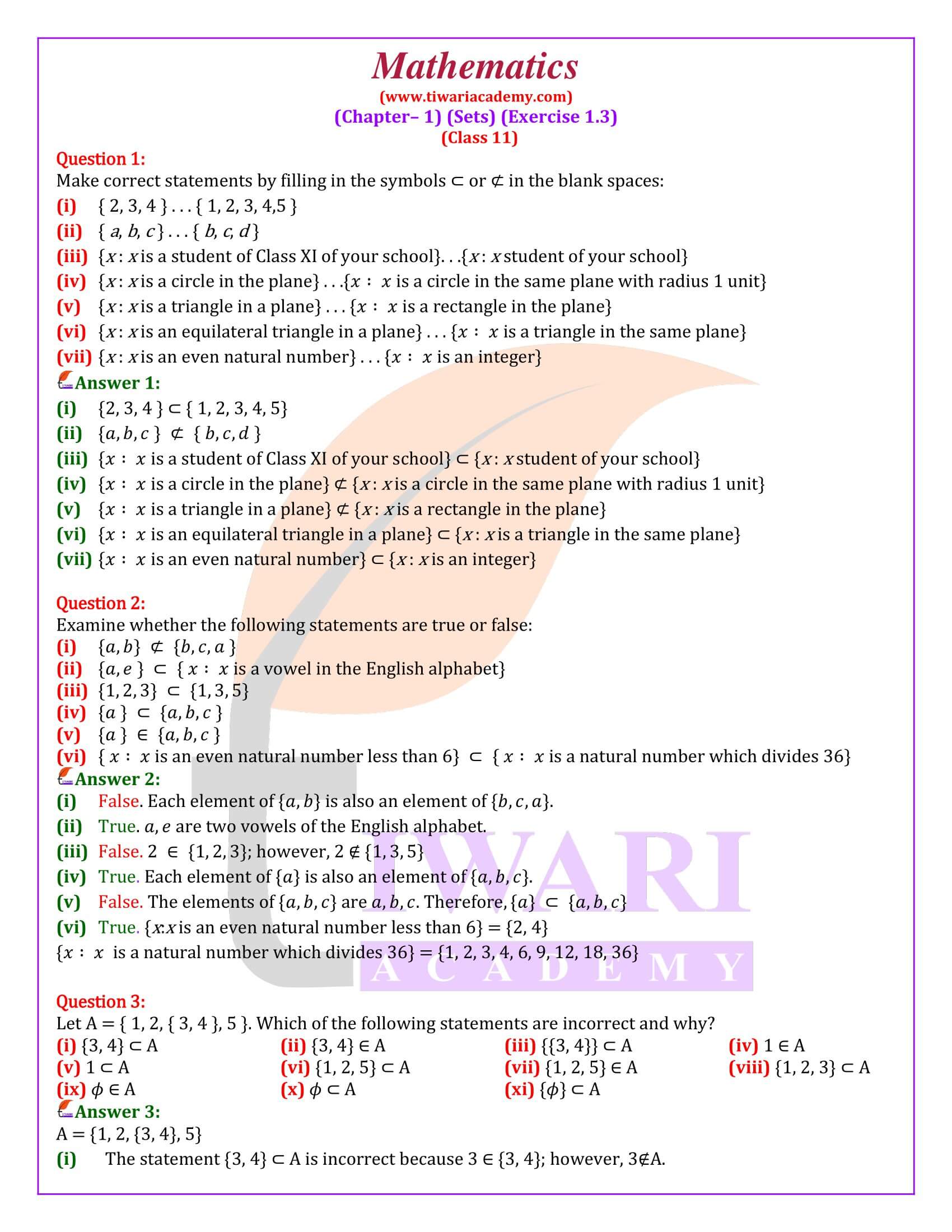
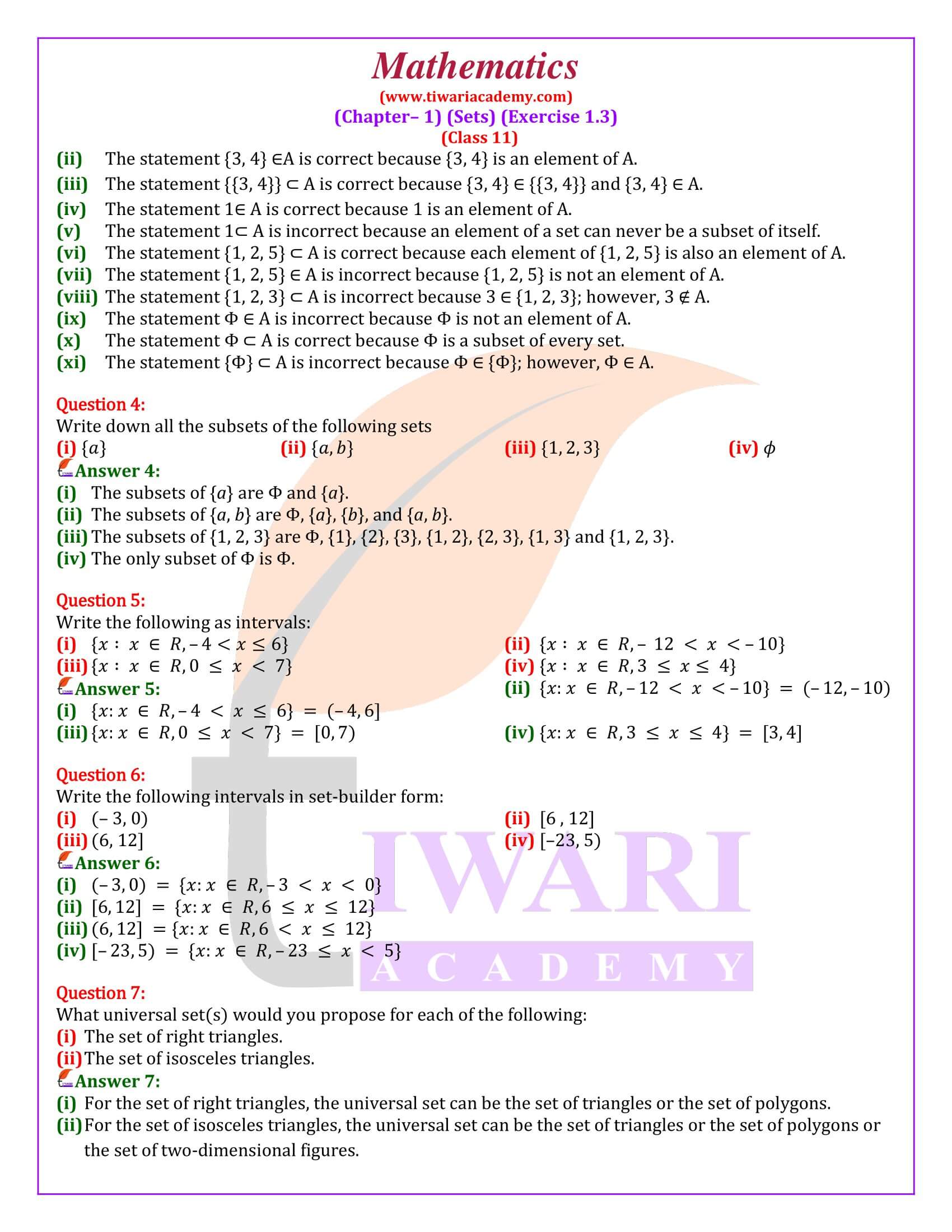
All the questions given in Exercise 1.3 of class 11 Maths are easy to solve. The solutions PDF and Videos are given here to better understanding.
Sets and Sub sets in Class 11 Maths Exercise 1.3
Since you have completed two of the major concepts in the sets, now you will study the subsets. Subsets are easy as its property imply makes things much easier to understand. You may feel complex to imply symbols. Understanding the meaning symbols are pretty easy. As per 11th NCERT book words, set A is said to be a subset of a set B, if each element of A is available in the element of B. Makes A ⊂ B. Implying this fact, if a ∈ A ⇒ a ∈ B. Now, if you see there are lots of symbols and if you translate in to words it will make a long sentence.
Subsets of real numbers in Exercise 1.3
Before you consider studying different parts of the Subset, consider the revision and take note of the some of the properties:
- Each set is a subset of the given set itself. This means A ⊂ A, or B ⊂ B, etc.
- Null set can work as a subset for any set.
- If B has a A as a subset, then A is contained in B.
- Implying the above statement makes Set B a superset of A.
Denoting Numbers as a Symbol in Exercise 1.3
These properties will help you in the upcoming paragraph of 11th Mathematics i.e. subsets of Real numbers. We will normally indicate natural numbers, real numbers, integers, whole numbers, rational, irrational numbers, and all in the form of symbols. The entire introduction is all about that where you will be taught to use another sign for the identification of numbers.
Set of all possible Subsets
A power set is described as the set of all the possible subsets of the set given. The definition seems a little confusing but in reality, power sets can be very easily understood by following CBSE textbook example.
Here is another example – set A = {2, 3}, Possible Subsets of A = { }, {2}, {3}, {2, 3}, and here the power set of A = P (A) = { { }, {2}, {3}, {2, 3}}. Here, some of the easier questions in the exercise 1.3 given as part of question number 1, 3 and 5. Rest of the questions are also not difficult but tricky.