To find the height of the poles and the distances from the point to each pole, we use trigonometry. Let’s denote the height of the poles as h and the distances from the point to the poles as d1 and d2.
For the Pole with a 60° Angle of Elevation: Using tan(60°) = √3, the equation is √3 = h/d1.
For the Pole with a 30° Angle of Elevation: Using tan(30°) = 1/√3, the equation is 1/√3 = h/d2.
Since the total width of the road is 80 m, d1 + d2 = 80 m. Solving these equations simultaneously, we find h = 40√3 m approximately 69.28 m), d1 = 40, and d2 = 40 m. Therefore, the height of the poles is about 69.28 meters, and the distances from the point to each pole are both 40 meters.
Let’s discuss in detail
Trigonometric Applications in Urban Planning
Trigonometry, an essential branch of mathematics, is often applied in urban planning and design, particularly in situations involving measurements and spatial relationships. A common application is determining the heights of structures and distances between them when direct measurement is not feasible. The problem at hand involves two poles of equal height on opposite sides of an 80-meter-wide road. From a point on the road, the angles of elevation to the tops of these poles are given. This scenario is a practical demonstration of how trigonometry can be used to solve real-world problems in urban and civil engineering.
Understanding the Problem: Two Poles and a Road
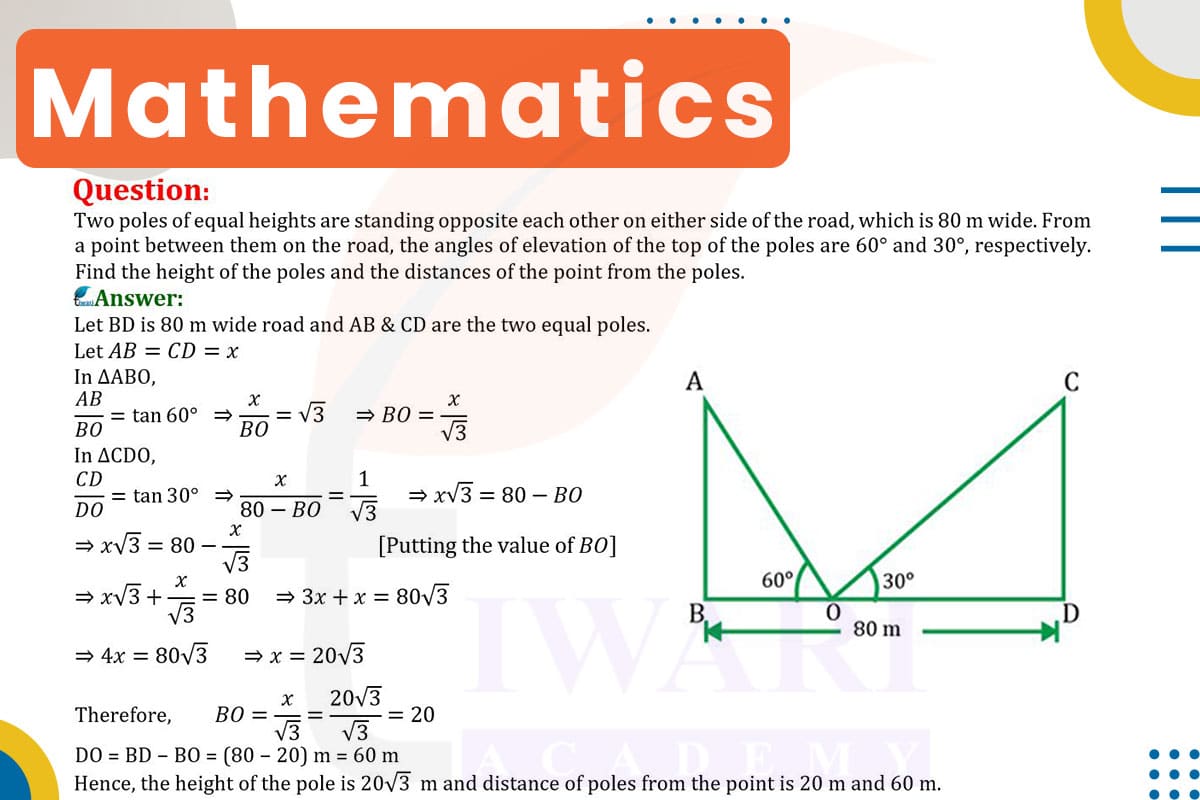
The problem presents two poles of equal height standing on either side of a road that is 80 meters wide. From a point on the road, the angles of elevation to the top of the poles are 60° and 30°, respectively. The objective is to determine the height of the poles and the distances from the point to each pole. This setup forms two right-angled triangles, one with each pole, sharing a common horizontal distance (the width of the road).
The Role of Tangent in Angle of Elevation
In trigonometry, the tangent of an angle in a right-angled triangle is the ratio of the length of the opposite side (height of the pole) to the length of the adjacent side (distance from the point to the pole). By applying the tangent function to the given angles of elevation, we can calculate the heights of the poles and the distances from the point to each pole.
Calculating the Distances to the Poles
First, we use the tangent function for the 60° angle of elevation to find the distance to one pole. The equation is tan(60°) = √3 = h/d1, where d1 is the distance to the first pole. Similarly, for the 30° angle of elevation to the other pole, the equation is tan(30°) = 1/√3 = h/d2, where d2 is the distance to the second pole.
Solving for the Height and Distances
Since the total width of the road is 80 meters, we have d1 + d2 = 80 meters. Solving the equations √3 = h/d1 and 1/√3 = h/d2 simultaneously with d1 + d2 = 80 meters, we find the height of the poles and the distances from the point to each pole.
Practical Implications of Trigonometry in Measurement
The solution reveals that the height of the poles is approximately 69.28 meters, and the distances from the point to each pole are both 40 meters. This example illustrates the practical application of trigonometry in urban and civil engineering, demonstrating how it can provide accurate measurements in complex scenarios. Trigonometry proves to be an invaluable tool in urban planning, offering a mathematical approach to spatial problem-solving where direct measurement is not possible.
Discuss this question in detail or visit to Class 10 Maths Chapter 9 for all questions.
Questions of 10th Maths Exercise 9.1 in Detail

