A kite is flying at a height of 60 m above the ground. The string

Updated on December 4, 2023
by Tiwari Academy

To find the length of the kite string, we use trigonometry, specifically the cosine function. The cosine of an angle in a right-angled triangle is the ratio of the adjacent side (height of the kite above the ground) to the hypotenuse (length of the string).
Given: Height of the kite = 60 m, Angle of inclination = 60°. The cosine of 60° is 1/2.
Using the formula cos(60°) = (60 m)/(length of the string), we have 1/2 = 60/length.
Solving for the length of the string, we get length of the string = 60 × 2, which is 120 meters. Therefore, the length of the string is 120 meters.

A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60°. Find the length of the string, assuming that there is no slack in string.

Let’s discuss in detail

Trigonometric Applications in Kite Flying

Trigonometry, a branch of mathematics, finds its application in various real-life scenarios, including the simple joy of kite flying. The problem at hand involves determining the length of a kite string when the kite is flying at a certain height and the string makes a specific angle with the ground. This scenario is a perfect example of how trigonometry can be used to solve practical problems. By applying trigonometric functions, one can calculate distances and lengths that are not directly measurable, such as the length of a kite string in flight.

Understanding the Kite Flying Scenario

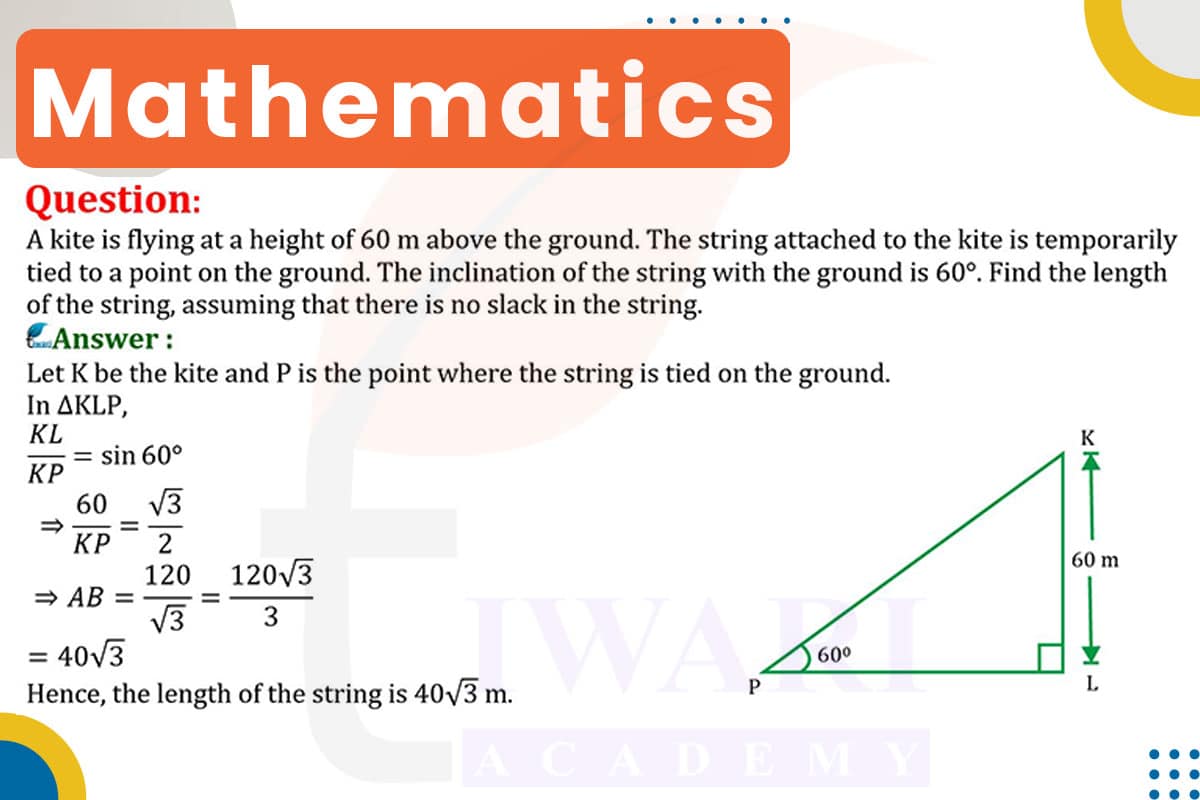
In this scenario, a kite is flying at a height of 60 meters above the ground. The string of the kite is tied to a point on the ground, forming an angle of 60° with it. This setup creates a right-angled triangle with the ground, the kite string, and the height of the kite. The challenge is to determine the length of the kite string, assuming it is taut and forms a straight line from the point where it’s tied to the kite itself.

The Role of Cosine in Trigonometry

To solve this problem, we use the cosine function from trigonometry. The cosine of an angle in a right-angled triangle is the ratio of the length of the adjacent side (in this case, the height of the kite) to the length of the hypotenuse (the kite string). The cosine function is particularly useful in scenarios where the length of one side of a triangle is known, and the length of the hypotenuse is to be determined.

Applying the Cosine Function
Applying the cosine function to the 60° angle, we use the formula cos(60°) = (height of the kite)/(length of the string). The cosine of 60° is known to be 1/2. Substituting the known values into the formula, we get 1/2 = (60 m)/(length of the string).

Calculating the Length of the Kite String

Solving the equation for the length of the kite string, we multiply both sides by the length of the string and then divide by 1/2. This gives us length of the string = 60 m × 2, which simplifies to 120 meters. This calculation shows that the kite string is 120 meters long, allowing the kite to reach the height of 60 meters at the given angle.

Trigonometry in Everyday Life

This example illustrates the practical application of trigonometry in everyday activities like kite flying. The ability to calculate lengths and distances using angles is a powerful tool, not just in theoretical mathematics but also in real-life situations. Trigonometry helps bridge the gap between abstract concepts and their practical uses, demonstrating the importance of mathematical principles in understanding and interacting with the world around us. This scenario, in particular, shows how trigonometry can provide solutions to problems that involve indirect measurement, making it an invaluable tool in various fields and hobbies.

Discuss this question in detail or visit to Class 10 Maths Chapter 9 for all questions.
Questions of 10th Maths Exercise 9.1 in Detail

A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30°.

A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree.

A contractor plans to install two slides for the children to play in a park. For the children below the age of 5 years, she prefers to have a slide whose top is at a height of 1.5 m, and is inclined at an angle of 30° to the ground, whereas for elder children, she wants to have a steep slide at a height of 3m, and inclined at an angle of 60° to the ground. What should be the length of the slide in each case?

The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30°. Find the height of the tower.

A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60°. Find the length of the string, assuming that there is no slack in the string.

A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increases from 30° to 60° as he walks towards the building. Find the distance he walked towards the building.

From a point on the ground, the angles of elevation of the bottom and the top of a transmission tower fixed at the top of a 20 m high building are 45° and 60° respectively. Find the height of the tower.

A statue, 1.6 m tall, stands on the top of a pedestal. From a point on the ground, the angle of elevation of the top of the statue is 60° and from the same point the angle of elevation of the top of the pedestal is 45°. Find the height of the pedestal.

The angle of elevation of the top of a building from the foot of the tower is 30° and the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.

Two poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60° and 30°, respectively. Find the height of the poles and the distances of the point from the poles.

A TV tower stands vertically on a bank of a canal. From a point on the other bank directly opposite the tower, the angle of elevation of the top of the tower is 60°. From another point 20 m away from this point on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is 30°. Find the height of the tower and the width of the canal.