To solve this problem, we use trigonometry, specifically the tangent function. Initially, the angle of elevation is 30°, and finally, it becomes 60°. Let’s denote the initial and final distances from the boy to the building as d1 and d2 respectively.
Initial Position (30° angle): Using tan(30°) = 1/√3, the height of the building minus the height of the boy is 30 m − 1.5 m = 28.5 m. So, 1/√3 = 28.5/d1. Solving for d1, we get d1 = 28.5√3.
Final Position (60° angle): Using tan(60°) = √3, we have √3 = 28.5/d2. Solving for d2, we get d2 = 28.5/√3.
The distance walked is d1 − d2 = 28.5√3 − 28.5/√3, which simplifies to approximately 24.75 meters. Therefore, the boy walked about 24.75 meters towards the building.
Let’s discuss in detail
Trigonometric Motion Analysis
Trigonometry, a branch of mathematics, is not only about solving theoretical problems but also about understanding real-world situations, such as the movement of a person in relation to a structure. The problem at hand involves a boy walking towards a building and the consequent change in the angle of elevation from his eyes to the top of the building. This scenario is a classic example of how trigonometry can be applied to analyze motion and distance in a physical context, demonstrating the practical utility of mathematical concepts in everyday life.
Motion Towards a Building
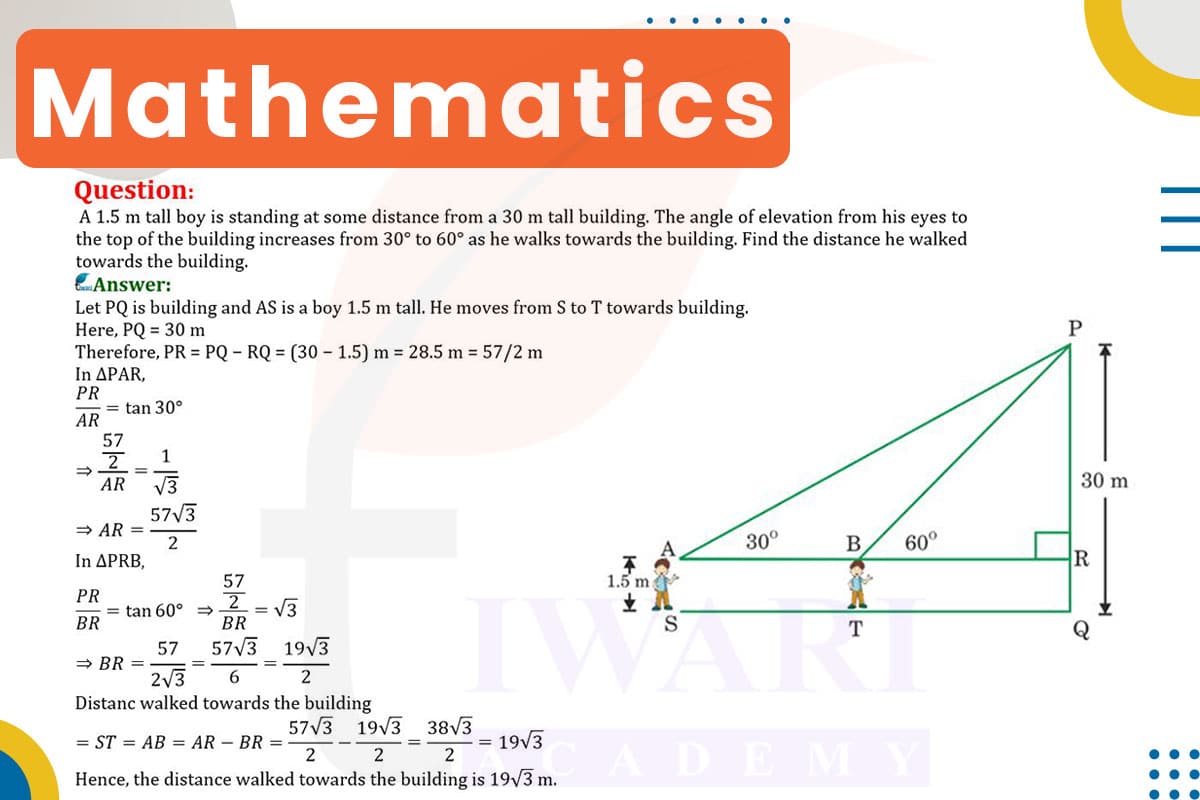
The problem presents a 1.5-meter-tall boy standing at a certain distance from a 30-meter-tall building. As the boy walks towards the building, the angle of elevation from his eye level to the top of the building increases from 30° to 60°. The objective is to calculate the distance the boy has walked towards the building. This scenario forms two different right-angled triangles at different instances – one at the initial position (30° angle) and the other at the final position (60° angle).
The Role of Tangent in Angle of Elevation
In trigonometry, the tangent of an angle in a right-angled triangle is the ratio of the opposite side (in this case, the difference in height between the boy’s eyes and the top of the building) to the adjacent side (the distance from the boy to the building). By applying the tangent function to the angles of elevation, we can determine the boy’s distances from the building at both the initial and final positions.
Calculating the Initial Distance
Initially, when the angle of elevation is 30°, we use the formula tan(30°) = opposite/adjacent. The opposite side is the height difference between the building and the boy’s eyes, which is 30 m − 1.5 m = 28.5 m. The tangent of 30° is 1/√3. Therefore, 1/√3 = 28.5/d1, where d1 is the initial distance. Solving for d1, we find d1 = 28.5√3 meters.
Calculating the Final Distance
At the final position, where the angle of elevation is 60°, we apply the formula tan(60°) = opposite/adjacent. The tangent of 60° is √3. Therefore, √3 = 28.5/d2, where d2 is the final distance. Solving for d2, we find d2 = 28.5/√3 meters.
Determining the Distance Walked
The distance the boy walked towards the building is the difference between the initial and final distances, d1 − d2. Substituting the values, we get 28.5√ − 28.5/√3, which simplifies to approximately 24.75 meters. This calculation not only demonstrates the application of trigonometry in analyzing real-world motion but also highlights the importance of understanding mathematical principles to interpret and solve practical problems. The scenario underscores how trigonometry can provide insights into everyday situations, bridging the gap between abstract mathematics and tangible experiences.
Discuss this question in detail or visit to Class 10 Maths Chapter 9 for all questions.
Questions of 10th Maths Exercise 9.1 in Detail

