by Tiwari Academy
NCERT Solutions for class 10 Maths Chapter 3 Exercise 3.6 in Hindi and English Medium updated for CBSE and other boards. Secure top grades in Class 10 Maths with the newly updated NCERT Solutions for Chapter 3 Exercise 3.6, tailored for CBSE and other educational boards. Offered in both Hindi and English medium, this resource provides expert guidance on advanced topics in Linear Equations in Two Variables. Ideal for comprehensive exam preparation, our solutions align with board guidelines to enhance your learning experience.
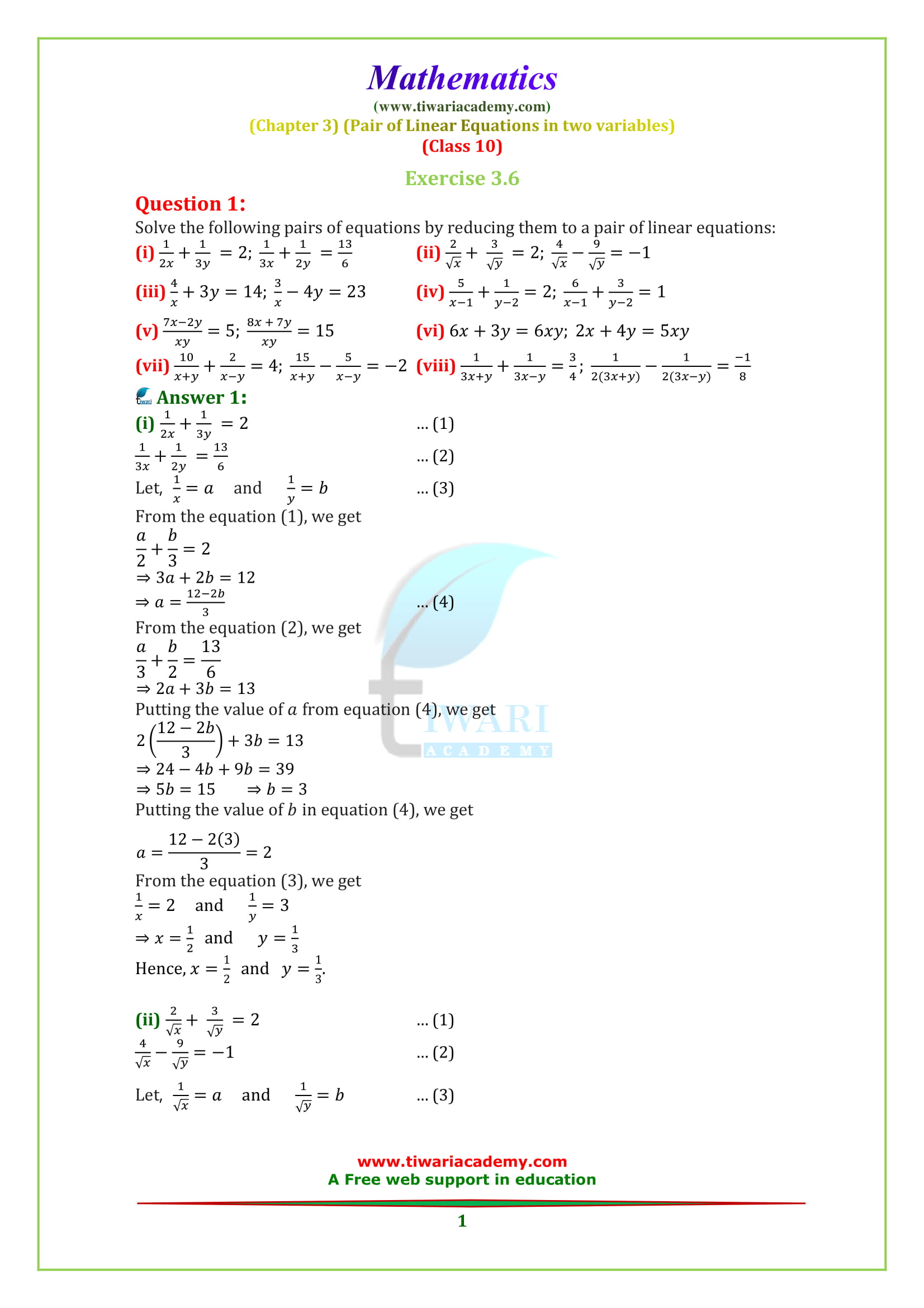
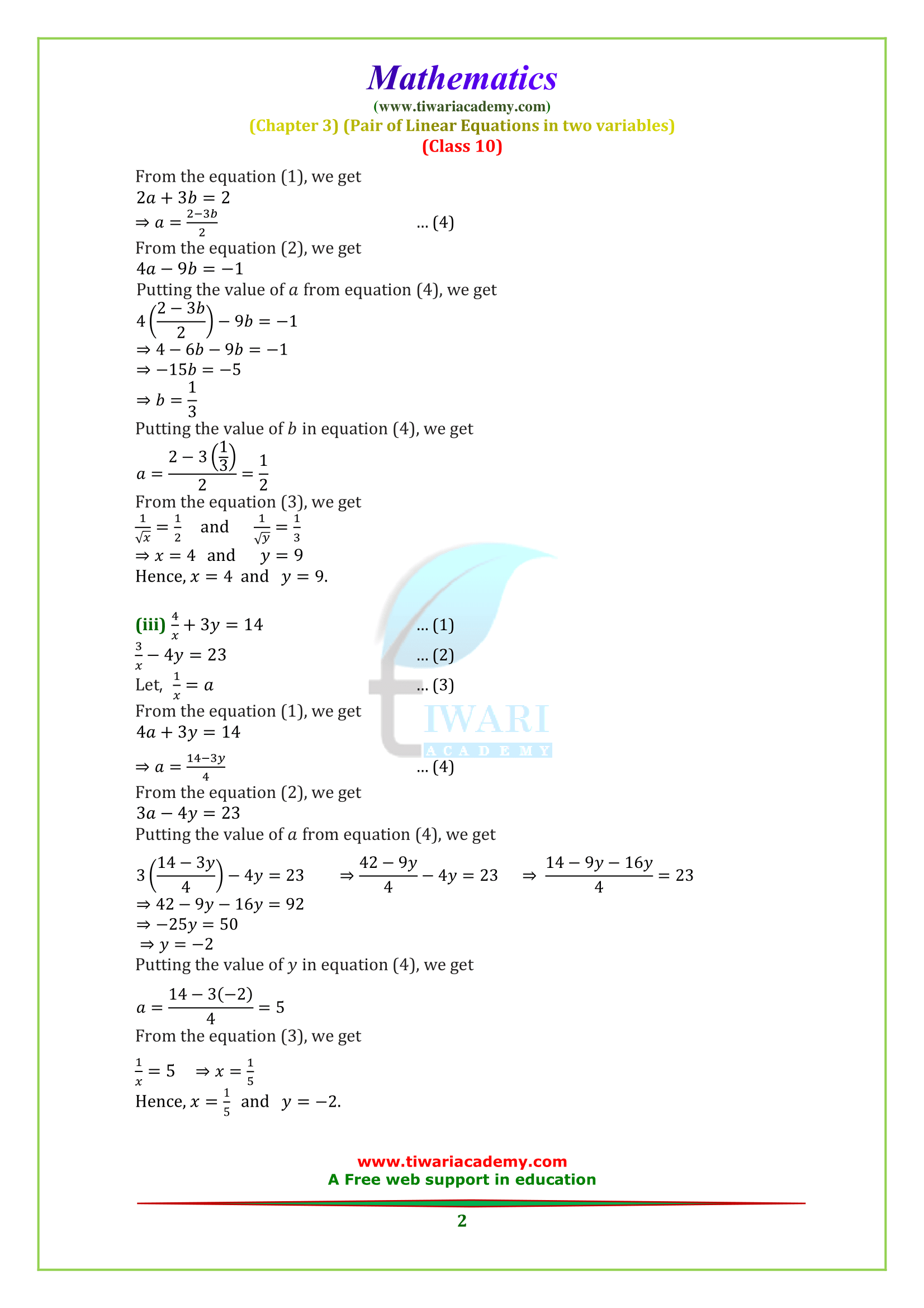
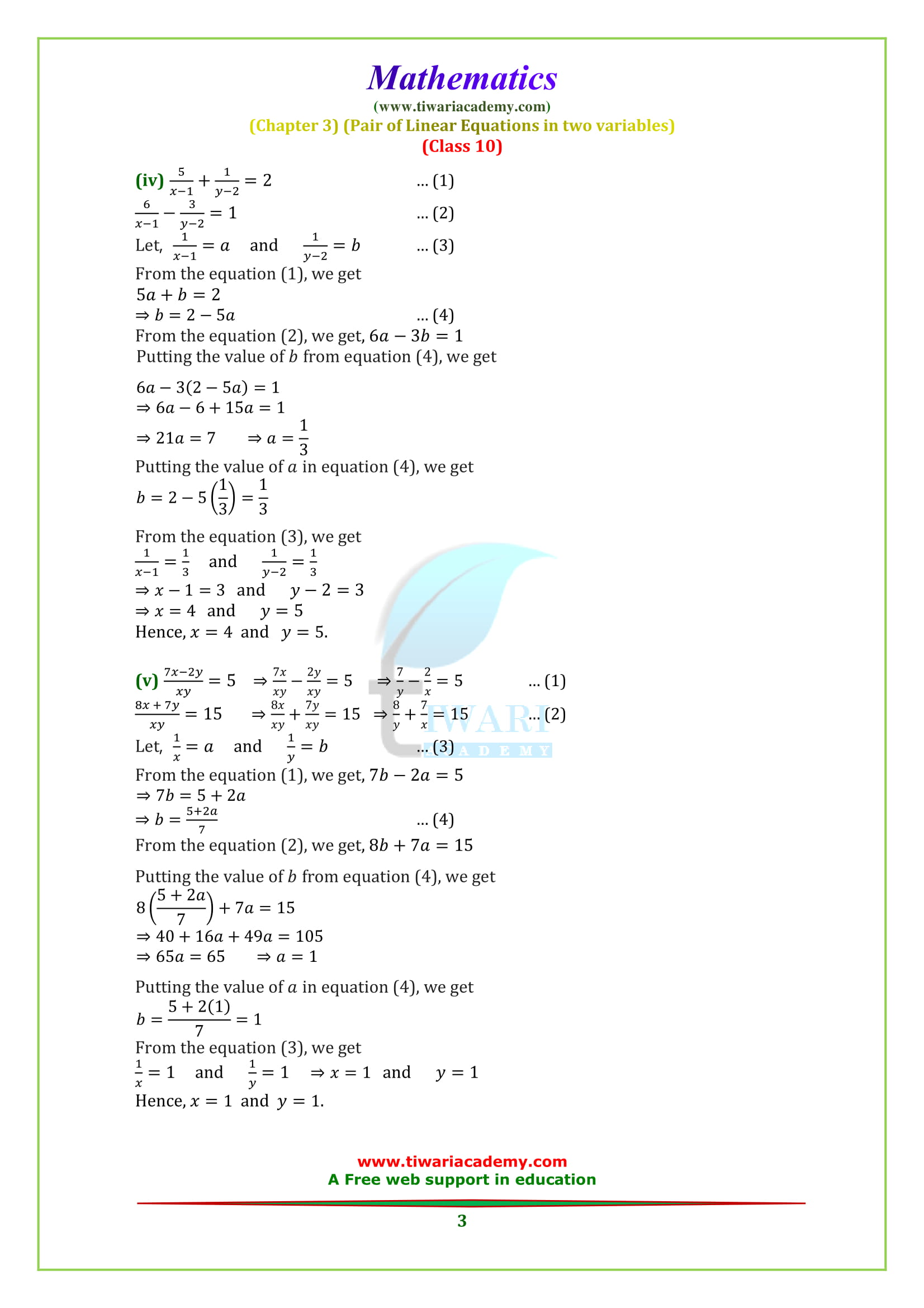
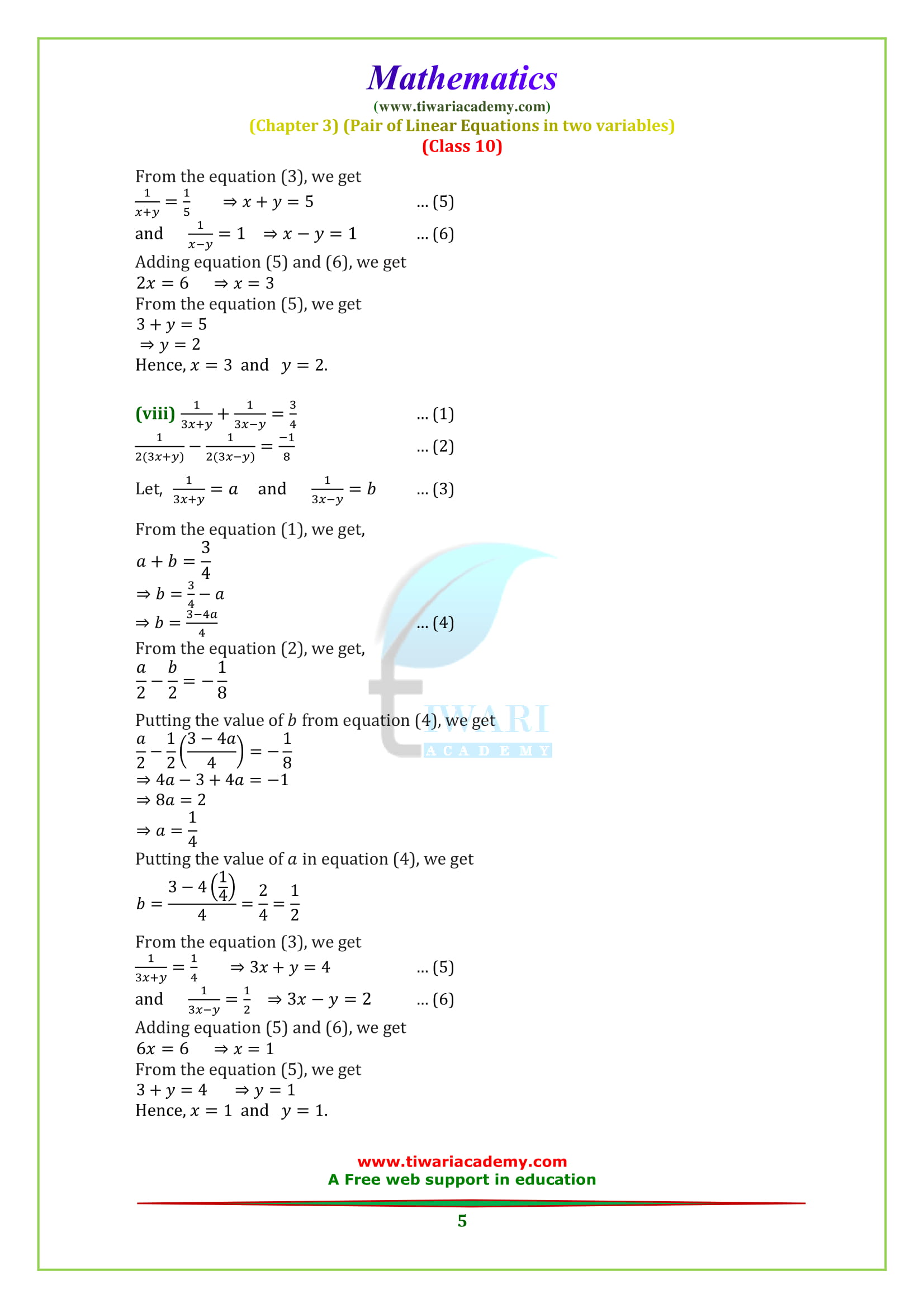
NCERT Solutions for class 10 Maths Chapter 3 Exercise 3.6
Class 10 Ex. 3.6 solution Pair of linear equations in two variables in Hindi Medium and English Medium. 10th Maths CBSE Solutions are also available in Video Format for all board Gujrat Board, UP Board and CBSE board based NCERT Books. UP Board High School students are also using NCERT Textbooks for their course, so they also can use these solutions for their help in Maths of class 10.
Download UP Board Solutions for Class 10 Maths Chapter 3 Exercise 3.6 in Hindi Medium free from this page. Download Online as well as Offline Apps for current session and CBSE Solutions updated on the basis of CBSE Curriculum. If someone is facing difficulty to access the contents of Tiwari Academy website or Tiwari Academy Apps, please contact us for help. We will help him as soon as possible and provide the suggestion at our level best.
10 Maths Chapter 3 Exercise 3.6 Solutions
NCERT Solutions for class 10 Maths Chapter 3 Exercise 3.6 in English Medium and Hindi medium given below updated for new academic year. In case you want to download these solutions in PDF, visit to NCERT Solutions for Class 10 Maths Chapter 3 main page to get all exercises.
| Class: 10 | Mathematics |
| Chapter 3: | Exercise 3.6 |
| Content: | NCERT Solution |
| Medium: | English and Hindi Medium |
Class 10 Maths Chapter 3 Exercise 3.6 Solution in Hindi Video
Important Questions – Answers for practice
- Sunita has some ₹ 50 and ₹ 100 notes amounting to a total of ₹15,500. If the total number of notes is 200, the find how many notes of ₹50 and ₹100 each, she has. [Answer: ₹50 notes = 90, ₹100 notes = 110]
- The electric bill of a certain establishment is partly fixed and partly varies as the number of units of electricity consumed. When in a certain month 200 units are consumed, the bill is ₹107. In another month, 300 units are consumed and the bill is ₹154. If yet another month, 500 units are consumed, find the bill for the month. [Answer: ₹248]
- Solve graphically the pair of linear equations 3x – 4y + 3 = 0 and 3x + 4y – 21 = 0. Find the co-ordinates of vertices of triangular region formed by these lines and x-axis. Also calculate the area of this triangle. [Answer: Solutions (3, 3). Vertices: (-1, 0), (7, 0) and (3, 3). Area = 12 square units]
Questions on Linear Equation from Board Papers
- A railway half ticket costs half the full fare and the reservation charges is same on half ticket as on full ticket. One reserved first class ticket from Mumbai to Ahmedabad costs ₹216 and one full and one half reserved first class ticket costs ₹327. What is the basic first class full fare and what is the reservation charge? [Answer: Fare = ₹210 reservation charge = ₹6]
- A man travels 600 km to his home partly by train and partly by bus. He takes 8 hours, if he travels 120 km by train and rest by bus. Further, it takes 20 minute longer, if he travels 200 km by train and rest by bus. Find the speeds of the train and the bus. [Answer: 60 km/h, 80 km/h]
Feedback and Suggestions
We always respond to the user’s suggestions. Your feedback is the strength of the Tiwari Academy Website. So, provide feedback and suggestions to improve the contents. Download CBSE NCERT Books for Class 10 and Offline Apps following new CBSE Syllabus. Ask your doubts related to NIOS or CBSE Board and share your knowledge with your friends and other users through Discussion Forum.
Important Questions 10th Maths Exercise 3.6
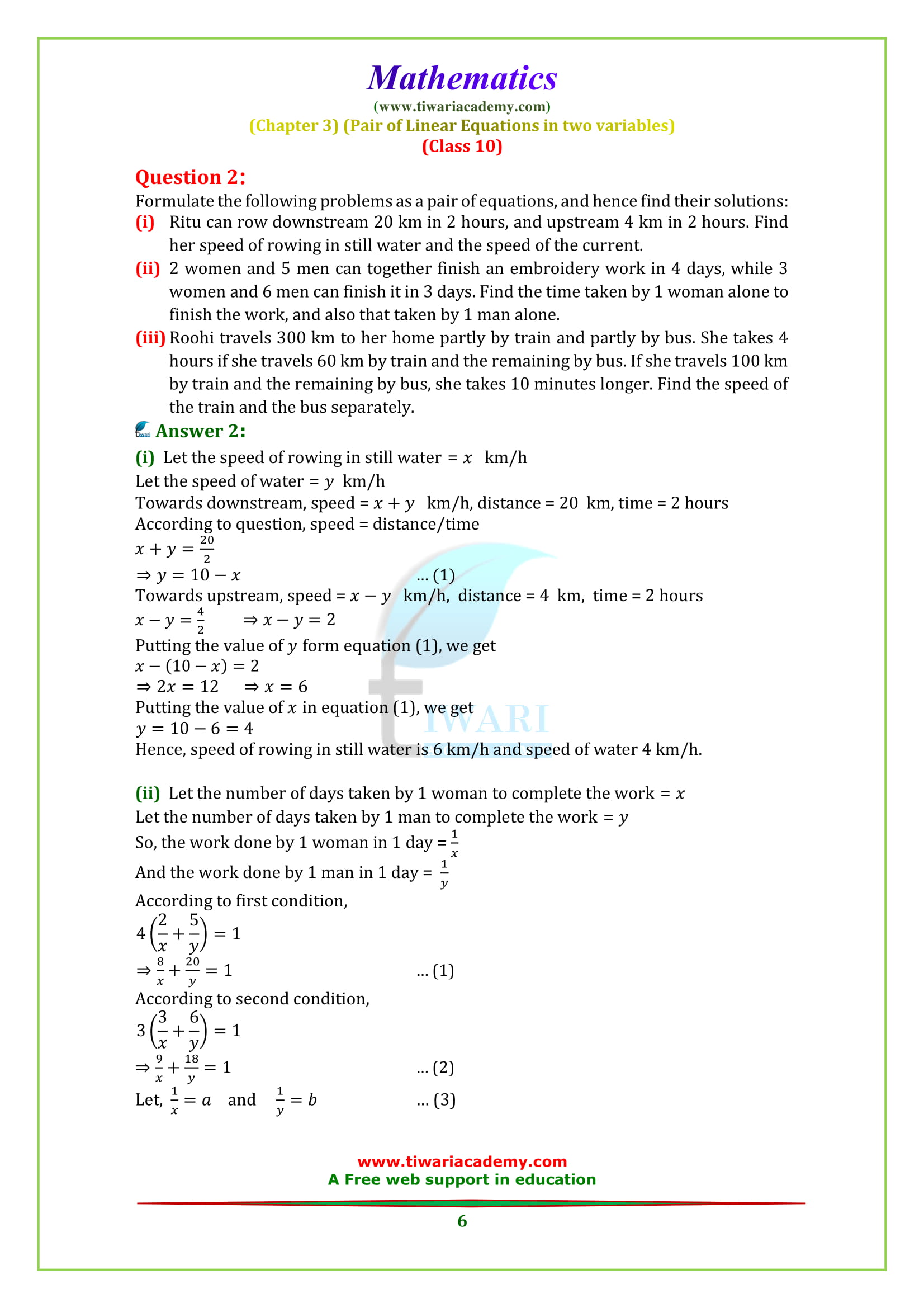
Ritu can row downstream 20 km in 2 hours, and upstream 4 km in 2 hours. Find her speed of rowing in still water and the speed of the current.
Let the speed of rowing in still water = x km/h
Let the speed of water = y km/h
Towards downstream, speed = x+y km/h, distance = 20 km, time = 2 hours
According to question, speed = distance/time
x + y = 20/2
⇒ y = 10 – x … (1)
Towards upstream, speed = x-y km/h, distance = 4 km, time = 2 hours
x – y = 4/2
⇒ x – y = 2
Putting the value of y form equation (1), we get
x – (10 – x) = 2
⇒ 2x = 12
⇒ x = 6
Putting the value of x in equation (1), we get
y= 10 – 6 = 4
Hence, speed of rowing in still water is 6 km/h and speed of water 4 km/h.
2 women and 5 men can together finish an embroidery work in 4 days, while 3 women and 6 men can finish it in 3 days. Find the time taken by 1 woman alone to finish the work, and also that taken by 1 man alone.
Let the number of days taken by 1 woman to complete the work = x
Let the number of days taken by 1 man to complete the work = y
So, the work done by 1 woman in 1 day = 1/x
And the work done by 1 man in 1 day = 1/y
According to first condition,
4(2/x + 5/y) = 1
⇒8/x + 20/y = 1 … (1)
According to second condition,
3(3/x + 6/y) = 1
⇒ 9/x + 18/y = 1 … (2)
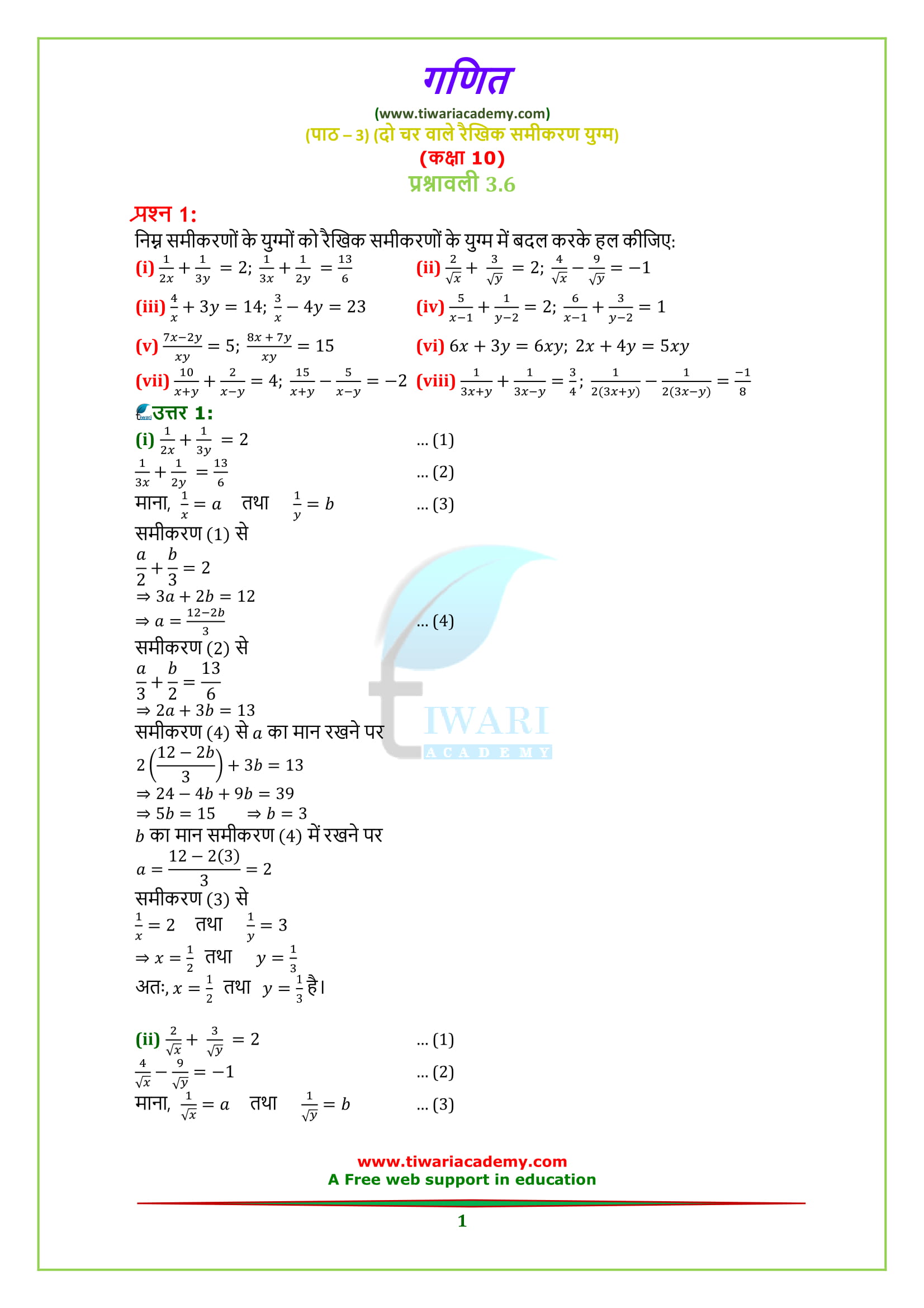
Let, 1/x = a and 1/y=b … (3)
From equation (1), we get
8a + 20b = 1
⇒ a = (1 – 20b)/8 … (4)
From equation (2), we get
9a + 18b = 1
Putting the value of a form equation (4), we get
9((1 – 20b)/8) + 18b = 1
⇒ 9 – 180b + 144b = 8
⇒ – 36b = – 1
⇒ b = 1/36
Putting the value of b in equation (4), we get
a = (1 – 20(1/36))/8 = 4/72 =1/18
From the equation (3), we get
1/x = 1/18 and 1/y = 1/36
⇒ x = 18 and y = 36
Hence, 1 woman takes 18 days and 1 man takes 36 days to complete the work.
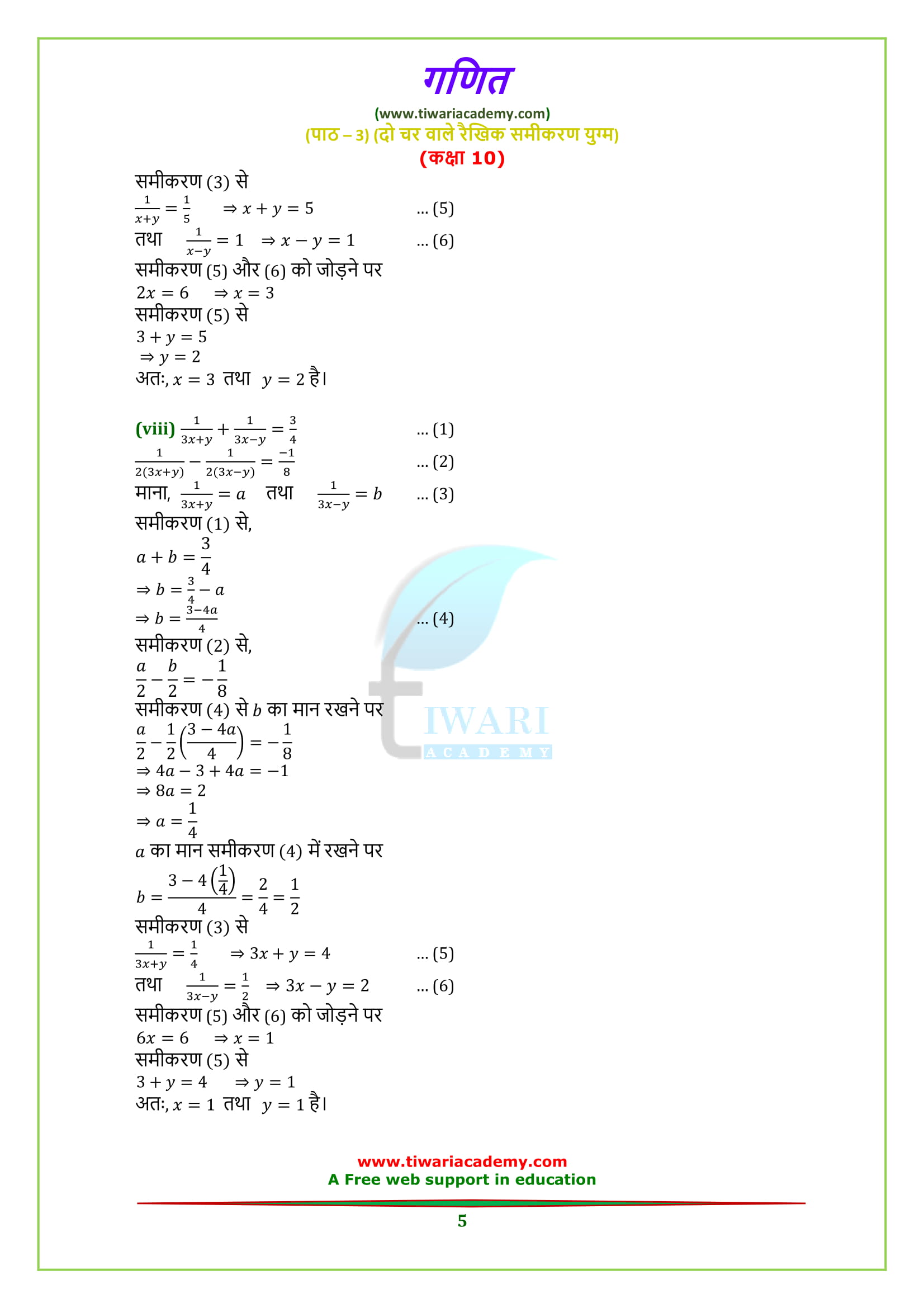
Roohi travels 300 km to her home partly by train and partly by bus. She takes 4 hours if she travels 60 km by train and the remaining by bus. If she travels 100 km by train and the remaining by bus, she takes 10 minutes longer. Find the speed of the train and the bus separately.
Let the speed of train = x km/h
Let the speed of bus = y km/h
If she travels 60 km by train and the remaining by bus, she take 4 hours, so, according to question,
60/x + 240/y = 4 … (1)
If she travels 100 km by train and the remaining by bus, she take 10 minutes more, so, according to question,
100/x + 200/y = 4+10/60
⇒ 100/x + 200/y = 25/6 … (2)
Let, 1/x = a and 1/y=b … (3)
From the equation (1), we get
60a + 240b = 4
⇒ a = (4 – 240b)/60 … (4)
From the equation (2), we get
100a + 200b = 25/6
Putting the value of a from equation (4), we get
100((4 – 240b)/60) + 200b = 25/6
⇒ 40 – 2400b + 1200b = 25
⇒ – 1200b = -15
⇒ b = 1/80
Putting the value of b in equation (4), we get
a = (4 – 240(1/80))/60 = 1/60
From the equation (3), we get
1/x = 1/60 and 1/y = 1/80
⇒ x = 60 and y = 80
Hence, the speed of train is 60 km/h and the speed of bus is 80 km/h.
How many questions are there in exercise 3.6 of class 10th mathematics?
There are in all 2 questions in exercise 3.6 of class 10th mathematics chapter 3 (Pair of linear equations in two variables). Q1 contains 8 parts and Q2 contains 3 word problems. Both Q1 and Q2 are important.
How many examples are based on exercise 3.6 of class 10th Maths?
Only 3 examples (examples 17, 18 and 19) are based on exercise 3.6 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics. Examples 18 and 19 are very important.
Which sums of exercise 3.6 chapter 3 class 10th Maths are difficult?
Students find example 19 and Q2 (all parts) of exercise 3.6 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics difficult.
Is exercise 3.6 of class 10th mathematics easy?
Exercise 3.6 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics is difficult exercise. But difficulty level of anything varies from student to student. So, Exercise 3.6 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics is easy or not depends on students also. Some students find it difficult some find it easy.
Which is the most important question in 10th Maths Exercise 3.6?
Most of the questions of Ex. 3.6 Class 10 Maths are important for Board Examination. So, practice all the questions properly to score good marks.
How can I get good marks in Exercise 3.6 of 10th Maths?
All the parts of exercise 3.6 question 1 need practice. Once by doing own, you need to practice once a week to be confident in exams. These questions are little bit difficult to solve but gradually with practice it become comfortable to solve and find answer.
