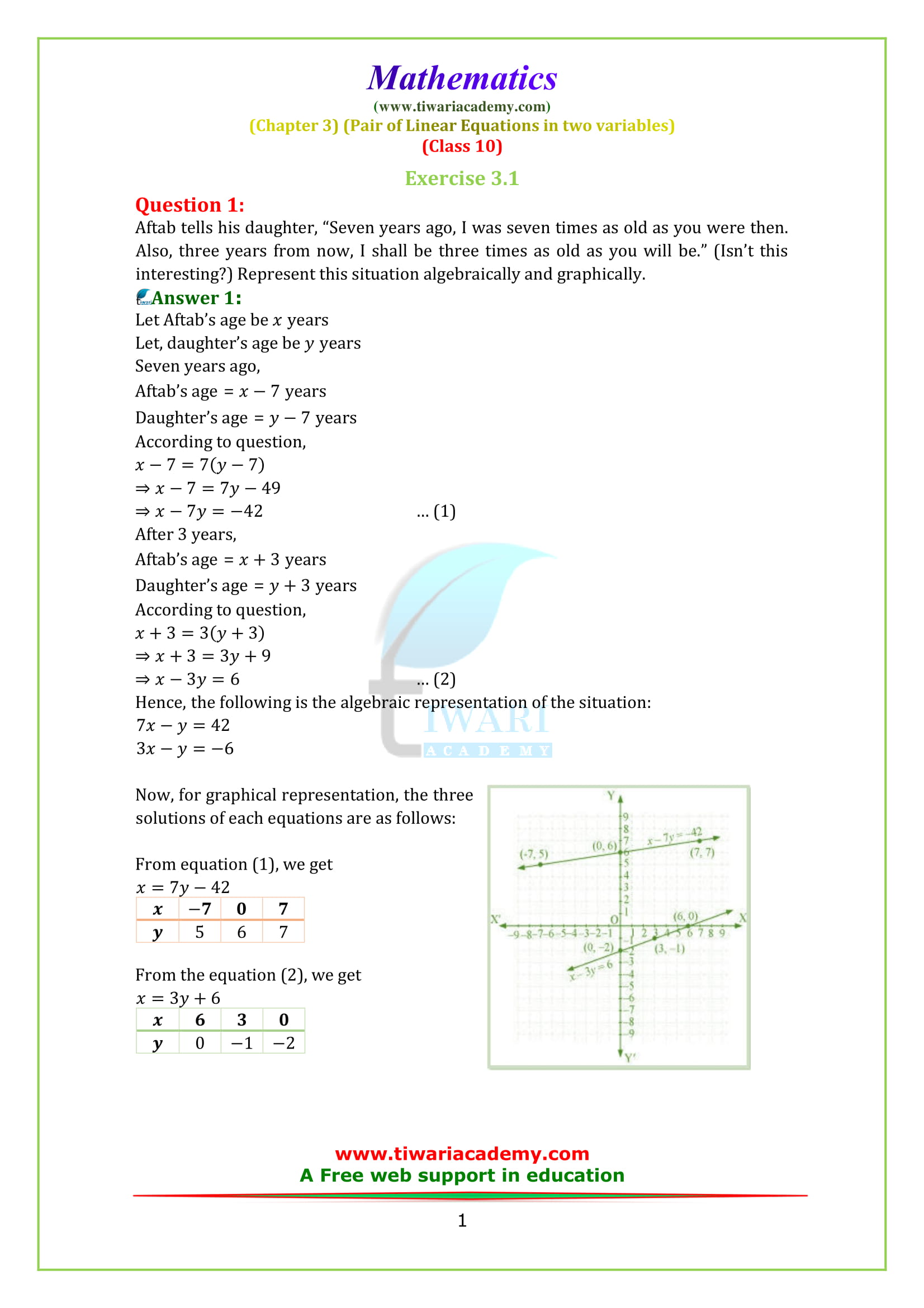
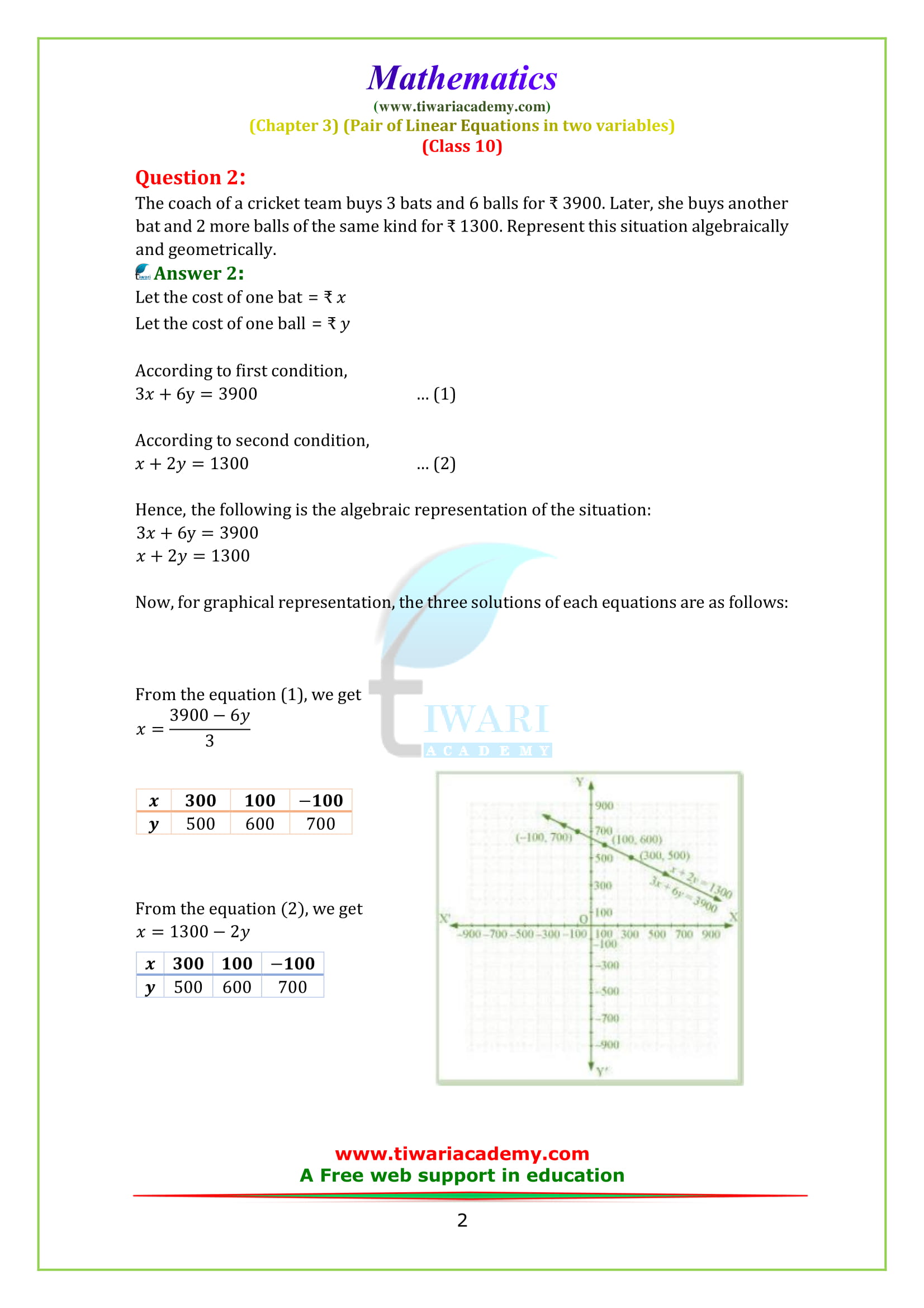
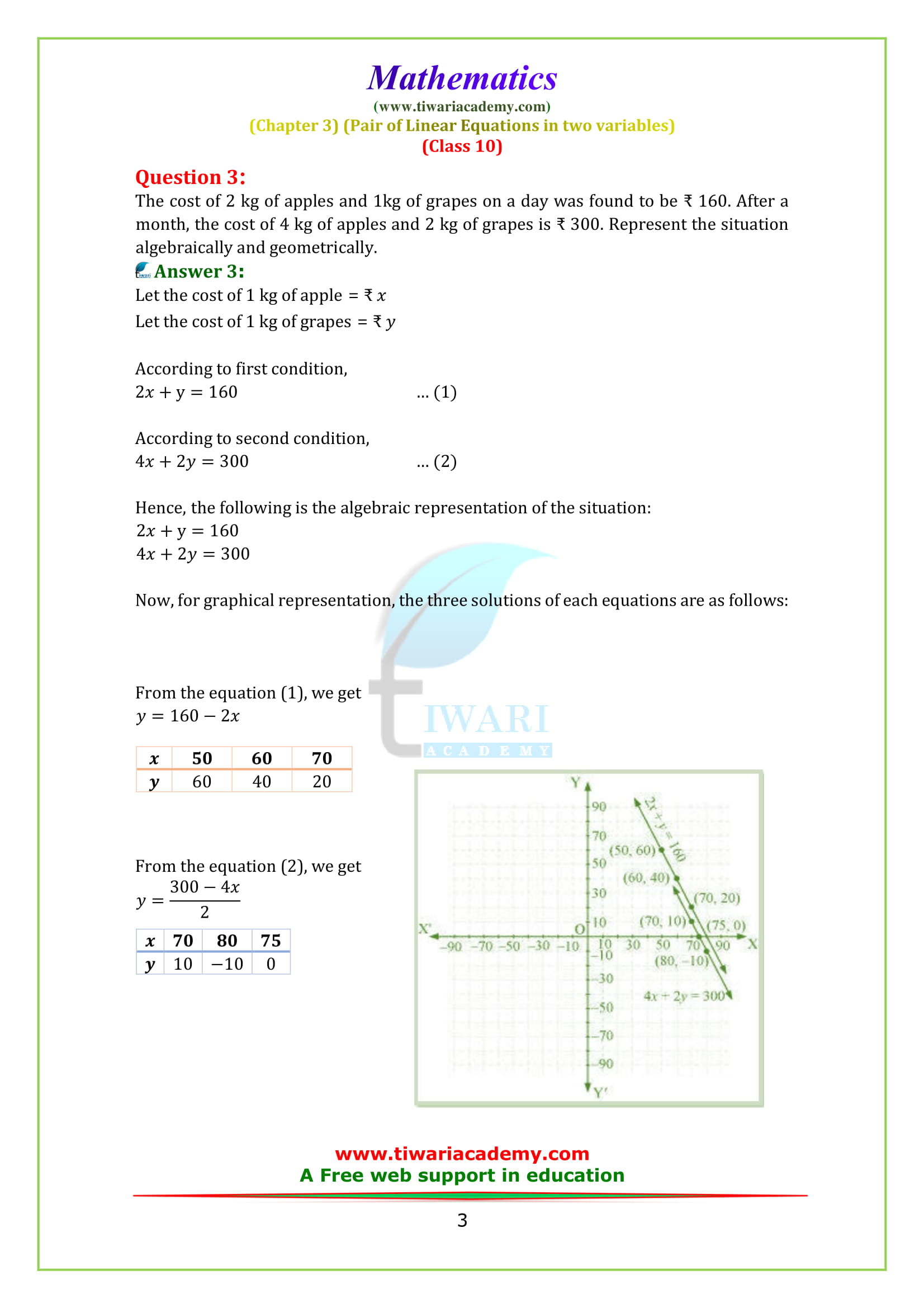
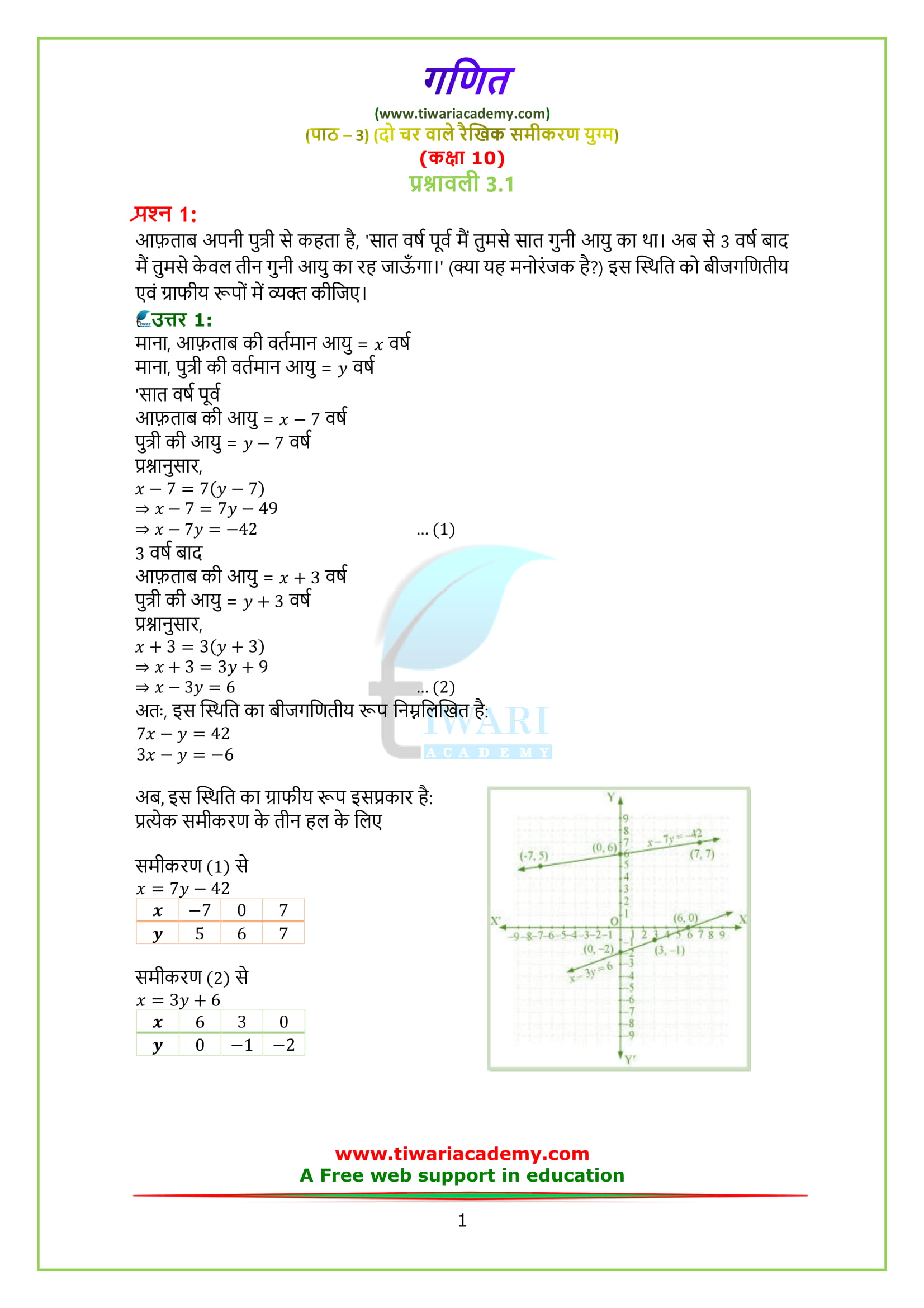
NCERT Solutions for class 10 Maths Chapter 3 Exercise 3.1 in Hindi and English medium prepared for all board. Excel in your Class 10 Maths exams with our specialized NCERT Solutions for Chapter 3 Exercise 3.1, available in both Hindi and English Medium. Designed to meet the needs of all boards including State Board, UP Board, and MP Board, our guide helps you master key concepts effortlessly. Boost your exam preparation now!
NCERT Solutions for class 10 Maths Chapter 3 Exercise 3.1
Class 10 Maths Ex. 3.1 solution pair of linear equations in two variables download in PDF file format or use it online. UP Board Students are also using NCERT Textbook for all boards. So, these solutions are helpful for their study also.
Download here the UP Board Solutions for Class 10 Maths Chapter 3 Exercise 3.1 in Hindi Medium. NCERT Solutions App for class 10 Maths are now updated for new academic session. NCERT Solutions Online and Offline Apps are free of cost.
In all the apps, solutions are modified as per latest CBSE Curriculum in both the medium (Hindi Medium and English Medium). These CBSE NCERT Solutions are also available in Video Format applicable for UP Board, Gujrat board as well as CBSE board NCERT Books. Download (Exercise 3.1) in PDF format.
| Class: 10 | Mathematics |
| Chapter 3: | Exercise 3.1 |
| Content: | NCERT Book Solutions |
| Medium: | English and Hindi Medium |
10 Maths Chapter 3 Exercise 3.1 Solutions
NCERT Solutions for class 10 Maths Chapter 3 Exercise 3.1 in English medium and Hindi Medium are given below updated for new academic session. Download options are given on the page or on main page of NCERT Solutions Class 10 Maths Chapter 3. Visit to Discussion Forum to ask your doubts and answers to the questions asked by others.
Class 10 Maths Chapter 3 Exercise 3.1 Solution in Hindi Medium Video
Important Terms for Pair of Linear Equations
The graph of a pair of linear equations in two variables is represented by two lines.
- If the lines intersect at a point, the pair of equations is consistent. The point of intersection gives the unique solution of the equations.
- If the lines are parallel, then there is no solution the pair of linear equations is inconsistent.
- If the lines coincide, then there are infinitely many solutions. The pair of linear equations is consistent. Each point on the line is a solution of both the equations.
Important Questions on Linear Equations for Practice
- If x = 3m –1 and y = 4 is a solution of the equation x + y = 6, then find the value of m. [Answer: m=1]
- What is the point of intersection of the line represented by 3x – 2y = 6 and the y-axis. [Answer: (0, -3)]
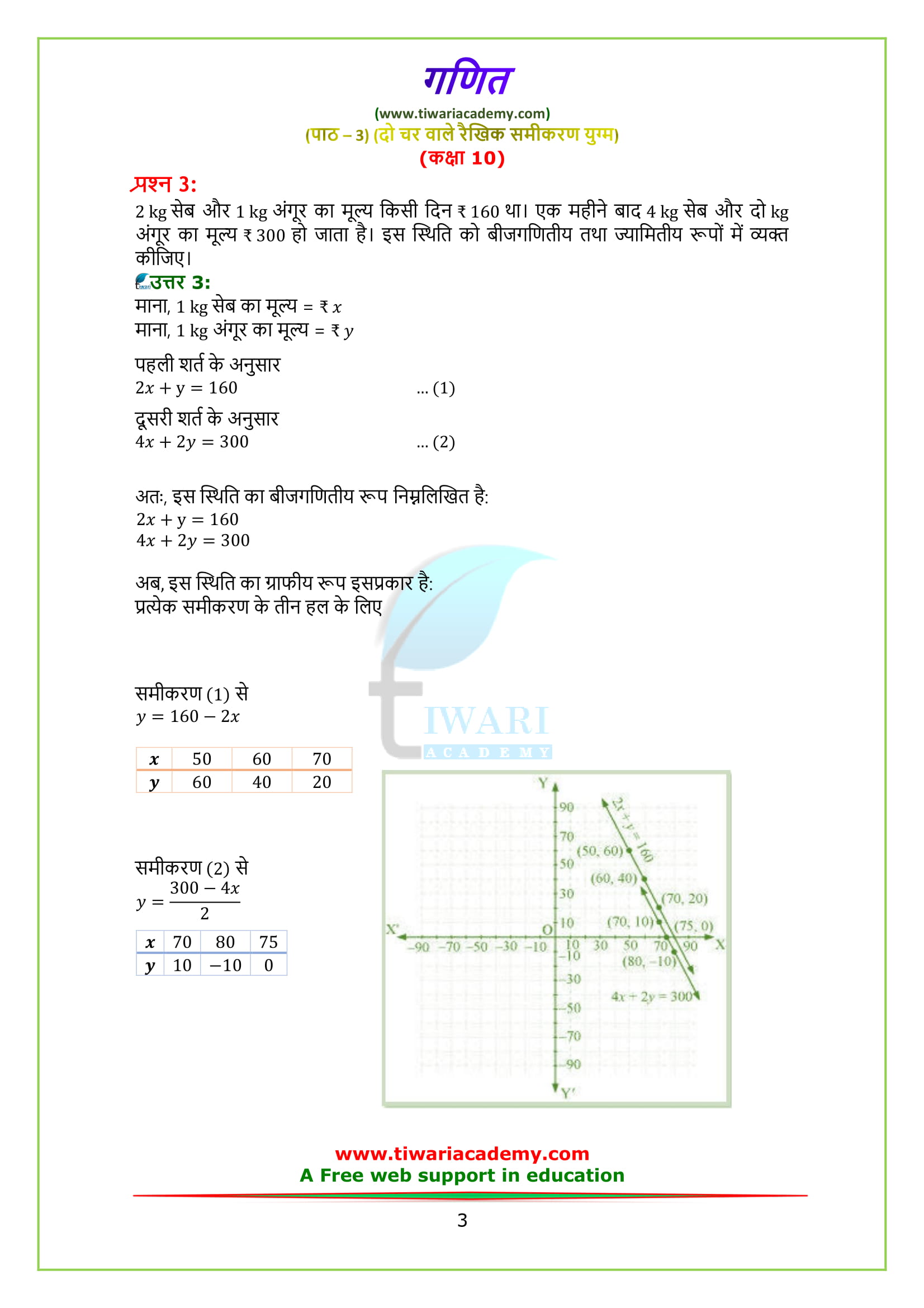
- In a deer park, the number of heads and number of legs of deer and human visitors were counted and it was found that there were 39 heads and 132 legs. Find the number of deer and human visitors in the park. [Answer: Dear: 27, Visitors: 12]
- For what value of p, system of equations 2x + py = 8 and x + y = 6 have no solution. [Answer: p=2]
- From Delhi station if we buy 2 tickets to station A and 3 tickets to station B, the total cost is ₹77, but if we buy 3 tickets to station A and 5 tickets to station B, the total cost is ₹124. What are fares from Delhi to station A and to station B? [Answer: ₹13, ₹17]
- A motor cyclist is moving along the line x – y = 2 and another motor cyclist is moving along the line x – y = 4 find out their moving direction. [Answer: move parallel]
- A farmer sold a calf and a cow for ₹760, thereby, making a profit of 25% on the calf and 10% on the cow. By selling them for ₹767.50, he would have realised a profit of 10% on the calf and 25% on the cow. Find the cost of each. [Answer: Cost of cow = ₹350, cost of calf = ₹300]
Important Questions 10th Maths Exercise 3.1
What do you mean by the solution of a linear Equation?
Every solution of the equation is a point on the line representing it.
How many solutions do a linear equation have?
There are infinite number of points on a line. So, a linear equations have infinite number of solutions.
How many solutions do a system of linear equations have?
A system of linear equations may have o solution or one solutions or infinite many solutions. For a parallel lines, there is no solution at all. for an intersecting lines, only one solutions is there but for coincident lines, infinitely many solutions are possible.
How do we check graphically that the system of linear equations have a unique solutions?
If all the lines are intersecting at one point, then we can say that the system of linear equations are consistent and have a unique solution.
Download NCERT Books and Offline Apps based on new CBSE Syllabus. Ask your doubts related to NIOS and CBSE and share your knowledge with your friends and other users through Discussion Forum.
How many questions are there in exercise 3.1 of 10th Maths?
There are only 3 questions in exercise 3.1 of class 10th mathematics chapter 3 (Pair of linear equations in two variables) and Q1 of this exercise is important. All 3 questions are of word problem.
How many examples are based on exercise 3.1 of class 10th mathematics?
Only 3 examples are based on exercise 3.1 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics and examples 1 and 2 are important. All three examples are of different type.
What students will learn in exercise 3.1 of class 10th Maths?
In exercise 3.1 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics students will learn how to represent the given situation algebraically and graphically (geometrically).
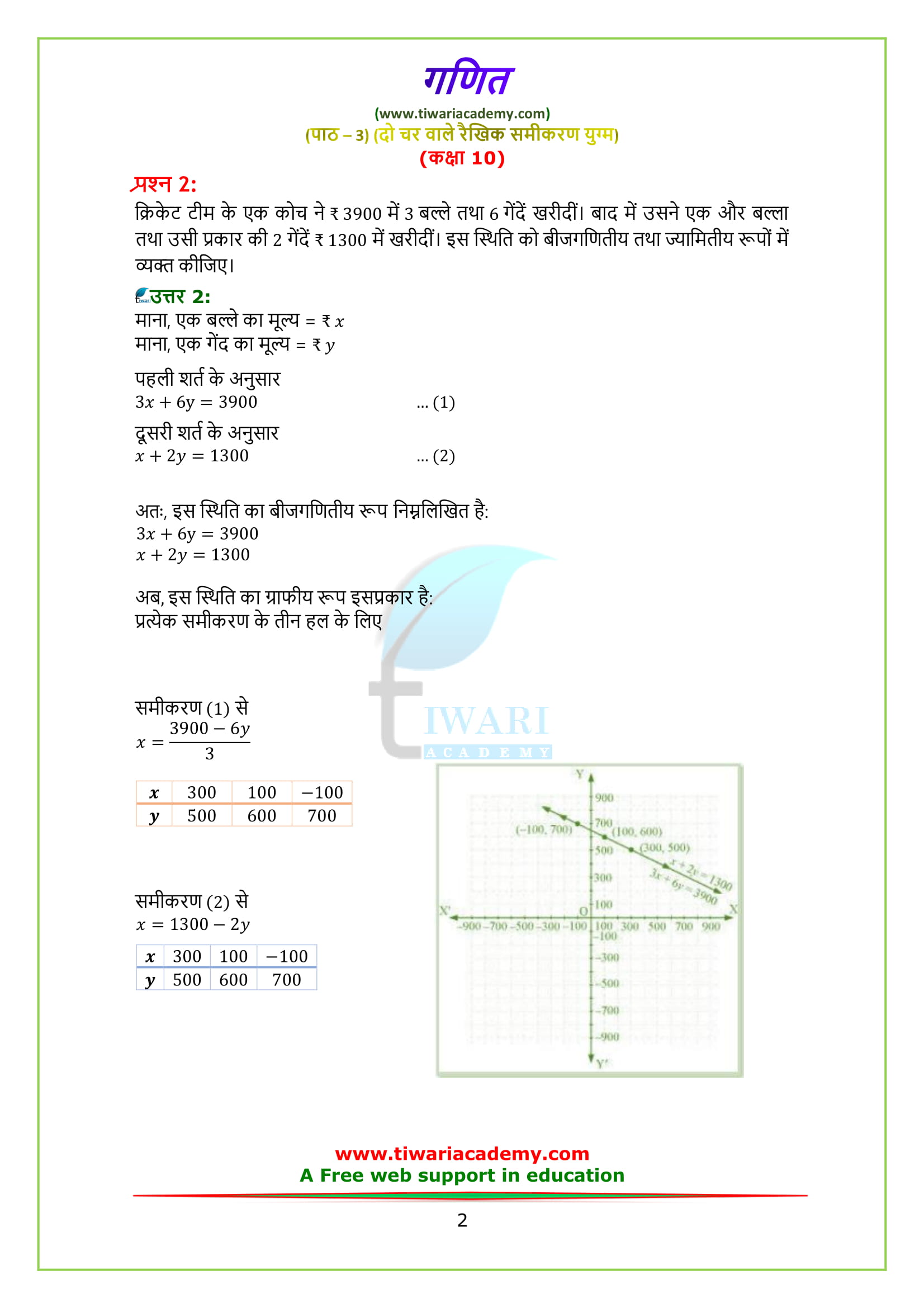
Example of a situation: The coach of a cricket team buys 3 bats and 6 balls for Rs 3900. Later, she buys another bat and 3 more balls of the same kind for Rs 1300. Represent this situation algebraically and graphically (geometrically).
Is exercise 3.1 of class 10th mathematics difficult?
Exercise 3.1 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics is easy exercise. But difficulty level of anything varies from student to student. So, Exercise 3.1 (chapter 3 Pair of linear equations in two variables) of class 10th mathematics is easy or not depends on students also. Some students find it difficult some find it easy.