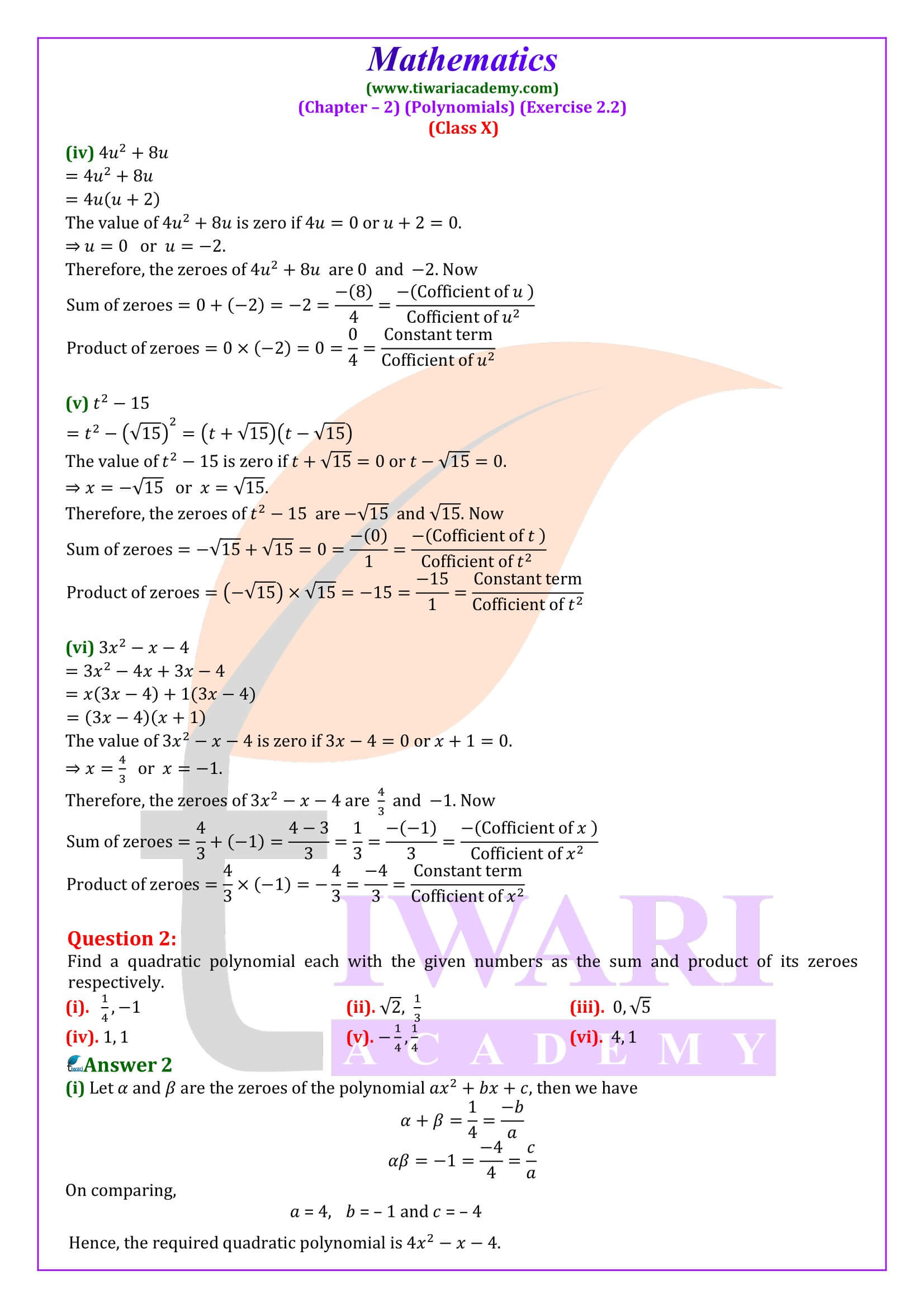
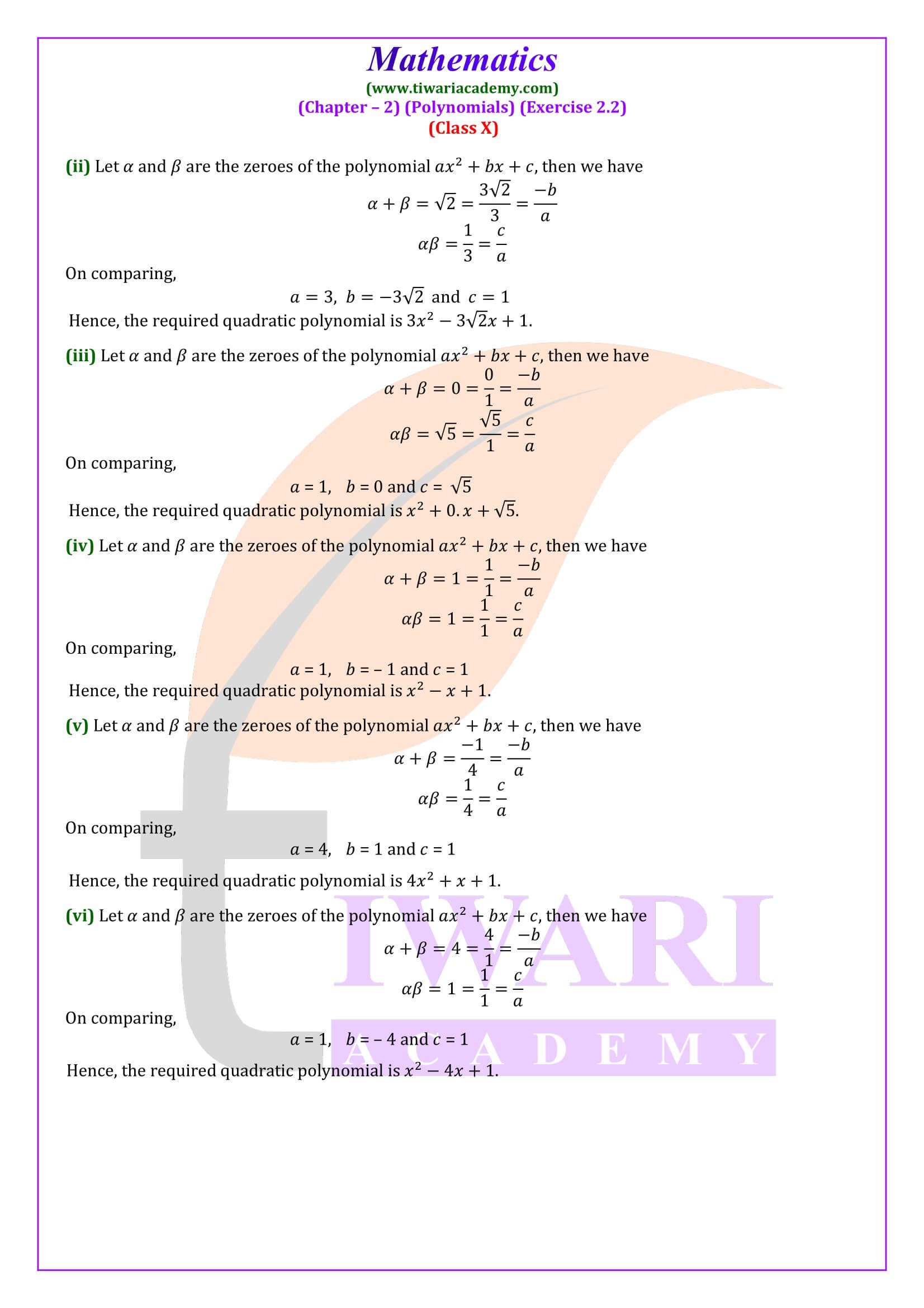
NCERT Solutions for Class 10 Maths Chapter 2 Exercise 2.2 Polynomials Updated for New Session 2025-26 in Hindi Medium and English Medium. Get the revised solutions of ex. 2.2 NCERT Class 10 mathematics based on new syllabus and new textbooks published for academic year 2025-26.
NCERT Exercise 2.2 Solutions for Class 10 Maths
Class 10 Maths Chapter 2 Exercise 2.2 Board Questions Solutions
1. (-2/5) is one of the zeroes of the polynomial 5x² + 2x – 7. (T/F) Justify your answer.
See SolutionFalse
Explanation:
Given polynomial:
f(x) = 5x² + 2x − 7
We are told that one of the zeroes is −2/5.
Let’s check if f(−2/5) = 0.
Substitute x = −2/5 into f(x):
f(−2/5) = 5(−2/5)² + 2(−2/5) − 7
= 5 × (4/25) − 4/5 − 7
= (20/25) − (4/5) − 7
= (4/5) − (4/5) − 7
= 0 − 7
−7 ≠ 0
2. f(x) = x² + 10x + 21. Find the zeroes of the above polynomial.
See Solutionx = -3 and x = -7
Explanation: To find the zeros, I’ll set f(x) = 0: x² + 10x + 21 = 0
Using the quadratic formula or factoring:
x² + 10x + 21 = 0
(x + 3)(x + 7) = 0
Therefore, x = -3 or x = -7 are the zeros of the polynomial.
3. Let p and q be zeroes of the polynomial 3x² + 4x – 4. Without finding the actual values of p and q, evaluate (1 – p)(1 – q).
See Solution(1 − p)(1 − q) = 1
Explanation: Given polynomial:
f(x) = 3x² + 4x − 4
Let the zeroes be: p and q
We are asked to find: (1 − p)(1 − q)
Step 1: Use identity:
(1 − p)(1 − q) = 1 − (p + q) + pq
We’ll use the formulas for sum and product of zeroes:
For a quadratic polynomial ax² + bx + c:
– Sum of zeroes (p + q) = −b/a = −4/3
– Product of zeroes (pq) = c/a = −4/3
Step 2: Plug into identity
(1 − p)(1 − q)
= 1 − (p + q) + pq
= 1 − (−4/3) + (−4/3)
= 1 + 4/3 − 4/3
= 1
4. The graph of a polynomial passes through (6, 0), (0, -2) and (-1, 0). Write two factors of the polynomial. Justify your answer.
See SolutionThe factors are (x – 6) and (x + 1)
Explanation: Given the polynomial passes through (6, 0), (0, -2) and (-1, 0).
Therefore, the polynomial intersects the X-axis at x = 6 and x = -1
Hence, the two factors of the polynomial passing through (6, 0), (0, -2) and (-1, 0) are (x – 6) and (x + 1).
5. p(x) is a polynomial given by ax² – 4x + 3, where a is a non-zero real number. One of the zeroes of p(x) is 3 times the other zero.
(i) Find the value of a. Show your work.
(ii) Based on the value of a, what would be the shape of the graph of p(x)? Give a reason for your answer.
See Solution(i) Value of a = 1
(ii) The graph of p(x) is a parabola opening upwards, because the leading coefficient a = 1 > 0.
Explanation: Given polynomial:
p(x) = ax² − 4x + 3, where a ≠ 0
One zero is 3 times the other zero.
Let the zeroes be:
Let the first zero = α
Then the second zero = 3α
Step 1: Use the relationship between zeroes and coefficients
For a quadratic polynomial ax² + bx + c:
– Sum of zeroes = −b / a
– Product of zeroes = c / a
Using:
– α + 3α = 4α = −(−4)/a = 4/a
⇒ 4α = 4/a
⇒ α = 1/a
Now use product of zeroes:
– α × 3α = 3α² = 3(1/a)² = 3/a²
Also, Product = c / a = 3 / a
So, 3/a² = 3/a
Multiply both sides by a²: 3 = 3a
⇒ a = 1
Step 2: Find the shape of the graph
Now that a = 1, the polynomial becomes: p(x) = x² − 4x + 3
Since:
– The coefficient of x² (a = 1) is positive,
– The graph is a parabola that opens upwards
6. Let p(x) = (x + 5)² – 7(x – k), where k is a constant. If p(x) is divisible by x, then find the value of k.
See Solutionk = 25/7
Explanation: If p(x) is divisible by x, then p(0) = 0.
p(0) = (0 + 5)² – 7(0 – k) = 0
25 + 7k = 0
7k = -25
k = -25/7
Actually, let us double-check this:
p(0) = (0 + 5)² – 7(0 – k)
= 25 – 7(-k)
= 25 + 7k
Setting this equal to 0:
25 + 7k = 0
7k = -25
k = -25/7
But we need to be careful with the signs. Let’s verify:
p(0) = (0 + 5)² – 7(0 – k)
= 25 – 7(-k)
= 25 + 7k
Setting equal to 0:
25 + 7k = 0
7k = -25
k = -25/7 = -3.57…
Actually, we think we made an error. Let me recalculate:
p(0) = (0 + 5)² – 7(0 – k) = 25 – 7(-k) = 25 + 7k
For p(0) = 0: 25 + 7k = 0
7k = -25
k = -25/7
7. Anand multiplied a variable with 6, subtracted 27 and added the square of the original variable. He expressed the final expression as a product of 2 factors. His friend, Amit said that the factors will always have a difference of 6. Is Amit right?
See SolutionNo, Amit is not right. The factors of the expression have a difference of 12, not 6.
Explanation: Let’s follow Anand’s steps one by one:
Let the variable be x.
Step 1: Multiply by 6 → 6x
Step 2: Subtract 27 → 6x − 27
Step 3: Add square of the original variable → x² + (6x − 27)
Final expression: x² + 6x − 27
Now, factor the expression:
We factor: x² + 6x − 27
We need two numbers whose:
– Product = −27
– Sum = 6
Try: 9 and −3
9 × (−3) = −27
9 + (−3) = 6
So, x² + 6x − 27 = (x + 9)(x − 3)
Check Amit’s claim: The two factors are:
(x + 9) and (x − 3)
Difference = (x + 9) − (x − 3)
= x + 9 − x + 3
= 12
So the difference between the two factors is 12, not 6.
8. State whether the following statement is true or false. Justify your answer. If the graph of a polynomial has exactly 2 zeroes, then the polynomial must be quadratic.
See SolutionFalse
Explanation: A polynomial of degree n can have at most n distinct real zeroes. A quadratic polynomial can have at most 2 distinct real zeroes.
However, higher-degree polynomials can also have exactly 2 zeroes. For example, the polynomial P(x) = x³ – x = x(x-1)(x+1) has zeroes at x = -1, 0, and 1, but if we consider P(x) = x²(x-1)(x+1), it has exactly 2 distinct zeroes (at x = -1 and x = 1) with a repeated zero at x = 0.
Therefore, having exactly 2 zeroes does not necessarily mean the polynomial is quadratic.
9. Aasira multiplied a variable with 4, subtracted 12 and added the square of the original variable. She expressed the final expression as a product of 2 factors. Her friend, Rishi, said that the factors will always have a difference of 8. Is Rishi right? Show your work.
See SolutionYes, Rishi is right.
Explanation: Let the original variable be x. The final expression is 4x – 12 + x²
Factoring: x² + 4x – 12 = (x + 6)(x – 2)
The difference between the factors is 6 – (-2) = 8.
Therefore, Rishi is correct – the factors have a difference of 8.
For Class 10 Maths NCERT Exercise 2.2, focus on mastering the algebraic relationships between the zeroes of polynomials and their coefficients. NCERT 10th Math exercise emphasizes finding zeroes of quadratic polynomials and verifying their relationships with coefficients. Begin by revisiting the factorization techniques you learned in earlier classes, especially the method of splitting the middle term. Solve a variety of problems where you calculate zeroes and then confirm their sum and product match the coefficients. This will help you get comfortable with applying these concepts during exams.
Practice solving 10th Math exercise problems without looking at the solution steps to simulate exam conditions. Make sure to work on quadratic polynomials with different forms to cover all possibilities. Reviewing the derivation of relationships between zeroes and coefficients can give you deeper insights, which is helpful for answering conceptual questions that may accompany calculation-based ones in the exam.
Class 10 Maths Exercise 2.2 Solutions in English and Hindi Medium
| Class: 10 | Mathematics |
| Chapter 2: | Exercise 2.2 |
| Content: | Solutions and Study Material |
| Mode of Content: | Images, PDF, Videos and Text |
| Academic Session: | Year 2025-26 |
| Medium: | English and Hindi Medium |
NCERT Exercise 2.2 Solutions for Class 10 Maths Chapter 2
Class 10 Ex. 2.2 solution Bahupad in Hindi Medium and English Medium free to download in PDF or study online without downloading, updated for new academic session 2025-26. Solutions are applicable for all the boards which are following the Textbooks of NCERT or equivalent books. For example, Uttar Pradesh Board, Prayagraj is now implemented NCERT Books for High School and Intermediate students. So, UP Board High School students can download UP Board Solutions for Class 10 Maths Chapter 2 Exercise 2.2 from this page. Videos related to 10th Maths Ex. 2.2 are also given below just after the PDF solutions. You can view in Video Format solutions both in online format or in offline format (PDF). Download CBSE NCERT Solutions Apps 2025-26 based on updated NCERT Solutions for the new session. Feel free to contact us for help. We are here to help the students in Education.
Important Questions of 10th Maths Exercise 2.2
What do you understand by value of p(x) at k?
If p(x) is a polynomial in x, and if k is any real number, then the value obtained by replacing x by k in p(x), is called the value of p(x) at x = k, and is denoted by p(k).
What is zero of a polynomial?
A real number k is said to be a zero of a polynomial p(x), if p(k) = 0.
How do we find the zeros of a quadratic polynomial graphically?
The zeroes of a quadratic polynomial ax² + bx + c, a ≠ 0, are precisely the x-coordinates of the points where the parabola representing y = ax² + bx + c intersects the x-axis.
How many zeros are there of a quadratic polynomial?
A polynomial of degree 2 has at most two zeroes.
10 Maths Chapter 2 Exercise 2.2 Solutions
Updated and simplified form of solutions fit for academic years 2025-26 as per CBSE Curriculum for CBSE board, Gujrat Board, MP Board and UP Board (High School) students using NCERT Books. NCERT Solutions for class 10 Maths Chapter 2 Exercise 2.2 Polynomials in English and Hindi Medium are given below. If you find any difficulty to understand these solutions, please specify us without any hesitation.
Important Questions with Answers on Polynomials
- If one zero of the polynomial (a² + 9)x² + 13x + 6a is the reciprocal of the other, find the value of a. [Answer: a = 3]
- How many (i) maximum (ii) minimum number of zeroes can a quadratic polynomial have? [Answer: (i) 2, (ii) 0]
- What will be the number of real zeroes of the polynomial x² + 1? [Answer: 0]
- If α and β are zeroes of polynomial 6x² – 7x – 3, then form a quadratic polynomial where zeroes are 2α and 2β. [Answer: 3x² – 7x – 6]
- If α and 1/α are zeroes of 4x² – 17x + k – 4, find value of k. [Answer: k = 8]
- What will be the number of zeroes of the polynomials whose graphs are parallel to (i) y-axis (ii) x-axis. [Answer: (i) 1, (ii) 0]
- Divide 2x² + x – 20 by x + 3 and verify division algorithm.
- What will be number of zeroes of the polynomials whose graphs are either touching or intersecting the axis only at the points: (i) (–3, 0), (0, 2) & (3, 0) (ii) (0, 4), (0, 0) and (0, –4). [Answer: (i) 2, (ii) 1]
- If –3 is one of the zeroes of the polynomial (k– 1)x² + k x + 1, find the value of k. [Answer: 4/3]
- If α and β are the zeroes of the polynomial p(x) = x² + x + 1, find the value of α² + β². [Answer: -1]
Questions from Board Papers
1. If the product of zeroes of ax² – 6x – 6 is 4, find the value of a. Hence find the sum of its zeroes. [Answer: a = -3/2, sum of zeroes = – 4]
2. If α and β are zeroes of the polynomial x² – a(x + 1) – b such that (α + 1) (β + 1) = 0, find the value of b. [Answer: 1]
3. It is given that 1 is one of the zeroes of the polynomial 7x – x³ – 6. Find its other zeroes. [Answer: -3, 2]
4. If zeroes of x² – kx + 6 are in the ratio 3 : 2, find k. [Answer: -5, 5]
5. If one zero of the quadratic polynomial (k² + k)x² + 68x + 6k is reciprocal of the other, find k. [Answer: 5]
What are the main topics in exercise 2.2 class 10th Maths to learn?
In exercise 2.2 (chapter 2 Polynomials) of class 10th mathematics students will learn the following things:
- How to find zeroes of quadratic polynomial.
- Relationship between zeroes and coefficients of quadratic polynomial.
- Relationship between zeroes and coefficients of cubic polynomial.
- How to find a quadratic polynomial, the sum and product of whose zeroes are given.
How many questions are there in exercise 2.2 of class 10th Mathematics?
There are in all 2 questions (each question have 6 parts) in exercise 2.2 of class 10th mathematics chapter 2 (Polynomials) and both the questions are important.
How many examples are there in exercise 2.2 of 10th Maths?
4 examples (example 2, 3, 4, 5) are based on exercise 2.2 (chapter 2 Polynomials) of class 10th mathematics. All examples are of different type and example 2, 3, 4 are important. If we compare examples with questions of exercise 2.2 then we can observe that example 2, 3 and question 1 are of same type. Example 4 and question 2 are of same type.
Is exercise 2.2 of 10th Maths easy?
Exercise 2.2 (chapter 2 Polynomials) of class 10th mathematics is not that easy and not that difficult it somewhere in middle of easy and difficult. But difficulty level of anything varies from student to student. So, Exercise 2.2 (chapter 2 Polynomials) of class 10th mathematics is easy or not depends on students also. Some students find it difficult some find it easy or some find it in middle of easy and difficult.