To find the volume of air contained in Rachel’s model, which is a cylinder with two cones attached at its ends, we need to calculate the volume of the entire model and then subtract the volume of the aluminium sheet.
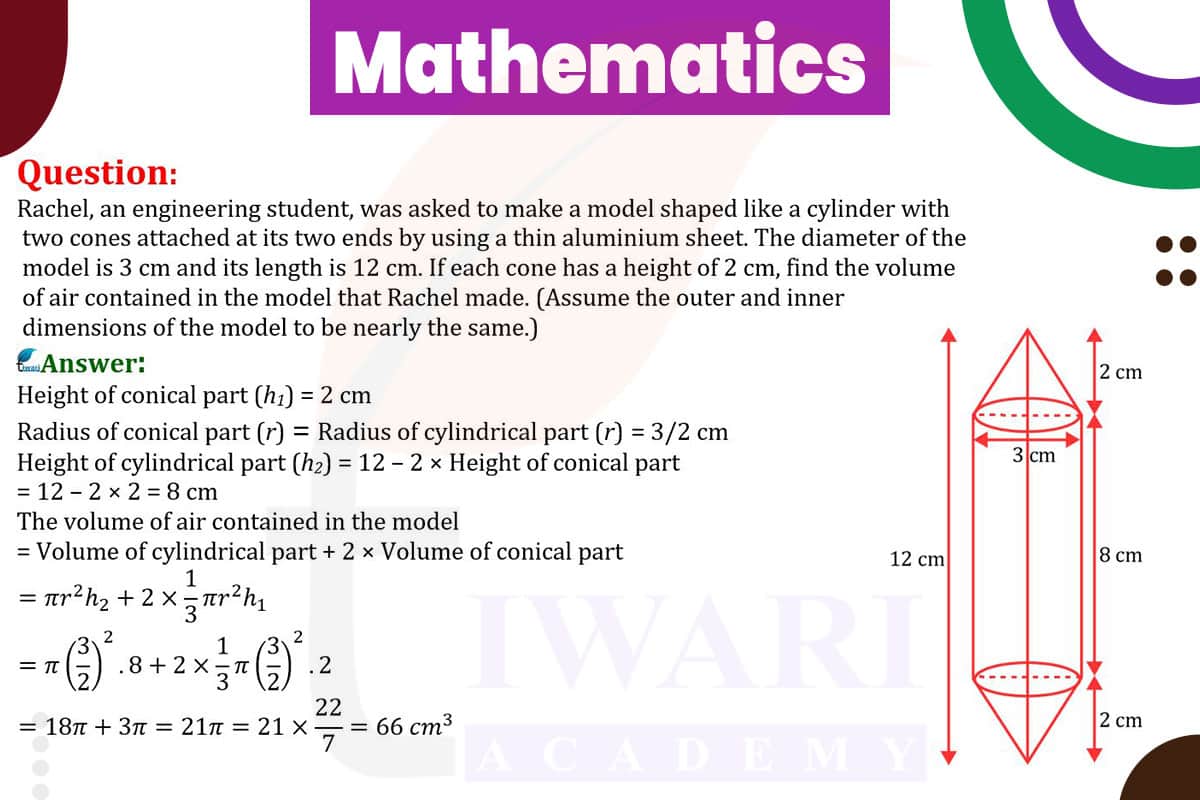
The diameter of the model is 3 cm, giving a radius of 1.5 cm. The total length of the model is 12 cm. Since each cone has a height of 2 cm, the length of the cylindrical part is 12cm − 2×2 cm = 8cm.
The volume of the cylinder is h = πr²h = π × 1.5² × 8. The volume of each cone is (1/3)πr²h = (1/3)π×1.5²×2. Since there are two cones, we multiply this by 2.
The total volume of the model is the sum of the volumes of the cylinder and the two cones. However, since the aluminium sheet is thin, its volume is negligible compared to the volume of air. Therefore, the volume of air contained in the model is approximately equal to the total volume of the model.
Let’s discuss in detail
Introduction to Geometric Modeling in Engineering
Rachel, an engineering student, faces a common challenge in the field of engineering: creating a model that combines multiple geometric shapes. Her task is to construct a model using a thin aluminium sheet, shaped like a cylinder with two cones attached at its ends. This type of model is not just an academic exercise; it’s a practical application of geometry in engineering and design. The model’s dimensions are given: a diameter of 3 cm and a total length of 12 cm, with each cone having a height of 2 cm. The objective is to calculate the volume of air contained within this composite structure.
Understanding the Dimensions of the Model
The first step in calculating the volume of the model is to understand its dimensions. The model consists of a central cylindrical part with two conical ends. The diameter of the entire model, including the cylinder and cones, is 3 cm, giving a radius of 1.5 cm (half of the diameter). The total length of the model is 12 cm. These dimensions are crucial for calculating the volume of each component of the model.
Calculating the Volume of the Cylindrical Part
The cylindrical part of the model forms the central section. To calculate its volume, we use the formula for the volume of a cylinder, πr²h, where r is the radius and h is the height. In this case, the height of the cylinder is the total length of the model minus the heights of the two cones. Since each cone is 2 cm high, the height of the cylinder is 12 cm − 2×2 cm = 8cm. Thus, the volume of the cylinder is π × 1.5² × 8 cm³.
Determining the Volume of the Conical Parts
Next, we calculate the volume of the conical parts. The formula for the volume of a cone is (1/3)πr²h. Each cone in the model has a radius of 1.5 cm and a height of 2 cm. Therefore, the volume of each cone is (1/3) π × 1.5² × 2 cm³. Since there are two identical cones, we multiply this volume by 2 to get the total volume of the conical parts.
Total Volume of the Model and the Contained Air
To find the total volume of the model, and hence the volume of air it contains, we add the volumes of the cylindrical and conical parts. This total volume represents the space within the model that is not occupied by the aluminium sheet. Given that the aluminium sheet is thin, its volume is negligible compared to the overall volume of the model. Therefore, the volume of air contained in the model is approximately equal to the total volume of the cylindrical and conical parts combined.
Practical Application of Geometric Principles
In conclusion, calculating the volume of air contained in Rachel’s cylindrical and conical model demonstrates the practical application of geometric principles in engineering and design. This exercise not only reinforces the understanding of how to calculate the volumes of composite shapes but also highlights the importance of these calculations in real-world applications. It showcases how theoretical geometric concepts are applied in practical scenarios, emphasizing the role of mathematics in engineering design and problem-solving. This problem-solving approach is not just about numbers and formulas; it’s about understanding the spatial properties of objects and their applications in the real world.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail

