A vessel is in the form of an inverted cone. Its height is 8 cm

Updated by Tiwari Academy
on November 28, 2023, 9:56 AM

To find the number of lead shots dropped into the vessel, we first calculate the volume of water that flows out, which is one-fourth of the vessel’s total volume. Then, we determine the volume of one lead shot and use this to find the number of shots.
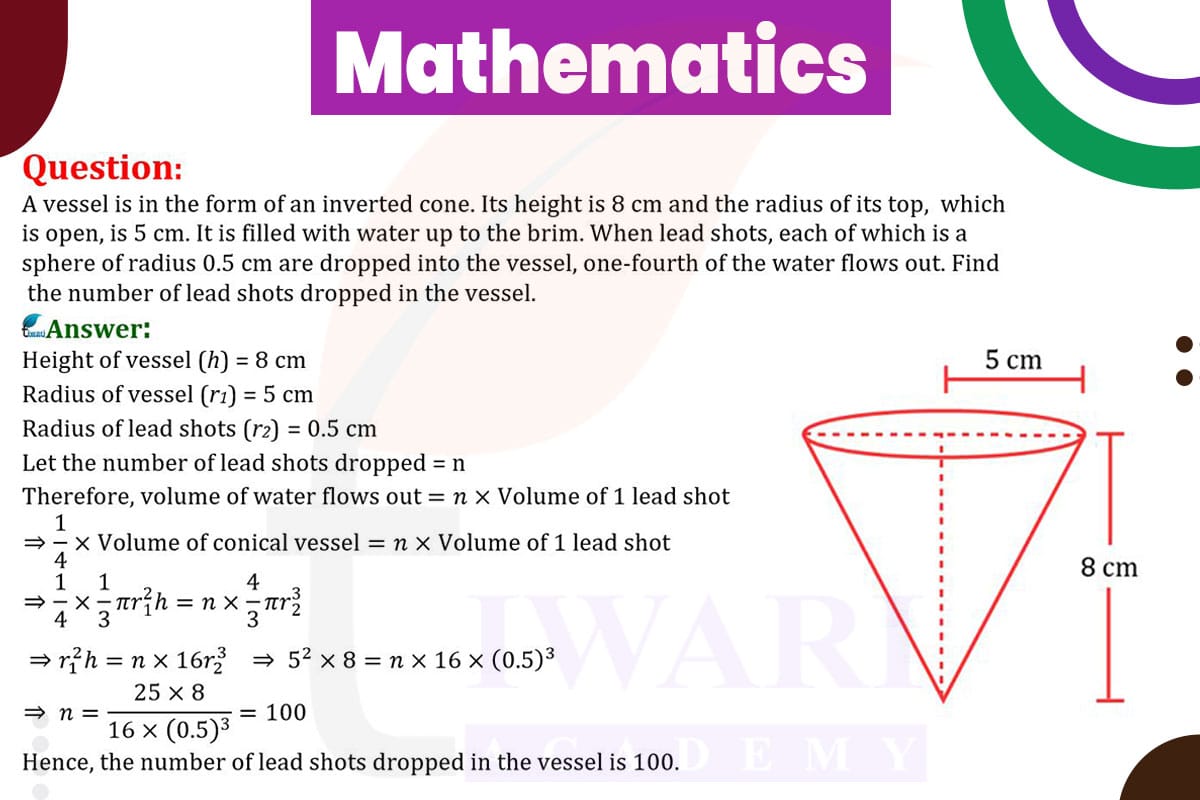
The vessel is an inverted cone with a height of 8 cm and a radius of 5 cm. The volume of a cone is (1/3)πr²h. So, the total volume is (1/3)π×5²×8.
One-fourth of this volume is the volume of water that overflows, which is equal to the total volume of the lead shots. The volume of one lead shot, which is a sphere with a radius of 0.5 cm, is (4/3)πr³ = (4/3)π×0.5³.
Dividing the total volume of overflowed water by the volume of one lead shot gives the number of lead shots dropped into the vessel.

A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water up to the brim. When lead shots, each of which is a sphere of radius 0.5 cm are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped.

Let’s discuss in detail

Volume Displacement in a Conical Vessel

The problem at hand involves understanding the concept of volume displacement in a conical vessel. This is a classic application of the principles of geometry and physics, particularly the concept of Archimedes’ principle, which states that the volume of fluid displaced is equal to the volume of the object submerged. In this scenario, an inverted conical vessel with a height of 8 cm and a radius of 5 cm is filled to the brim with water. When lead shots, each being a small sphere with a radius of 0.5 cm, are dropped into the vessel, one-fourth of the water overflows. The goal is to determine the number of lead shots added to the vessel.

Calculating the Volume of the Conical Vessel

The first step is to calculate the total volume of the conical vessel. The formula for the volume of a cone is (1/3)πr²h, where r is the radius and h is the height. For our vessel, with a radius of 5 cm and a height of 8 cm, the total volume is (1/3)π×5²×8 cubic centimeters. This volume represents the maximum amount of water the vessel can hold before any lead shots are added.

Understanding the Volume Displacement by Lead Shots

When the lead shots are dropped into the vessel, they displace a certain volume of water, causing it to overflow. Since one-fourth of the water overflows, the volume of water displaced by the lead shots is equal to one-fourth of the total volume of the vessel. This relationship between the displaced water and the volume of the lead shots is key to solving the problem, as it allows us to equate the volume of displaced water to the combined volume of the lead shots.

Calculating the Volume of a Single Lead Shot
Each lead shot is a sphere with a radius of 0.5 cm. The volume of a sphere is given by (4/3)πr³. Therefore, the volume of one lead shot is (4/3)×0.5³ cubic centimeters. This calculation is crucial as it gives us the volume of a single lead shot, which will be used to determine how many such shots can fit into the volume of water that was displaced.

Determining the Number of Lead Shots

To find the number of lead shots dropped into the vessel, we divide the total volume of water displaced (one-fourth of the vessel’s volume) by the volume of a single lead shot. This division gives us the number of lead shots that would collectively displace an amount of water equal to one-fourth of the vessel’s total volume. This calculation is a direct application of the principle of volume displacement and provides the solution to our problem.

Practical Implications of Geometric Calculations

In conclusion, this problem illustrates the practical application of geometric principles in understanding real-world phenomena like volume displacement. It not only reinforces the understanding of how to calculate the volumes of different shapes but also highlights the importance of these calculations in understanding physical phenomena. Such problems bridge the gap between theoretical mathematics and practical physics, showcasing the relevance and application of mathematical concepts in everyday life. This exercise is a testament to the importance of geometry in solving practical problems and understanding the world around us.

Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail

A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π.

Rachel, an engineering student, was asked to make a model shaped like a cylinder with two cones attached at its two ends by using a thin aluminium sheet. The diameter of the model is 3 cm and its length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model that Rachel made.

A gulab jamun, contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 gulab jamuns, each shaped like a cylinder with two hemispherical ends with length 5 cm and diameter 2.8 cm.

A pen stand made of wood is in the shape of a cuboid with four conical depressions to hold pens. The dimensions of the cuboid are 15 cm by 10 cm by 3.5 cm. The radius of each of the depressions is 0.5 cm and the depth is 1.4 cm. Find the volume of wood in the entire stand.

A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water up to the brim. When lead shots, each of which is a sphere of radius 0.5 cm are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped in the vessel.

A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1 cm³ of iron has approximately 8g mass.

A solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm.

A spherical glass vessel has a cylindrical neck 8 cm long, 2 cm in diameter; the diameter of the spherical part is 8.5 cm. By measuring the amount of water it holds, a child finds its volume to be 345 cm³. Check whether she is correct, taking the above as the inside measurements, and π = 3.14.