To check the child’s measurement of the volume of the spherical glass vessel, we calculate the volume of both the spherical part and the cylindrical neck and sum them.
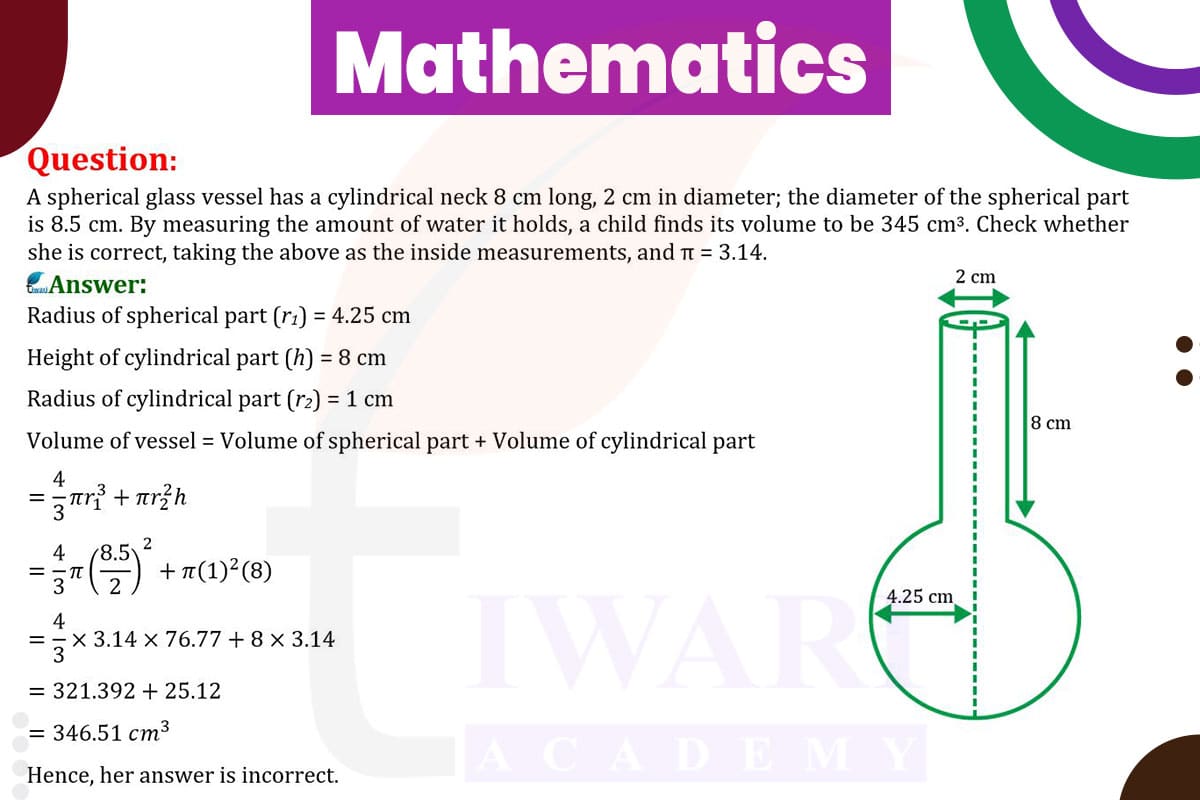
The diameter of the spherical part is 8.5 cm, giving a radius of 4.25 cm. The volume of a sphere is (4/3)πr³. So, the volume of the spherical part is (4/3)×3.14×4.25³.
The cylindrical neck is 8 cm long with a diameter of 2 cm, giving a radius of 1 cm. The volume of a cylinder is πr²h. So, the volume of the neck is 3.14×1²×8.
Adding the volumes of the spherical part and the cylindrical neck gives the total volume of the vessel. This total volume can be compared with the child’s measurement of 345 cm³ to check its accuracy.
Let’s discuss in detail
Volume Calculation of a Composite Object
The task involves verifying the accuracy of a child’s measurement of the volume of a spherical glass vessel with a cylindrical neck. This problem is a practical application of geometry, specifically in calculating the volume of composite shapes. The vessel consists of two distinct parts: a spherical section and a cylindrical neck. The spherical part has a diameter of 8.5 cm, and the cylindrical neck is 8 cm long with a diameter of 2 cm. Using these dimensions, we aim to calculate the total volume of the vessel and compare it with the child’s measurement of 345 cm³ to determine its accuracy.
Calculating the Volume of the Spherical Part
The first step is to calculate the volume of the spherical part of the vessel. The formula for the volume of a sphere is (4/3)πr³, where r is the radius. The diameter of the sphere is given as 8.5 cm, which makes the radius 4.25 cm (half of the diameter). Substituting these values into the formula, we get (4/3)×3.14×4.25³ cubic centimeters as the volume of the spherical part. This calculation is crucial as it represents the major portion of the vessel’s volume.
Determining the Volume of the Cylindrical Neck
Next, we calculate the volume of the cylindrical neck of the vessel. The formula for the volume of a cylinder is πr²h, where r is the radius and h is the height (or length). The neck has a diameter of 2 cm, giving a radius of 1 cm, and a length of 8 cm. Therefore, the volume of the cylindrical neck is 3.14×1²×8 cubic centimeters. This volume, though smaller than that of the spherical part, is significant in contributing to the total volume of the vessel.
Total Volume of the Glass Vessel
To find the total volume of the glass vessel, we add the volumes of the spherical part and the cylindrical neck. The sum of these volumes gives the total volume of the vessel. This step is essential because it combines the volumes of the two different geometric shapes that make up the vessel, providing a comprehensive calculation of its capacity.
Comparing Calculated Volume with the Child’s Measurement
With the total volume calculated, the next step is to compare it with the child’s measurement of 345 cm³. This comparison is crucial to determine the accuracy of the child’s measurement. If the calculated volume closely matches the child’s measurement, it validates her findings. If there is a significant discrepancy, it may indicate a miscalculation or an error in the measurement process.
Importance of Accurate Volume Calculation
In conclusion, this exercise demonstrates the importance of accurate volume calculation in everyday scenarios. It highlights the practical application of geometric principles in real-life situations, such as measuring the capacity of containers. This problem-solving approach is not just about applying mathematical formulas; it’s about understanding the spatial properties of objects and their practical implications. Such calculations are essential in various fields, including manufacturing, cooking, and science experiments, where precise measurements are crucial for success and accuracy.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail

