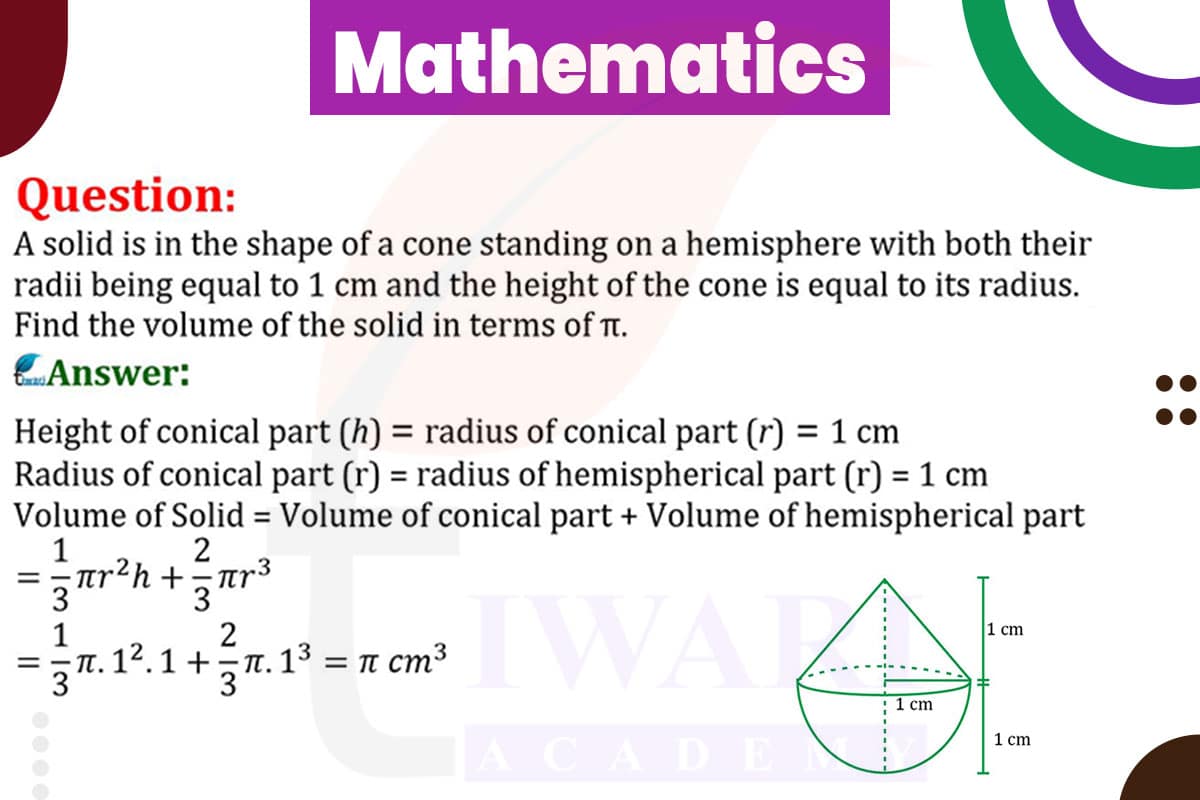
To find the volume of a solid composed of a cone standing on a hemisphere, where both have a radius of 1 cm and the height of the cone is also 1 cm, we calculate the volumes of the cone and the hemisphere separately and then add them.
The volume of a cone is given by (1/3)πr²h. With r = 1 cm and h = 1 cm, the volume of the cone is (1/3)π × 1² × 1 = (1/3)π cm³.
The volume of a hemisphere is half the volume of a sphere, which is
(2/3)πr³. With r = 1 cm, the volume of the hemisphere is (2/3) π × 1³ = (2/3) π cm³.
Adding these, the total volume of the solid is (1/3) π + (2/3) π = π cm³. This represents the volume of the solid in terms of π.
Let’s discuss in detail
Introduction to Composite Geometric Shapes
The calculation of the volume of a solid composed of a cone standing on a hemisphere is a classic problem in geometry that demonstrates how to deal with composite shapes. This problem is not just a theoretical exercise in spatial reasoning; it has practical applications in fields such as design and engineering. In this case, both the cone and the hemisphere have a radius of 1 cm, and the height of the cone is also 1 cm. Understanding how to calculate the volume of such a composite shape requires a clear grasp of the formulas for the volumes of individual shapes and how these volumes combine.
Understanding the Cone’s Volume
The first step in solving this problem is to calculate the volume of the cone. The formula for the volume of a cone is (1/3)πr²h, where r is the radius and h is the height. In our case, both the radius and the height of the cone are 1 cm. This simplifies the calculation, as squaring the radius (1 cm) and multiplying by the height (1 cm) results in the same value. The volume of the cone thus becomes (1/3)×1²×1 = (1/3)π cm³.
Calculating the Hemisphere’s Volume
Next, we calculate the volume of the hemisphere. The volume of a hemisphere is half the volume of a sphere, which is given by (4/3)πr³. Therefore, the volume of the hemisphere is (2/3)πr³. With a radius of 1 cm, the calculation simplifies to (2/3)π×1³ = (2/3)π cm³. This step is crucial as it gives us the volume of the lower half of our composite solid, which is the base upon which the cone stands.
Total Volume of the Composite Solid
To find the total volume of the composite solid, we add the volumes of the cone and the hemisphere. The sum of (1/3) π cm³ (the volume of the cone) and (2/3)π cm³ (the volume of the hemisphere) gives us the total volume. This addition is straightforward since both volumes are expressed in terms of π, facilitating easy calculation. The total volume of the solid is thus (1/3)π + (2/3)π = π cm³.
Practical Implications of the Calculation
This calculation has practical implications in various fields. For instance, in manufacturing and design, understanding the volume of composite shapes is crucial for material estimation, cost calculation, and design efficiency. This problem exemplifies how geometric principles are applied in real-world scenarios, bridging the gap between theoretical mathematics and practical application. It highlights the importance of precision and accuracy in calculations, which are essential in these fields.
The Elegance of Geometric Calculations
In conclusion, the process of calculating the volume of a solid composed of a cone on a hemisphere demonstrates the elegance and utility of geometric calculations. This exercise reinforces the understanding of how to deal with composite shapes and the importance of accurate calculations in practical applications. It showcases the beauty of geometry in solving complex problems and its relevance in everyday practical situations. This problem-solving approach is not just about numbers and formulas; it’s about understanding the spatial properties of objects and their applications in the real world.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail

