To find the mass of the solid iron pole, we first calculate the volume of each cylindrical part and then find the total volume. The mass is then determined by multiplying the total volume by the mass per unit volume of iron.
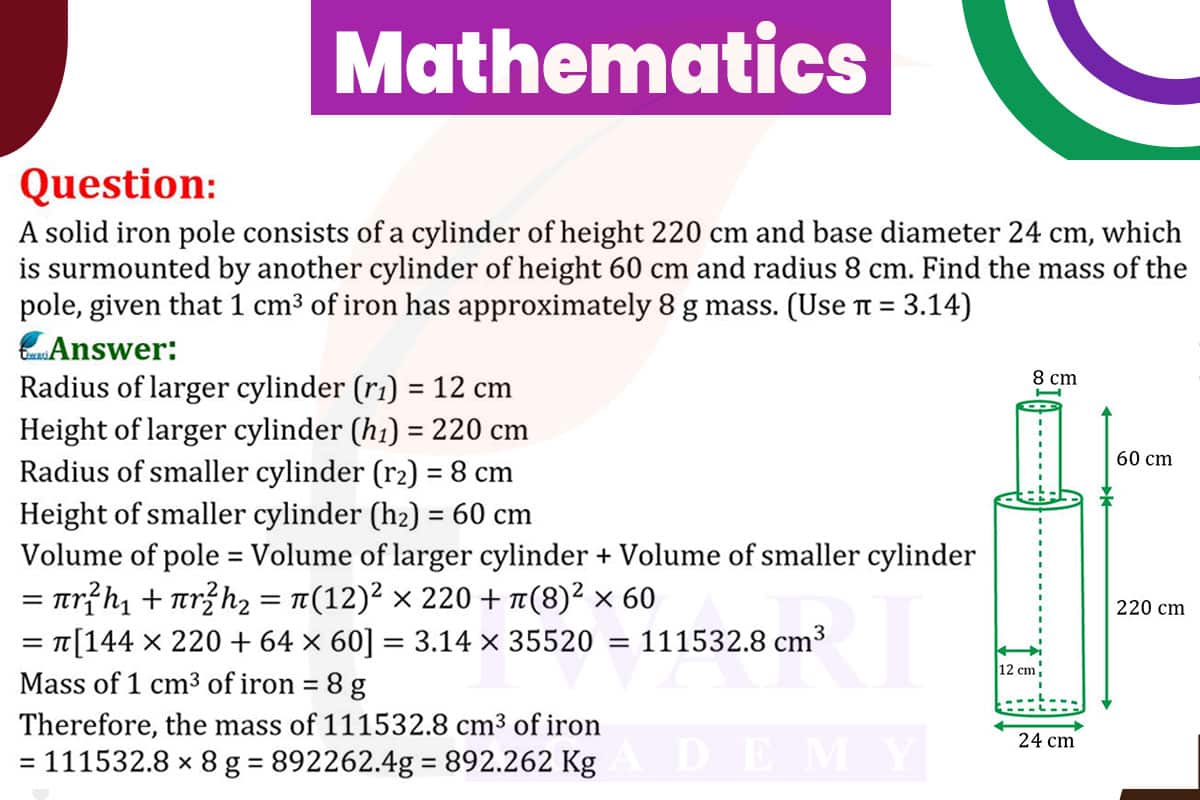
The first cylinder has a height of 220 cm and a base diameter of 24 cm, giving a radius of 12 cm. Its volume is πr²h = 3.14×12²×220.
The second cylinder has a height of 60 cm and a radius of 8 cm. Its volume is πr²h = 3.14×8²×60.
Adding these volumes gives the total volume of the pole. Multiplying this total volume by the mass per unit volume of iron (8g per cm³) gives the total mass of the pole.
Let’s discuss in detail
Calculating the Mass of a Composite Cylindrical Structure
The problem at hand involves calculating the mass of a solid iron pole, which is a composite structure consisting of two cylindrical sections. This task is a practical application of geometry and physics, particularly in understanding how to calculate the volume of composite shapes and then use density to find mass. The pole is made up of a larger cylinder with a height of 220 cm and a base diameter of 24 cm, surmounted by a smaller cylinder with a height of 60 cm and a radius of 8 cm. Given that the mass of 1 cm³ of iron is approximately 8g, the challenge is to determine the total mass of the pole.
Calculating the Volume of the Larger Cylinder
The first step is to calculate the volume of the larger cylinder. The formula for the volume of a cylinder is πr²h, where r is the radius and h is the height. For the larger cylinder, the diameter is 24 cm, giving a radius of 12 cm (half of the diameter). The height is 220 cm. Therefore, the volume of the larger cylinder is 3.14 × 12² ×
220 cubic centimeters. This calculation is crucial as it represents the bulk of the pole’s volume.
Determining the Volume of the Smaller Cylinder
Next, we calculate the volume of the smaller cylinder that surmounts the larger one. Using the same volume formula, πr²h, and the given dimensions of a radius of 8 cm and a height of 60 cm, the volume of the smaller cylinder is 3.14 × 8² × 60 cubic centimeters. This volume, though smaller than that of the larger cylinder, is significant in contributing to the total volume of the pole.
Total Volume of the Iron Pole
To find the total volume of the iron pole, we add the volumes of the larger and smaller cylinders. The sum of these volumes gives us the total volume of iron used in the pole. This step is essential because the mass of the pole is directly proportional to its total volume, given the uniform density of the material.
Calculating the Mass of the Pole
With the total volume of the pole determined, the next step is to calculate its mass. The mass of an object is the product of its volume and density. Given that the density of iron is approximately 8g per cm³, we multiply the total volume of the pole by this density. This multiplication gives us the total mass of the pole in grams, providing a practical understanding of the weight of such a composite cylindrical structure.
Practical Implications in Engineering and Design
In conclusion, this exercise of calculating the mass of a solid iron pole with two cylindrical sections demonstrates the practical application of geometric and physical principles in engineering and design. It highlights the importance of understanding the properties of materials and the role of geometry in determining the physical characteristics of structures. Such calculations are crucial in fields like construction, manufacturing, and material science, where accurate estimations of mass and volume are essential for the design, stability, and functionality of various structures and objects. This problem-solving approach underscores the interconnectedness of mathematics, physics, and practical applications in the real world.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail

