A solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm.

Let’s discuss in detail

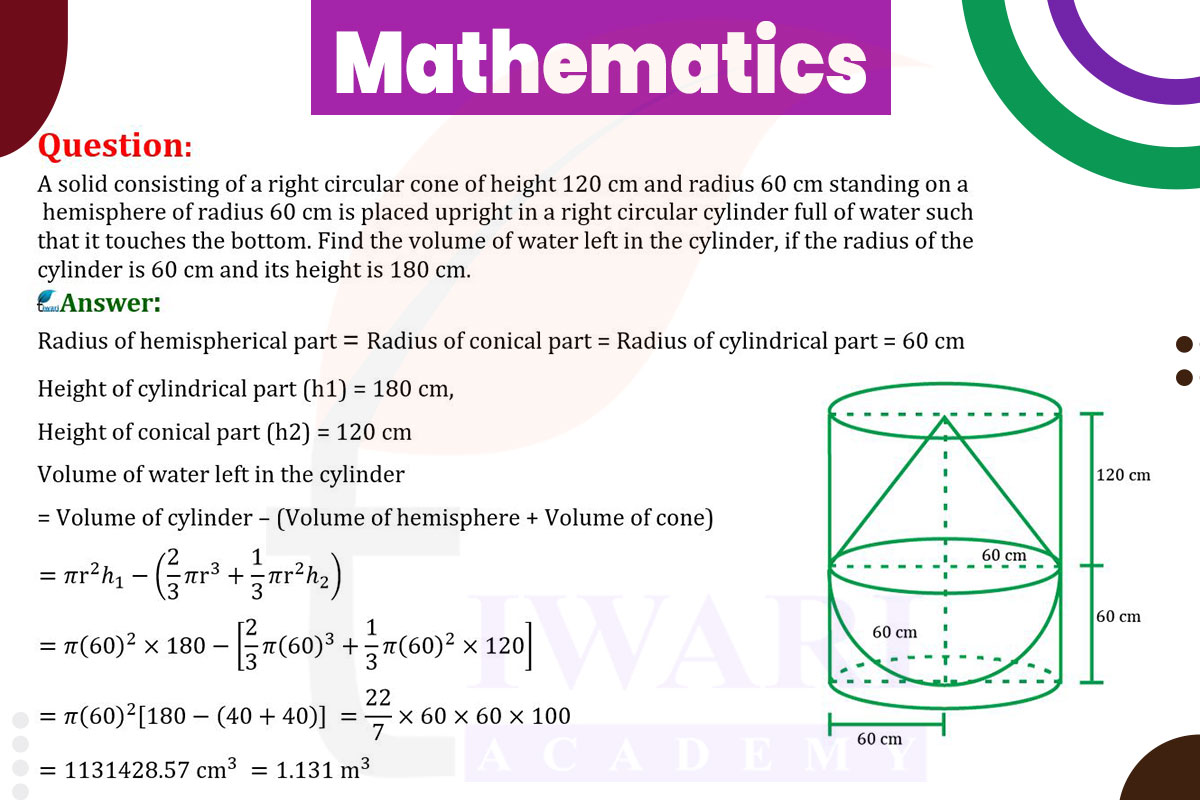
The problem presents a classic scenario in fluid mechanics and geometry: calculating the volume of water displaced by a solid object submerged in a cylinder. This is a practical application of the principle of displacement, first discovered by Archimedes. The solid object in question is a composite shape, consisting of a right circular cone standing on a hemisphere, both with a radius of 60 cm. The cone has a height of 120 cm. This solid is placed upright in a right circular cylinder, which is full of water. The cylinder has a radius of 60 cm and a height of 180 cm. The objective is to determine the volume of water remaining in the cylinder after the solid is submerged.

The first step in solving this problem is to calculate the total volume of the cylinder, which represents the initial volume of water. The formula for the volume of a cylinder is πr²h, where r is the radius and h is the height. For our cylinder, with a radius of 60 cm and a height of 180 cm, the volume is π × 60² × 180 cubic centimeters. This volume is crucial as it sets the maximum capacity of water the cylinder can hold.

Next, we calculate the volume of the cone, which is part of the solid object submerged in the water. The formula for the volume of a cone is (1/3)πr²h. With a radius of 60 cm and a height of 120 cm, the volume of the cone is (1/3)π×60²×120 cubic centimeters. This volume is significant as it represents a portion of the space that the solid occupies in the cylinder.

Determining the Volume of the Hemisphere
The other part of the solid object is a hemisphere. The formula for the volume of a hemisphere is (2/3)πr³. With a radius of 60 cm, the volume of the hemisphere is (2/3)π×60³ cubic centimeters. This volume, combined with that of the cone, gives the total volume of the solid object that displaces the water in the cylinder.

To find the total volume displaced by the solid, we add the volumes of the cone and the hemisphere. This total volume represents the amount of water that will be displaced when the solid is submerged in the cylinder. The principle of displacement states that the volume of fluid displaced by a submerged object is equal to the volume of the object. Therefore, the combined volume of the cone and hemisphere is the key to determining how much water is displaced from the cylinder.

Calculating the Volume of Water Left in the Cylinder
The final step is to calculate the volume of water left in the cylinder. This is done by subtracting the total volume displaced by the solid (the sum of the volumes of the cone and hemisphere) from the total volume of the cylinder. The result gives us the volume of water that remains in the cylinder after the solid object is submerged. This calculation is essential for understanding the relationship between the volume of a submerged object and the volume of fluid it displaces.

In conclusion, this exercise demonstrates the practical application of geometric principles and the principle of displacement in fluid mechanics. It highlights the importance of understanding the volumes of various shapes and how they interact with fluids. Such calculations are crucial in fields like engineering, design, and physics, where understanding the behavior of fluids and solids in confined spaces is essential. This problem-solving approach not only reinforces the understanding of geometric calculations but also illustrates the real-world applications of these concepts in understanding and predicting the behavior of physical systems.

Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail