A gulab jamun, contains sugar syrup up to about 30% of its volume.

Updated by Tiwari Academy
on November 28, 2023, 9:24 AM

To find the amount of syrup in 45 gulab jamuns, each shaped like a cylinder with two hemispherical ends, we first calculate the volume of one gulab jamun and then determine 30% of this volume.
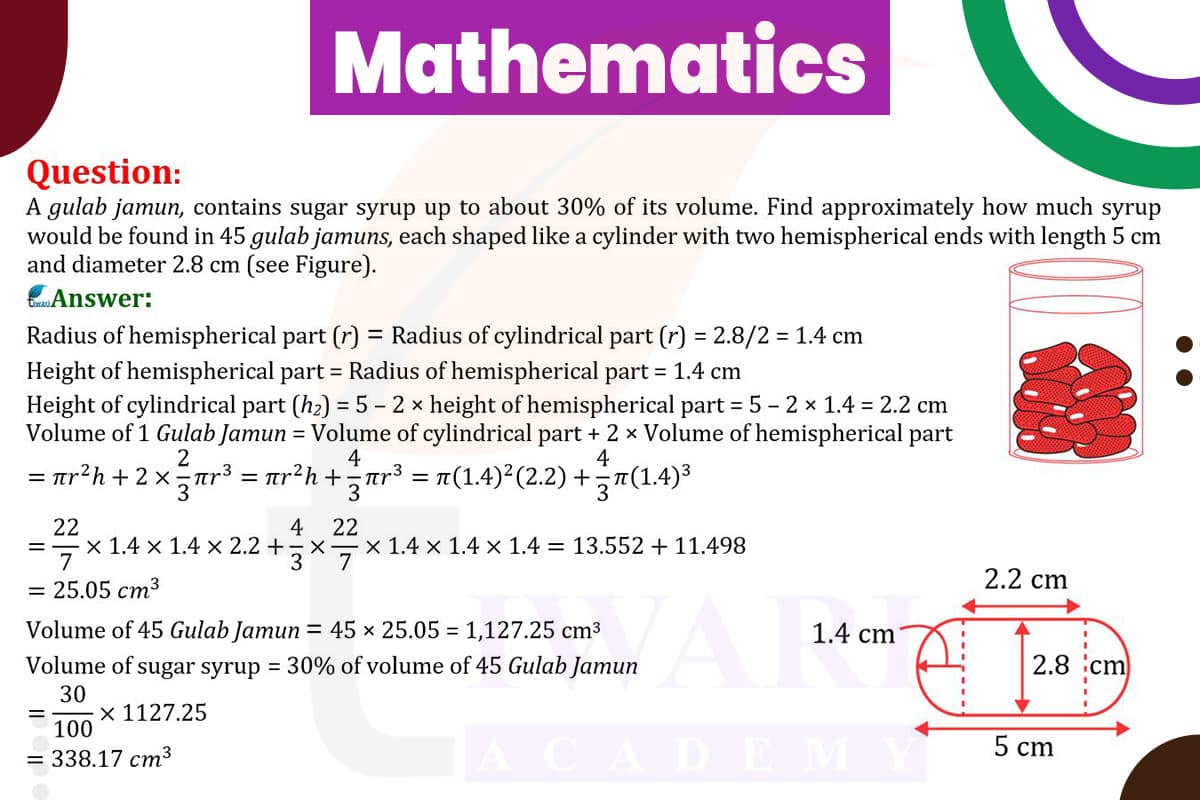
The total length of each gulab jamun is 5 cm and the diameter is 2.8 cm, giving a radius of 1.4 cm. The length of the cylindrical part is the total length minus the diameter of the two hemispheres, so it’s
5 cm − 2 × 1.4 cm = 2.2cm.
The volume of the cylindrical part is πr²h = π × 1.4² ×2.2. The volume of each hemisphere is (2/3)πr³, and there are two hemispheres, so their combined volume is 2 × (2/3) π × 1.4³.
The total volume of one gulab jamun is the sum of the volumes of the cylinder and the two hemispheres. 30% of this volume is the syrup content in one gulab jamun. Multiply this by 45 to find the syrup content in 45 gulab jamuns.

A gulab jamun, contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 gulab jamuns, each shaped like a cylinder with two hemispherical ends with length 5 cm and dia 2.8 cm.

Let’s discuss in detail

Volume Calculation in Culinary Applications

The task of calculating the volume of syrup in a batch of 45 gulab jamuns, a popular South Asian sweet, is an intriguing application of geometry in culinary arts. Each gulab jamun is shaped like a cylinder with two hemispherical ends, a common design in many food items. Understanding the volume of these sweets is not just a theoretical exercise; it has practical implications in recipe formulation and nutritional analysis. Given the dimensions of each gulab jamun – a length of 5 cm and a diameter of 2.8 cm – the challenge is to calculate the total volume and then determine the volume of syrup, which constitutes 30% of each sweet.

Analyzing the Geometric Shape of Gulab Jamuns

Each gulab jamun is a composite shape, combining a cylindrical middle section with hemispherical ends. This shape is typical in various culinary designs due to its aesthetic appeal and functional form. The diameter of 2.8 cm gives a radius of 1.4 cm for both the cylinder and the hemispheres. The total length of the gulab jamun is 5 cm, which includes the cylindrical part and the two hemispheres. Understanding these dimensions is crucial for accurately calculating the volume of each component of the gulab jamun.

Calculating the Volume of the Cylindrical Section

The first step in the volume calculation is to determine the length of the cylindrical section. This is done by subtracting the diameters of the two hemispheres from the total length of the gulab jamun. Since the diameter of each hemisphere is equal to the diameter of the cylinder (2.8 cm), the length of the cylindrical section is 5cm − 2×1.4 cm = 2.2cm. The volume of the cylindrical part is then calculated using the formula πr²h, where r is the radius and h is the height (or length) of the cylinder.

Determining the Volume of the Hemispherical Ends
Next, we calculate the volume of the hemispherical ends. The volume of a hemisphere is half that of a sphere, given by (2/3)πr³. Since each gulab jamun has two hemispherical ends, we calculate the volume of one hemisphere and then double it to account for both. This calculation is essential to determine the total volume of the gulab jamun, as it contributes significantly to the overall size of the sweet.

Total Volume and Syrup Content Calculation

After calculating the volumes of the cylindrical section and the hemispherical ends, we sum these to find the total volume of one gulab jamun. The syrup content in each gulab jamun is 30% of this total volume. This percentage is crucial in culinary preparations, as it determines the sweetness and texture of the sweet. By calculating 30% of the total volume of one gulab jamun and then multiplying this by 45, we can estimate the total volume of syrup in the entire batch of gulab jamuns.

Practical Applications of Geometry in Culinary Arts

In conclusion, this exercise of calculating the syrup content in gulab jamuns demonstrates the practical application of geometric principles in the culinary arts. It highlights the importance of precision in recipe formulation and the role of mathematics in understanding the composition of food items. This problem-solving approach is not just about applying formulas; it’s about understanding the spatial properties of food items and their implications in culinary preparation and presentation. Such calculations are essential for chefs, food technologists, and culinary enthusiasts who seek to perfect their recipes and understand the science behind their culinary creations.

Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail

A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π.

Rachel, an engineering student, was asked to make a model shaped like a cylinder with two cones attached at its two ends by using a thin aluminium sheet. The diameter of the model is 3 cm and its length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model that Rachel made.

A gulab jamun, contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 gulab jamuns, each shaped like a cylinder with two hemispherical ends with length 5 cm and diameter 2.8 cm.

A pen stand made of wood is in the shape of a cuboid with four conical depressions to hold pens. The dimensions of the cuboid are 15 cm by 10 cm by 3.5 cm. The radius of each of the depressions is 0.5 cm and the depth is 1.4 cm. Find the volume of wood in the entire stand.

A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water up to the brim. When lead shots, each of which is a sphere of radius 0.5 cm are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped in the vessel.

A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1 cm³ of iron has approximately 8g mass.

A solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm.

A spherical glass vessel has a cylindrical neck 8 cm long, 2 cm in diameter; the diameter of the spherical part is 8.5 cm. By measuring the amount of water it holds, a child finds its volume to be 345 cm³. Check whether she is correct, taking the above as the inside measurements, and π = 3.14.