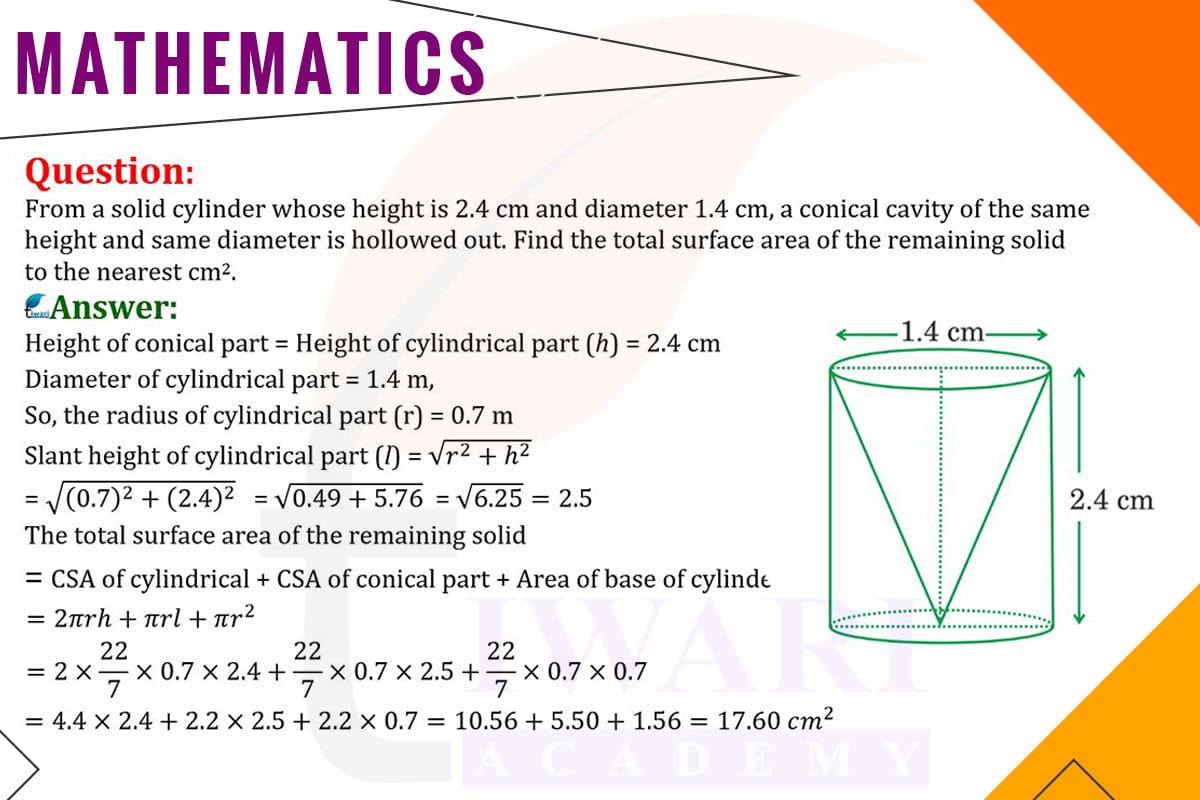
To find the total surface area of the remaining solid after hollowing out a conical cavity from a cylinder, we need to calculate the surface areas of the cylindrical part and the inner conical cavity.
The height of both the cylinder and the cone is 2.4 cm, and their diameter is 1.4 cm, giving a radius of 0.7 cm. The surface area of the cylindrical part is the sum of its lateral surface area and one base (since the other base is removed to form the cavity). The lateral surface area of the cylinder is 2πrh = 2π × 0.7 × 2.4, and the area of one base is πr² = π × 0.7². The surface area of the conical cavity is just its lateral surface area, πrl, where l is the slant height. The slant height can be found using Pythagoras’ theorem, √[r² + h²]. After calculating l, substitute it into the formula for the cone’s surface area.
Adding the cylindrical part’s surface area and the conical cavity’s surface area gives the total surface area of the remaining solid.
Let’s discuss in detail
Introduction to Complex Geometric Structures
The task of calculating the total surface area of a solid formed by hollowing out a conical cavity from a cylinder presents a fascinating challenge in the field of geometry. This problem involves a cylinder and a cone that share the same height and diameter, creating a unique composite shape. The objective is to determine the surface area of this complex structure, which requires an understanding of the surface areas of both the cylinder and the cone. This type of problem is not just a mathematical exercise; it’s a practical application of geometry in fields such as engineering and design, where understanding the properties of combined shapes is crucial.
Understanding the Dimensions of the Cylinder
The first step in our calculation is to understand the dimensions of the cylinder. Given a height of 2.4 cm and a diameter of 1.4 cm, we can determine the radius as half the diameter, which is 0.7 cm. The surface area of the cylindrical part includes its lateral surface area and the area of one of its circular bases (since the other base forms the opening for the conical cavity). The lateral surface area is calculated using the formula 2πrh, where r is the radius and h is the height.
Calculating the Surface Area of the Cylindrical Part
To calculate the surface area of the cylindrical part, we use the radius of 0.7 cm and the height of 2.4 cm. The lateral surface area is 2π × 0.7 × 2.4, and the area of the base is π × 0.7². These calculations give us the total surface area of the cylindrical part of the solid, which forms the external boundary of the structure.
Analyzing the Conical Cavity
Next, we focus on the conical cavity that has been hollowed out. The cone shares the same height and diameter as the cylinder, so its radius is also 0.7 cm, and its height is 2.4 cm. The surface area of the conical cavity is its lateral surface area, calculated using the formula πrl, where l is the slant height. The slant height can be found using Pythagoras’ theorem, √[r² + h²].
Calculating the Surface Area of the Conical Cavity
After determining the slant height of the cone, we substitute the values into the formula for the cone’s surface area. This calculation gives us the area of the inner surface of the conical cavity. It’s important to note that this area represents the inner surface that has been removed from the original cylinder, contributing to the total surface area of the remaining solid.
Total Surface Area of the Remaining Solid
To find the total surface area of the remaining solid, we add the surface areas of the cylindrical part and the conical cavity. This sum represents the entire surface area of the solid, encompassing both the outer surface of the cylinder and the inner surface of the conical cavity. This calculation is essential in understanding the extent of the surface that covers the entire structure, providing insights into the material requirements if the solid were to be constructed or covered. The result is a comprehensive understanding of the surface area of a complex geometric structure formed by combining a cylinder and a cone.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail

