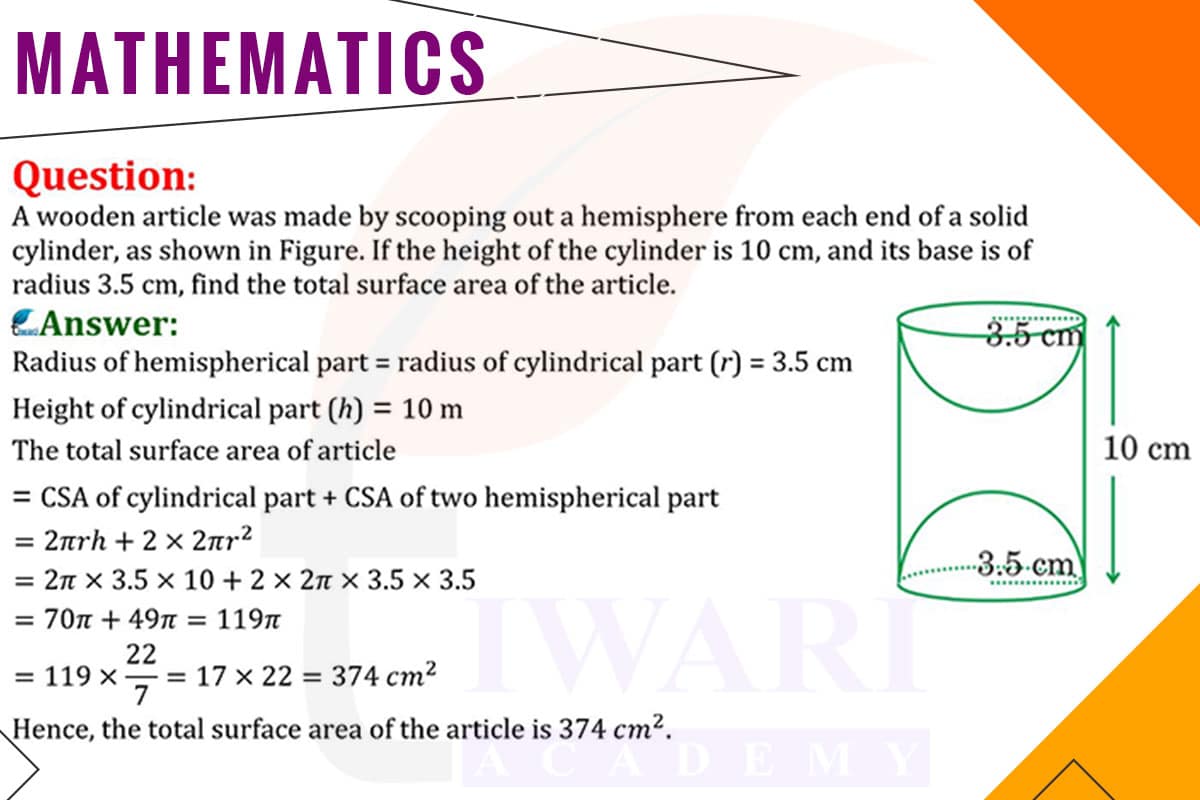
To find the total surface area of a wooden article made by scooping out a hemisphere from each end of a solid cylinder, we calculate the surface areas of the cylindrical part and the hemispherical cavities.
The height of the cylinder is 10 cm, and the radius of its base (and the hemispheres) is 3.5 cm. The surface area of the cylindrical part is the lateral surface area, given by 2πrh = 2π × 3.5 × 10.
The surface area of each hemispherical cavity is half the surface area of a sphere, 2πr². Since there are two such cavities, their combined surface area is 2×2πr².
The total surface area of the article is the sum of the cylindrical part’s surface area and the surface areas of the two hemispherical cavities. This calculation gives the total surface area of the wooden article.
Let’s discuss in detail
Introduction to Geometric Design in Woodworking
The creation of a wooden article by scooping out a hemisphere from each end of a solid cylinder is a classic example of applying geometric principles in woodworking and design. This task involves understanding the interaction between cylindrical and hemispherical shapes to determine the total surface area of the resulting object. The cylinder has a height of 10 cm and a base radius of 3.5 cm, and hemispheres of the same radius are removed from both ends. Calculating the total surface area of this composite shape not only requires a grasp of geometric formulas but also illustrates how geometry is used in practical design and craftsmanship.
Analyzing the Cylindrical Component
The first step in our calculation is to analyze the cylindrical part of the article. The cylinder, before the hemispheres are scooped out, has a lateral surface area and two circular bases. However, once the hemispheres are removed, the bases are no longer part of the surface area. The lateral surface area of a cylinder is calculated using the formula 2πrh, where r is the radius and h is the height. For our cylinder, this translates to 2π × 3.5 × 10, which will give us the area of the sides of the cylinder.
Understanding the Hemispherical Scoops
Next, we consider the hemispherical scoops removed from each end of the cylinder. The surface area of a hemisphere is half that of a full sphere, which is calculated by the formula 2πr². Since there are two hemispheres, their combined surface area is 2×2πr². However, it’s important to note that scooping out the hemispheres exposes their internal surfaces, which then become part of the article’s total surface area.
Calculating the Total Surface Area
To find the total surface area of the wooden article, we add the surface area of the cylindrical part and the internal surface areas of the two hemispheres. This involves summing the lateral surface area of the cylinder and the surface areas of the two hemispherical scoops. The calculation is straightforward but requires careful consideration of the surfaces that contribute to the total area, especially since the bases of the cylinder are removed in the process.
Practical Implications in Woodworking
This calculation has practical implications in woodworking and manufacturing. Understanding the total surface area is crucial for material estimation, cost calculation, and aesthetic design. For instance, if the article is to be painted or varnished, knowing the total surface area helps in determining the amount of paint or varnish needed. This problem exemplifies how geometric principles are applied in real-world scenarios, highlighting the importance of precision and practical application in design and craftsmanship.
Geometry in Everyday Objects
In conclusion, calculating the surface area of a wooden article made by scooping out hemispheres from a cylinder demonstrates the practical application of geometry in everyday objects. This exercise not only reinforces our understanding of geometric shapes and their properties but also highlights the importance of these calculations in practical design and manufacturing. It showcases the integration of mathematical concepts in real-world applications, emphasizing the role of geometry in the design and creation of functional and aesthetic objects.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail

