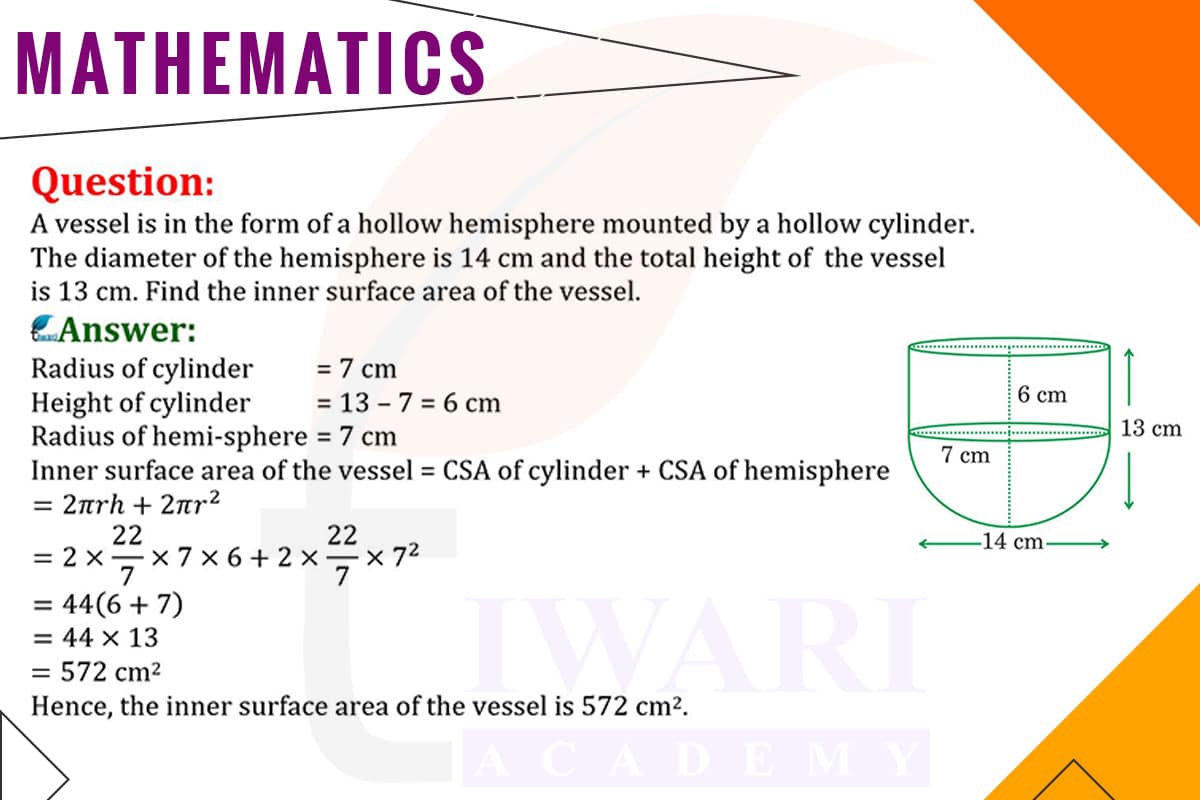
To find the inner surface area of a vessel composed of a hollow hemisphere mounted by a hollow cylinder, we need to calculate the surface areas of both parts separately and then sum them up. The diameter of the hemisphere is given as 14 cm, so its radius r is 7 cm. The total height of the vessel is 13 cm.
The height of the cylindrical part can be found by subtracting the radius of the hemisphere from the total height of the vessel, which is 13cm − 7cm = 6cm.
The inner surface area of the hemisphere is 2πr², and the inner surface area of the cylinder is 2πrh (since we only consider the lateral surface area for the hollow part). Substituting the values, we get 2π × 7² for the hemisphere and 2π × 7 × 6 for the cylinder.
Calculating these, the total inner surface area of the vessel is
308π + 84π = 392π square cm. Thus, the inner surface area of the vessel is 392π cm², which is approximately 1232.16 cm².
Let’s discuss in detail
Introduction to Geometric Problem Solving
In the fascinating world of geometry, solving problems often involves dissecting complex shapes into simpler, more manageable components. This approach is particularly useful when dealing with composite shapes, such as a vessel made of a hollow hemisphere mounted on a hollow cylinder. The challenge here is to find the inner surface area of this vessel. This task not only tests our understanding of basic geometric formulas but also our ability to apply these formulas to real-world objects. By breaking down the vessel into its constituent parts – the hemisphere and the cylinder – we can methodically calculate the surface area of each and combine them to find the total inner surface area.
Understanding the Hemisphere Component
The first step in our calculation involves understanding the dimensions of the hemisphere. Given that the diameter of the hemisphere is 14 cm, its radius (r) is half of this, which is 7 cm. The inner surface area of a hemisphere is calculated using the formula
2πr². This formula accounts for the fact that a hemisphere is essentially half of a sphere, and thus, its surface area is half that of a sphere’s outer surface. The calculation of the hemisphere’s surface area is crucial as it represents a significant portion of the vessel’s inner surface.
Calculating the Cylinder’s Dimensions
Next, we turn our attention to the cylindrical part of the vessel. The total height of the vessel is given as 13 cm. To find the height of the cylinder, we subtract the radius of the hemisphere from the total height. Since the radius of the hemisphere is 7 cm, the height of the cylinder is 13cm − 7cm = 6cm. This height is essential for calculating the inner surface area of the cylindrical portion, which, unlike a full cylinder, does not include the top and bottom surfaces.
Computing the Cylinder’s Surface Area
The inner surface area of the cylinder is given by the formula 2πrh, where r is the radius and h is the height of the cylinder. This formula calculates the lateral surface area of the cylinder, which is the area of the curved surface excluding the top and bottom. In our case, the radius is 7 cm, and the height is 6 cm. By substituting these values into the formula, we can determine the inner surface area of the cylindrical part of the vessel.
Total Inner Surface Area of the Vessel
Having calculated the inner surface areas of both the hemisphere and the cylinder, we now combine these to find the total inner surface area of the vessel. This involves simply adding the surface area of the hemisphere and the cylinder. The sum of these areas gives us the total area that the inner surface of the vessel covers. This step is crucial as it brings together the individual calculations into a single, comprehensive answer, reflecting the total inner surface area of the composite vessel.
The Elegance of Geometric Calculation
In conclusion, the process of calculating the inner surface area of a vessel composed of a hollow hemisphere and a hollow cylinder beautifully illustrates the elegance and precision of geometric problem-solving. By breaking down the problem into smaller parts, applying relevant formulas, and combining the results, we arrive at a clear and accurate solution. This exercise not only reinforces our understanding of geometric principles but also demonstrates their practical application in analyzing real-world objects. It is a testament to the power of mathematical reasoning in solving complex problems by methodically working through each step of the process.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail

