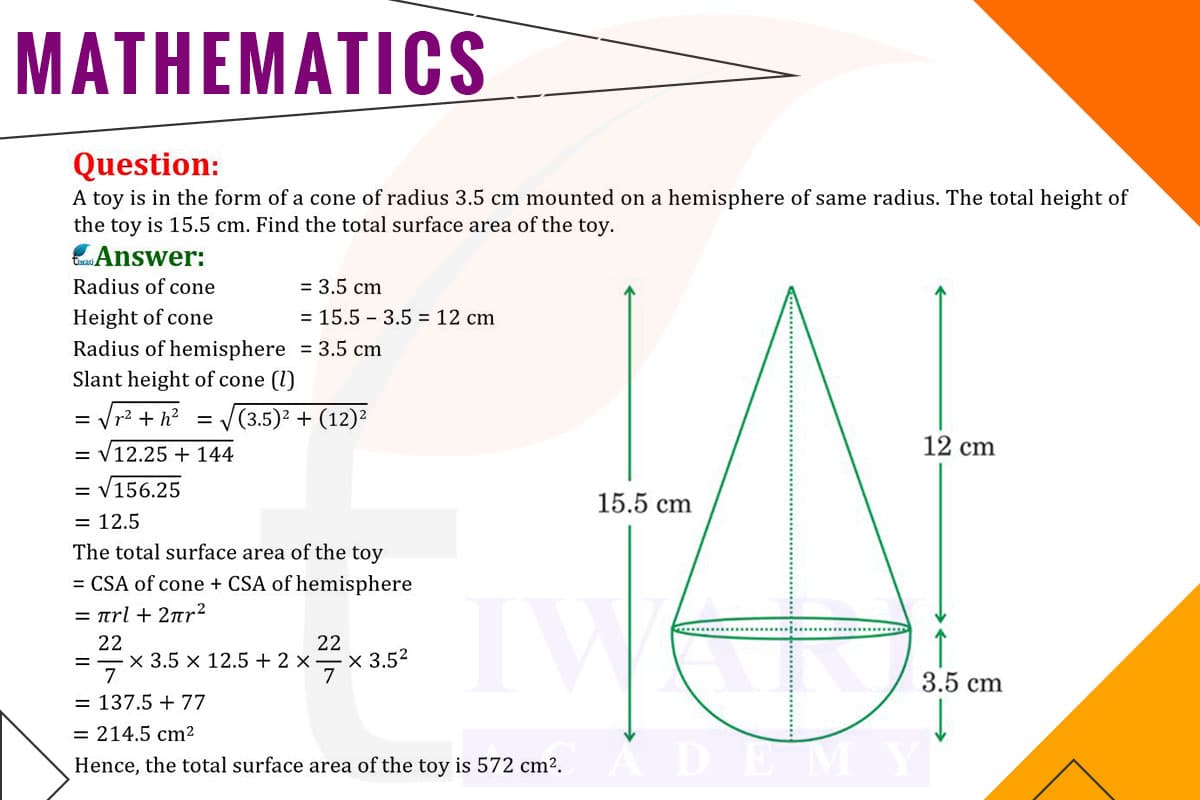
To find the total surface area of a toy consisting of a cone mounted on a hemisphere, both with a radius of 3.5 cm, and a total height of 15.5 cm, we first determine the height of the cone. The height of the cone is the total height minus the radius of the hemisphere, which is 15.5cm − 3.5cm = 12cm. The surface area of the cone (excluding the base) is given by πrl, where l is the slant height. The slant height can be found using Pythagoras’ theorem, l = √[r² + h²] = √[3.5² + 12²]. The surface area of the hemisphere is 2πr². Adding these two areas gives the total surface area of the toy.
Let’s discuss in detail
Geometric Analysis of a Toy
In the realm of geometry, analyzing composite shapes like a toy comprising a cone mounted on a hemisphere presents an intriguing challenge. This toy, with a radius of 3.5 cm for both the cone and the hemisphere and a total height of 15.5 cm, serves as an excellent example of applying geometric principles to real-world objects. The task at hand is to calculate the total surface area of this toy, which requires a methodical approach. By breaking down the toy into its constituent geometric shapes and applying relevant formulas, we can determine the total surface area. This process not only demonstrates the practical application of geometry but also enhances our understanding of how different shapes interact to form a composite structure.
Determining the Dimensions of the Cone
The first step in our calculation is to ascertain the dimensions of the cone. The total height of the toy is given as 15.5 cm, which includes the height of the cone and the radius of the hemisphere. Since the radius of the hemisphere is 3.5 cm, the height of the cone is the total height minus the radius of the hemisphere, resulting in 15.5cm − 3.5cm = 12cm. This height is crucial for calculating the cone’s surface area, as it directly influences the slant height, a key component in our calculations.
Calculating the Slant Height of the Cone
To calculate the surface area of the cone, we need to determine its slant height. The slant height (l) of a cone can be found using Pythagoras’ theorem, expressed as l = √[r² + h²], where r is the radius and h is the height of the cone. Substituting the known values, l = √[3.5² + 12²], we can calculate the slant height. This slant height is essential for determining the lateral surface area of the cone, which is a significant part of the toy’s total surface area.
Surface Area of the Cone
With the slant height determined, we can now calculate the surface area of the cone. The formula for the lateral surface area of a cone is πrl, where r is the radius and l is the slant height. By substituting the values for the radius and the calculated slant height, we obtain the surface area of the cone. This area represents the curved surface of the cone, excluding the base, which is not visible as it is attached to the hemisphere.
Surface Area of the Hemisphere
Next, we calculate the surface area of the hemisphere. The formula for the surface area of a hemisphere is 2πr². Since the radius of the hemisphere is 3.5 cm, the same as that of the cone, we can directly apply this formula. This calculation gives us the area of the curved surface of the hemisphere. It’s important to note that we only consider the outer surface area of the hemisphere, as the base of the hemisphere forms the joint with the cone.
Total Surface Area of the Toy
To find the total surface area of the toy, we add the surface areas of the cone and the hemisphere. This sum represents the entire outer surface area of the toy, encompassing both the curved surface of the cone and the hemisphere. This step is crucial as it combines the individual calculations into a comprehensive total, reflecting the complete surface area that the toy occupies.
Synthesizing Geometric Concepts
In conclusion, the calculation of the total surface area of a toy comprising a cone and a hemisphere exemplifies the elegance and utility of geometric principles. By dissecting the toy into simpler shapes, applying specific formulas, and synthesizing the results, we arrive at a clear understanding of the toy’s surface area. This exercise not only reinforces our knowledge of geometry but also highlights its practical applications in analyzing and understanding the world around us. It underscores the significance of geometry in everyday life, demonstrating how mathematical concepts are integral to interpreting and appreciating the objects we encounter.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail

