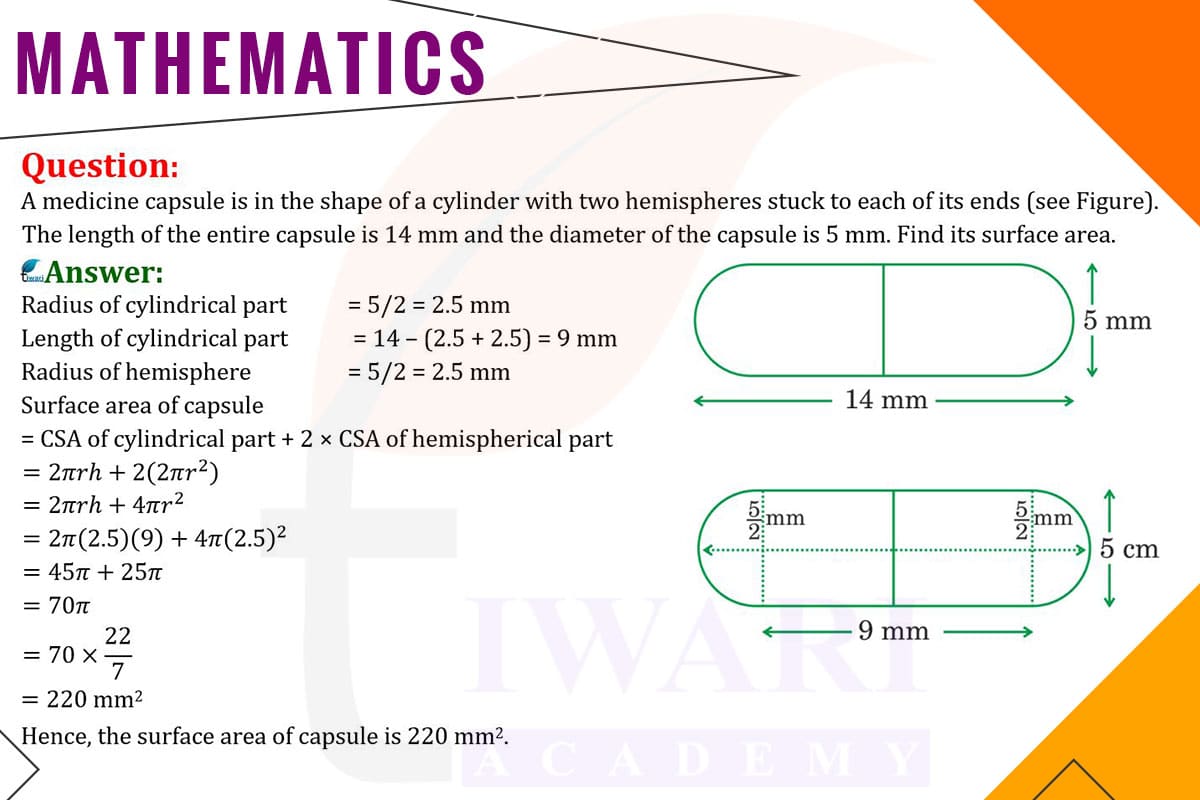
To find the surface area of a medicine capsule shaped like a cylinder with two hemispheres stuck to each end, we first calculate the surface areas of the cylinder and the hemispheres separately and then sum them up.
The total length of the capsule is 14 mm, and the diameter of the capsule (and hence the hemispheres) is 5 mm, giving a radius of 2.5 mm. The length of the cylindrical part is the total length minus the diameter of the two hemispheres, which is 14 mm − 2×2.5 mm = 9 mm.
The surface area of the cylinder (excluding the ends) is 2πrh, and the total surface area of the two hemispheres is 2 × 2πr² (since the surface area of a sphere is 4πr²). Substituting the values, we get 2π × 2.5 × 9 for the cylinder and 2 × 2π × 2.5² for the hemispheres.
Adding these, the total surface area of the capsule is 45π + 25π = 70π mm², which is approximately 219.9 mm².
Let’s discuss in detail
Geometric Analysis of a Medicine Capsule
The geometric analysis of a medicine capsule, which is a combination of cylindrical and hemispherical shapes, presents an interesting challenge. This capsule, with a total length of 14 mm and a diameter of 5 mm, is a perfect example of how different geometric shapes can be combined to form everyday objects. The task at hand is to calculate the surface area of this capsule, which requires understanding the properties of both the cylinder and the hemispheres. This type of problem is not just a mathematical exercise; it’s a practical application of geometry in the pharmaceutical world, demonstrating how geometry is integral to the design of functional and efficient objects.
Understanding the Dimensions of the Capsule
The first step in calculating the surface area is to understand the dimensions of the capsule. The total length of the capsule is 14 mm, and its diameter is 5 mm. These dimensions are crucial as they determine the size of both the cylindrical and hemispherical parts of the capsule. The diameter of 5 mm gives us a radius of 2.5 mm for the hemispheres. The length of the cylindrical part is the total length of the capsule minus the diameter of the two hemispheres, which is 14mm − 5mm = 9mm. This step is essential for breaking down the capsule into its constituent geometric shapes.
Calculating the Surface Area of the Cylinder
The next step is to calculate the surface area of the cylindrical part of the capsule. The formula for the lateral surface area of a cylinder is 2πrh, where r is the radius and h is the height (or length) of the cylinder. In our case, the radius is 2.5 mm, and the height is 9 mm. Substituting these values into the formula, we get the surface area of the cylindrical part of the capsule. This calculation is important as it represents the central part of the capsule’s surface.
Computing the Surface Area of the Hemispheres
After calculating the surface area of the cylinder, we focus on the hemispheres. The total surface area of the two hemispheres is equivalent to the surface area of a full sphere, which is 4πr². Since we have two hemispheres, each with a radius of 2.5 mm, we use this radius in the formula. This calculation gives us the combined surface area of the rounded ends of the capsule, which is a significant portion of the total surface area.
Total Surface Area of the Medicine Capsule
To find the total surface area of the medicine capsule, we add the surface areas of the cylinder and the hemispheres. The total surface area is the sum of the cylindrical surface area and the surface area of the two hemispheres. This step is crucial as it combines the individual calculations into a comprehensive total, reflecting the complete surface area that the capsule occupies. This total surface area is significant in practical applications, such as determining the amount of coating needed for the capsule.
The Practicality of Geometric Calculations
In conclusion, calculating the surface area of a medicine capsule shaped like a cylinder with hemispheres at both ends is a clear demonstration of the practical application of geometric principles. By dissecting the capsule into simpler shapes, applying specific formulas, and synthesizing the results, we arrive at a comprehensive understanding of the capsule’s surface area. This exercise not only reinforces our knowledge of geometry but also highlights its practical applications in fields like pharmaceuticals. It underscores the importance of geometry in designing and understanding objects used in everyday life, emphasizing the role of precise calculation and spatial reasoning.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail

