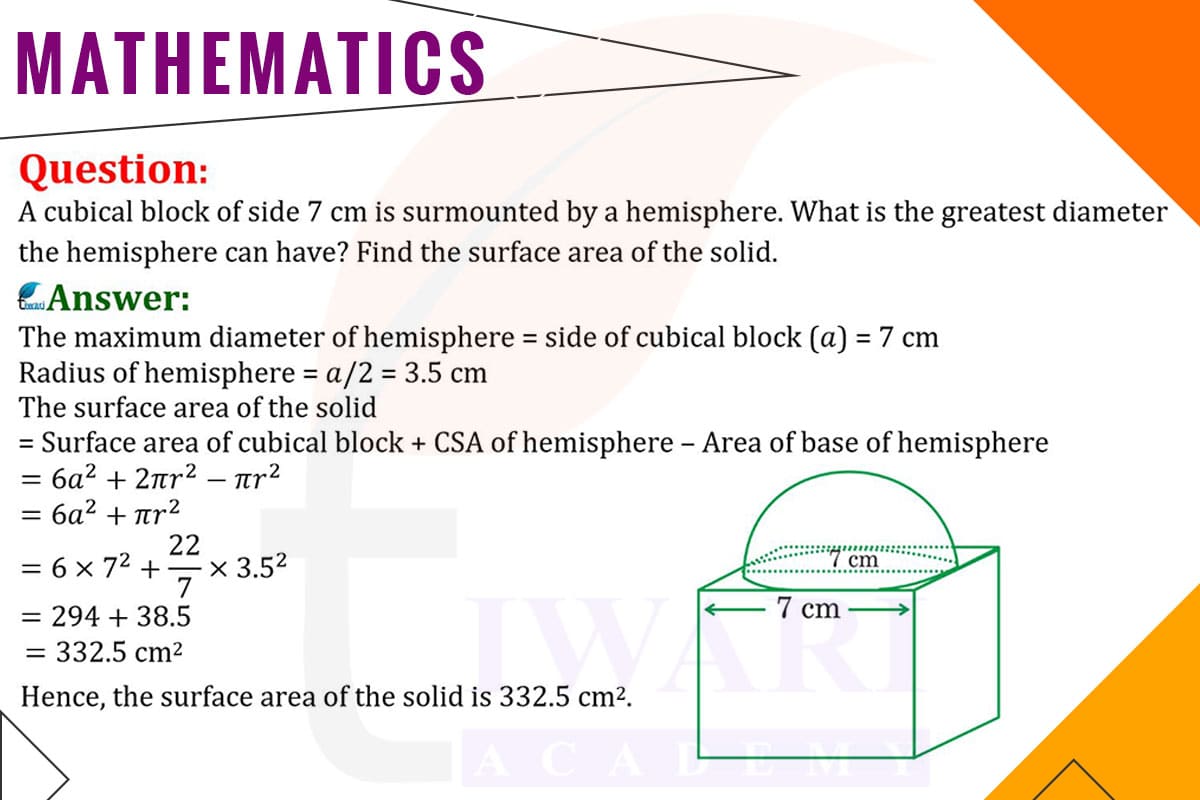
A cubical block of side 7 cm is surmounted by a hemisphere. What is the greatest diameter the hemisphere can have? Find the surface area of the solid.

Let’s discuss in detail

In the fascinating study of geometry, combining different shapes to form a new solid is a common practice. A perfect example of this is a cubical block surmounted by a hemisphere. This combination not only presents an interesting visual but also a challenging problem in terms of calculating its dimensions and surface area. The key to solving this problem lies in understanding the properties of both the cube and the hemisphere and how they interact when combined. This exercise is not just about applying formulas; it’s about visualizing how different geometric shapes can coalesce to form a unique structure.

The first step in our analysis is to understand the dimensions of the cube. In this case, we have a cube with each side measuring 7 cm. The cube, being a regular solid, has equal lengths on all sides, which simplifies our calculations. The significance of the cube’s dimensions becomes apparent when we consider the size of the hemisphere that can be placed on it. The cube’s uniformity in dimensions provides a base with a specific area on which the hemisphere can rest, thereby determining the maximum possible diameter of the hemisphere.

The greatest diameter that the hemisphere can have when placed on the cube is directly related to the dimensions of the cube. Since the cube has a side length of 7 cm, the maximum diameter of the hemisphere that can sit on it without overhanging is also 7 cm. This is because the diameter of the hemisphere must be equal to or less than the side of the cube to ensure a perfect fit. This constraint is crucial in maintaining the structural integrity and aesthetic of the combined solid.

Calculating the Surface Area of the Cube
To calculate the total surface area of the combined solid, we start with the cube. The surface area of a cube is calculated using the formula 6 × side². For our cube with a side of 7 cm, the surface area is 6×7² = 294 cm² . This area represents the total outer surface of the cube, which forms the base of our combined solid. It’s important to note that this calculation covers all six faces of the cube.

Next, we calculate the curved surface area of the hemisphere. The formula for this is 2πr², where r is the radius of the hemisphere. Since the diameter of the hemisphere is the same as the side of the cube (7 cm), the radius is half of this, which is 3.5 cm. Substituting this into the formula, we get 2 × π × 3.5² = 77 cm². This calculation gives us the area of the curved surface of the hemisphere, which is the visible part of the hemisphere when mounted on the cube.

Total Surface Area of the Combined Solid
Finally, to find the total surface area of the solid, we add the surface areas of the cube and the hemisphere. The total surface area is 294 cm² + 77 cm² = 371 cm². This total surface area represents the entire outer surface of the combined solid, encompassing both the cube and the hemisphere. This calculation is essential in understanding the extent of the surface that covers the entire structure, providing insights into the material requirements if the solid were to be constructed or covered.

In conclusion, the process of calculating the total surface area of a cubical block surmounted by a hemisphere demonstrates the beauty and precision of geometric calculations. By dissecting the problem into smaller parts, applying specific formulas, and combining the results, we arrive at a comprehensive understanding of the surface area of the combined solid. This exercise not only reinforces our knowledge of geometry but also showcases its practical applications in real-world scenarios. It highlights the importance of geometry in understanding and creating complex structures, emphasizing the interconnectedness of different geometric shapes.

Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail