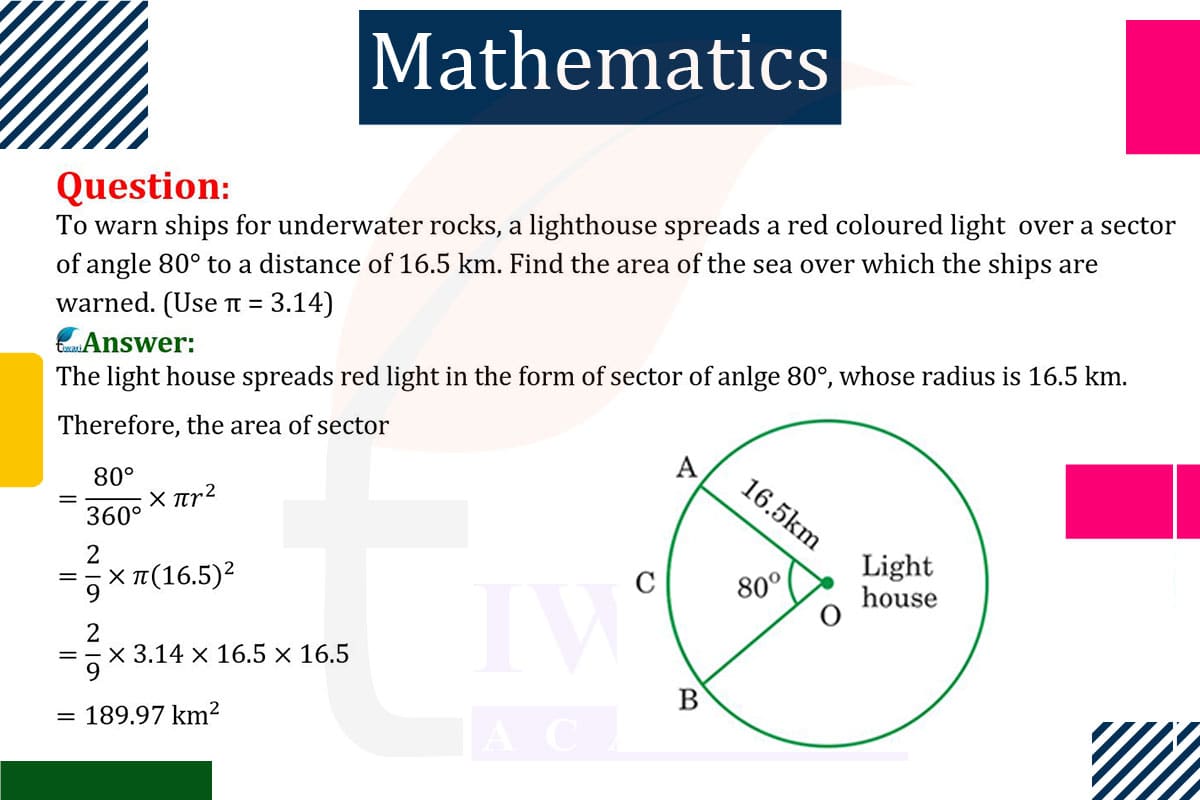
To find the area of the sea over which ships are warned by the lighthouse’s red light:
Area of the Sector: The lighthouse spreads light over a sector with an angle of 80°. The area of a sector is given by (θ/360)×πr², where θ is the angle in degrees and r is the radius (distance the light reaches).
Calculation: With θ = 80° and r = 16.5 km (16500 m for consistency in units), the area is (80/360) × 3.14 × 16500² square meters.
This calculation gives the area of the sea over which ships are warned by the lighthouse.
Let’s discuss in detail
Navigational Safety and Lighthouse Function
The role of a lighthouse in maritime navigation is crucial, especially in warning ships about underwater hazards like rocks. One of the key functions of a lighthouse is to project light over specific areas of the sea to alert ships. In this scenario, a lighthouse uses a red light to cover a sector of the sea with an angle of 80°, extending to a distance of 16.5 km. Calculating the area of the sea over which this warning light is spread not only demonstrates a practical application of geometry but also underscores the importance of lighthouses in maritime safety.
The Geometry of a Lighthouse’s Light
The light from a lighthouse can be modeled geometrically as a sector of a circle. The central angle of this sector, in this case, is 80°, and the radius is the distance the light reaches, which is 16.5 km. This sector represents the area of the sea illuminated by the lighthouse’s light. Understanding the geometry of this illuminated area is essential for maritime authorities to ensure the safety of ships navigating near hazardous areas.
Calculating the Area of Illumination
To calculate the area of the sea illuminated by the lighthouse, we use the formula for the area of a sector: (θ/360)×πr², where θ is the angle in degrees and r is the radius. In our case, θ = 80° and r = 16.5 km, which needs to be converted to meters (16500 m) for consistency in units. This formula will give us the area in square meters over which the ships are warned.
Practical Implications of the Calculation
The calculated area is significant for maritime navigation as it determines the extent of the warning zone around underwater rocks. Ships within this area need to be cautious and possibly alter their course to avoid potential hazards. The calculation also helps in positioning the lighthouse optimally to maximize the area of warning. This is crucial in areas with frequent shipping traffic or where underwater hazards are particularly dangerous.
The Role of Lighthouses in Modern Navigation
While modern navigation systems have advanced significantly, lighthouses still play a vital role in maritime safety. They serve as reliable, fail-safe warning systems, especially in regions where electronic systems might be unreliable or where ships might not have the most advanced navigational tools. The calculation of the illuminated area by a lighthouse is a testament to the blend of traditional methods and mathematical precision in ensuring maritime safety.
Geometry in Service of Maritime Safety
In conclusion, the calculation of the area over which a lighthouse warns ships of underwater rocks is a practical application of geometry that has direct implications for maritime safety. It illustrates how mathematical calculations are integral to navigational safety measures. This example highlights the enduring importance of lighthouses in maritime navigation and the role of geometry in safeguarding the lives of those at sea. It’s a perfect example of how traditional methods and modern calculations work together to ensure safety in our natural and technological world.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

