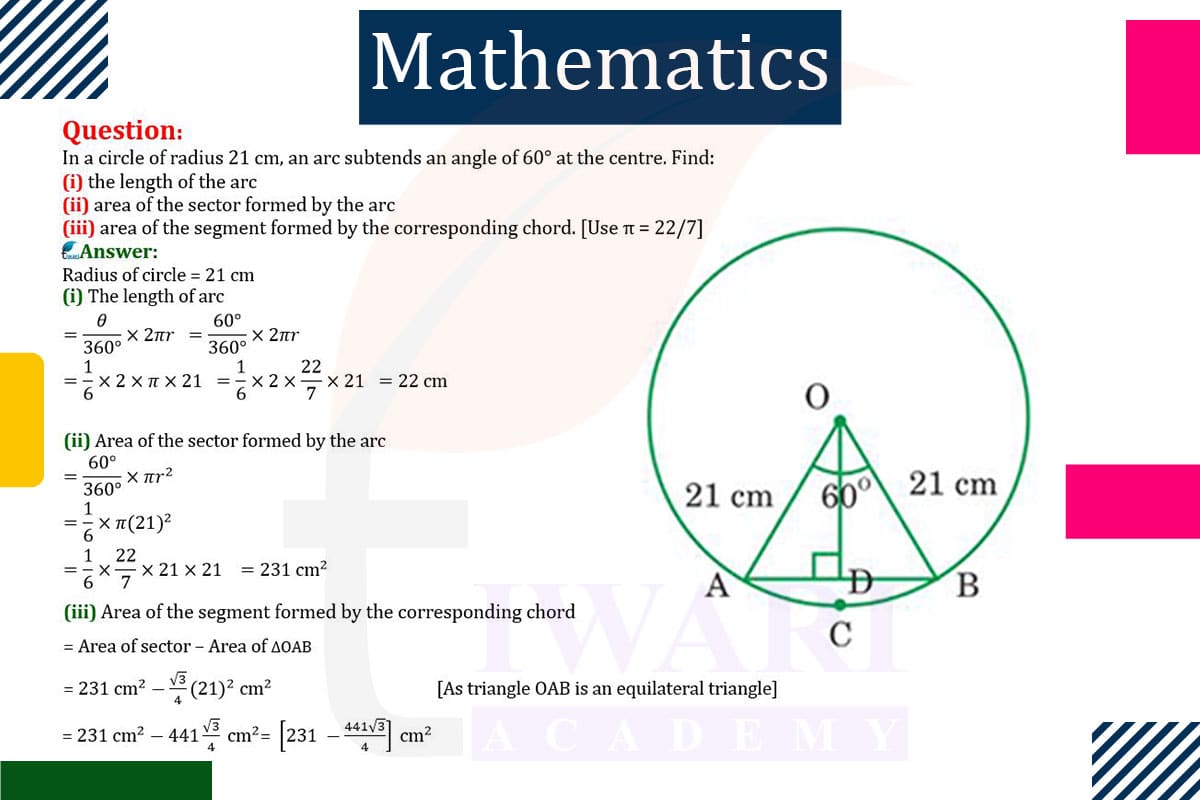
Length of the Arc: The length of an arc is given by Arc Length =
(θ/360)×2πr, where θ is the angle in degrees and r is the radius. For a 60° angle and radius 21 cm, it’s (60/360)×2π×21, which equals 22 cm.
Area of the Sector: The area of a sector is Area = (θ/360)×πr². Substituting θ = 60° and r=21 cm, the area is (60/360)×π×21², which equals 231π cm².
Area of the Segment: The area of the segment is the area of the sector minus the area of the triangle formed by the radii and the chord. The triangle’s area, for a 60° angle at the center, is (1/2)×r²sin(θ), which is (1/2)×21²×sin(60°). Subtracting this from the sector area gives the segment area.
Let’s discuss in detail
Introduction to Circle Geometry
In the realm of geometry, circles present a fascinating area of study, especially when it comes to understanding the relationships between their various components. In this specific problem, we explore a circle with a radius of 21 cm, focusing on an arc that subtends a 60° angle at the center. This scenario allows us to delve into three distinct but interrelated calculations: the length of the arc, the area of the sector formed by the arc, and the area of the segment formed by the corresponding chord. These calculations are not only fundamental in geometry but also have practical applications in various fields, including engineering, architecture, and design.
Calculating the Length of the Arc
The length of an arc in a circle is directly proportional to the angle it subtends at the center. The formula for calculating the arc length is Arc Length = (θ/360)×2πr, where θ is the angle in degrees and r is the radius of the circle. In our case, with a 60° angle and a radius of 21 cm, the calculation becomes a straightforward application of this formula. The result gives us the exact length of the arc, which is a crucial measure in various practical applications, such as designing circular tracks or machinery parts.
Determining the Area of the Sector
The area of a sector in a circle, like the arc length, depends on the angle the sector subtends at the center. The formula used is Area = (θ/360)×πr². By substituting the given values of θ = 60° and r = 21 cm into this formula, we can calculate the area of the sector. This area represents a portion of the circle’s total area, corresponding to the 60° angle. Understanding how to calculate sector areas is essential in fields like land surveying and architectural design, where precise measurements are necessary.
Computing the Area of the Segment
The area of the segment, which is the region bounded by the chord and the arc, can be a bit more complex to calculate. It involves finding the area of the sector and then subtracting the area of the triangular portion formed by the radii and the chord. For a 60° angle at the center, the triangle formed is an equilateral triangle, and its area can be calculated using trigonometric functions. The segment’s area is thus the difference between the sector’s area and the triangle’s area, providing a measure of the space enclosed by the arc and the chord.
Practical Implications of These Calculations
The practical implications of calculating arc lengths, sector areas, and segment areas are vast. These calculations are crucial in various engineering and architectural designs, where precise measurements are essential. For example, in constructing arches or designing gears, the length of an arc or the area of a sector might determine the materials needed or the strength of the structure. In landscaping and urban planning, these calculations help in designing circular plots or segments of land. The ability to calculate these measures accurately is therefore not just of academic interest but of real-world utility.
The Elegance of Circle Geometry
In conclusion, the study of circle geometry, as demonstrated in these calculations, reveals the elegance and complexity of mathematical relationships in simple shapes. Calculating the length of an arc, the area of a sector, and the area of a segment involves understanding and applying fundamental geometric principles. These calculations highlight the beauty of geometry in its ability to provide precise and practical solutions to real-world problems. The circle, with its simple shape, thus becomes a gateway to a deeper understanding of the world around us, both in its natural forms and in the structures we create.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

