To find the area of a sector of a circle with a radius of 6 cm and an angle of 60°, use the formula: Area = (θ/360) × π × r², where θ is the angle in degrees and r is the radius. Plugging in the values, we get: Area = (60/360) × π × 6². Simplifying this, Area = (1/6) × π × 36. Therefore, the area of the sector is 6π cm². This formula calculates the proportional area of the circle that the sector occupies, based on the fraction of the full 360° circle that the 60° sector represents.
Let’s discuss in detail
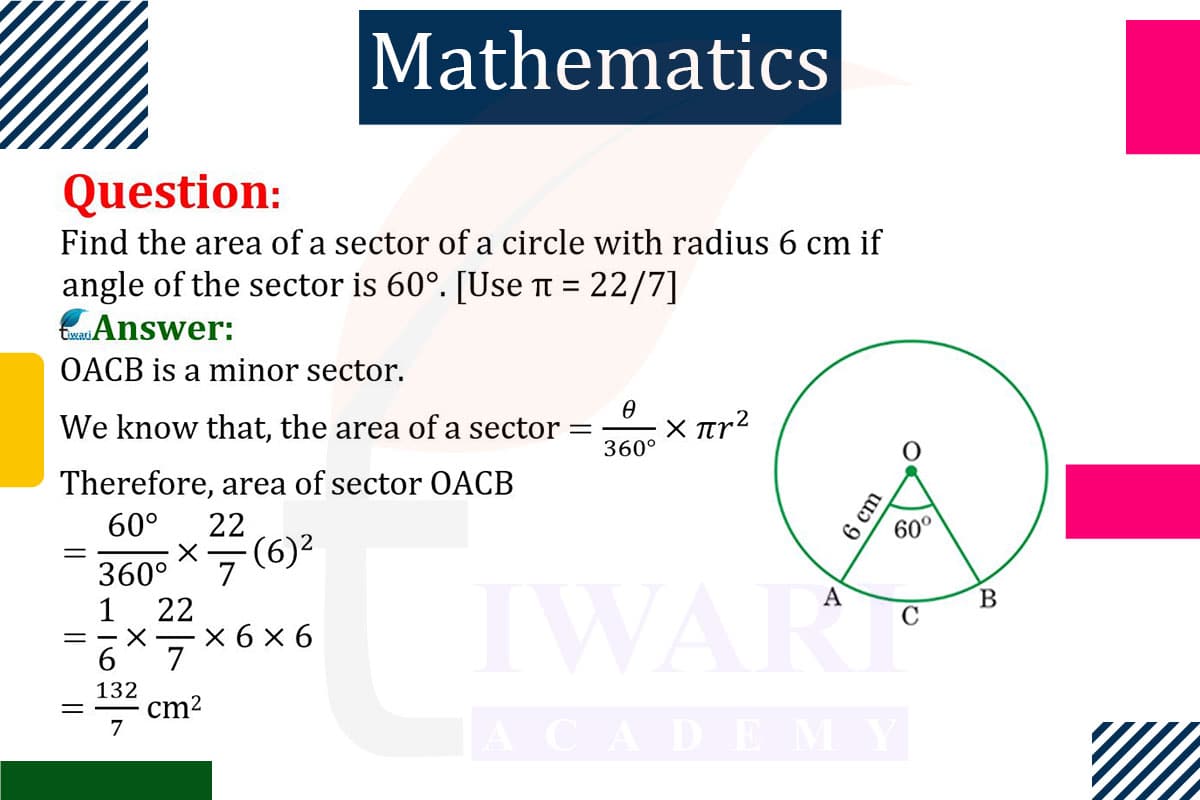
Introduction to Sector Area Calculation
Understanding the area of a sector of a circle is a fundamental concept in geometry, often applied in various fields including engineering, architecture, and design. A sector is a portion of a circle enclosed by two radii and an arc. The area of a sector is proportional to the angle it subtends at the center of the circle. To calculate this area, one must understand the relationship between the angle of the sector and the total area of the circle. The formula for the area of a circle is πr², where r is the radius. However, for a sector, this formula is adjusted to account for the fraction of the circle the sector represents.
Understanding the Formula
The formula for calculating the area of a sector is derived from the area of a circle. Since a circle is 360 degrees, the area of a sector depends on what fraction of 360 degrees its central angle represents. The formula is given as Area = (θ/360) × π × r², where θ is the central angle in degrees and r is the radius of the circle. This formula essentially calculates the area of the entire circle and then multiplies it by the fraction of the circle that the sector represents.
Calculating Sector Area with a 60° Angle
When calculating the area of a sector with a specific angle, such as 60°, it’s important to substitute the values accurately into the formula. For a circle with a radius of 6 cm and a sector angle of 60°, the calculation becomes straightforward. The angle of 60° is one-sixth of 360°, indicating that the sector occupies one-sixth of the total area of the circle. This understanding is crucial in simplifying the calculation process.
Step-by-Step Calculation
To calculate the area of the sector, first determine the area of the full circle using the formula πr². For a radius of 6 cm, the area of the circle is π × 6² = 36π cm². Since the sector’s angle is 60°, which is one-sixth of a full circle, the sector’s area is one-sixth of the circle’s total area. Therefore, the area of the sector is (1/6) × 36π = 6π cm². This step-by-step approach helps in understanding how the sector’s area relates to the circle’s total area.
Practical Implications
The calculation of a sector’s area has practical implications in various real-world scenarios. For instance, in architectural design, understanding the area of a sector can help in designing rounded elements or segments of a structure. In fields like engineering, it can assist in calculating material requirements for circular components. The concept is also used in everyday situations, such as determining the size of a slice of pizza or a pie, where each slice can be considered a sector of a circle.
Conclusion of Solutions
In conclusion, calculating the area of a sector of a circle requires understanding the relationship between the sector’s angle and the total area of the circle. By applying the formula (θ/360) × π × r², one can easily determine the area of a sector for any given radius and angle. This concept not only has academic significance in geometry but also practical applications in various professional and everyday contexts. Understanding and applying this formula is a key skill in geometry, providing a foundation for more complex geometrical calculations and applications.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

