Find the area of a quadrant of a circle whose circumference is 22 cm.

Updated on June 11, 2024
by Tiwari Academy

To find the area of a quadrant of a circle with a circumference of 22 cm, first determine the radius of the circle. The circumference C of a circle is given by C = 2πr, where r is the radius. Solving for r, we get r = C/(2π). Substituting C = 22 cm, r becomes approximately 3.5 cm. The area A of a circle is πr², so for our circle, A ≈ 3.14×(3.5)² cm². A quadrant is one-fourth of a circle, so its area is A/4, which is approximately 9.62 cm². This calculation shows how the circumference can be used to find the area of a specific section of a circle.

Find the area of a quadrant of a circle whose circumference is 22.

Let’s discuss in detail

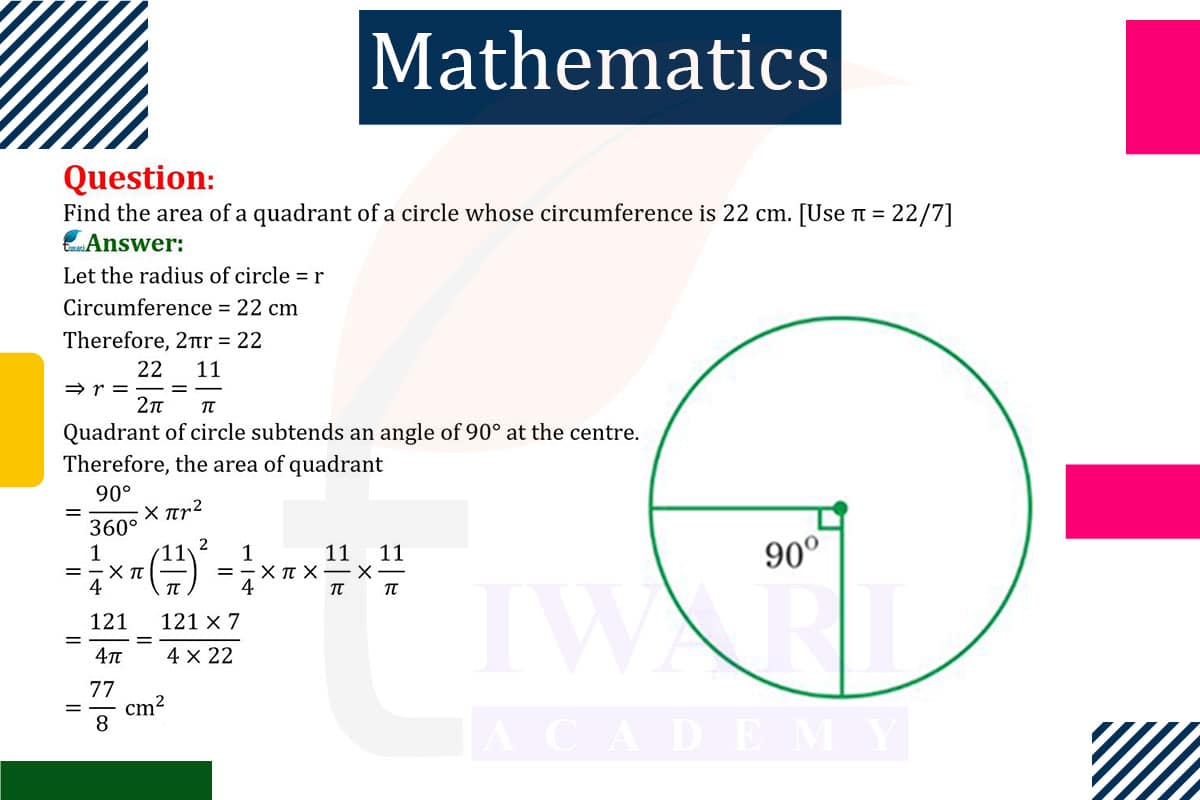
Quadrant Area Calculation

The concept of finding the area of a quadrant of a circle is a fundamental aspect of geometry, especially in understanding the properties of circles. A quadrant is one-fourth of a circle, making it a crucial shape in various mathematical and practical applications. To calculate the area of a quadrant, one must first understand the relationship between the circumference of the entire circle and its radius, as the area of any part of a circle is directly related to its radius. This calculation is not only important in theoretical mathematics but also has practical applications in fields like engineering, architecture, and design.

Understanding the Circle’s Circumference

The circumference of a circle is the total distance around its edge, which is directly proportional to its diameter. The standard formula to calculate the circumference is C = 2πr, where C is the circumference and r is the radius of the circle. The value of π (pi) is approximately 3.14, which is a constant representing the ratio of the circumference of any circle to its diameter. In our scenario, the given circumference of 22 cm will be the starting point to find the radius, which is essential for calculating the area of the quadrant.

Calculating the Radius from Circumference

To find the radius of the circle from its circumference, we rearrange the circumference formula to r = C/(2π). By substituting the given circumference (22 cm) into this formula, we can calculate the radius. This step is crucial because the radius is a key component in determining the area of the circle, and consequently, the area of the quadrant. The radius found from this calculation will be used in the next step to find the area of the entire circle.

Determining the Area of the Circle

Once the radius is known, the next step is to calculate the area of the entire circle using the formula A = πr². This formula is derived from the concept that the area of a circle is proportional to the square of its radius. By plugging in the radius value obtained from the circumference, we can calculate the total area of the circle. This total area is important as it forms the basis for determining the area of the quadrant, which is a fraction of the total area.

Calculating the Area of the Quadrant

A quadrant being one-fourth of a circle, its area is simply one-fourth of the total area of the circle. Therefore, the area of the quadrant is calculated by dividing the total area of the circle by four. This calculation is straightforward but requires accurate computation of the previous steps, particularly ensuring the correct radius is used. The result gives us the area of the quadrant, which is a significant measure in various practical and theoretical applications.

In conclusion, calculating the area of a quadrant of a circle involves finding the radius from the given circumference, determining the area of the whole circle, and then dividing this area by four to find the quadrant’s area. This process highlights the interconnectedness of different geometric properties of a circle. The ability to calculate the area of a quadrant has practical implications in numerous fields, including engineering, design, and everyday problem-solving. It demonstrates the practical utility of geometric principles in real-world scenarios, making it a valuable skill in both academic and professional contexts.

Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60°.

Find the area of a quadrant of a circle whose circumference is 22 cm.

The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes.

A chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding (i) minor segment (ii) major sector.

In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find: (i) the length of the arc (ii) area of the sector formed by the arc (iii) area of the segment formed by the corresponding chord.

A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle.

A chord of a circle of radius 12 cm subtends an angle of 120° at the centre. Find the area of the corresponding segment of the circle.

A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope. Find (i) the area of that part of the field in which the horse can graze. (ii) the increase in the grazing area if the rope were 10 m long instead of 5 m.

A brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire is also used in making 5 diameters which divide the circle into 10 equal sectors. Find (i) the total length of the silver wire required. (ii) the area of each sector of the brooch.

An umbrella has 8 ribs which are equally spaced. Assuming umbrella to be a flat circle of radius 45 cm, find the area between the two consecutive ribs of the umbrella.

A car has two wipers which do not overlap. Each wiper has a blade of length 25 cm sweeping through an angle of 115°. Find the total area cleaned at each sweep of the blades.