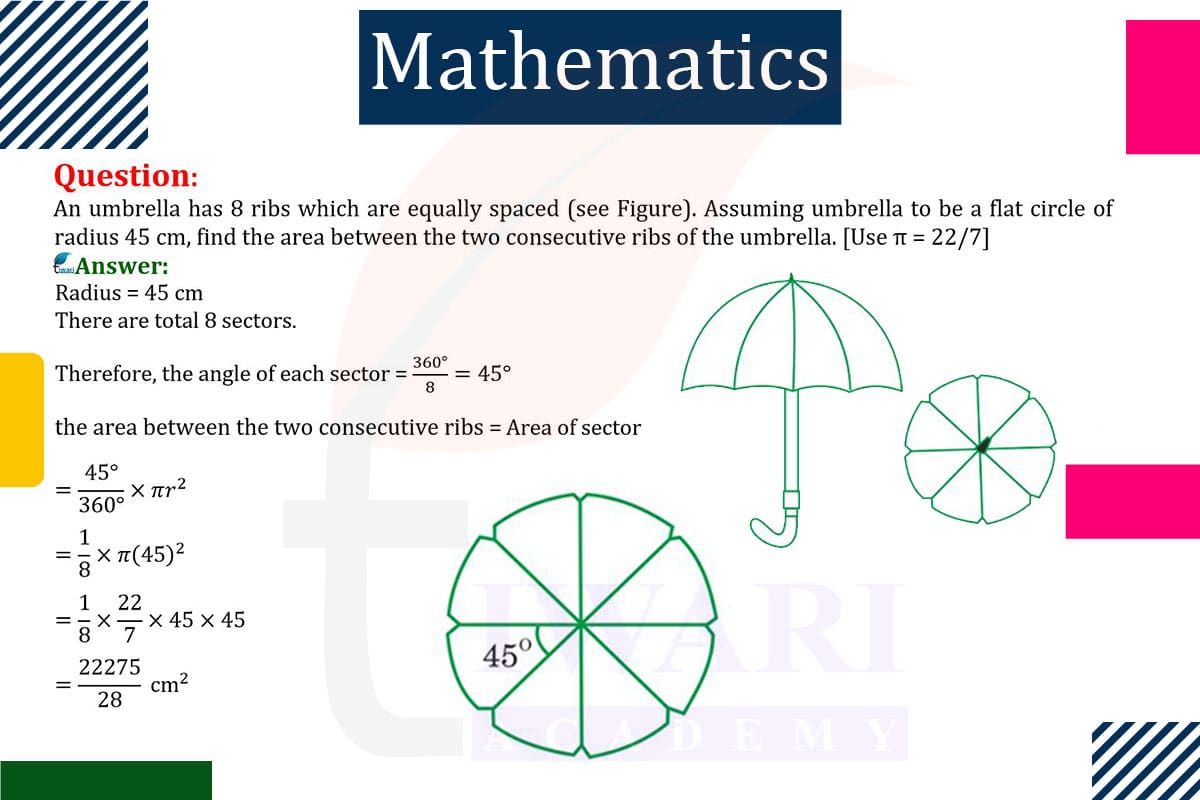
To find the area between two consecutive ribs of an umbrella assumed to be a flat circle with a radius of 45 cm:
Total Area of the Umbrella: The total area of the umbrella, which is a circle, is calculated using the formula Area = πr². With r = 45 cm, the total area is π×45² cm².
Area Between Two Ribs: Since there are 8 ribs, the umbrella is divided into 8 equal sectors. The area of each sector is (1/8) of the total area. Therefore, the area between two consecutive ribs is (1/8)×π×45² cm².
This calculation gives the area of the sector between any two consecutive ribs of the umbrella.
Let’s discuss in detail
Geometric Application in Everyday Objects
The problem of calculating the area between two consecutive ribs of an umbrella is a fascinating example of how geometry applies to everyday objects. An umbrella, typically seen as a practical item for protection against rain or sun, also presents an interesting geometric shape. Assuming the umbrella to be a flat circle with a radius of 45 cm, and knowing that it has 8 equally spaced ribs, we can apply principles of circle geometry to find the area of the sector between any two ribs. This exercise not only demonstrates the practical application of geometry but also enriches our understanding of the shapes and patterns in everyday life.
Understanding the Geometry of an Umbrella
An umbrella can be modeled as a circle when opened and viewed from above. The ribs of the umbrella act like radii of this circle, dividing it into equal segments. In this case, with 8 ribs, the umbrella is divided into 8 equal sectors. Each sector represents a slice of the circle, bounded by two ribs (radii) and a part of the circle’s circumference. The challenge is to calculate the area of one of these sectors, which represents the area between two consecutive ribs of the umbrella.
Calculating the Total Area of the Umbrella
The first step in solving this problem is to calculate the total area of the umbrella, which is a circle. The area of a circle is given by the formula A = πr², where r is the radius. For an umbrella with a radius of 45 cm, the total area is π×45² square centimeters. This calculation gives us the entire surface area covered by the umbrella when it is fully opened.
Dividing the Circle into Equal Sectors
Since the umbrella has 8 ribs, it is divided into 8 equal sectors. Each sector is a slice of the circle that has an angle of (360°/8) = 45° at the center. The area of each sector is a fraction of the total area of the circle. Specifically, each sector occupies (1/8)th of the total area of the umbrella. This fraction is key to determining the area between two consecutive ribs.
Finding the Area Between Two Consecutive Ribs
To find the area between two consecutive ribs, we calculate the area of one of these 8 sectors. Since each sector is (1/8)th of the total circle, the area of one sector is (1/8)×π×45² square centimeters. This formula gives us the exact area of the umbrella that is covered between any two of its ribs. It is a straightforward application of circle geometry, dividing the total area by the number of sectors.
Geometry in Practical Design
In conclusion, this problem illustrates the practical application of geometric principles in the design and functionality of everyday objects like umbrellas. By understanding the geometry of a circle and its division into equal sectors, we can calculate specific areas such as the space between the ribs of an umbrella. This exercise not only provides insight into the mathematical aspects of design but also highlights the omnipresence of geometry in our daily lives. It is a perfect example of how mathematics is not just an abstract concept but a tool that shapes the world around us.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

