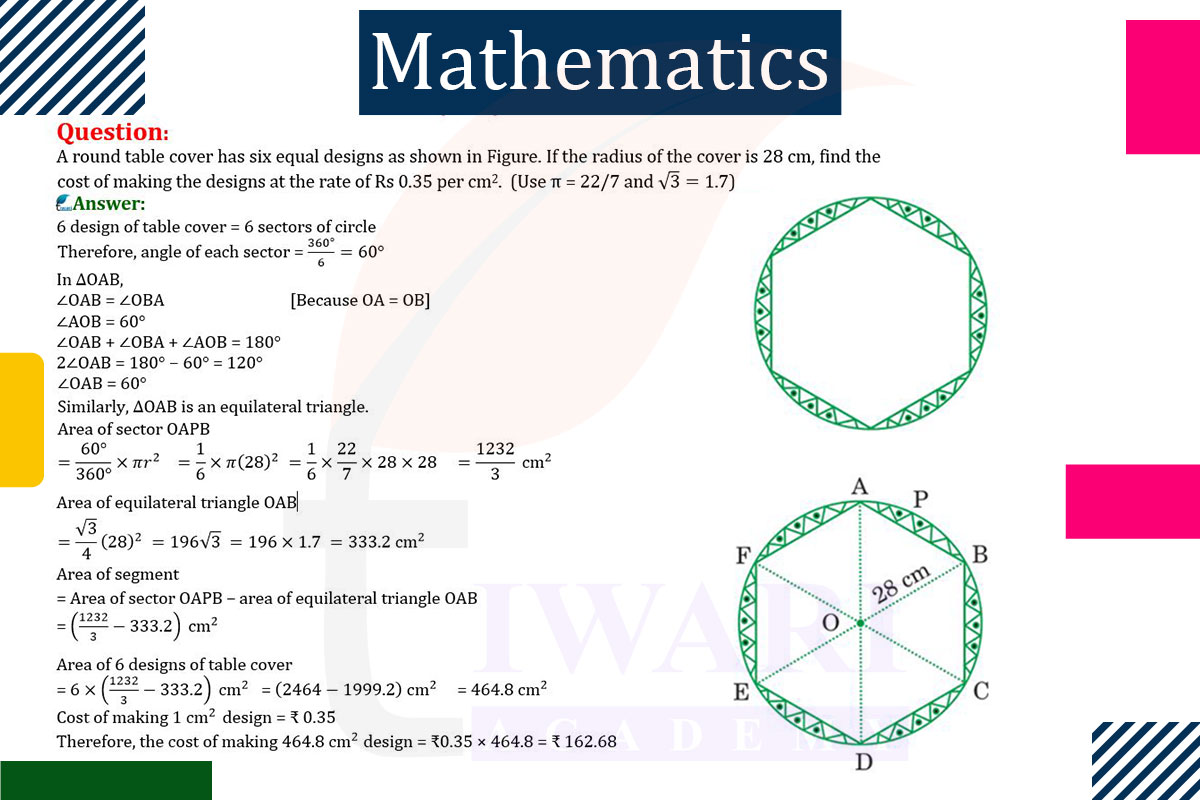
To find the cost of making the designs on a round table cover with six equal designs:
Area of One Design: The table cover is divided into six equal parts, each part being a sector of the circle. The area of a sector is given by (θ/360)×πr², where θ is the angle of the sector and r is the radius. Since there are six designs, each sector has an angle of 360°/6 = 60°. With r = 28 cm, the area of one design is (60/360)×π×28² cm².
Total Area for Six Designs: Multiply the area of one design by six.
Cost Calculation: Multiply the total area by the cost per cm², ₹0.35.
The final calculation gives the total cost of making the designs on the table cover.
Let’s discuss in detail
Crafting Designs on a Round Table Cover
The task of calculating the cost of making designs on a round table cover is a practical application of geometry in the realm of textile design and craftsmanship. In this scenario, a round table cover, with a radius of 28 cm, features six equal designs. Each design is a sector of the circle that forms the table cover. The cost of crafting these designs is determined by the area they cover and the rate charged per square centimeter. This problem not only involves geometric calculations but also reflects the intersection of art, design, and mathematics.
Geometric Division of the Table Cover
The round table cover is divided into six equal parts for the designs. This division implies that each design occupies a sector of the circle. The angle of each sector in a circle is determined by dividing the total angle of the circle (360 degrees) by the number of sectors. Since there are six designs, each sector has an angle of (360°/6) = 60°. This division is crucial for calculating the area that each design will occupy on the table cover.
Calculating the Area of a Single Design
To calculate the area of one design, we use the formula for the area of a sector: (θ/360)×πr², where θ is the angle of the sector, and r is the radius of the circle. With a radius of 28 cm and a sector angle of 60°, the area of one design is (60/360)×π×28² square centimeters. This calculation gives us the area occupied by one of the six designs on the table cover.
Total Area Covered by All Designs
After calculating the area of one design, the next step is to determine the total area covered by all six designs. This is simply six times the area of one design, as all designs are equal. The total area for the six designs is 6×(60/360)×π×28² square centimeters. This total area is essential for calculating the overall cost of making the designs on the table cover.
Cost Calculation for the Designs
The cost of making the designs is calculated by multiplying the total area covered by the designs by the rate charged per square centimeter. Given the rate is ₹0.35 per square centimeter, the total cost is the product of this rate and the total area calculated in the previous step. This step translates the geometric calculation into a monetary value, providing the total cost for crafting the designs on the table cover.
The Intersection of Geometry and Craftsmanship
In conclusion, this problem illustrates the practical application of geometry in textile design and craftsmanship. By calculating the area of each design and subsequently the total area for all designs, we can determine the cost of creating these patterns on a round table cover. This exercise not only highlights the importance of geometry in design and manufacturing but also shows how mathematical concepts are integral in various aspects of practical life, including crafting and economics. It’s a fascinating example of how geometry aids in bridging creativity with practicality.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

