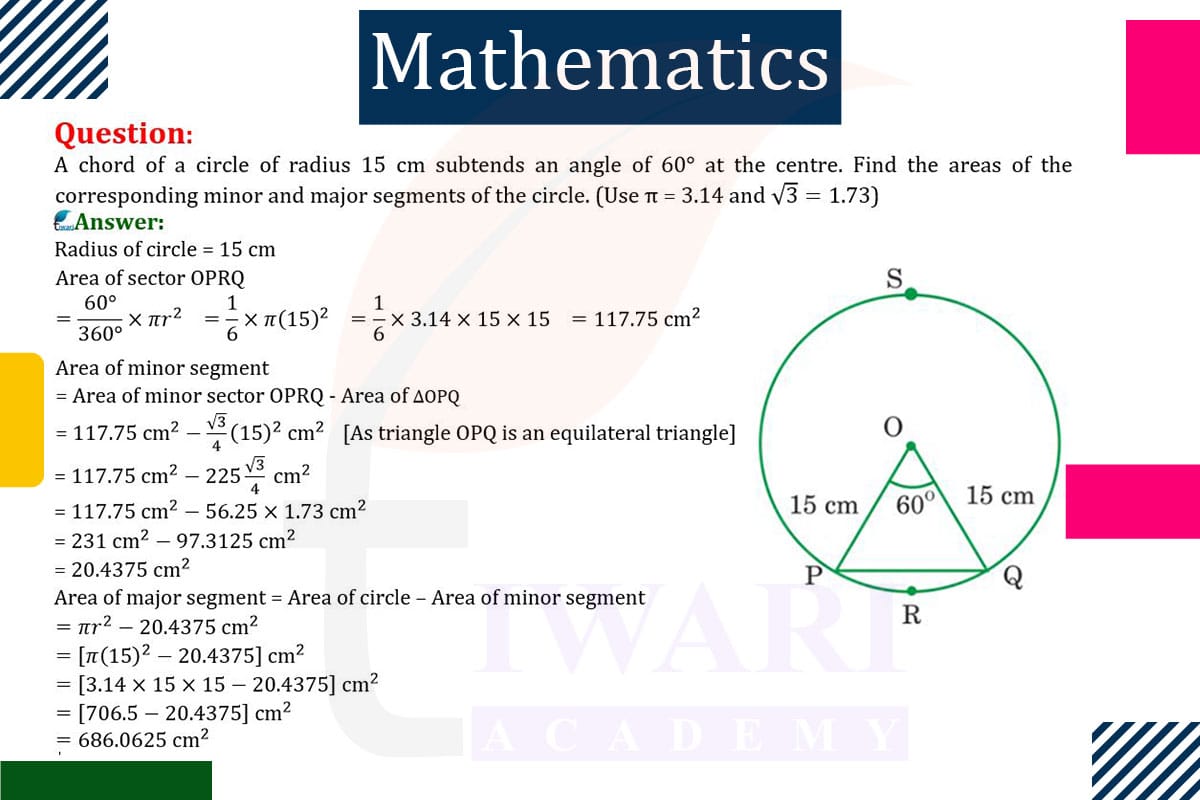
A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle.

Let’s discuss in detail

In the study of geometry, the calculation of areas within a circle, such as segments and sectors, is a fundamental aspect that finds application in various fields. In this specific problem, we focus on a circle with a radius of 15 cm, where a chord subtends a 60° angle at the center. This scenario creates two distinct areas within the circle: the minor segment and the major segment. Calculating the areas of these segments involves understanding the relationships between the circle’s radius, the central angle, and the properties of the segments. These calculations are not only important for academic purposes but also have practical applications in fields like engineering, architecture, and design.

The first step in solving this problem is to calculate the area of the minor segment. This involves two main calculations: finding the area of the sector formed by the 60° angle and then subtracting the area of the triangle formed by the chord and the radii. The sector’s area is calculated using the formula Area(sector) = (θ/360)×πr², where θ is the angle and r is the radius. For a 60° angle and a radius of 15 cm, this calculation yields the sector’s area. The triangle, being equilateral due to the 60° angle, has its area calculated using the formula for an equilateral triangle.

The area of the equilateral triangle formed by the radii and the chord is a crucial component in calculating the minor segment’s area. The formula for the area of an equilateral triangle is Area(triangle) = (√3/4)×side². In this case, the side of the triangle is equal to the radius of the circle, 15 cm. By substituting this value and using √3 = 1.73, we can determine the area of the triangle. This area is then subtracted from the sector’s area to find the area of the minor segment.

Computing the Area of the Major Segment
After calculating the minor segment’s area, the next step is to determine the area of the major segment. The major segment is essentially the remaining part of the circle after the minor segment is removed. Therefore, its area is calculated by subtracting the minor segment’s area from the total area of the circle. The total area of the circle is given by πr², and by using the radius of 15 cm and π = 3.14, we can find the circle’s total area. The difference between this total area and the minor segment’s area gives us the area of the major segment.

The calculation of areas of segments in a circle has practical implications in various real-world scenarios. For instance, in architectural design and engineering, understanding the area of a circular segment can be crucial for material estimation and structural design. In fields like astronomy and physics, these calculations assist in modeling and understanding celestial bodies and their paths. The ability to accurately calculate these areas is therefore not just of academic interest but also of significant practical importance in various professional fields.

In conclusion, the problem of calculating the areas of the minor and major segments of a circle with a given radius and chord angle demonstrates the relevance and application of geometric principles. These calculations highlight the beauty and complexity of geometry in solving practical problems. The circle, with its simple shape, offers a wide range of study and application, from basic geometric calculations to complex real-world problem-solving. This example serves as a testament to the importance of geometry in understanding and interpreting the world around us, both in its natural forms and in the structures we create.

Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail