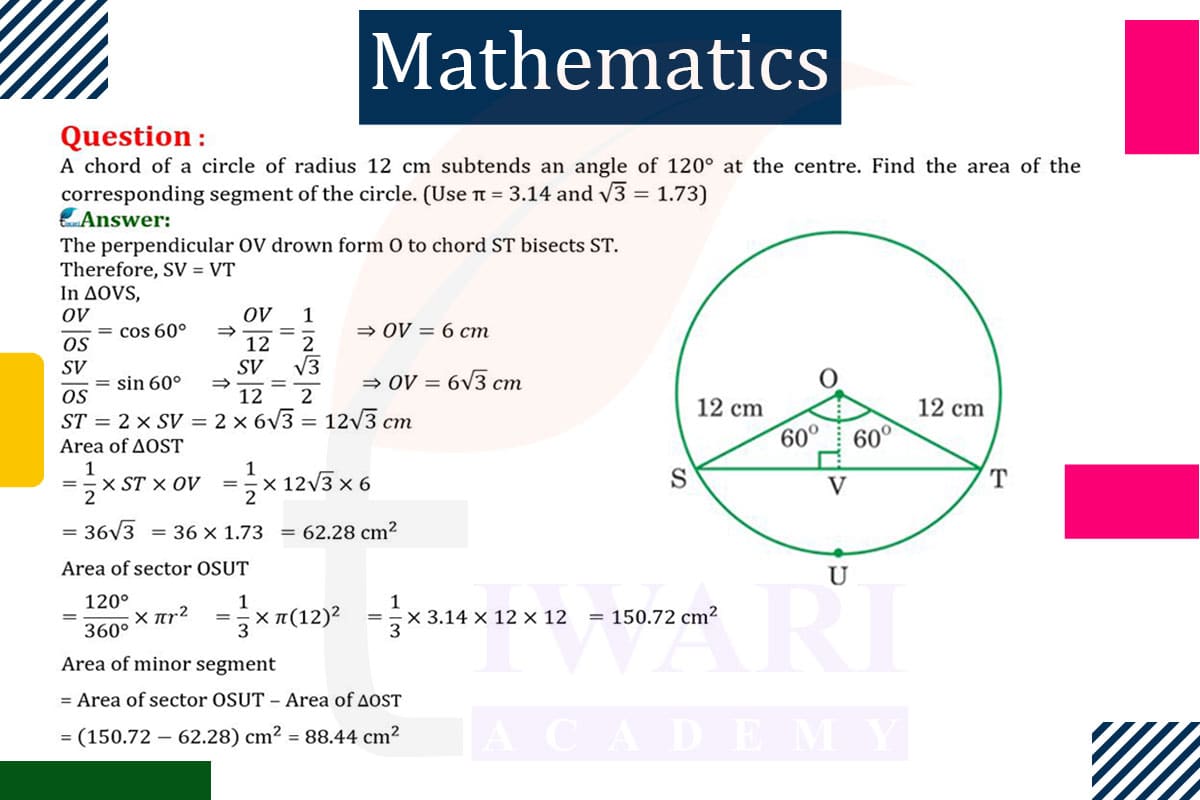
To find the area of the segment of a circle with a radius of 12 cm, where the chord subtends a 120° angle at the center:
Area of the Sector: First, calculate the sector’s area using Area(sector) = (θ/360)×πr². With θ = 120°, r = 12 cm, and π = 3.14, this gives (120/360)×3.14×12² ≈ 150.72 cm².
Area of the Triangle: The triangle formed by the radii and the chord is an isosceles triangle. Its area is (1/2)×r²sin(θ), which is (1/2)×12²×sin(120°). Using sin(120°) = sin(60°) = √3/2 and √3 = 1.73, the triangle’s area is approximately 112.32 cm².
Area of the Segment: The segment’s area is the sector’s area minus the triangle’s area, which is 150.72−112.32≈38.4 cm².
This calculation demonstrates how to find the area of a segment in a circle using basic principles of geometry.
Let’s discuss in detail
Circle Geometry and Segment Area Calculation
In the realm of geometry, the circle is a fundamental shape that offers a wide range of interesting problems, particularly when it comes to calculating areas of specific segments. In this scenario, we are presented with a circle of radius 12 cm, where a chord subtends a 120° angle at the center. This setup leads us to the challenge of calculating the area of the corresponding segment. This type of problem is not only a classic example of applying geometric principles but also has practical applications in various fields such as engineering, architecture, and design, where precise calculations of curved surfaces are essential.
Understanding the Circle and the Segment
The circle in question has a radius of 12 cm, and the segment we are focusing on is defined by a chord that creates a 120° angle at the center of the circle. This segment is a portion of the circle bounded by the arc and the chord. To calculate its area, we need to understand the relationship between the circle’s radius, the central angle, and the properties of the segment. The segment’s area is essentially the difference between the area of the sector (formed by the central angle) and the area of the triangle (formed by the radii and the chord).
Calculating the Area of the Sector
The first step in determining the segment’s area is to calculate the area of the sector formed by the 120° angle. The formula for the area of a sector is Area(sector) = (θ/360)×πr², where θ is the central angle in degrees and r is the radius. By substituting θ = 120°, r = 12 cm, and π = 3.14, we can compute the sector’s area. This area represents a larger portion of the circle from which we will subtract the area of the triangle to get the segment’s area.
Determining the Area of the Triangle
The next step involves calculating the area of the triangle formed by the two radii and the chord. This triangle, given the 120° angle at the center, is an isosceles triangle. The area of such a triangle can be calculated using the formula (1/2)×r²sin(θ). Using the given values, r = 12 cm and sin(120°) = sin(60°) = √3/2 with √3 = 1.73, we find the area of the triangle. This triangular area is a part of the sector that does not belong to the segment.
Computing the Area of the Segment
After calculating both the sector’s and the triangle’s areas, the area of the segment is found by subtracting the triangle’s area from the sector’s area. This difference gives us the area of the segment, which is the region enclosed by the chord and the arc. This calculation requires precision and a good understanding of the geometric properties of circles and triangles. The resulting area of the segment is a key measure in various practical applications, such as designing arches or calculating land areas in circular plots.
Practical Applications and Geometric Beauty
In conclusion, the calculation of the area of a segment in a circle demonstrates the practical application of geometric principles. This problem not only reinforces the understanding of circle geometry but also illustrates the application of these concepts in solving real-world problems. The ability to calculate segment areas is crucial in many professional fields, including architecture, engineering, and design. This example serves as a testament to the importance and beauty of geometry in understanding and interpreting the natural and constructed world around us.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

